Abstract
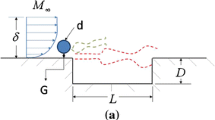
The interaction of turbulent flows with the external structure of ground vehicles generates uncomfortable noise and a lot of attention is devoted to find new mechanisms for its suppression. The present work is concerned with open cavity flows, very often found in the automotive industry. A three-dimensional rectangular very wide open cavity with aspect ratio \(L/D=4\) at Reynolds number \(Re_D=5000\) and Mach number \(M=0.1\) is considered. The passive control technique is based on eight different geometrical modifications: the length of the cavity, the radius of the trailing, leading and bottom edges and the difference in heights between the left and right wall of the cavity. Wall-resolved Large Eddy Simulations (LES) are used to obtain the flow fields and a post-process based on Curle’s analogy is applied to evaluate the acoustic radiation and the effectiveness of the control mechanisms. The results show that the modifications on the trailing edge are the most effective to control the flow. They allow to reduce the pressure fluctuations produced by the recirculation confined inside the cavity and the abrupt ejection of the flow at the trailing edge. As a consequence, the overall sound pressure level can be decreased up to 9 dB.

















Similar content being viewed by others
Abbreviations
- \(a_0\) :
-
= Speed of sound
- \(C_D\) :
-
= Cavity drag coefficient, \(\frac{F_D}{\frac{1}{2}\rho _{\infty }U_{\infty }^2DW}\)
- D :
-
= Cavity depth
- f :
-
= Fundamental frequency
- \(F_D\) :
-
= Force contribution from the cavity walls
- H :
-
= Shape factor, \(H=\frac{\delta ^*}{\theta }\)
- L :
-
= Cavity length
- l :
-
= Unitary vector pointing from source point to observation point.
- OASPL:
-
= Overall averaged sound pressure level, \(20log_{10}\left( \frac{p_{rms}'}{p_{ref}'}\right)\)
- M :
-
= Mach number, \(\frac{U_{\infty }}{a_0}\)
- p :
-
= Non-dimensional pressure, \(\frac{p_d}{\rho _{\infty }U_{\infty }^2}\)
- \(p_d\) :
-
= Dimensional pressure
- \(p_{rms}'\) :
-
= Root mean square of the acoustic pressure
- \(p_{ref}'\) :
-
= Reference acoustic pressure, \(\sqrt{\rho _{\infty } a_0\times 10^{-12}}\)
- n :
-
= Surface normal vector pointing to the surface
- \({\mathcal {Q}}\) :
-
= Second invariant of the velocity gradient tensor, \(-\frac{1}{2}\frac{\partial u_i}{\partial x_j}\frac{\partial u_j}{\partial x_i}\)
- r :
-
= Distance between an observer position and a source point
- \(R_{ii}\) :
-
= Autocorrelation coefficient
- \(Re_{D,L,\theta }\) :
-
= Reynolds number, \(\frac{U_{\infty }D}{\nu }\), \(\frac{U_{\infty }L}{\nu }\), \(\frac{U_{\infty }\theta }{\nu }\)
- \(St_L\) :
-
= Strouhal number based on L, \(St_L = \frac{f L}{U_{\infty }}\)
- s :
-
= Distance from the leading edge along the wall
- t :
-
= Time
- \({\tilde{t}}\) :
-
= Retarded time, \(t-r/a_0\)
- TU :
-
= Time units, \(\frac{t U_{\infty }}{D}\)
- U :
-
= Non-dimensional velocity, \(\frac{U_d}{U_{\infty }}\)
- \(U_d\) :
-
= Dimensional velocity
- \(u^*\) :
-
= Friction velocity, \(\sqrt{\frac{\tau _w}{\rho }}\)
- \(U_{\infty }\) :
-
= Freestream velocity
- W :
-
= Cavity width
- x :
-
= Streamwise coordinate
- y :
-
= Cross-stream coordinate
- \(y_n\) :
-
= Wall normal coordinate
- \(y^+\) :
-
= Dimensionless wall-normal distance, \(y^+=\frac{u^*y_n}{\nu }\)
- z :
-
= Spanwise coordinate
- \(\alpha\) :
-
= Counterclockwise angle taken from the downstream wall of the cavity
- \(\delta ^*\) :
-
= Displacement thickness, \(\int _0^{\delta _{99}}\left( 1-\frac{\rho (y_n)u(y_n)}{\rho _{\infty }U_{\infty }}\right) {\mathrm {d}}y_n\)
- \(\delta _{ij}\) :
-
= Kronecker delta
- \(\varDelta x^+\) :
-
= Wall spacing in the x-axis, \(\varDelta x^+=\frac{u^*\varDelta x}{\nu }\)
- \(\varDelta z^+\) :
-
= Wall spacing in the z-axis, \(\varDelta z^+=\frac{u^*\varDelta z}{\nu }\)
- \(\theta\) :
-
= Momentum thickness, \(\int _0^{\delta _{99}}\frac{\rho (y_n)u(y_n)}{\rho _{\infty }U_{\infty }}\left( 1-\frac{u(y_n)}{U_{\infty }}\right) {\mathrm {d}}y_n\)
- \(\mu\) :
-
= Dynamic viscosity
- \(\nu\) :
-
= Kinematic viscosity
- \(\rho\) :
-
= Density
- \(\rho _{\infty }\) :
-
= Freestream density
- \(\tau _W\) :
-
= Wall shear stress, \(\mu \left( \frac{\partial u }{\partial y }\right) _{y=0}\)
References
Capuano, F., Coppola, G., Rández, L., de Luca, L.: Explicit Runge-Kutta schemes for incompressible flow with improved energy-conservation properties. J. Comput. Phys. 328, 86–94 (2017)
Cetin, M.O., Koh, S.-R., Meinke, M., Schröder, W.: Numerical analysis of the impact of the interior nozzle geometry on low Mach number jet acoustics. Flow Turbul. Combust. (2016). https://doi.org/10.1007/s10494-016-9764-z
Charnyi, S., Heister, T., Olshanskii, M.A., Rebholz, L.G.: On conservation laws of Navier-Stokes Galerkin discretizations. J. Comput. Phys. 337, 289–308 (2017)
Codina, R.: Pressure stability in fractional step finite element methods for incompressible flows. J. Comput. Phys. 170(1), 112–140 (2001)
Colonius, T., Basu, A., Rowley, C.: Numerical investigation of the flow past a cavity. In: Proceedings of the 5th AIAA/CEAS Aeroacoustics Conference and Exhibit 1999, p. 1912 (1999)
Curle, N.: The influence of solid boundaries upon aerodynamic sound. In: Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 1955. The Royal Society 505–514 (1955)
Erbig, L., Maihöfer, M.: A hybrid RANS/LES for automotive gap noise simulations. In: Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, p. 2445 (2019)
Eriksson, C., Pershagen, G., Nilsson, M.: Biological mechanisms related to cardiovascular and metabolic effects by environmental noise. World Health Organization (2018)
Fabris, D., Williams, D.: Experimental Measurements of cavity and shear layer response to unsteady bleed forcing. In: Proceedings of the 37th Aerospace Sciences Meeting and Exhibit, p. 605 (1999)
Farassat, F., Myers, M.: Extension of Kirchhoff’s formula to radiation from moving surfaces. J. Sound Vib. 123(3), 451–460 (1988)
Fröhlich, J., Mellen, C.P., Rodi, W., Temmerman, L., Leschziner, M.A.: Highly resolved large-eddy simulation of separated flow in a channel with streamwise periodic constrictions. J. Fluid Mech. 526, 19–66 (2005)
Gharib, M., Roshko, A.: The effect of flow oscillations on cavity drag. J. Fluid Mech. 177, 501–530 (1987)
Hao, J., Wang, M., Ji, M., Wang, K.: Flow noise induced by small gaps in low-Mach-number turbulent boundary layers. Phys. Fluids 25(11), 110821 (2013)
Hartmann, M., Ocker, J., Lemke, T., Mutzke, A., Schwarz, V., Tokuno, H., Toppinga, R., Unterlechner, P., Wickern, G.: Wind noise caused by the side-mirror and a-pillar of a generic vehicle model. In: Proceedings of the 18th AIAA/CEAS Aeroacoustics Conference (33rd AIAA Aeroacoustics Conference), p. 2205 (2012)
Hsu, J., Ahuja, K.: Cavity noise control using Helmholtz resonators. In: Aeroacoustics Conference, p. 1675 (1996)
Jofre, L., Lehmkuhl, O., Ventosa, J., Trias, F.X., Oliva, A.: Conservation properties of unstructured finite-volume mesh schemes for the Navier-Stokes equations. Numer. Heat Trans. B Fundam. 65(1), 53–79 (2014)
Khalighi, B., Snegirev, A., Shinder, J., Lupuleac, S., Chen, K.-H.: Simulations of flow and noise generated by automobile outside rear-view mirrors. Int. J. Aeroacoust. 11(1), 137–156 (2012)
Kirchhoff, G.: Zur theorie der lichtstrahlen. Ann. Phys. 254(4), 663–695 (1883)
Knowles, K., Khanal, B., Bray, D., Geraldes, P.: Passive control of cavity instabilities and noise. In: Proceedings of the 27th International congress of the aeronautical sciences (2010)
Lai, H., Luo, K.H.: A conceptual study of cavity aeroacoustics control using porous media inserts. Flow Turbul. Combust. 80(3), 375–391 (2008)
Lamp, A.M., Chokani, N.: Computation of cavity flows with suppression using jet blowing. J. Aircraft 34(4), 545–551 (1997)
Langtry, R., Larssen, J., Winkler, C., Dorgan, A., Mani, M.: DDES and acoustic prediction of rudimentary landing gear experiment using unstructured finite volume methods. Flow Turbul. Combust. 91(3), 717–745 (2013)
Lehmkuhl, O., Houzeaux, G., Owen, H., Chrysokentis, G., Rodriguez, I.: A low-dissipation finite element scheme for scale resolving simulations of turbulent flows. J. Comput. Phys. 390, 51–65 (2019)
Lighthill, M.J.: On sound generated aerodynamically. I. General theory. In: Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences 1952. The Royal Society 564–587 (1952)
Liu, Q., Gómez, F.: Role of trailing-edge geometry in open cavity flow control. AIAA J. 57(2), 876–878 (2018)
Lomb, N.R.: Least-squares frequency analysis of unequally spaced data. Astrophys. Space Sci. 39(2), 447–462 (1976)
Mancini, S., Kolb, A., Gonzalez-Martino, I., Casalino, D.: Effects of Wall modifications on pressure oscillations in high-subsonic and supersonic flows over rectangular cavities. In: Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, p. 2692 (2019)
Martin, R., Soria, M., Lehmkuhl, O., Gorobets, A., Duben, A.: Noise radiated by an open cavity at low Mach number: effect of the cavity oscillation mode. Int. J. Aeroacoust. 18(6–7), 647–668 (2019)
McGrath, S., Shaw, L.: Active control of shallow cavity acoustic resonance. In: Fluid Dynamics Conference, p. 1949 (1996)
Mendoza, J., Ahuja, K.: Cavity noise control through upstream mass injection from a Coanda surface. In: Aeroacoustics Conference, p. 1767 (1996)
Pereira, J., Sousa, J.: Influence of impingement edge geometry on cavity flow oscillations. AIAA J. 32(8), 1737–1740 (1994)
Pérot, F., Gloerfelt, X., Bailly, C., Auger, J.-M., Giardi, H.: Numerical prediction of the noise radiated by a cylinder. In: Proceedings of the 9th AIAA/CEAS Aeroacoustics Conference and Exhibit 2003, p. 3240 (2003)
Piomelli, U., Chasnov, J.R.: “Large-eddy simulations: theory and applications”. In: “Turbulence and Transition Modelling,” Springer, pp. 269–336 (1996)
Rienstra, S.W., Hirschberg, A.: An introduction to acoustics. Report IWDE, pp. 92–06 (2004)
Rossiter, J.: Wind tunnel experiments on the flow over rectangular cavities at subsonic and transonic speeds. In: Technical report, Ministry of Aviation; Royal Aircraft Establishment; RAE Farnborough (1964)
Rowley, C.W., Colonius, T., Basu, A.J.: On self-sustained oscillations in two-dimensional compressible flow over rectangular cavities. J. Fluid Mech. 455, 315–346 (2002)
Saddington, A.J., Thangamani, V., Knowles, K.: Comparison of passive flow control methods for a cavity in transonic flow. J. Aircraft 53(5), 1439–1447 (2016)
Sarohia, V., Massier, P.: Control of cavity noise. J. Aircraft 14(9), 833–837 (1977)
Scargle, J.D.: Studies in astronomical time series analysis. II-Statistical aspects of spectral analysis of unevenly spaced data. Astrophys. J. 263, 835–853 (1982)
Schlichting, H., Gersten, K.: Boundary layer theory. Springer, New York (2016)
Shaaban, M., Mohany, A.: Passive control of flow-excited acoustic resonance in rectangular cavities using upstream mounted blocks. Exp. Fluids 56(4), 72 (2015)
Shaw, L.L.: Suppression of aerodynamically induced cavity pressure oscillations. J. Acoust. Soc. Am. 66(3), 880–884 (1979)
Souliez, F.J., Long, L.N., Morris, P.J., Sharma, A.: Landing gear aerodynamic noise prediction using unstructured grids. Int. J. Aeroacoust. 1(2), 115–135 (2002)
Stanek, M., Ross, J., Odedra, J., Peto, J.: High frequency acoustic suppression-the mystery of the rod-in-crossflow revealed. In: Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, p. 7 (2003)
Suponitsky, V., Avital, E., Gaster, M.: On three-dimensionality and control of incompressible cavity flow. Phys. Fluids 17(10), 104103 (2005)
Tan, X.-M., Yang, Z.-G., Tan, X.-M., Wu, X.-L., Zhang, J.: Vortex structures and aeroacoustic performance of the flow field of the pantograph. J. Sound Vib. 432, 17–32 (2018)
Ukeiley, L.S., Ponton, M.K., Seiner, J.M., Jansen, B.: Suppression of pressure loads in cavity flows. AIAA J. 42(1), 70–79 (2004)
van der Velden, W.C., van Zuijlen, A., de Jong, A., Bijl, H.: Noise estimation of beveled trailing edges using an integral and boundary element method. In: Proceedings of the 54th AIAA Aerospace Sciences Meeting, p. 0260 (2016)
Vázquez, M., Houzeaux, G., Koric, S., Artigues, A., Aguado-Sierra, J., Arís, R., Mira, D., Calmet, H., Cucchietti, F., Owen, H., Taha, A., Burness, E.D., Cela, J.M., Valero, M.: Alya: multiphysics engineering simulation toward exascale. J. Comput. Sci. 14, 15–27 (2016)
Vreman, A.: An eddy-viscosity subgrid-scale model for turbulent shear flow: algebraic theory and applications. Phys. Fluids 16(10), 3670–3681 (2004)
Wang, Z., He, P., Lv, Y., Zhou, J., Fan, J., Cen, K.: Direct numerical simulation of subsonic round turbulent jet. Flow Turbul. Combust. 84(4), 669–686 (2010)
Yao, H.-D., Davidson, L., Eriksson, L.-E.: Noise radiated by low-Reynolds number flows past a hemisphere at Ma = 0.3. Phys. Fluids 29(7), 076102 (2017)
Zhang, X., Chen, X., Rona, A., Edwards, J.: Attenuation of cavity flow oscillation through leading edge flow control. J. Sound Vib. 221(1), 23–47 (1999)
Zhao, K., Liang, Y., Yue, T., Wang, Y., Bennett, G.J.: Rectangular cavity flow noise suppression using chevron treatment to the front edge at subsonic speeds. In: Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, p. 2693 (2019)
Acknowledgements
The work of Rocio Martin was carried out within the framework of an Industrial Doctorate project from the government of Catalonia and was sponsored by the Agència de Gestió d’Ajuts Universitaris i de Recerca (AGAUR) and the automotive company SEAT S.A (2016 DI068). The work of Manel Soria has been partially supported by the Spanish Ministry (MEC) project FIS2016-77849-R. Oriol Lehmkuhl has been partially supported by a Ramón y Cajal postdoctoral contract (RYC2018-025949-I). The results were obtained using MareNotrum IV (FI-2019-1-0016 and IM-2019-2-0025) supercomputers of Red Española de Surpercomputación. The authors thankfully acknowledge these institutions.
Funding
This research received funding from AGAUR (2016 DI068) and the Spanish Ministry (FIS2016-77849-R, RYC2018-025949-I).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no confict of interest.
Rights and permissions
About this article
Cite this article
Martin, R., Soria, M., Rodriguez, I. et al. On the Flow and Passive Noise Control of an Open Cavity at Re = 5000. Flow Turbulence Combust 108, 123–148 (2022). https://doi.org/10.1007/s10494-021-00265-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10494-021-00265-y