Effects of the third-party rebate platform and information sharing on the supply chain

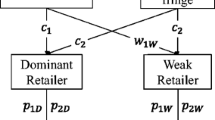
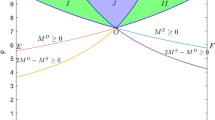
Abstract

Consumer rebates provided by third-party rebate platforms (3RPs) are prevalent in retailing. In this study, we consider the retailer’s decision to introduce a 3RP and whether to share information with the manufacturer by considering the consumers’ sensitivity to rebates, the cost of redeeming rebates, and the proportion of redeeming rebates. We developed a game-theoretic model consisting of a manufacturer, a retailer, and a 3RP. Our main results are summarized as follows: (1) The 3RP should generate reasonable unit sale commission and rebates that can stimulate the retailer to introduce the 3RP and thus maintain the 3RP’s profitability, (2) The entry of the 3RP decreases the wholesale price and retail price but increases the consumers’ demand, (3) The 3RP causes a “win-lose” situation for the retailer and the manufacturer in the non-information sharing case. However, in the information sharing case, the 3RP benefits the manufacturer but harms the retailer, (4) The information sharing benefits the manufacturer but harms the retailer in the supply chain with and without the 3RP. Moreover, information sharing benefits the 3RP. Our analysis provides insights for firms on whether to introduce the 3RP and conditions when the retailer and the 3RP are willing to share information with the manufacturer.

Appendices

Appendix A

1.1 Proof of the main models

1.1.1 Derivation of the equilibrium in the case with the 3RP under non-information sharing (Model NP)

We find the sub-game perfect equilibrium by backward induction. In stage 4, the wholesale price is $w$, the retail price is $p$, and the unit sale commission is $T$. The retailer decides on whether to introduce the 3RP or not. When the 3RP does not exist, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon }} \right)$; when the 3RP exists, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} = \left( {p - w} \right)q_{n} + \left( {p - w - T} \right)q_{p}$. Thus, the retailer will introduce the 3RP if and only if $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} \ge E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR}$ i.e., $T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$; and the retailer will not introduce the 3RP if and only if $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} < E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR}$, i.e., $T > \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$.

In stage 3, given the wholesale price $w$ and retail price $p$, the 3RP decides on the unit sale commission $T$ to maximize its profit given by:

$$ E\left[ {\pi_{p} |\varepsilon = \hat{\varepsilon }} \right]^{NP} = \left\{ {\begin{array}{*{20}l} {\left( {T - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right)} & {if} & {T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - {\text{c}}}}} \\ 0 & {if} & {T > \frac{{\left( {p - w} \right)\left( {\theta r - {\text{c}}} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}} \\ \end{array} } \right.. $$

(A.1)

Setting $T > \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$ leads to zero profit and is a dominated strategy for the 3RP. Thus, the 3RP’s optimal decision should satisfy $T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$, when we have ${ }\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} }}{\partial T} = q_{p} \ge 0$, i.e., the 3RP’s profit is non-decreasing in $T$. The optimal decision of the 3RP is,

$$ T^{NP*} = \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - {\text{c}}}}. $$

(A.2)

In addition, the 3RP should set the unit sale rebate to the consumer. The 3RP’s expected profit is $E\left[ {\pi_{p} |\varepsilon = \hat{\varepsilon }} \right]^{NP} = \left( {T - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right)$. The 3RP sets the unit sale rebate $r$ to maximize profit. And substituting $T^{NP*} = \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \delta \theta r - c}}$ into $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP}$, we have,

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} = \left( {\frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + r - {\text{c}}}} - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right). $$

(A.3)

Since $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP}$ is concave in $r$ $\left( {\frac{{\partial^{2} \pi_{p} }}{{\partial r^{2} }} = - 2\lambda {\updelta }^{2} \theta < 0} \right)$, we can obtain the optimal unit sale rebate by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} }}{\partial r} = 0$, i.e.,

$$ r^{{NP{*}}} = \frac{{\left( {p - w} \right)\theta + \left( {c + p - \hat{\varepsilon } - 1} \right)\delta }}{2\delta \theta }. $$

(A.4)

In stage 2, given$w$, the retailer determines the retailer price $p$ to maximize its expected profit. Substituting Eq. (A.4) into $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP}$, i.e.,

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon } + \lambda \left( {\theta r - {\text{c}}} \right)} \right) - \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \delta \theta r - {\text{c}}}}\left( {1 - p + \hat{\varepsilon } + \theta r - {\text{c}}} \right)\lambda . $$

(A.5)

Since $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP}$ is concave in $p$ $\left( {\frac{{\partial^{2} E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} }}{{\partial p^{2} }} = - 2 < 0} \right)$, we can obtain the best response $p$ by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NP} }}{\partial p} = 0$, i.e.,

$$ p = \frac{{w + 1 + \hat{\varepsilon }}}{2}. $$

(A.6)

In stage 1, anticipating the best response of the retailer and the 3RP. The manufacturer decides on the optimal $w$ to maximize its expected profit given by:

$$ E\left[ {\pi_{m} } \right] = w\left( {1 - \frac{w + 1 + \xi }{2} + \xi + \lambda \left( {\theta r - c} \right)} \right), $$

(A.7)

subject to $w < p$. Because $E\left[ {\pi_{m} } \right]$ is a concave function in $w$, we can derive the optimal decision by solving $\frac{{\partial { }E\left[ {\pi_{m} } \right]}}{\partial w} = 0$, i.e.,

$$ w^{NP*} = \frac{1 + \xi }{2} - \frac{{\lambda \delta {\text{c}}}}{{\lambda \theta - \lambda \delta + 2{\updelta }}}, $$

(A.8)

which satisfies $w < p$.

By substituting Eqs. (A.8)–(A.6), we have the equilibrium retail price:

$$ p^{NP*} = \frac{{3 + \xi + 2\hat{\varepsilon }}}{4} - \frac{\lambda \delta c}{{2\left( {\lambda \theta - \lambda \delta + 2\delta } \right)}}. $$

(A.9)

By substituting Eqs. (A.8–A.9) into Eqs. (A.2–A.4), we have:

$$ r^{{NP{*}}} = \frac{ \theta - \delta }{{4\delta \theta }}\left( {\frac{A}{2} - \frac{\lambda \delta c}{{\varvec{B}}}} \right) + \frac{c}{2\theta }, $$

(A.10)

$$ T^{NP*} = \frac{{\left( {B^{2} - 2\lambda c\delta } \right)\left( {\left( {\theta - \delta } \right)\left( {AB - 2\lambda c\delta } \right) - 4B\delta c} \right)}}{{8AB\left( {8\theta - 6\delta } \right) - 32\delta c\left( {\lambda \theta + B} \right)}}. $$

(A.11)

We set $A = 1 - \xi + 2\hat{\varepsilon }$, $B = \lambda \theta - \lambda \delta + 2\delta$.

By substituting Eqs. (A.8–A.11) to $E\left[ {\pi_{m} } \right]$, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]$, and $E[\pi_{p} |\varepsilon = \hat{\varepsilon }]$, we have:

$$ E[\pi_{m}^{NP*} ] = \frac{{\left( {\left( {1 + \xi } \right)B - 2\lambda \delta c} \right)\left( {\delta \left( {AB + 4\lambda \delta c} \right) + 4B\lambda \left( {\left( {\theta - \delta } \right)a - \delta c} \right)} \right))}}{{16\delta B^{2} }}, $$

(A.12)

$$ E\left[ {\pi_{p}^{NP*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\lambda \left( {a^{2} \theta \left( {\theta - \delta } \right) - a\delta \left( {\theta - \delta + 2\theta c} \right) - \delta^{2} c} \right)\left( {\left( {\delta + \theta } \right)a - \delta c} \right)}}{{4 \delta^{2} \theta }}. $$

(A.13)

We set $a = \frac{AB + 4\lambda \delta c}{{8B}}$. The unconditional expected profits of the retailer and the 3RP are:

$$ E[\pi_{r}^{NP*} ] = E\left[ E \right[\pi_{r}^{NP*} |\varepsilon = \hat{\varepsilon }]] = \frac{{\xi^{2} }}{16} + \frac{{\left[ {\left( {1 + \xi } \right)\left( {\lambda \theta - \lambda \delta + 2\delta } \right) + 2C_{r} \delta \lambda } \right]^{2} }}{{16\left( {\lambda \theta - \lambda \delta + 2\delta } \right)^{2} }}, $$

(A.14)

$$ E[\pi_{p}^{NP*} ] = E\left[ {E\left[ {\pi_{p}^{NP*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{{\lambda \left( {E[a^{2} } \right]\theta \left( {\theta - \delta } \right) - E\left( a \right)\delta \left( {\theta - \delta + 2\theta c} \right) - \delta^{2} c)\left( {\left( {\delta + \theta } \right)E\left( a \right) - \delta c} \right)}}{{4\delta^{2} \theta }}. $$

(A.15)

We set $E\left[ a \right] = \frac{{B\left( {1 - \xi } \right) + 4\lambda \delta c)}}{8B}$, $E\left[ {a^{2} } \right] = \frac{{B\left( {1 - \xi } \right) + 4\lambda \delta c)}}{8B}\left( {\frac{{B\left( {9 - \xi } \right) + 4\lambda \delta c)}}{8B}} \right) + \frac{{\xi^{2} }}{16}$.

1.1.2 Derivation of the equilibrium in the case without the 3RP under non-information sharing (Model NR)

When the 3RP does not exist, the game sequence is: in Stage 1, the manufacturer sets the wholesale price w; in Stage 2, the retailer set the retail price p. Using backward induction, we can get the equilibrium

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon }} \right), $$

(A.16)

The expected profit of the retailer $E\left[ {\hat{\pi }_{r} |\varepsilon = \hat{\varepsilon }} \right]$ is concave in p $\left( {\frac{{\partial^{2} E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR} }}{{\partial p^{2} }} = - 2 < 0} \right)$, we can get the optimal retail price by calculating $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NR} }}{\partial p} = 0$, i.e.,

$$ p = \frac{{w + 1 + \hat{\varepsilon }}}{2}. $$

(A.17)

In stage 1, the manufacturer predicts the retailer’s decision, and sets the optimal wholesale price $w$ to maximize its expected profit:

$$ E\left[ {\hat{\pi }_{m} } \right] = w\left( {1 - p + \xi } \right), $$

(A.18)

Subject to ${ }w < p$. Because $E\left[ {\hat{\pi }_{m} } \right]$ is a concave function of ${ }w$, we can obtain the manufacturer’s optimal decision by calculating ${ }\frac{{\partial E\left[ {\hat{\pi }_{m} } \right]}}{\partial w} = 0$, i.e.,

$$ \hat{w}^{NR*} = \frac{1 + \xi }{2}, $$

(A.19)

which self satisfies $w < p$.

By substituting Eqs. (A.17–A.19), we can get the optimal retail price:

$$ \hat{p}^{NR*} = \frac{{3 + \xi + \hat{\varepsilon }}}{4}. $$

(A.20)

Thus, we can get the expected profit of the manufacturer as follows:

$$ E\left[ {\hat{\pi }_{m}^{NR*} } \right] = \frac{{\left( {1 + \xi } \right)^{2} }}{8}. $$

(A.21)

We can get the expected profit and the unconditional expected profit of the retailer as follows:

$$ E[\hat{\pi }_{r}^{NR*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {1 - \xi + 2\hat{\varepsilon }} \right)^{2} }}{16}, $$

(A.22)

$$ E\left[ {\hat{\pi }_{r}^{NR*} } \right] = \frac{{3\left( {1 + \xi } \right)^{2} + 4\xi^{2} }}{48}. $$

(A.23)

1.1.3 Derivation of the equilibrium in the information sharing case with the 3RP (Model IP)

In Stage 1, based on predicting the decision of the retailer and the 3RP, the manufacturer sets the wholesale price to maximize its expected profit:

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IP} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon }} \right), $$

(A.24)

subject to ${ }w < p$. The manufacturer’s expected profit $E\left[ {\pi_{m} {|}\varepsilon = \hat{\varepsilon }} \right]$ is a concave function of w by calculating ${ }\frac{{\partial E\left[ {\pi_{m} |\varepsilon = \hat{\varepsilon }} \right]^{IP} }}{\partial w} = 0$, i.e.,

$$ w^{IP*} = \frac{{1 + \hat{\varepsilon }}}{2} - \frac{{\lambda \delta {\text{c}}}}{{\lambda \theta - \lambda \delta + 2{\updelta }}}, $$

(A.25)

which self satisfies $w < p$.

By substituting Eq. (A.26) into Eq. (A.18), we can get the optimal retail price:

$$ p^{IP*} = \frac{{3 + 3\hat{\varepsilon }}}{4} - \frac{\lambda \delta c}{{2\left( {\lambda \theta - \lambda \delta + 2\delta } \right)}}. $$

(A.26)

By substituting Eqs. (A.25–A.26) into Eqs. (A.2) and (A.4), we can get:

$$ r^{IP*} = \frac{\theta - \delta }{{4\delta \theta }}\left( {\frac{A}{2} - \frac{\lambda \delta c}{{\varvec{B}}}} \right) + \frac{c}{2\theta }, $$

(A.27)

$$ T^{IP*} = \frac{{\left( {B^{2} - 2\lambda c\delta } \right)\left( {\left( {\theta - \delta } \right)\left( {AB - 2\lambda c\delta } \right) - 4B\delta c} \right)}}{{8AB\left( {8\theta - 6\delta } \right) - 32\delta c\left( {\lambda \theta + B} \right)}}. $$

(A.28)

By substituting Eqs. (25)-(28) into $E[\pi_{p} |\varepsilon = \hat{\varepsilon }],E[\pi_{r} |\varepsilon = \hat{\varepsilon }]$, $E[\pi_{m} |\varepsilon = \hat{\varepsilon }]$, we can get:

$$ E\left[ {\pi_{p}^{IP*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\lambda \left( {a^{2} \theta \left( {\theta - \delta } \right) - a\delta \left( {\theta - \delta + 2\theta c} \right) - \delta^{2} c} \right)\left( {\left( {\delta + \theta } \right)a - \delta c} \right)}}{{4\delta^{2} \theta }}, $$

(A.29)

$$ E[\pi_{r}^{IP*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {AB + 2c\delta \lambda } \right)^{2} }}{{16{\text{B}}^{2} }}, $$

(A.30)

$$ E[\pi_{m}^{IP*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {B - 2\lambda \delta c} \right)\left( {\delta \left( {AB + 4\lambda \delta c} \right) + 4B\lambda \left( {\left( {\theta - \delta } \right)a - \delta c} \right)} \right)}}{{16\delta B^{2} }}. $$

(A.31)

We set $A = 1 + \hat{\varepsilon }$, $B = \lambda \theta - \lambda \delta + 2\delta$ and $a = \frac{AB + 4\lambda \delta c}{{8B}}$.

The unconditional expected profits of the 3RP, the retailer and the manufacturer are:

$$ E[\pi_{p}^{IP*} ] = E\left[ {E\left[ {\pi_{p}^{IP*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right]\frac{{\lambda \left( {E\left[ {a^{2} } \right]\theta \left( {\theta - \delta } \right) - E\left( a \right)\delta \left( {\theta - \delta + 2\theta c} \right) - \delta^{2} c} \right)\left( {\left( {\delta + \theta } \right)E\left( a \right) - \delta c} \right)}}{{4\delta^{2} \theta }}, $$

(A.32)

$$ E[\pi_{r} ]^{IP*} = E\left[ E \right[\pi_{r}^{IP*} |\varepsilon = \hat{\varepsilon }]] = \frac{{\xi^{2} }}{16} + \frac{{\left[ {B + 2c\delta \lambda } \right]^{2} }}{{16B^{2} }}, $$

(A.33)

$$ E[\pi_{m} ]^{IP*} = E\left[ E \right[\pi_{m}^{I*} |\varepsilon = \hat{\varepsilon }]] = \frac{{\left( {B - 2\lambda \delta c} \right)\left( {\delta \left( {B + 4\lambda \delta c} \right) + 4B\lambda \left( {\left( {\theta - \delta } \right)E\left( a \right) - \delta c} \right)} \right)}}{{16\delta B^{2} }}. $$

(A.34)

We set $E\left[ a \right] = \frac{B + 4\lambda \delta c}{{8B}}$ and $E\left[ {a^{2} } \right] = \frac{{\left( {B + 4\lambda \delta c} \right)\left( {2B + \lambda \delta c} \right)}}{{16B^{2} }} + \frac{{\xi^{2} }}{16}$.

1.1.4 Derivation of the equilibrium in the case without the 3RP under information sharing (Model IR)

When the 3RP does not exist, in Stage 1, the manufacturer sets the wholesale price w; in Stage 2, the retailer sets retail price p. we derive the equilibrium by backward induction.

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IR} = w\left( {1 - p + \xi } \right). $$

(A.35)

Since $E\left[ {\hat{\pi }_{r} |\varepsilon = \hat{\varepsilon }} \right]$ is concave in p $\left( {\frac{{\partial^{2} E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IR} }}{{\partial p^{2} }} = - 2 < 0} \right)$, we can obtain the best response p by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IR} }}{\partial p} = { }0$, i.e.,

$$ p = \frac{{w + 1 + \hat{\varepsilon }}}{2}. $$

(A.36)

In Stage 1, the manufacturer predicts the retailer’s decision, and sets the optimal wholesale price to maximize its expected profits:

$$ E\left[ {\pi_{m} |\varepsilon = \hat{\varepsilon }} \right]^{IR} = w\left( {1 - p + \hat{\varepsilon }} \right), $$

(A.37)

subject to ${ }w < p$. Because $E\left[ {\pi_{m} |\varepsilon = \hat{\varepsilon }} \right]^{IR}$ is a concave function of ${ }w$. By calculating $\frac{{\partial E\left[ {\pi_{m} |\varepsilon = \hat{\varepsilon }} \right]^{IR} }}{\partial w} = 0$, we can get the manufacturer’s optimal decision, i.e.,

$$ \hat{w}^{IR*} = \frac{{1 + \hat{\varepsilon }}}{2}, $$

(A.38)

Which self satisfies $w < p$.

By calculating Eq. (38) into Eq. (7), we can get the optimal retail price:

$$ \hat{p}^{IR*} = \frac{{3 + 3\hat{\varepsilon }}}{4}. $$

(A.39)

We can get the expected profits of the manufacturer and the retailer:

$$ E[\hat{\pi }_{m}^{IR*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {1 + \hat{\varepsilon }} \right)^{2} }}{8}, $$

(A.40)

$$ E\left[ {\hat{\pi }_{r}^{IR*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\left( {1 + \hat{\varepsilon }} \right)^{2} }}{16}. $$

(A.41)

The unconditional expected profits of the retailer and the manufacturer are

$$ { }E[\pi_{r} ]^{{IR{*}}} = E\left[ E \right[\pi_{r}^{{IR{*}}} |\varepsilon = \hat{\varepsilon }]] = \frac{{\xi^{2} + 3\left( {1 + \xi } \right)^{2} }}{48}, $$

(A.42)

$$ E[\pi_{m} ]^{{IR{*}}} = E\left[ E \right[\pi_{m}^{{IR{*}}} |\varepsilon = \hat{\varepsilon }]] = \frac{{4\xi^{2} + 6\xi + 3}}{24}. $$

(A.43)

Thus, the unconditional expected profit of the supply chain is

$$ E[\pi_{c}^{{IR{*}}} ] = \frac{{3\xi^{2} + \left( {1 + \xi } \right)^{2} + 4\xi + 2}}{16}. $$

(A.44)

1.1.5 Proof of Propositions 1–2

The results can be straightforwardly obtained by comparing the equilibrium with and without the 3RP, the details are omitted.

1.1.6 Proof of Propositions 3–5

The results can be straightforwardly obtained by comparing the equilibrium in the non-information sharing case and the information sharing case, the details are omitted.

Appendix B

In this section, we analyze and discuss the robustness of our insights into several alternative modeling assumptions. First, we provide an analysis of a model where consumers are uncertain to whether they will redeem the rebate. Second, we extend the model to consider a different game sequence that the 3RP moves first to determine the consumer rebate and then the manufacturer and retailer determine the wholesale price and retail price, respectively.

2.1 Proof of extension 1: considering consumer’s rebate redemption uncertainty

In the main model, we consider consumers who use the 3RP believe they will redeem rebates. However, some consumers do not fully believe they will redeem the rebate in the reality. Thus, we consider that $1 - \lambda$ proportion ensures they purchase the product through the retailer, $\lambda$ proportion purchases the product through the 3RP, in which $\left( {1 - \alpha } \right)\lambda$ proportion ensures they redeem the rebate, and the remaining purchase the product through the 3RP but uncertain whether they will redeem the rebate. We consider $\beta$ proportion consumers who do not fully believe they will remember to redeem the rebate, and the remaining fail to redeem the rebate. Consistent with the main model, we also consider $\delta$ proportion of the 3RP users who will redeem rebates successfully, and the remaining $1 - \delta$ proportion of 3RP users who will fail to redeem rebates. Based on the above and following Fan et al. (2020), we can find that the demand in this section is the same as the main model. And the profit of the 3RP is as follows,

$$ \pi_{p} = \left( {T - \left( {1 - \alpha + \alpha \beta } \right)\delta r} \right)q_{p} . $$

(B.1)

2.2 Derivation of the equilibrium in the case with the 3RP under non-information sharing (Model NPU)

We find the sub-game perfect equilibriums in the case considering consumer’s rebate redemption uncertainty by backward induction which is the same as the main model. We get the equilibriums in the case with the 3RP under non-information sharing (Model NPU), the details are omitted. Note that we set $A = 1 - \xi + 2\hat{\varepsilon }$, $B = 1 - \alpha + \alpha \beta$.

$$ w^{NPU*} = \frac{{2\delta B\left( {1 + \xi - \left( {c + \frac{\xi }{2} + \frac{1}{2}} \right)\lambda } \right) + \lambda \theta \left( {1 + \xi } \right)}}{{2\delta B\left( {2 - \lambda } \right) + 2\lambda \theta }}, $$

$$ p^{NPU*} = \frac{{2\delta B\left( {3 + 2\hat{\varepsilon } + \xi - \left( {c + \frac{\xi }{2} + \hat{\varepsilon } + \frac{3}{2}} \right)\lambda } \right) + \lambda \theta \left( {3 + \xi + 2\hat{\varepsilon }} \right)}}{{4\delta B\left( {2 - \lambda } \right) + 4\lambda \theta }}, $$

$$ r^{{NPU{*}}} = \frac{{\delta^{2} B^{2} \left( {\left( {2A - c} \right)\lambda - 2A + 8c} \right) + \theta \delta B\left( {2\left( {c - A} \right)\lambda + 2A} \right) - \theta^{2} \lambda A}}{{8\delta \theta B\left( {\left( {2 - \lambda } \right)B + \lambda \theta } \right)}}, $$

$$ T^{NPU*} = \frac{{\left( {\left( {c + \frac{A}{2}} \right)\lambda - 4c - A} \right)\delta^{2} B^{2} - \left( {\left( {\left( {B + A} \right)\lambda - A} \right)\theta \delta B + \frac{{\theta^{2} \lambda A}}{2}} \right)\left( {\left( {\left( {c - \frac{A}{2}} \right)\lambda + A} \right)B\delta + \frac{\theta \lambda A}{2}} \right)}}{{\left( {\delta B\left( {2 - \lambda } \right) + \lambda \theta } \right)\left( {\left( {\left( {c - A} \right)\lambda + 2B - 8c} \right)\delta^{2} B^{2} - \frac{{\theta \delta B\left( {c\lambda - A} \right)}}{3} + \frac{{\theta^{2} \lambda A}}{6}} \right)}}. $$

$$ E[\pi_{m}^{NPU*} ] = \frac{{\left( {\left( {\left( {c + \frac{\xi }{2} + \frac{1}{2}} \right)\lambda - \xi - 1} \right)B\delta - \frac{\lambda \theta }{2}\left( {1 + \xi } \right)} \right)\left( {\left( {\left( {c + \frac{A}{2}} \right)\lambda - A} \right)B\delta - \frac{\theta \lambda A}{2}} \right)}}{{4\delta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)}}, $$

$$ E\left[ {\pi_{r}^{NPU*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\left( {\left( {\left( {c - \frac{A}{2}} \right)\lambda + A} \right)B\delta + \frac{\theta \lambda A}{2}} \right)^{2} }}{{4\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}, $$

$$ E\left[ {\pi_{p}^{NPU*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{\begin{gathered} 9\left( {\left( {c - \frac{A}{6}} \right)\lambda - \frac{4c}{3} + \frac{A}{3}} \right)^{2} B^{4} \delta^{4} - \theta \delta^{3} B^{3} (\left( {4\hat{\varepsilon }^{2} + \left( {4 - 2c - 4\xi } \right)\hat{\varepsilon } + \xi^{2} + \left( {5c - 2} \right)\xi + 10c^{2} - 5c + 1} \right)\lambda^{2} \hfill \\ - \left( {12\hat{\varepsilon }^{2} + \left( {12 - 8c - 12\xi } \right)\hat{\varepsilon } + 3\xi^{2} - \left( {6 - 4c} \right)\xi + 3 + 8c^{2} - 4c} \right)\lambda + 2A\left( {A + 4c} \right)) \hfill \\ + \theta^{2} \delta^{2} B^{2} \left( {\left( {6\hat{\varepsilon }^{2} + \left( {6 - 2c - 6\xi } \right)\hat{\varepsilon } + \frac{3}{2}\hat{\varepsilon }^{2} - \left( {3 - c} \right)\xi + c^{2} - c + \frac{3}{2}} \right)\lambda^{2} - 6A\left( {c + \frac{A}{2}} \right)\lambda + A^{2} } \right) \hfill \\ + \left( {A - \left( {c + A} \right)\lambda } \right)A\theta^{3} \delta \lambda B + \frac{1}{4}\theta^{4} \lambda^{2} B^{2} \hfill \\ \end{gathered} }{{16\delta \theta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}. $$

The unconditional expected profits of the retailer and the 3RP are:

$$E[\pi_{r}^{NPU*} ] = E\left[ {E\left[ {\pi_{r}^{NPU*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{{\xi^{2} }}{{48\left({\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)}}+ \frac{{\left( {\left( {2c - \xi - 1} \right)\lambda + 2\xi + 2}\right)B\delta + \theta \lambda \left( {1 + \xi } \right)}}{{8\left({\left( {2 - \lambda } \right)\delta B + \lambda \theta }\right)^{2} }}, $$

$$E[\pi_{p}^{NPU*} ] = E\left[ {E\left[ {\pi_{p}^{NPU*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{\begin{gathered}9\lambda ((B^{4} \delta^{4} \left( {c - \frac{\xi }{6} -\frac{1}{6}} \right)\lambda^{2} - \left( {\left( {\frac{{\delta^{4}}}{72} + \frac{1}{9}} \right)\xi^{2} + \left( {\frac{2}{9} -\frac{10c}{9}} \right)\xi + \frac{{8c^{2} }}{3} - \frac{10c}{9} +\frac{1}{9}} \right)\lambda + \left( {\frac{{\delta^{4} }}{36} +\frac{1}{9}} \right)\xi^{2} + \left( {\frac{2}{9} - \frac{8c}{9}}\right)\xi \hfill \\ + \frac{16}{9}\left( {c - \frac{1}{4}} \right)^{2}- \frac{10}{9}B^{2} \delta^{2} \theta \left( {2\xi^{2} + \left({3 - c} \right)\xi + c^{2} - c + \frac{3}{2}} \right)\lambda^{2} -\left( {4\xi^{2} + 6\left( {1 + c} \right)\xi + 6c + 3}\right)\lambda + \frac{4}{3}\xi^{2} + 2\xi + 1) \hfill \\ +\frac{1}{10}B\theta^{2} \lambda \delta \left( {\left({\frac{4}{3}\xi^{2} + \left( {c + 2} \right)\xi + c + 1}\right)\lambda - \frac{4}{3}\xi^{2} - 2\xi - 1} \right) -\frac{1}{30}\theta^{3} \lambda^{2} \left( {\xi^{2} + \frac{3}{2}\xi + \frac{3}{4}} \right)) \hfill \\ \end{gathered} }{{8\delta \theta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta }\right)^{2} }}. $$

2.3 Derivation of the equilibrium in the case without the 3RP under non-information sharing (Model NRU)

The equilibriums in the case without the 3RP under non-information sharing (Model NRU) are the same with that in Model NR.

2.4 Derivation of the equilibrium in the information sharing case with the 3RP (Model IPU)

We find the sub-game perfect equilibriums in the case considering consumer’s rebate redemption uncertainty by backward induction which is the same as the main model. We get the equilibriums in the case with the 3RP under information sharing (Model IPU), the details are omitted. Note that we set $A = 1 + \hat{\varepsilon }$, $B = 1 - \alpha + \beta \alpha$, $C = 1 + \xi$.

$$ w^{NPU*} = \frac{{2\delta B\left( {1 + \hat{\varepsilon } - \left( {c + \frac{{\hat{\varepsilon }}}{2} + \frac{1}{2}} \right)\lambda } \right) + \lambda \theta \left( {1 + \hat{\varepsilon }} \right)}}{{2\delta B\left( {2 - \lambda } \right) + 2\lambda \theta }}, $$

$$ p^{NPU*} = \frac{{2\delta B\left( {3 + 3\hat{\varepsilon } - \left( {c + \frac{{3\hat{\varepsilon }}}{2} + \frac{3}{2}} \right)\lambda } \right) + 3\lambda \theta \left( {\hat{\varepsilon } + 1} \right)}}{{4\delta B\left( {2 - \lambda } \right) + 4\lambda \theta }}, $$

$$ r^{{NPU{*}}} = \frac{{\delta^{2} B^{2} \left( {\left( {2A - c} \right)\lambda - 2A + 8c} \right) + \theta \delta B\left( {2\left( {c - A} \right)\lambda + 2A} \right) - \theta^{2} \lambda A}}{{8\delta \theta B\left( {\left( {2 - \lambda } \right)B + \lambda \theta } \right)}}, $$

$$ T^{NPU*} = \frac{{\left( {\left( {c + \frac{A}{2}} \right)\lambda - 4c - A} \right)\delta^{2} B^{2} - \left( {\left( {\left( {B + A} \right)\lambda - A} \right)\theta \delta B + \frac{{\theta^{2} \lambda A}}{2}} \right)\left( {\left( {\left( {c - \frac{A}{2}} \right)\lambda + A} \right)B\delta + \frac{\theta \lambda A}{2}} \right)}}{{\left( {\delta B\left( {2 - \lambda } \right) + \lambda \theta } \right)\left( {\left( {\left( {c - A} \right)\lambda + 2B - 8c} \right)\delta^{2} B^{2} - \frac{{\theta \delta B\left( {c\lambda - A} \right)}}{3} + \frac{{\theta^{2} \lambda A}}{6}} \right)}}. $$

$$ E[\pi_{m}^{NPU*} ] = \frac{{\left( {\left( {\left( {c + \frac{1}{2}A} \right)\lambda - A} \right)B\delta - \frac{\lambda \theta }{2}A} \right)^{2} }}{{4\delta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)}}, $$

$$ E\left[ {\pi_{r}^{NPU*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\left( {\left( {\left( {c - \frac{A}{2}} \right)\lambda + A} \right)B\delta + \frac{\theta \lambda A}{2}} \right)^{2} }}{{4\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}, $$

$$ E\left[ {\pi_{p}^{NPU*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{\begin{gathered} 9\lambda \left( {\left( {c - \frac{A}{6}} \right)\lambda - \frac{4c}{3} + \frac{A}{3}} \right)^{2} B^{4} \delta^{4} - \theta \delta^{3} B^{3} (\left( {\hat{\varepsilon }^{2} + \left( {2 + 3c} \right)\hat{\varepsilon } + 10c^{2} - 5c + 1} \right)\lambda^{2} \hfill \\ - \left( {\left( {6 - 4c} \right)\hat{\varepsilon } + 3\hat{\varepsilon }^{2} + 3 + 8c^{2} - 4c} \right)\lambda + 2A\left( {A + 4c} \right)) \hfill \\ + \theta^{2} \delta^{2} B^{2} \left( {\left( {\left( {3 - c} \right)\hat{\varepsilon } + \frac{3}{2}\hat{\varepsilon }^{2} + c^{2} - c + \frac{3}{2}} \right)\lambda^{2} - 6A\left( {c + \frac{A}{2}} \right)\lambda + A^{2} } \right) \hfill \\ + \left( {A - \left( {c + A} \right)\lambda } \right)A\theta^{3} \delta \lambda B + \frac{1}{4}\theta^{4} \lambda^{2} B^{2} \hfill \\ \end{gathered} }{{16\delta \theta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}. $$

The unconditional expected profits of the retailer and the 3RP are:

$$ E[\pi_{m} ]^{{NPU{*}}} = E\left[ {E\left[ {\pi_{m}^{{NPU{*}}} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{{\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)\xi^{2} }}{{96\delta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)}} + \left( {\frac{{\left( {\left( {c + \frac{C}{2}} \right)\lambda - C} \right)\delta B - \frac{\lambda \theta C}{2}}}{{4\delta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)}}} \right)^{2} . $$

$$ E[\pi_{r}^{NPU*} ] = E\left[ {E\left[ {\pi_{r}^{NPU*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{{\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)\xi^{2} }}{{96\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }} + \left( {\frac{{\left( {\left( {c + \frac{C}{2}} \right)\lambda - C} \right)\delta B - \frac{\lambda \theta C}{2}}}{{4\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}} \right)^{2} , $$

$$ E[\pi_{p}^{NPU*} ] = E\left[ {E\left[ {\pi_{p}^{NPU*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{\begin{gathered} 9\lambda (\left( {B^{4} \delta^{4} \left( {\frac{2}{15}\xi^{2} + \left( {\frac{1}{5} - \frac{c}{2}} \right)\xi + c^{2} - \frac{c}{2} + \frac{1}{10}} \right)\lambda^{2} - \left( {\frac{2}{5}\xi^{2} + \left( {\frac{3}{5} - \frac{2c}{3}} \right)\xi + \frac{{4c^{2} }}{5} - \frac{2c}{5} + \frac{3}{10}} \right)\lambda + \frac{4}{5}\left( {1 + \xi } \right)\left( {\frac{1}{4} + \frac{\xi }{4} + c} \right)} \right) \hfill \\ - \frac{10}{9}B^{2} \delta^{2} \theta \left( {2\xi^{2} + \left( {3 - c} \right)\xi + c^{2} - c + \frac{3}{2}} \right)\lambda^{2} - \left( {4\xi^{2} + 6\left( {1 + c} \right)\xi + 6c + 3} \right)\lambda + \frac{4}{3}\xi^{2} + 2\xi + 1) \hfill \\ + \frac{1}{9}B\theta^{2} \lambda \delta \left( {\left( {\frac{4}{3}\xi^{2} + \left( {c + 2} \right)\xi + c + 1} \right)\lambda - \frac{4}{3}\xi^{2} - 2\xi - 1} \right) - \frac{1}{36}\theta^{3} \lambda^{2} \left( {\xi^{2} + \frac{3}{2}\xi + \frac{3}{4}} \right)) \hfill \\ \end{gathered} }{{16\delta \theta B\left( {\left( {2 - \lambda } \right)\delta B + \lambda \theta } \right)^{2} }}. $$

2.5 Derivation of the equilibrium in the case without the 3RP under non-information sharing (Model IRU)

The equilibriums in the case without the 3RP under information sharing (Model IRU) are the same with that in Model IR.

2.6 Effects of the 3RP entry

Because the theoretical comparison of the expected profits is difficult to make, a numerical study is used here. We depicted with $\delta = 0.7$, $\xi = 0.5$, $\beta = 0.2$, and $\alpha = 0.4$. By comparing the cases of 3RP entry and without the 3RP entry in information sharing and non-information sharing scenarios, we examine the value of the 3RP entry when considering consumer’s rebate redemption uncertainty. Let $V_{m}^{i} = E\left[ {\pi_{m}^{{iPU{*}}} } \right] - E\left[ {\pi_{m}^{{iRU{*}}} } \right]$ (where i = N or I) denote the value of the 3RP for the manufacturer in the non-information sharing and information sharing cases, respectively. Let $V_{r}^{i} = E\left[ {\pi_{r}^{{iPU{*}}} } \right] - E\left[ {\pi_{r}^{{iRU{*}}} } \right]$ (where i = N or I) denote the value of the 3RP for the retailer in the cases of non-information sharing and information sharing. 

Fig. 4

Effects of the 3RP entry under non-information sharing and information sharing

We find the effect of the 3RP for the manufacturer and the retailer are the same with that in the main model. The figures show that when $\lambda$ is higher, the 3RP provides more benefits for the retailer but harms the manufacturer more in the non-information sharing case. However, in the information sharing case, the 3RP provides less benefit for the manufacturer and harms the retailer less with the increase of $\lambda$.

2.7 Effects of information sharing

We compared the cases of information sharing and non-information sharing when the 3RP was introduced and determined the value of information sharing. Let $V_{m}^{IN} = E\left[ {\pi_{m}^{{IP{*}}} } \right] - E\left[ {\pi_{m}^{NP*} } \right]$ denote the value of information sharing for the manufacturer. Let $V_{r}^{IN} = E\left[ {\pi_{r}^{{IP{*}}} } \right] - E\left[ {\pi_{r}^{NP*} } \right]$ denote the value of information sharing for the retailer. We also use $V_{p}^{IN} = E\left[ {\pi_{p}^{{IP{*}}} } \right] - E\left[ {\pi_{p}^{NP*} } \right]$ to denote the value of information sharing for the 3RP. Consistent with our analytical results, we have $V_{m}^{IN} > 0$, $V_{r}^{IN} < 0$ and $V_{p}^{IN} > 0$. Specifically, the numerical experiments show that, as the increase of $\lambda$, information sharing harms the retailer more, benefits the manufacturer less, and lastly creates more value for the 3RP. In addition, as the increase of $\delta$, information sharing benefits the manufacturer and harms both the 3RP and the retailer more (Fig. 

Fig. 5

Effects of information sharing in the supply chain with the 3RP

5).

2.8 Proof of extension 2: the 3RP as the Stackelberg leader

In the main model, we consider the 3RP sets the commission and rebate after the retailer sets the retail price and the manufacturer sets the wholesale price. However, the 3RP sets the rebate before the manufacturer and the retailer set the wholesale price and retail price, respectively in the reality. Thus, we extend the model to consider a different game sequence that the 3RP moves first to determine the consumer rebate and then the manufacturer and retailer determine the wholesale price and retail price, respectively. Lastly, the 3RP determines the unit sale commission combined with the retail price.

2.9 Derivation of the equilibrium in the case with the 3RP under non-information sharing (Model NPD)

In stage 4, the retailer decides on whether to introduce the 3RP or not. When the 3RP does not exist, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon }} \right)$; when the 3RP exists, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left( {p - w} \right)q_{n} + \left( {p - w - T} \right)q_{p}$. Thus, the retailer will introduce the 3RP if and only if $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} \ge E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$ i.e., $T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$; and the retailer will not introduce the 3RP if and only if $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} < E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$, i.e., $T > \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$.

In stage 1, anticipating the best response of the retailer and the manufacturer. The 3RP decides on the optimal $T$ to maximize its expected profit given by:

$$ E\left[ {\pi_{p} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left\{ {\begin{array}{*{20}c} {\left( {T - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right)} & {if} & {T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - {\text{c}}}}} \\ 0 & {if} & {T > \frac{{\left( {p - w} \right)\left( {\theta r - {\text{c}}} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}} \\ \end{array} } \right.. $$

(B.2)

Setting $T > \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$ leads to zero profit and is a dominated strategy for the 3RP. Thus, the 3RP’s optimal decision should satisfy $T \le \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}$, when we have ${ }\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} }}{\partial T} = q_{p} \ge 0$, i.e., the 3RP’s profit is non-decreasing in $T$. The optimal decision of the 3RP is,

$$ T^{NPD*} = \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \theta r - c}}. $$

(B.3)

We find the sub-game perfect equilibrium by backward induction. In stage 3, given $w$, and $r$, the retailer determines the retailer price $p$ to maximize its expected profit. $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$, i.e.,

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left( {p - w} \right)\left( {1 - p + \hat{\varepsilon } + \lambda \left( {\theta r - {\text{c}}} \right)} \right) - T\left( {1 - p + \hat{\varepsilon } + \theta r - {\text{c}}} \right)\lambda . $$

(B.4)

Since $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$ is concave in $p$ $\left( {\frac{{\partial^{2} E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} }}{{\partial p^{2} }} = - 2 < 0} \right)$, we can obtain the best response $p$ by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} }}{\partial p} = 0$, i.e.,

$$ p = \frac{{w + 1 + \hat{\varepsilon }}}{2}. $$

(B.5)

In stage 2, anticipating the best response of the retailer. The manufacturer decides on the optimal $w$ to maximize its expected profit given by:

$$ E\left[ {\pi_{m} } \right]^{NPD*} = w\left( {1 - \frac{w + 1 + \xi }{2} + \xi + \lambda \left( {\theta r - c} \right)} \right), $$

(B.6)

subject to $w < p$. Because $E\left[ {\pi_{m} } \right]$ is a concave function in $w$, we can derive the optimal decision by solving $\frac{{\partial { }E\left[ {\pi_{m} } \right]}}{\partial w} = 0$, i.e.,

$$ w^{NPD*} = \frac{1 + \xi }{2} + \lambda \left( {r\theta - {\text{c}}} \right), $$

(B.7)

which satisfies $w < p$.

In addition, the 3RP should set the unit sale rebate to the consumer. The 3RP’s expected profit is $E\left[ {\pi_{p} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left( {T - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right)$. The 3RP sets the unit sale rebate $r$ to maximize profit. And substituting $T^{NPD*} = \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \delta \theta r - c}}$ into $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$, we have,

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} = \left( {\frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + r - {\text{c}}}} - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right). $$

(B.8)

Since $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD}$ is concave in $r$ $\left( {\frac{{\partial^{2} \pi_{p} }}{{\partial r^{2} }} = - 2\lambda {\updelta }^{2} \theta < 0} \right)$, we can obtain the optimal unit sale rebate by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{NPD} }}{\partial r} = 0$, i.e.,

$$ r^{{NPD{*}}} = \frac{{4\theta \left( {4c\lambda + A} \right) - 4\delta \left( {2c\lambda - 4c + A} \right)}}{4\theta B}. $$

(B.9)

We have the equilibriums:

$$ w^{NPD*} = \frac{{2c\delta \lambda^{2} + 4\delta \left( {1 + \xi } \right) - \left( {\left( {4c + \xi + 2\hat{\varepsilon } + 3} \right)\delta - \left( {\xi + 2\hat{\varepsilon } + 3} \right)\theta } \right)\lambda }}{{4{\text{B}}}}. $$

(B.10)

$$ p^{{NPD{*}}} = \frac{{2c\delta \lambda^{2} + 4\delta \left( {\xi + 2\hat{\varepsilon } + 3} \right) - \left( {\left( {4c + \xi + 6\hat{\varepsilon } + 7} \right)\delta - \theta \left( {\xi + 6\hat{\varepsilon } + 7} \right)} \right)\lambda }}{8B}, $$

(B.11)

$$ T^{NPD*} = \frac{{\left( {\frac{\theta \lambda }{2}A - \left( {c\lambda^{2} + \left( {\frac{A}{2} - 2c} \right)\lambda - 2A} \right)\delta } \right)\left( {\left( {c\lambda - 2c - \frac{A}{2}} \right)\delta + \frac{1}{2}\theta A} \right)}}{{2B\left( {\left( {2 - \lambda } \right)\delta \left( {c\lambda - 2c - \frac{1}{2}\xi + \hat{\varepsilon } + \frac{1}{2}} \right) + \frac{1}{2}\theta \left( {\lambda + 2} \right)A} \right)}}. $$

(B.12)

By substituting Eqs. (B.10–B.13) to $E\left[ {\pi_{m} } \right]$, $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]$, and $E[\pi_{p} |\varepsilon = \hat{\varepsilon }]$, we have:

$$ E\left[ {E\left[ {\pi_{r}^{NPD*} {|}\varepsilon = \hat{\varepsilon }} \right]} \right] = \frac{{\left( {c\delta \lambda^{2} + \left( {\left( {\frac{A}{2} - 2c} \right)\delta - \frac{1}{2}A\theta } \right)\lambda - 2\delta A} \right)^{2} }}{{16B^{2} }}, $$

(B.13)

$$ E[\pi_{m}^{NPD*} ] = \frac{{\left( {c\delta \lambda^{2} + \left( {\left( { - 2c - \frac{3\xi }{2} - \frac{3}{2}} \right)\delta + \frac{{3\theta \left( {\xi + 1} \right)}}{2}} \right)\lambda + 2\delta \left( {\xi + 1} \right)} \right)^{2} }}{{8B^{2} }}, $$

(B.14)

$$ E\left[ {\pi_{p}^{NPD*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\left( {\left( {\left( {\lambda - 2} \right)c + \frac{A}{2}} \right)^{2} \delta^{2} - \theta A\left( {\left( {\lambda + 2} \right)c + \frac{A}{2}} \right)\delta + \frac{1}{4}\theta^{2} A^{2} } \right)\lambda }}{8\theta B}. $$

(B.15)

Note that we set $A = 1 - \xi + 2\hat{\varepsilon }$, $B = \lambda \theta - \lambda \delta + 2\delta$.

2.10 Derivation of the equilibrium in the case without the 3RP under non-information sharing (Model NRD)

The equilibriums in the case without the 3RP under non-information sharing (Model NRD) are the same with that in Model NR.

2.11 Derivation of the equilibrium in the information sharing case with the 3RP (Model IPD)

In Stage 1, based on predicting the decision of the retailer and the 3RP, the manufacturer sets the wholesale price to maximize its expected profit:

$$ E\left[ {\pi_{m} |\varepsilon = \hat{\varepsilon }} \right]^{IPD*} = w\left( {1 - \frac{w + 1 + \xi }{2} + \xi + \lambda \left( {\theta r - c} \right)} \right), $$

(B.16)

subject to ${ }w < p$. The manufacturer’s expected profit $E\left[ {\pi_{m} {|}\varepsilon = \hat{\varepsilon }} \right]$ is a concave function of w by calculating $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IPD} }}{\partial w} = 0$, i.e.,

$$ w^{IPD*} = \frac{{1 + \hat{\varepsilon }}}{2} + \lambda \left( {r\theta - {\text{c}}} \right), $$

(B.17)

which self satisfies $w < p$.

In addition, the 3RP should set the unit sale rebate to the consumer. The 3RP’s expected profit is $E\left[ {\pi_{p} |\varepsilon = \hat{\varepsilon }} \right]^{IPD} = \left( {T - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right)$. The 3RP sets the unit sale rebate $r$ to maximize profit. And substituting $T^{IPD*} = \frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + \delta \theta r - c}}$ into $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IPD}$, we have,

$$ E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IPD} = \left( {\frac{{\left( {p - w} \right)\left( {\theta r - c} \right)}}{{1 - p + \hat{\varepsilon } + r - {\text{c}}}} - \delta r} \right)\lambda \left( {1 - p + \hat{\varepsilon } + \theta r - c} \right). $$

(B.18)

Since $E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IPD}$ is concave in $r$ $\left( {\frac{{\partial^{2} \pi_{p} }}{{\partial r^{2} }} = - 2\lambda {\updelta }^{2} \theta < 0} \right)$, we can obtain the optimal unit sale rebate by solving the first order condition, $\frac{{\partial E\left[ {\pi_{r} |\varepsilon = \hat{\varepsilon }} \right]^{IPD} }}{\partial r} = 0$, i.e.,

$$ r^{{IPD{*}}} = \frac{{4\theta \left( {4c\lambda + A} \right) - 4\delta \left( {2c\lambda - 4c + A} \right)}}{4\theta B}. $$

(B.19)

We have the equilibriums:

$$ w^{IPD*} = \frac{{2c\delta \lambda^{2} - \left( {\left( {4c + 3A} \right)\delta - 3\theta A} \right)\lambda + 4A\delta }}{4B}. $$

(B.20)

$$ p^{{IPD{*}}} = \frac{{2c\delta \lambda^{2} + 12\delta A - \left( {\left( {4c + 7A} \right)\delta - 7\theta A} \right)\lambda }}{8B}, $$

(B.21)

$$ T^{IPD*} = - \frac{{\left( {\frac{\theta \lambda }{2}A - \left( {c\lambda^{2} + \left( {\frac{A}{2} - 2c} \right)\lambda - 2A} \right)\delta } \right)\left( {\left( {c\lambda - 2c - \frac{A}{2}} \right)\delta + \frac{1}{2}\theta A} \right)}}{{2B\left( {\left( {2 - \lambda } \right)\delta \left( {c\lambda - 2c + \frac{1}{2}A} \right) + \frac{1}{2}\theta \left( {\lambda + 2} \right)A} \right)}}. $$

(B.22)

By substituting Eqs. (B.19–B.22) into $E[\pi_{p} |\varepsilon = \hat{\varepsilon }],E[\pi_{r} |\varepsilon = \hat{\varepsilon }]$, and $E[\pi_{m} |\varepsilon = \hat{\varepsilon }]$, we can get:

$$ E\left[ {\pi_{p}^{IPD*} |\varepsilon = \hat{\varepsilon }} \right] = \frac{{\left( {\left( {\left( {\lambda - 2} \right)c + \frac{1}{2}\hat{\varepsilon } + \frac{1}{2}} \right)^{2} \delta^{2} - \theta \left( {\hat{\varepsilon } + 1} \right)\left( {\left( {\lambda + 2} \right)c + \frac{1}{2}\hat{\varepsilon } + \frac{1}{2}} \right)\delta + \frac{1}{4}\theta^{2} \left( {\hat{\varepsilon } + 1} \right)^{2} } \right)\lambda }}{8B}, $$

(B.23)

$$ E[\pi_{r}^{IPD*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {c\delta \lambda^{2} + \left( {\left( { - 2c + \frac{A}{2}} \right)\delta - \frac{1}{2}\theta A} \right)\lambda - 2A\delta } \right)^{2} }}{{16B^{2} }}, $$

(B.24)

$$ E[\pi_{m}^{IPD*} |\varepsilon = \hat{\varepsilon }] = \frac{{\left( {c\delta \lambda^{2} + \left( {\left( { - 2c - \frac{3}{2}A} \right)\delta + \frac{3}{2}\theta A} \right)\lambda + 2\delta A} \right)^{2} }}{{8B^{2} }}. $$

(B.25)

We set $A = 1 + \hat{\varepsilon }$, and $B = \lambda \theta - \lambda \delta + 2\delta$.

2.12 Derivation of the equilibrium in the case without the 3RP under non-information sharing (Model IRD)

The equilibriums in the case without the 3RP under information sharing (Model IRD) are the same with that in Model IR.

2.13 Effects of the 3RP entry

Because the theoretical comparison of the expected profits is difficult to make, a numerical study is used here. We depicted with $\delta = 0.7$., $\xi = 0.5$, $\beta = 0.2$, and $\alpha = 0.4$. By comparing the cases of 3RP entry and without the 3RP entry in information sharing and non-information sharing scenarios, we examine the value of the 3RP entry when considering consumer’s rebate redemption uncertainty. Let $V_{m}^{i} = E\left[ {\pi_{m}^{{iPD{*}}} } \right] - E \left[ {\pi_{m}^{{iRD{*}}} } \right]$ (where i = N or I) denote the value of the 3RP for the manufacturer in the non-informaon sharing and information sharing cases, respectively. Let $V_{r}^{i} = E\left[ {\pi_{r}^{{iPD{*}}} } \right] - E\left[ {\pi_{r}^{{iRD{*}}} } \right]$ (where i = N or I) denote the value of the 3RP for the retailer in the cases of non-information sharing and information sharing, respectively. We find the effect of the 3RP for the manufacturer and the retailer are the same with that in the main model. The figures show that when $\lambda$ is higher, the 3RP provides more benefits for the retailer but harms the manufacturer more in the non-information sharing case. However, in the information sharing case, the 3RP provides less benefit for the manufacturer and harms the retailer less with the increase of $\lambda$ (Fig. 

Fig. 6

Effects of the 3RP entry under non-information sharing and information sharing

6).

2.14 Effects of information sharing

We compared the cases of information sharing and non-information sharing when the 3RP was introduced and determined the value of information sharing. Let $V_{m}^{IN} = E\left[ {\pi_{m}^{{IP{*}}} } \right] - E\left[ {\pi_{m}^{NP*} } \right]$ denote the value of information sharing for the manufacturer. Let $V_{r}^{IN} = E\left[ {\pi_{r}^{{IP{*}}} } \right] - E\left[ {\pi_{r}^{NP*} } \right]$ denote the value of information sharing for the retailer. We also use $V_{p}^{IN} = E\left[ {\pi_{p}^{{IP{*}}} } \right] - E\left[ {\pi_{p}^{NP*} } \right]$ to denote the value of information sharing for the 3RP. Consistent with our analytical results, we have $V_{m}^{IN} > 0$, $V_{r}^{IN} < 0$ and $V_{p}^{IN} > 0$. Specifically, the numerical experiments show that, as the increase of $\lambda$, information sharing harms the retailer more, benefits the manufacturer less, and lastly creates more value for the 3RP. In addition, as the increase of $\delta$, information sharing benefits the manufacturer and harms both the 3RP and the retailer more (Fig. 

Fig. 7

Effects of information sharing in the supply chain with the 3RP

7).