An equilibrium model for the cement sector: EU-ETS analysis with power contracts

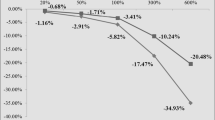
Abstract

The gradual relocation of part of the energy-intensive industries (EIIs) outside of Europe is one of the possible consequences of the combination of emission charges and higher electricity prices entailed by the EU-Emission Trading System (EU-ETS). The geographical distribution of cement plants is a relevant factor in relocation decisions because cement sector is characterized by high transportation costs. In order to mitigate this effect, EIIs have asked for CO$_2$ allowance grandfathering and long-term power contracts whereby they would be supplied from dedicated power capacities at a lower price. We model this situation on a prototype cement international market calibrated on ETS regulated and unregulated countries, with a particular focus on the Italian market. The analysis is based on an oligopolistic partial equilibrium model with a detailed technological representation of the whole production process. The model is a Generalized Nash game that accounts for the interactions of cement companies. In particular, we investigate the role played by the transportation costs in the clinker/cement production relocation and evaluates the effectiveness of CO$_2$ allowance grandfathering and of the application of long-term power contracts in mitigating this phenomenon. To this aim, we conduct empirical experiments taking into account different transportation costs and progressively higher CO$_2$ allowance prices with and without long-term contracts. Our results show that the European and Italian cement markets are affected by the EU-ETS and react by importing clinker from unregulated regions. Both allowance grandfathering and long-term power contracts only partially mitigate this relocation phenomenon.

Appendices

Appendix 1: Mathematical structure

Let X be a nonempty, closed and convex subset of the n-dimensional Euclidean space $\mathbb R^n$ and $F:X\rightarrow \mathbb R^n$ a continuous mapping. The variational inequality problem (VI for short) is the problem of finding a point $x^*\in X$ such that

$$\begin{aligned} F(x^{*})^\top (x-x^*) \ge 0, \ \ \ \forall \ x\in X, \end{aligned}$$

(18)

where we denote by $F(x^*)^{\top }$ the transpose of $F(x^{*})$.²⁶ The solution set of VI (18) is denoted by SOL(X, F).

Most existence results of solutions for VIs are proved by using various fixed point theorems. For instance, it is well known that, as a consequence of Brouwer fixed point theorem, VI (18) has a solution if X is compact and F is continuous.

Theorem 1

(Hartman and Stampacchia 1966) If X is a nonempty, compact and convex set and F is continuous on X, then VI (18) admits at least one solution.

In general, a VI can have more than one solution. Theorem 3 below recalls a condition under which VI (18) has a unique solution; this result needs a generalized monotonicity assumption.

Definition 1

Let X be a convex set in ${\mathbb {R}}^{n}$. A mapping $F:X\subseteq {\mathbb {R}}^{n}\rightarrow {\mathbb {R}}^{n}$ is said to be

• $\mathrm {monotone}$ on X if $(F(x)-F(y))^\top (x-y)\geqslant 0$, $\forall x,y\in X$;

• $\mathrm {strictly \ monotone}$ on X if $(F(x)-F(y))^\top (x-y)>0$, $\forall x,y\in X$ and $x\ne y$.

For a continuously differentiable mapping $F:X\rightarrow \mathbb {R}^{n}$, we recall the following well-known monotonicity criteria, where $\nabla F$ denotes the matrix that has as its i-th column the gradient of $F_i$, $\nabla F_i$.

Theorem 2

(Ortega and Rheinboldt 1970) Let X be an open convex set in ${\mathbb {R}}^n$ and let $F:X\subseteq {\mathbb {R}}^{n}\rightarrow {\mathbb {R}}^{n}$ be continuously differentiable on X.

• F is monotone on X if and only if $\nabla F$ is positive semidefinite on X;

• F is strictly monotone on X if $\nabla F$ is positive definite on X.

Under assumptions of monotonicity of F and compactness of the set X, we can establish existence and uniqueness of the solutions of a VI.

Theorem 3

(Harker and Pang 1990) If F is strictly monotone, then VI (18) has at most one solution.

VIs are closely related to many problems of Nonlinear Analysis, such as complementarity, fixed point and optimization problems. A complementarity problem (CP) is problem (18) in the case where X is a cone. In particular, when $X= {\mathbb {R}}_+^n$, the non-negative orthant of ${\mathbb {R}}^{n}$, the CP is the problem of finding a point $x^{*}$ such that:

$$\begin{aligned} 0\le x^* \ \bot \ F(x^*) \ge 0, \end{aligned}$$

(19)

where $F: {\mathbb {R}}_+^{n} \rightarrow {\mathbb {R}}^{n}$. We recall that condition (19) can be explicitly written as:

$$\begin{aligned} x^{*} \ge 0, \quad F(x^{*})\ge 0, \quad F(x^{*})^{\top } x^{*}=0. \end{aligned}$$

(20)

Let us now consider a Generalized Nash Equilibrium Problem (GNEP) with N players. Let $x^{\nu }\in {\mathbb {R}}^{n_{\nu }}$, $\nu = 1,\dots ,N$, denote the variables controlled by player $\nu$ and ${\mathbf {x}}=\left( (x^1)^{\top },(x^{2})^{\top },\dots , (x^{N})^{\top }\right) ^{\top }$ be the vector of all decision variables (see Facchinei and Kanzow 2007 for a complete review on the topic). Let us also denote by ${\mathbf {x}}^{-\nu }$ the vector formed by all players’ decision variables except those of player $\nu$. The problem of player $\nu \in \{1,\dots ,N\}$, given the other players’ strategies, is to solve:

$$\begin{aligned} \hbox {min}_{x^{\nu }}\ f_{v}(x^{\nu }, {\mathbf {x}}^{-\nu })\nonumber \\ \hbox { sub to}\ x^{\nu }\in X_{\nu }({\mathbf {x}}^{-\nu }) \end{aligned}$$

(21)

where $f_{v}$ is the cost function of player $\nu$ and $X_{\nu }({\mathbf {x}}^{-\nu })\in {\mathbb {R}}^{n_{\nu }}$ is the set of strategies depending on the rival players’ strategies, ${{\mathbf {x}}}^{-\nu }$. For any ${{\mathbf {x}}}^{-\nu }$, the solution set of problem (21) is denoted by ${\mathcal {S}}_{\nu }({{\mathbf {x}}}^{-\nu })$. The GNEP can be defined as follows.

Definition 2

The GNEP is the problem of finding a vector $\bar{\mathbf {x}}$ such that

$$\begin{aligned} {\bar{x}^{\nu }} \in \mathcal {S}_{\nu }(\bar{\mathbf {x}}^{-\nu }), \qquad \forall \nu \in \{1,\dots ,N\}. \end{aligned}$$

The GNEP can be interpreted as the problem of finding a vector of equilibrium strategies for all players $\nu = 1,\dots ,N$, where the feasible region of each player is defined by two sets of constraints: the set of constraints only depending on decision variables of player $\nu$ and the set of common constraints. In electricity markets, for instance, common constraints arise in modeling transmission lines capacities. Equilibria of GNEPs, however, are extremely difficult to compute. A particular case is that of jointly convex common constraints. We recall that jointly convex common constraints mean that the (convex) feasible sets of all players still depend on the rivals’ strategies, but are the same for all players. More precisely, we assume that there is a common strategy space ${\mathbf {X}} \subseteq {\mathbb {R}}^n$, $n = \sum _\nu n_\nu$ such that the feasible set of player $\nu =1,\dots , N$ is given by

$$\begin{aligned} X_{\nu }({\mathbf {x}}^{-\nu })=\{x^{\nu }:(x^{\nu },{\mathbf {x}}^{-\nu })\in \mathbf {X}\} \end{aligned}$$

(22)

Theoretical results show that, under the assumption of jointly convex constraints, there exist equilibria of GNEPs that are also solutions to particular Variational Inequalities (VIs) and viceversa.

The following theorem (see Facchinei et al. 2007) highlights the relationship between the solution of a VI and the solutions of a GNEP. Recall that a function $f: {\mathbb {R}}^{p} \rightarrow {\mathbb {R}}$ is called pseudo-convex on a set $K \subseteq \mathbb R^p$ if there exists an open superset A of K such that f is continuously differentiable on A and, for all $x,y \in A$,

$$\begin{aligned} \nabla f(x)^\top (y-x) \ge 0 \quad \Longrightarrow \quad f(y) \ge f(x). \end{aligned}$$

Theorem 4

(Facchinei et al. 2007) Let us suppose that the GNEP satisfies the following assumptions for all $\nu = 1,\dots ,N$:

• i) for every player $\nu \in N$, $f_{\nu }$ is continuously differentiable in ${\mathbf {x}}$;

• ii) for every player $\nu \in N$, $f_{\nu }$, the function $f_{\nu }(\cdot , {\mathbf {x}}^{-\nu })$ is pseudo-convex in $x^{\nu }$;

• iii) the feasible set of player $\nu$ can be written as $X_{\nu }({\mathbf {x}}^{-\nu })=\{x^{\nu }:(x^{\nu },{\mathbf {x}}^{-\nu })\in \mathbf {X}\}$, with $\mathbf {X}$ closed and convex.

Then, every solution of the VI$({\mathbf {X}}, {\mathbf {F}})$ is a solution of the GNEP, where ${\mathbf {F}}:{\mathbb {R}}^{n} \rightarrow {\mathbb {R}}^{n}$ is defined as follows:

$$\begin{aligned} {\mathbf {F}}(x)=\left( \nabla _{x^1}f_1({\mathbf {x}})^\top , \nabla _{x^2}f_2({\mathbf {x}})^\top ,\dots ,\nabla _{x^N}f_N({\mathbf {x}})^\top \right) ^\top . \end{aligned}$$

Theorem 4 states that a solution of a VI is also a solution of a GNEP. In this light, existence results for the solutions of a VI also ensure existence of equilibria in the GNEP. An existence result for a GNEP complying with the set-up of Theorem 4 is stated in the following result, which combines Theorems 1 and 4.

Corollary 1

Let assumptions i), ii), iii) of Theorem 4 hold and let $\mathbf {F}$ as well be defined as in Theorem 4. Furthermore, suppose that the set $\mathbf {X}$ is compact. Then a solution of VI$(\mathbf {X},\mathbf {F})$ exists and this is also a solution of the corresponding GNEP.

A tighter relation between the solution sets of VIs and GNEPs can be derived in the particular case where $\mathbf {X}$ is defined as follows:

$$\begin{aligned} {\mathbf {X}}=\{{\mathbf {x}}\in \mathbb {R}^n:g({\mathbf {x}})\le 0\}, \end{aligned}$$

(23)

with $n = \sum _{\nu = 1}^{N} n_\nu$, where $n_\nu$ is the dimension of the decision variables of player $\nu$, and $g:{\mathbb {R}}^n\rightarrow {\mathbb {R}}^m$ is such that $g_i: {\mathbb {R}}^n\rightarrow {\mathbb {R}}$ is convex and continuously differentiable for all $i=1,\dots ,m$.

Suppose also that ${\mathbf {x}}$ is a solution of the GNEP. Then the following classical Karush–Kuhn–Tucker (KKT) conditions are satisfied for each player $\nu = 1,\dots ,N$:

$$\begin{aligned}&\nabla _{x^{\nu }}f(x^{\nu }, {\mathbf {x}}^{-\nu })+ \nabla _{x^{\nu }}g(x^{\nu }, {\mathbf {x}}^{-\nu })\lambda ^{\nu }=0,\qquad 0\le \lambda ^{\nu }\bot g(x^{\nu }, {\mathbf {x}}^{-\nu })\le 0, \qquad \quad \end{aligned}$$

(24)

where $\lambda ^{\nu } \in {\mathbb {R}}^m$ is a vector of multipliers. The KKT conditions for VI$(\mathbf {X},\mathbf {F})$ are the following:

$$\begin{aligned}&\mathbf {F}({\mathbf {x}})+ \nabla _{{\mathbf {x}}}g({\mathbf {x}})\lambda =0,\qquad 0\le \lambda \bot g({\mathbf {x}})\le 0, \end{aligned}$$

(25)

where $\lambda \in {\mathbb {R}}^m$ is a vector of multipliers.

The following theorem, among the solutions of a GNEP, characterizes those that are also VI’s solutions.

Theorem 5

(Facchinei et al. 2007) Let us suppose that the GNEP satisfies assumptions i), ii) and iii) of Theorem 4 and the set ${\mathbf {X}}$ is given by (23). Vector ${\mathbf {x}}$ is a solution of VI$({\mathbf {X}},{\mathbf {F}})$ at which the KKT conditions (25) hold if and only if ${\mathbf {x}}$ is a solution of the GNEP at which KKT conditions (24) hold with $\lambda ^1=\dots =\lambda ^N=\lambda$.

Appendix 2: Notation: sets, parameters and variables of the model

In this appendix we list the sets, the parameters and the variables used in our models.

Sets

J :

Set of cement companies operating in the market.

I :

Set of zones in which the market is divided. We define $I = I_{{\textit{ETS}}}\cup I_{{\textit{NETS}}}$, where $I_{{\textit{ETS}}}$ includes all zones subject to the EU-ETS while $I_{{\textit{NETS}}}$ indicates those zones without environmental regulation. Each zone $i\in I$ can be further partitioned in $\widehat{l}$ homogeneous regions that we indicate as $i_1, i_2,\dots , i_{\widehat{l}}$. We denote $Z_i=\{i_l: l\in L\}$, where $L=\{1,\dots ,\widehat{l}\}$, for all $i\in I$. For example $l=1,2$ distinguish between coastal and inland regions in each zone $i\in I$.

W :

Set of technologies used for producing clinker.

V :

Set of technologies used for producing cement.

G :

Set of fuel employed in clinker production.

$N_{j,i_l}$ :

Set of plants of company $j \in J$ located in the region $i_l \in Z_l$, where $i\in I$ and $l\in L$.

$W_n$ :

Set of clinker technologies available in plant $n \in N_{j,i_l}$.

$V_n$ :

Set of cement technologies available in plant $n \in N_{j,i_l}$.

Parameters and variables

• Clinker parameters

  $t^k_{j,i_l,h_r}$ :

  Clinker transportation cost sustained by company $j \in J$ to move clinker from region $i_l \in Z_l$ to region $h_r \in Z_h$.

  $p^{mk}_{i}$ :

  Price of the stones (limestone, chalk, marl and shale) in zone $i\in I$ used as raw material for producing clinker.

  $\gamma ^k_{g,i,w}$ :

  Proportion of fuel $g\in G$ used in clinker production in zone $i\in I$, with technology $w\in W$ (ton/ton).

  $p^f_{g,i}$ :

  Price of fuel $g \in G$ in zone i used in clinker production.

  ${\alpha _w}$ :

  Electricity consumption per tons of clinker produced with technology $w \in W$ (KWh/ton).

  ${Q}^k_{n,w}$ :

  Capacity of the kiln of technology $w \in W_n$ of plant $n \in N_{j,i_l}$.

  $p^k_i$ :

  Price of clinker for each zone $i\in I$ (euro/ton).

  $\rho _{i}$ :

  Clinker to cement ratio applied in zone $i\in I$ (%).

  $\sigma _{i}$ :

  Raw material to clinker ratio applied in zone $i\in I$ (%).

• Cement related parameters

  $t^c_{j,i_l,h_r}$ :

  Cement transportation cost sustained by company $j \in J$ to move cement from region $i_l \in Z_l$ to region $h_r \in Z_r$.

  $p^{mc}_i$ :

  Price of the material (gypsum, slag, limestone) used for producing cement in zone $i\in I$.

  ${Q}^c_{n,v}$ :

  Grinding mill capacity of technology $v \in V_n$ of plant $n \in N_{j,i_l}$.

  ${\beta _v}$ :

  Electricity consumption per tons of cement produced with technology $v \in V$ (KWh/ton).

• Other parameters

  ${p^e_i}$ :

  Average electricity price in zone $i\in I$.

  $p^{CO_2}$ :

  Allowance price (€/ton CO$_2$).

  ${GA}_{n,w}$ :

  Amount of grandfathered allowances for plant $n \in N_{j,i_l}$ with technology $w\in W_n$ (ton/year).

  $\tau _{i,w}$ :

  Average emission factor per ton of clinker produced depending on the zone $i\in I$ and technology $w \in W$.

Variables

• Clinker variables

  $q^k_{n,w}$ :

  Clinker produced by plant $n \in N_{j,i_l}$ with technology $w \in W_n$ (ton/year).

  $s^k{j,i_l,\bar{j},h_r}$ :

  Clinker produced by company $j\in J$ in region $i_l\in Z_l$ and sold to company $\bar{j}\in J, \bar{j}\ne j$, in region $h_r \in Z_h$ (ton/year).

  $b^k_{j,i_l,\bar{j},h_r}$ :

  Clinker bought by company $j\in J$ to satisfy demand in region $i_l\in Z_l$ from company $\bar{j}\in J, \bar{j}\ne j$, in region $h_r \in Z_h$ (ton/year).

  $u^k_{j,i_l}$ :

  Clinker produced by company $j\in J$ in region $i_l\in Z_l$ and used in the same region (ton/year).

  $m^k_{n}$ :

  Raw material (limestone, chalk, marl and shale) used by plant $n \in N_{j,i_l}$ to produce clinker (ton/year).

  $e^k_{n}$ :

  Electricity used by plant $n \in N_{j,i_l}$ to produce clinker (KWh/year).

  $f^k_{g,n,w}$ :

  Fuel of type $g \in G$ used by plant $n \in N_{j,i_l}$ to produce clinker with technology $w \in W_n$ (ton/year).

• Cement variables

  $q^c_{n,v}$ :

  Cement produced by plant $n \in N_{j,i_l}$ with technology $v \in V_n$ (ton/year).

  $s^c_{j,i_l,h_r}$ :

  Cement produced by company $j\in J$ in region $i_l\in Z_l$ and sold in region $h_r \in Z_h$ (ton/year).

  $e^c_{n}$ :

  Electricity used by plant $n \in N_{j,i_l}$ to produce cement (KWh/year).

  $m^c_{n}$ :

  Material (gypsum, slag, limestone) used by plant $n \in N_{j,i_l}$ to produce cement (ton/year).

Appendix 3: Complementarity version of the clinker and cement producer problem

• Clinker complementarity conditions

  $$\begin{aligned} 0\le & {} - \lambda s^k_{\widehat{j},i_l} + \sigma _i \cdot \lambda m^k_n + \alpha _w \cdot \lambda e^k_n + \gamma _{g,i,w} \cdot \lambda f_{g,n,w} \nonumber \\&+ \,\lambda Q^k_{n,w} + \tau _{i,w} \cdot p^{CO_2} \bot \ q^k_{n,w}\ge 0, \quad \forall \ \ i \in I_{{\textit{ETS}}}, \ i_l\in Z_i,\ n \in N_{\widehat{j},i_l}, w \in W_n \end{aligned}$$

  (26)
  $$\begin{aligned} 0\le & {} - \lambda s^k_{\widehat{j},i_l} + \sigma _i \cdot \lambda m^k_n + \alpha _w \cdot \lambda e^k_n + \gamma _{g,i,w} \cdot \lambda f_{g,n,w} \nonumber \\&+\, \lambda Q^k_{n,w} \bot \ q^k_{n,w}\ge 0, \quad \forall \ \ i \in I_{{\textit{NETS}}}, \ i_l\in Z_i,\ n \in N_{\widehat{j},i_l}, w \in W_n \end{aligned}$$

  (27)
  $$\begin{aligned} 0\le & {} -p^k_h{ +} \lambda sb^k_{\widehat{j},i_l,j,h_r}+\lambda s^k_{\widehat{j},i_l} \ \bot \ s^k_{\widehat{j},i_l,j,h_r}\ge 0, \forall i,h \in I, \ i_l\in Z_i, \ h_r\in Z_h, \ j\in J, \ j\ne \widehat{j}\nonumber \\ \end{aligned}$$

  (28)
  $$\begin{aligned} 0\le & {} { \lambda q^k_{i_l}} +\lambda s^k_{\widehat{j},i_l} - { \lambda b^k_{\widehat{j}}} \ \bot \ u^k_{\widehat{j},i_l}\ge 0,\qquad \forall \ \ i \in I, \ i_l\in Z_i \end{aligned}$$

  (29)
  $$\begin{aligned} 0\le & {} \lambda q^k_{i_l}+p^k_i+t^k_{\widehat{j},h_r,i_l} - \lambda bs^k_{j,h_r,\widehat{j},i_l}-\lambda b^k_{\widehat{j}}\ \bot \ b^k_{\widehat{j},i_l,j,h_r}\ge 0,\quad \nonumber \\&\forall \ i\in I,h\in I, \ i_l\in Z_i,\ h_r\in Z_h, \ j\in J, j\ne \widehat{j} \end{aligned}$$

  (30)
  $$\begin{aligned} 0\le & {} p^{mk}_i-\lambda m^k_{n}\ \bot \ m^k_{n}\ge 0,\quad \forall \ i \in I, \ i_l\in Z_i,\ n \in N_{\widehat{j},i_l} \end{aligned}$$

  (31)
  $$\begin{aligned} 0\le & {} p^e_i -\lambda e^k_{n} \ \bot \ e^k_{n}\ge 0,\quad \forall \ i \in I, \ i_l\in Z_i,\ n \in N_{\widehat{j},i_l} \end{aligned}$$

  (32)
  $$\begin{aligned} 0\le & {} p^f_{g,i}-\lambda f_{g,n,w}\ \bot \ f_{g,n,w}\ge 0,\quad \forall \ i\in I, \ i_l\in Z_i, \ n \in N_{\widehat{j},i_l},\ w\in W_n,\ g\in G \end{aligned}$$

  (33)
  $$\begin{aligned} 0\le & {} Q^k_{n,w} - q^k_{n,w} \ \bot \ \lambda Q^k_{n,w}\ge 0, \quad \forall \ i\in I,\ i_l\in Z_i, \ n \in N_{\widehat{j},i_l},\ w\in W_n \end{aligned}$$

  (34)

• Cement complementarity conditions

  $$\begin{aligned}&0\le -\lambda q^c_{\widehat{j},i_l}+\rho _i \cdot \lambda b^k_{\widehat{j}}+(1-\rho _i)\cdot \lambda m^c_{n}+\beta _v\cdot \lambda e^c_{n}\nonumber \\&\quad -0.8\cdot \lambda q^k_{i_l}+\lambda Q^c_{n,v}\ \bot \ q^c_{n,v} \ge 0,\quad \forall \ i\in I, \ i_l\in Z_i, \ n \in N_{\widehat{j},i_l}, \ v\in V_n \end{aligned}$$

  (35)
  $$\begin{aligned}&0\le p^m_i -\lambda m^k_{n}\ \bot \ m^k_{n}\ge 0,\quad \forall \ i\in I,\ i_l\in Z_i, \ n \in N_{\widehat{j},i_l} \end{aligned}$$

  (36)
  $$\begin{aligned}&0\le p^e_i -\lambda e^c_{n}\ \bot \ e^c_{n}\ge 0,\quad \forall \ i\in I,\ i_l\in Z_i, \ n \in N_{\widehat{j},i_l} \end{aligned}$$

  (37)
  $$\begin{aligned}&0\le -P_{h_r} { -} \frac{\partial P_{h_r}}{\partial s^c_{\widehat{j},i_l,h_r}}\cdot \displaystyle { \sum _{\begin{array}{c} d \in I,\ d_o\in Z_d,\\ h_p\in Z_h \end{array} }} s^c_{\widehat{j},d_o,h_p}+t^c_{\widehat{j},i_l,h_r} \nonumber \\&\quad +\,\lambda q^c_{\widehat{j},i_l}\bot \ { s^c_{\widehat{j},i_l,h_r}} \ge 0 \quad \forall \ i\in I,\ i_l\in Z_i, \ n \in N_{\widehat{j},i_l} \end{aligned}$$

  (38)
  $$\begin{aligned}&0 \le Q^c_{n,v} - q^c_{n,v} \ \bot \ \lambda Q^c_{n,v}\ge 0, \quad \forall \ i\in I,\ i_l\in Z_i, \ n \in N_{\widehat{j},i_l}, \ v\in V_n \end{aligned}$$

  (39)