Skip to main content
SpringerLink
Log in

Adaptive finite element approximation for steady-state Poisson-Nernst-Planck equations

  • Published:
Advances in Computational Mathematics Aims and scope Submit manuscript

Abstract

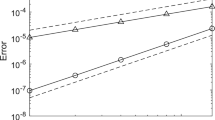
In this paper, we develop an adaptive finite element method for the nonlinear steady-state Poisson-Nernst-Planck equations, where the spatial adaptivity for geometrical singularities and boundary layer effects are mainly considered. As a key contribution, the steady-state Poisson-Nernst-Planck equations are studied systematically and rigorous analysis for a residual-based a posteriori error estimate of the nonlinear system is presented. With the regularity of the linearized system derived by taking G-derivatives of the nonlinear system, we show the robust relationship between the error of solution and the a posteriori error estimator. Numerical experiments are given to validate the efficiency of the a posteriori error estimator and demonstrate the expected rate of convergence. In further tests, adaptive mesh refinements for geometrical singularities and boundary layer effects are successfully observed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adams, R.A.: Sobolev Spaces. Academic Press, New York (1975)

    MATH  Google Scholar 

  2. Ainsworth, M., Babuška, I.: Reliable and robust a posteriori error estimation for singularly perturbed reaction-diffusion problems. SIAM J. Numer. Anal. 36(2), 331–353 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  3. Ainsworth, M., Oden, J.T.: A posteriori error estimation in finite element analysis. Wiley, New York (2000)

    Book  MATH  Google Scholar 

  4. Ainsworth, M., Vejchodskẏ, T.: Fully computable robust a posteriori error bounds for singularly perturbed reaction-diffusion problems. Numer. Math. 119(2), 219–243 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  5. Barcilon, V., Chen, D., Eisenberg, R.S., Jerome, J.W.: Qualitative properties of steady-state Poisson-Nernst-Planck systems: perturbation and simulation study. SIAM J. Appl. Math. 57(3), 631–648 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  6. Binev, P., Dahmen, W., Devore, R.: Adaptive finite element methods with convergence rates. Numer. Math. 97(2), 219–268 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bolintineanu, D.S., Sayyed-Ahmad, A., Davis, H.T., Kaznessis, Y.N.: Poisson-Nernst-Planck models of nonequilibrium ion electrodiffusion through a protegrin transmembrane pore. PLOS Comput. Biol. 5(1), e1000277 (2009)

  8. Bousquet, A., Hu, X., Metti, M.S., Xu, J.: Newton solvers for drift-diffusion and electrokinetic equations. SIAM J. Sci. Comput. 40(3), B982–B1006 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  9. Brenner, S.C., Scott, L.R.: The mathematical theory of finite element methods. Springer (1998)

  10. Brezzi, F., Capelo, A.C.S., Gastaldi, L.: A singular perturbation analysis of reverse-biased semiconductor diodes. SIAM J. Math. Anal. 20(2), 372–387 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  11. Brezzi, F., Marini, L.D., Micheletti, S., Pietra, P., Sacco, R., Wang, S.: Discretization, of semiconductor device problems. Handb. Numer. Anal. 13, 317–441 (2005)

    MathSciNet  MATH  Google Scholar 

  12. Bubuka, I., Vogelius, M.: Feedback and adaptive finite element solution of one-dimensional boundary value problems. Numer. Math. 44(1), 75–102 (1984)

    Article  MathSciNet  Google Scholar 

  13. Cárdenas, A. E., Coalson, R.D., Kurnikova, M.G.: Three-dimensional Poisson-Nernst-Planck theory studies: influence of membrane electrostatics on gramicidin a channel conductance. Biophys. J. 79(1), 80–93 (2000)

    Article  Google Scholar 

  14. Carstensen, C., Dolzmann, G.: A posteriori error estimates for mixed finite element method in elasticity. Numer. Math. 81(2), 187–209 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  15. Cheddadi, I., Fuči̇k, R., Prieto, M.I., Vohralik, M.: Guaranteed and robust a posteriori error estimates for singularly perturbed reaction-diffusion problems. ESAIM-Math. Model. Num. 43(5), 867–888 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  16. Chen, L., Holst, M., Xu, J.: The finite element approximation of the nonlinear Poisson-Boltzmann equation. SIAM J. Numer. Anal. 45(6), 2298–2320 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Ciarlet, P.: The finite element method for elliptic problems. Publishing Company, North-Holland (1978)

  18. Demlow, A., Kopteva, N.: Maximum-norm a posteriori error estimates for singularly perturbed elliptic reaction-diffusion problems. Numer. Math. 133(4), 707–742 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  19. Ding, J., Wang, Z., Zhou, S.: Positivity preserving finite difference methods for Poisson-Nernst-Planck equations with steric interactions: Application to slit-shaped nanopore conductance. J. Comput. Phys., 108864 (2019)

  20. Dione, I., Doyon, N., Deteix, J.: Sensitivity analysis of the Poisson-Nernst-Planck equations: a finite element approximation for the sensitive analysis of an electrodiffusion model. J. Math. Biol., 1–36 (2018)

  21. Dörfler, W.: A convergent adaptive algorithm for Poisson’s equation. SIAM J. Numer. Anal. 33(3), 1106–1124 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  22. Eriksson, K., Johnson, C.: Error estimates and automatic time step control for nonlinear parabolic problems, I. SIAM J. Numer. Anal. 24(1), 12–23 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  23. Gajewski, H.: On uniqueness and stability of Steady-State carrier distributions in semiconductors. Springer, Berlin (1986)

  24. Gajewski, H., Groger, K.: On the basic equations for carrier transport in semiconductors. J. Math. Anal. Appl. 113(1), 12–35 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  25. Gao, H., Sun, P.: A linearized local conservative mixed finite element method for Poisson-Nernst-Planck equations. J. Sci. Comput. 77(2), 793–817 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  26. Golovnev, A., Trimper, S.: Steady state solution of the Poisson-Nernst-Planck equations. Phys. Lett. A 374(28), 2886–2889 (2010)

    Article  MATH  Google Scholar 

  27. Golovnev, A, Trimper, S.: Analytical solution of the Poisson-Nernst-Planck equations in the linear regime at an applied dc-voltage. J. Chem. Phys. 134(15), 154902 (2011)

  28. Hayeck, N., Nachaoui, A., Nassif, N.R.: Existence and regularity for Van Roosbroeck systems with general mixed boundary conditions. Compel. 9(4), 217–228 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  29. He, M., Sun, P.: Mixed finite element analysis for the Poisson-Nernst-Planck/Stokes coupling. J. Comput. Appl. Math. 341, 61–79 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  30. Hollerbach, U., Chen, D.P., Eisenberg, R.S.: Two- and three-dimensional Poisson-Nernst-Planck simulations of current flow through gramicidin A. J. Sci. Comput. 16(4), 373–409 (2001)

    Article  MATH  Google Scholar 

  31. Holst, M., Baker, N.A., Wang, F.: Adaptive multilevel finite element solution of the Poisson-Boltzmann equation I: Algorithms and examples. J. Comput. Chem. 21(15), 1319–1342 (2000)

    Article  Google Scholar 

  32. Horng, T., Lin, T., Liu, C., Eisenberg, B.: PNP equations with steric effects: A model of ion flow through channels. J. Phys. Chem. B 116 (37), 11422–11441 (2012)

    Article  Google Scholar 

  33. Hu, J., Huang, X.: A fully discrete positivity-preserving and energy-dissipative finite difference scheme for Poisson–Nernst–Planck equations. Numer. Math., 1–39 (2020)

  34. Jasielec, J.J., Filipek, R., Szyszkiewicz, K., Fausek, J., Danielewski, M., Lewenstam, A.: Computer simulations of electrodiffusion problems based on Nernst-Planck and Poisson equations. Comp. Mater. Sci. 63(none), 75–90 (2012)

    Article  Google Scholar 

  35. Jerome, J.W.: Consistency of semiconductor modeling: An existence/stability analysis for the stationary Van Roosbroeck system. SIAM J. Appl. Math. 45(4), 565–590 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  36. Jerome, J.W., Kerkhoven, T.: A finite element approximation theory for the drift diffusion semiconductor model. SIAM J. Numer. Anal. 28(2), 403–422 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  37. Jiang, J., Cao, D., Jiang, D.E., Wu, J.: Time-dependent density functional theory for ion diffusion in electrochemical systems. J. Phys. Condens. Mat. 26(28), 284102 (2014)

  38. Kilic, M.S., Bazant, M.Z., Ajdari, A.: Steric effects in the dynamics of electrolytes at large applied voltages. II. modified Poisson-Nernst-Planck equations. Phys. Rev. E 75(2), 021503 (2007)

  39. Li, Y.: Analysis of novel adaptive two-grid finite element algorithms for linear and nonlinear problems. arXiv:1805.07887 (2018)

  40. Lions, J.L., Magenes, E.: Nonhomogeneous boundary value problems and applications. Springer, New York (1970)

    MATH  Google Scholar 

  41. Liu, H., Wang, Z.: A free energy satisfying discontinuous galerkin method for one-dimensional Poisson-Nernst-Planck systems. J. Comput. Phys. 328, 413–437 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  42. Liu, W.: Geometric singular perturbation approach to steady-state Poisson-Nernst-Planck systems. SIAM J. Appl. Math. 65(3), 754–766 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  43. Lu, B., Holst, M., Mccammon, J.A., Zhou, Y.: Poisson-Nernst-Planck, equations for simulating biomolecular diffusion-reaction processes I: Finite element solutions. J. Comput. Phys. 229(19), 6979–6994 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  44. Lu, B., Zhou, Y.: Poisson-Nernst-Planck equations for simulating biomolecular diffusion-reaction processes II: Size effects on ionic distributions and diffusion-reaction rates. Biophys. J. 100(10), 2475–2485 (2011)

    Article  Google Scholar 

  45. Lu, B., Zhou, Y., Huber, G.A., Bond, S.D., Holst, M.J., Mccammon, J.A.: Electrodiffusion: a continuum modeling framework for biomolecular systems with realistic spatiotemporal resolution. J. Chem. Phys. 127(13), 135102 (2007)

  46. Mathur, S.R., Murthy, J.Y.: A multigrid method for the Poisson-Nernst-Planck equations. Int. J. Heat. Mass. Tran. 52(17), 4031–4039 (2009)

    Article  MATH  Google Scholar 

  47. Mauri, A., Bortolossi, A., Novielli, G., Sacco, R.: 3D, finite element modeling and simulation of industrial semiconductor devices including impact ionization. J. Math. Ind. 5(1), 1 (2015)

    Article  MathSciNet  Google Scholar 

  48. Metti, M.S., Xu, J., Liu, C.: Energetically stable discretizations for charge transport and electrokinetic models. J. Comput. Phys. 306, 1–18 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  49. Mock, M.S.: Analysis of mathematical models of semiconductor devices. Boole Press, Dublin (1983)

    MATH  Google Scholar 

  50. Morin, P., Nochetto, R.H., Siebert, K.G.: Data oscillation and convergence of adaptive FEM. SIAM J. Numer. Anal. 38(2), 466–488 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  51. Schonke, J.: Unsteady analytical solutions to the Poisson-Nernst-Planck equations. J. Phys. A-Math. Theor. Math. Comp. 45(45), 455204 (2012)

  52. Singer, A., Gillespie, D., Norbury, J., Eisenberg, R.S.: Singular perturbation analysis of the steady-state Poisson-Nernst-Planck system: Applications to ion channels. Eur. J. Appl. Math. 19(5), 541–560 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  53. Song, Y., Zhang, Y., Bajaj, C.L., Baker, N.A.: Continuum diffusion reaction rate calculations of wild-type and mutant mouse acetylcholinesterase: Adaptive finite element analysis. Biophys. J. 87(3), 1558–1566 (2004)

    Article  Google Scholar 

  54. Song, Y., Zhang, Y., Shen, T., Bajaj, C.L., Mccammon, J.A., Baker, N.A.: Finite element solution of the steady-state Smoluchowski equation for rate constant calculations. Biophys. J. 86(4), 2017–2029 (2004)

    Article  Google Scholar 

  55. Tu, B., Xie, Y., Zhang, L., Lu, B.: Stabilized finite element methods to simulate the conductances of ion channels. Comput. Phys. Commun. 188, 131–139 (2015)

    Article  MATH  Google Scholar 

  56. Verfürth, R.: A posteriori error estimators for the Stokes equations. Numer. Math. 55(3), 309–325 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  57. Verfürth, R.: A posteriori error estimates for nonlinear problems. Finite element discretizations of elliptic equations. Math. Comp. 62(206), 445–475 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  58. Verfu̇rth, R.: A posteriori error estimation and adaptive mesh-refinement techniques. J. Comput. Appl. Math. 50(1C3), 67–83 (1996)

    MathSciNet  Google Scholar 

  59. Verfürth, R.: Robust a posteriori error estimators for a singularly perturbed reaction-diffusion equation. Numer. Math. 78(3), 479–493 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  60. Wei, G.W., Zheng, Q., Chen, Z., Xia, K.: Variational multiscale models for charge transport. SIAM Rev. 54(4), 699–754 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  61. Wu, J., Srinivasan, V., Xu, J., Wang, C.: Newton-Krylov-Multigrid algorithms for battery simulation. J. Electrochem. Soc. 149(10), A1342–A1348 (2002)

    Article  Google Scholar 

  62. Xie, Y., Cheng, J., Lu, B., Zhang, L.: Parallel adaptive finite element algorithms for solving the coupled electro-diffusion equations. Mol. Based Math. Biol. 1, 90–108 (2013)

    MATH  Google Scholar 

  63. Xu, J.: A new class of iterative methods for nonselfadjoint or indefinite problems. SIAM J. Numer. Anal. 29(2), 303–319 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  64. Xu, J., Zikatanov, L.: A monotone finite element scheme for convection-diffusion equations. Math. Comput. 68(228), 1429–1446 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  65. Xu, S., Chen, M., Majd, S., Yue, X., Liu, C.: Modeling and simulating asymmetrical conductance changes in gramicidin pores. Mol. Based Math. Biol. 2(1), 34–55 (2014)

    MATH  Google Scholar 

  66. Xu, Z., Ma, M., Liu, P.: Self-energy-modified Poisson-Nernst-Planck equations: WKB approximation and finite-difference approaches. Phys. Rev. E 90(1), 013307 (2014)

  67. Yang, Y., Lu, B., Xie, Y.: A decoupling two-grid method for the steady-state Poisson-Nernst-Planck equations. arXiv:1609.02277 (2016)

  68. Zeidler, E.: Nonlinear functional analysis and its applications. Springer, Berlin (1988)

    Book  MATH  Google Scholar 

  69. Zhang, B., Chen, S., Zhao, J.: Guaranteed a posteriori error estimates for nonconforming finite element approximations to a singularly perturbed reaction-diffusion problem. Appl. Numer. Math. 94, 1–15 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  70. Zhao, J., Chen, S.: Robust a posteriori error estimates for conforming discretizations of a singularly perturbed reaction-diffusion problem on anisotropic meshes. Adv. Comput. Math. 40(4), 797–818 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  71. Zheng, Q., Chen, D., Wei, G.W.: Second-order Poisson-Nernst-Planck solver for ion channel transport. J. Comput. Phys. 230(13), 5239–5262 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  72. Zhou, Y., Lu, B., Huber, G.A., Holst, M.J., McCammon, J.A.: Continuum simulations of acetylcholine consumption by acetylcholinesterase: A Poisson-Nernst-Planck approach. J. Phys. Chem. B 112(2), 270–275 (2008)

    Article  Google Scholar 

Download references

Funding

T. Hao and X. Xu received financial support from NSFC (No. 11671302). M. Ma received financial support from NSFC (No. 11701428), “Chen Guang” project supported by Shanghai Municipal Education Commission and Shanghai Education Development Foundation, and the Fundamental Research Funds for the Central Universities.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Tongji University, Shanghai, 200092, China

    Tingting Hao, Manman Ma & Xuejun Xu

  2. HUA LOOKENG Honors College, Changzhou University, Changzhou, 213164, China

    Tingting Hao

  3. LSEC, Institute of Computational Mathematics and Scientific/Engineering Computing, Academy of Mathematics and System Sciences, Chinese Academy of Sciences, Beijing, 100190, China

    Xuejun Xu

Authors
  1. Tingting Hao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Manman Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xuejun Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Manman Ma.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Communicated by: Paul Houston

Data availability

The datasets generated during and/or analysed during the current study are available from the corresponding author on reasonable request.

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hao, T., Ma, M. & Xu, X. Adaptive finite element approximation for steady-state Poisson-Nernst-Planck equations. Adv Comput Math 48, 49 (2022). https://doi.org/10.1007/s10444-022-09938-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10444-022-09938-2

Keywords

Mathematics Subject Classification (2010)

Search

Navigation