Skip to main content
SpringerLink
Log in

Accuracy and Reproducibility of Patient-Specific Hemodynamic Models of Stented Intracranial Aneurysms: Report on the Virtual Intracranial Stenting Challenge 2011

  • Published:
Annals of Biomedical Engineering Aims and scope Submit manuscript

Abstract

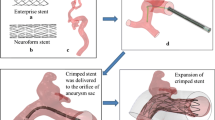
Validation studies are prerequisites for computational fluid dynamics (CFD) simulations to be accepted as part of clinical decision-making. This paper reports on the 2011 edition of the Virtual Intracranial Stenting Challenge. The challenge aimed to assess the reproducibility with which research groups can simulate the velocity field in an intracranial aneurysm, both untreated and treated with five different configurations of high-porosity stents. Particle imaging velocimetry (PIV) measurements were obtained to validate the untreated velocity field. Six participants, totaling three CFD solvers, were provided with surface meshes of the vascular geometry and the deployed stent geometries, and flow rate boundary conditions for all inlets and outlets. As output, they were invited to submit an abstract to the 8th International Interdisciplinary Cerebrovascular Symposium 2011 (ICS’11), outlining their methods and giving their interpretation of the performance of each stent configuration. After the challenge, all CFD solutions were collected and analyzed. To quantitatively analyze the data, we calculated the root-mean-square error (RMSE) over uniformly distributed nodes on a plane slicing the main flow jet along its axis and normalized it with the maximum velocity on the slice of the untreated case (NRMSE). Good agreement was found between CFD and PIV with a NRMSE of 7.28%. Excellent agreement was found between CFD solutions, both untreated and treated. The maximum difference between any two groups (along a line perpendicular to the main flow jet) was 4.0 mm/s, i.e. 4.1% of the maximum velocity of the untreated case, and the average NRMSE was 0.47% (range 0.28–1.03%). In conclusion, given geometry and flow rates, research groups can accurately simulate the velocity field inside an intracranial aneurysm—as assessed by comparison with in vitro measurements—and find excellent agreement on the hemodynamic effect of different stent configurations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
Figure 8
Figure 9
Figure 10
Figure 11

Similar content being viewed by others

Notes

  1. 10.6084/m9.figshare.1060443

  2. 10.6084/m9.figshare.1060453

  3. 10.6084/m9.figshare.1060464

  4. http://www.fing.edu.uy/imfia/caffa3d.MB

  5. http://www.fing.edu.uy/imfia/caffa3d.MB/CaffaViscTutorial.tar.gz

References

  1. Antiga, L., M. Piccinelli, L. A. Botti, B. Ene-Iordache, A. Remuzzi, and D. A. Steinman. An image-based modeling framework for patient-specific computational hemodynamics. Med. Biol. Eng. Comput. 46(11):1097–1112, 2008.

    Article  PubMed  Google Scholar 

  2. Appanaboyina, S., F. Mut, R. Löhner, C. M. Putman, and J. R. Cebral. Simulation of intracranial aneurysm stenting: techniques and challenges. Comput. Methods Appl. Mech. Eng. 198(45–46):3567–3582, 2009.

    Article  Google Scholar 

  3. Augsburger, L., P. Reymond, D. A. Rüfenacht, and N. Stergiopulos. Intracranial stents being modeled as a porous medium: flow simulation in stented cerebral aneurysms. Ann. Biomed. Eng. 39(2):850–863, 2011.

    Article  CAS  PubMed  Google Scholar 

  4. Babiker, M. H., B. Chong, L. F. Gonzalez, S. Cheema, and D. H. Frakes. Finite element modeling of embolic coil deployment: multifactor characterization of treatment effects on cerebral aneurysm hemodynamics. J. Biomech. 46(16):2809–2816, 2013.

    Article  PubMed  Google Scholar 

  5. Babiker, M. H., L. F. Gonzalez, J. Ryan, F. Albuquerque, D. Collins, A. Elvikis, and D. H. Frakes. Influence of stent configuration on cerebral aneurysm fluid dynamics. J. Biomech. 45(3):440–447, 2012.

    Article  PubMed  Google Scholar 

  6. Bernardini, A., I. Larrabide, H. G. Morales, G. Pennati, S. Cito L. Petrini, and A. F. Frangi. Influence of different computational approaches for stent deployment on cerebral aneurysm haemodynamics. Interface Focus 1(3):338–348, 2011.

    Article  PubMed Central  PubMed  Google Scholar 

  7. Cavazzuti, M., M. Atherton, M. Collins, and G. Barozzi. Beyond the Virtual Intracranial Stenting Challenge 2007: non-Newtonian and flow pulsatility effects. J. Biomech. 43(13):2645–2647, 2010.

    Article  PubMed  Google Scholar 

  8. Cebral, J. R., F. Mut, M. Raschi, E. Scrivano, R. Ceratto, P. Lylyk, and C. M. Putman. Aneurysm rupture following treatment with flow-diverting stents: computational hemodynamics analysis of treatment. Am. J. Neuroradiol. 32(1):27–33, 2011.

    CAS  PubMed  Google Scholar 

  9. Cekirge, H. S., K. Yavuz, S. Geyik, and I. Saatci. A novel “Y” stent flow diversion technique for the endovascular treatment of bifurcation aneurysms without endosaccular coiling. Am. J. Neuroradiol. 32(7):1262–1268, 2011.

    Article  CAS  PubMed  Google Scholar 

  10. Cito, S., A. J. Geers, M. P. Arroyo, J. Pallares, A. Vernet, V. R. Palero, J. Lobera, J. Blasco, L. San-Roman, and A. F. Frangi. Virtual Intracranial Stenting Challenge 2011. 8th International Interdisciplinary Cerebrovascular Symposium, Shanghai, China, 2011.

  11. Ford, M. D., H. N. Nikolov, J. S. Milner, S. P. Lownie, E. M. Demont, W. Kalata, F. Loth, D. W. Holdsworth, and D. A. Steinman. PIV-measured vs. CFD-predicted flow dynamics in anatomically realistic cerebral aneurysm models. J. Biomech. Eng. 130(2):21015, 2008.

    Article  Google Scholar 

  12. Fu, W., Z. Gu, X. Meng, B. Chu, and A. K. Qiao. Numerical simulation of hemodynamics in stented internal carotid aneurysm based on patient-specific model. J. Biomech. 43(7):1337–1342, 2010.

    Article  PubMed  Google Scholar 

  13. Geers, A. J., I. Larrabide, A. G. Radaelli, H. Bogunović, M. Kim, H. A. F. Gratama van Andel, C. B. Majoie, E. VanBavel, and A. F. Frangi. Patient-specific computational hemodynamics of intracranial aneurysms from 3D rotational angiography and CT angiography: an in vivo reproducibility study. Am. J. Neuroradiol. 32(3):581–586, 2011.

    Article  CAS  PubMed  Google Scholar 

  14. Grinberg L., and G. E. Karniadakis. Outflow boundary conditions for arterial networks with multiple outlets. Ann. Biomed. Eng. 36(9):1496–1514, 2008.

    Article  PubMed  Google Scholar 

  15. Hoi, Y., S. H. Woodward, M. Kim, D. B. Taulbee, and H. Meng. Validation of CFD simulations of cerebral aneurysms with implication of geometric variations. J. Biomech. Eng. 128(6):844–851, 2006.

    Article  PubMed Central  PubMed  Google Scholar 

  16. Hunter, J. D. Matplotlib: a 2D graphics environment. Comput. Sci. Eng. 9(3):90–95, 2007.

    Article  Google Scholar 

  17. Izar, B., A. Rai, K. Raghuram, J. Rotruck, and J. Carpenter. Comparison of devices used for stent-assisted coiling of intracranial aneurysms. PLOS ONE 6(9):e24875, 2011.

    Article  CAS  PubMed Central  PubMed  Google Scholar 

  18. Janiga, G., C. Rössl, M. Skalej, and D. Thévenin. Realistic virtual intracranial stenting and computational fluid dynamics for treatment analysis. J. Biomech. 46(1):7–12, 2013.

    Article  PubMed  Google Scholar 

  19. Kakalis, N. M., A. P. Mitsos, J. V. Byrne, and Y. P. Ventikos. The haemodynamics of endovascular aneurysm treatment: a computational modelling approach for estimating the influence of multiple coil deployment. IEEE Trans. Med. Imaging 27(6):814–824, 2008.

    Article  PubMed  Google Scholar 

  20. Karmonik, C., C. Yen, O. Diaz, R. Klucznik, R. G. Grossman, and G. Benndorf. Temporal variations of wall shear stress parameters in intracranial aneurysms-importance of patient-specific inflow waveforms for CFD calculations. Acta Neurochirurgica 152(8):1391–1398, 2010.

    Article  PubMed  Google Scholar 

  21. Kim, M., E. I. Levy, H. Meng, and L. N. Hopkins. Quantification of hemodynamic changes induced by virtual placement of multiple stents across a wide-necked basilar trunk aneurysm. Neurosurgery 61(6):1305–1312, 2007.

    Article  PubMed Central  PubMed  Google Scholar 

  22. Kono K., and T. Terada. Hemodynamics of 8 different configurations of stenting for bifurcation aneurysms. Am. J. Neuroradiol. 34(10):1980–1986, 2013.

    Article  CAS  PubMed  Google Scholar 

  23. Larrabide, I., M. L. Aguilar, H. G. Morales, A. J. Geers, Z. Kulcsár, D. Rüfenacht, and A.F. Frangi. Intra-aneurysmal pressure and flow changes induced by flow diverters: relation to aneurysm size and shape. Am. J. Neuroradiol. 34(4):816–822, 2013.

    Article  CAS  PubMed  Google Scholar 

  24. Larrabide, I., M. Kim, L. Augsburger, M. C. Villa-Uriol, D. A. Rüfenacht, and A.F. Frangi. Fast virtual deployment of self-expandable stents: method and in vitro evaluation for intracranial aneurysmal stenting. Med. Image Anal. 16(3):721–730, 2012.

    Article  PubMed  Google Scholar 

  25. Lawson, M. F., W. C. Newman, Y. Chi, J. D. Mocco, and B. L. Hoh. Stent-associated flow remodeling causes further occlusion of incompletely coiled aneurysms. Neurosurgery 69(3):598–603, 2011.

    Article  PubMed  Google Scholar 

  26. Ma, D., J. Xiang, H. Choi, T. M. Dumont, S. K. Natarajan, A. H. Siddiquiand, and H. Meng. Enhanced aneurysmal flow diversion using a dynamic push-pull technique: an experimental and modeling study. Am. J. Neuroradiol. 2014. DOI: 10.3174/ajnr.A3933.

    Google Scholar 

  27. McGah, P. M., M. R. Levitt, M. C. Barbour, R. P. Morton, J. D. Nerva, P. D. Mourad, B. V. Ghodke, D. K.Hallam, L. N. Sekhar, L. J. Kim, and A. Aliseda. Accuracy of computational cerebral aneurysm hemodynamics using patient-specific endovascular measurements. Am. J. Neuroradiol. 42(3):503–514, 2014.

    Google Scholar 

  28. Metcalfe, R. W. The promise of computational fluid dynamics as a tool for delineating therapeutic options in the treatment of aneurysms. Am. J. Neuroradiol. 24(4):553–554, 2003.

    PubMed  Google Scholar 

  29. Morales, H. G., I. Larrabide, A. J. Geers, L. San Roman, J. Blasco, J. M. Macho, and A. F. Frangi. A virtual coiling technique for image-based aneurysm models by dynamic path planning. IEEE Trans. Med. Imaging 32(1):119–129, 2013.

    Article  PubMed  Google Scholar 

  30. Nealen, A., T. Igarashi, O. Sorkine, and M. Alexa. Laplacian mesh optimization. Proceedings of the 4th International Conference on Computer Graphics and Interactive Techniques in Australasia and Southeast Asia (GRAPHITE), Kuala Lumpur, Malaysia, 2006, pp. 381–389.

  31. Palero, V., J. Lobera, and M. Arroyo. Three-component velocity field measurement in confined liquid flows with high-speed digital image plane holography. Exp. Fluids 49(2):471–483, 2010.

    Article  Google Scholar 

  32. Radaelli, A. G., L. Augsburger, J. R. Cebral, M. Ohta, D. A. Rüfenacht, R. Balossino, G. Benndorf, D. R. Hose, A. Marzo, R. W. Metcalfe, P. Mortier, F. Mut, P. Reymond, L. Socci, B. Verhegghe, and A. F. Frangi. Reproducibility of haemodynamical simulations in a subject-specific stented aneurysm model: a report on the Virtual Intracranial Stenting Challenge 2007. J. Biomech. 41(10):2069–2081, 2008.

    Article  CAS  PubMed  Google Scholar 

  33. Raffel, M., C. E. Willert, S. T. Wereley, and J. Kompenhans. Particle image velocimetry: a practical guide, 2nd ed. Berlin: Springer, 2007.

  34. Raschi, M., F. Mut, G. Byrne, C. M. Putman, S. Tateshima, F. Viñuela, T. Tanoue, K. Tanishita, and J. R. Cebral. CFD and PIV analysis of hemodynamics in a growing intracranial aneurysm. Int. J. Numer. Methods Biomed. Eng. 28(2):214–228, 2012.

    Article  PubMed Central  PubMed  Google Scholar 

  35. Schneiders, J. J., H. A. Marquering, L. Antiga, R. Van den Berg, E. VanBavel, and C. B. Majoie. Intracranial aneurysm neck size overestimation with 3D rotational angiography: the impact on intra-aneurysmal hemodynamics simulated with computational fluid dynamics. Am. J. Neuroradiol. 34(1):121–128, 2013.

    Article  CAS  PubMed  Google Scholar 

  36. Sforza, D. M., C. M. Putman, and J. R. Cebral. Hemodynamics of cerebral aneurysms. Annu. Rev. Fluid Mech. 41:91–107, 2009.

    Article  PubMed Central  PubMed  Google Scholar 

  37. Shimogonya, Y., T. Ishikawa, Y. Imai, N. Matsuki, and T. Yamaguchi. Can temporal fluctuation in spatial wall shear stress gradient initiate a cerebral aneurysm? A proposed novel hemodynamic index, the gradient oscillatory number (GON). J. Biomech. 42(4):550–554, 2009.

    Article  PubMed  Google Scholar 

  38. Steinman, D. A., Y. Hoi, P. Fahy, L. Morris, M. T. Walsh, N. Aristokleous, A. S. Anayiotos, Y. Papaharilaou, A. Arzani, S. C. Shadden, P. Berg, G. Janiga, J. Bols, P. Segers, N. W. Bressloff, M. Cibis, F. H. Gijsen, S. Cito, J. Pallareacutes, L. D. Browne, J. A. Costelloe, A. G. Lynch, J. Degroote, J. Vierendeels, W. Fu, A. Qiao, S. Hodis, D. F. Kallmes, H. Kalsi, Q. Long, V. O. Kheyfets, E. A. Finol, K. Kono, A. M. Malek, A. Lauric, P. G. Menon, K. Pekkan, M. Esmaily Moghadam, A. L. Marsden, M. Oshima, K. Katagiri, V. Peiffer, Y. Mohamied, S. J. Sherwin, J. Schaller, L. Goubergrits, G. Usera, M. Mendina, K. Valen-Sendstad, D. F. Habets, J. Xiang, H. Meng, Y. Yu, G. E. Karniadakis, N. Shaffer, and F. Loth. Variability of computational fluid dynamics solutions for pressure and flow in a giant aneurysm: the ASME 2012 Summer Bioengineering Conference CFD challenge. J. Biomech. Eng. 135(2):021016, 2013.

  39. Steinman, D. A., and C. A. Taylor. Flow imaging and computing: large artery hemodynamics. Ann. Biomed. Eng. 33(12):1704–1709, 2005.

    Article  PubMed  Google Scholar 

  40. Tremmel, M., J. Xiang, S. K. Natarajan, L. N. Hopkins, A. H. Siddiqui, E. I. Levy, and H. Meng. Alteration of intra-aneurysmal hemodynamics for flow diversion using enterprise and vision stents. World Neurosurg. 74(716):306–315, 2010.

    Article  PubMed Central  PubMed  Google Scholar 

  41. Ugron, Á., M. Farinas, L. Kiss, and G. Paál. Unsteady velocity measurements in a realistic intracranial aneurysm model. Exp. Fluids 52(1):37–52, 2011.

  42. Usera, G., A. Vernet, and J. A. Ferré. A parallel block-structured finite volume method for flows in complex geometry with sliding interfaces. Flow Turbul. Combust. 81(3):471–495, 2008.

    Article  Google Scholar 

  43. van Ooij, P., A. Guédon, C. Poelma, J. Schneiders, M. C. M. Rutten, H. A. Marquering, C. B. Majoie, E. VanBavel, and A. J. Nederveen. Complex flow patterns in a real-size intracranial aneurysm phantom: phase contrast MRI compared with particle image velocimetry and computational fluid dynamics. NMR Biomed. 25(1):14–26, 2012.

    Article  PubMed  Google Scholar 

  44. Wootton, D. M., and D. N. Ku. Fluid mechanics of vascular systems, diseases, and thrombosis. Annu. Rev. Biomed. Eng. 1:299–329, 1999.

    Article  CAS  PubMed  Google Scholar 

  45. Zenteno, M. A., J. A. Santos-Franco, J. M. Freitas-Modenesi, C. Gómez, L. Murillo-Bonilla, Y. Aburto-Murrieta, R. Díaz-Romero, E. Nathal, S. Gómez-Llata, and A. Lee. Use of the sole stenting technique for the management of aneurysms in the posterior circulation in a prospective series of 20 patients. J. Neurosurg. 108(6):1104–18, 2008.

    Article  PubMed  Google Scholar 

Download references

Acknowledgments

S. Cito, J. Pallares and A. Vernet received funding from projects DPI2010-17212 and CTQ2013-46799-C2-1-P of the Spanish Ministerio de Economía y Competitividad, S. Cito, A.J. Geers and A.F. Frangi received funding through the Spanish project cvREMOD (CEN-20091044, funded by the CENIT programme of the Industrial and Technological Development Center) and A. Qiao received funding through the National Natural Science Foundation of China (81171107).

Conflict of interest

None of the authors in this work has conflict of interests with other people and organizations.

Author information

Authors and Affiliations

  1. University of Helsinki, Helsinki, Finland

    S. Cito

  2. Universitat Rovira i Virgili, Tarragona, Spain

    S. Cito, J. Pallarés & A. Vernet

  3. Universitat Pompeu Fabra, Barcelona, Spain

    S. Cito, A. J. Geers & A. F. Frangi

  4. Universidad de Zaragoza, Zaragoza, Spain

    M. P. Arroyo & V. R. Palero

  5. Hospital Clínic, Barcelona, Spain

    J. Blasco & L. San Román

  6. Beijing Union University, Beijing, China

    W. Fu

  7. Beijing University of Technology, Beijing, China

    A. Qiao

  8. University of Magdeburg, Magdeburg, Germany

    G. Janiga

  9. Tohoku University, Tohoku, Japan

    Y. Miura & M. Ohta

  10. Universidad de la República, Montevideo, Uruguay

    M. Mendina & G. Usera

  11. University of Sheffield, Sheffield, UK

    A. F. Frangi

Authors
  1. S. Cito
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. J. Geers
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. P. Arroyo
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. V. R. Palero
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. J. Pallarés
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. A. Vernet
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. J. Blasco
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. L. San Román
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. W. Fu
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. A. Qiao
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. G. Janiga
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. Y. Miura
    View author publications

    You can also search for this author in PubMed Google Scholar

  13. M. Ohta
    View author publications

    You can also search for this author in PubMed Google Scholar

  14. M. Mendina
    View author publications

    You can also search for this author in PubMed Google Scholar

  15. G. Usera
    View author publications

    You can also search for this author in PubMed Google Scholar

  16. A. F. Frangi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to S. Cito or A. J. Geers.

Additional information

Associate Editor Diego Gallo oversaw the review of this article.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cito, S., Geers, A.J., Arroyo, M.P. et al. Accuracy and Reproducibility of Patient-Specific Hemodynamic Models of Stented Intracranial Aneurysms: Report on the Virtual Intracranial Stenting Challenge 2011. Ann Biomed Eng 43, 154–167 (2015). https://doi.org/10.1007/s10439-014-1082-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10439-014-1082-9

Keywords

Search

Navigation