Symplectic perturbation series methodology for non-conservative linear Hamiltonian system with damping

Abstract

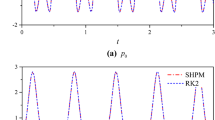
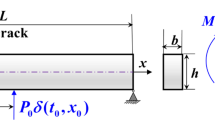
In this paper, the symplectic perturbation series methodology of the non-conservative linear Hamiltonian system is presented for the structural dynamic response with damping. Firstly, the linear Hamiltonian system is briefly introduced and its conservation law is proved based on the properties of the exterior products. Then the symplectic perturbation series methodology is proposed to deal with the non-conservative linear Hamiltonian system and its conservation law is further proved. The structural dynamic response problem with eternal load and damping is transformed as the non-conservative linear Hamiltonian system and the symplectic difference schemes for the non-conservative linear Hamiltonian system are established. The applicability and validity of the proposed method are demonstrated by three engineering examples. The results demonstrate that the presented methodology is better than the traditional Runge–Kutta algorithm in the prediction of long-time structural dynamic response under the same time step.

Appendices

Appendix A: Conservation law of linear Hamiltonian System

The symplectic structure of the Hamiltonian system can be written as

$$ \omega = \sum\limits_{i = 1}^{n} {\text{d}z_{i} \wedge \text{d}z_{n + i} } , $$

(A.1)

where the notation “$\wedge$” denotes the exterior product. The matrix form of Eq. (A.1) is expressed as follows:

$$ \omega = \left( {\begin{array}{*{20}c} {\text{d}z_{1} } \\ \vdots \\ {\text{d}z_{n} } \\ \end{array} } \right)^{\text{T}} \wedge \left( {\begin{array}{*{20}c} {\text{d}z_{n + 1} } \\ \vdots \\ {\text{d}z_{2n} } \\ \end{array} } \right). $$

(A.2)

Considering $\text{d}z_{j} \wedge \text{d}z_{k} = - \text{d}z_{k} \wedge \text{d}z_{j} ,(1 \le j \le n,n + 1 \le k \le 2n)$, Eq. (A.2) is equivalent to

$$ \omega = \frac{1}{2}\left( {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\text{d}z_{1} } \\ \vdots \\ {\text{d}z_{n} } \\ \end{array} } \\ {\text{d}z_{n + 1} } \\ \vdots \\ {\text{d}z_{2n} } \\ \end{array} } \right)^{\text{T}} \wedge \left( {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\text{d}z_{n + 1} } \\ \vdots \\ {\text{d}z_{2n} } \\ \end{array} } \\ { - \text{d}z_{1} } \\ \vdots \\ { - \text{d}z_{n} } \\ \end{array} } \right).$$

(A.3)

Equation (A.3) can be rewritten as

$$ \omega = \frac{1}{2}\left( {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\text{d}z_{1} } \\ \vdots \\ {\text{d}z_{n} } \\ \end{array} } \\ {\text{d}z_{n + 1} } \\ \vdots \\ {\text{d}z_{2n} } \\ \end{array} } \right)^{\text{T}} \wedge \left( {\begin{array}{*{20}c} {\mathbf{0}} & {{\varvec{I}}_{n} } \\ { - {\varvec{I}}_{n} } & {\mathbf{0}} \\ \end{array} } \right)\left( {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\text{d}z_{1} } \\ \vdots \\ {\text{d}z_{n} } \\ \end{array} } \\ {\text{d}z_{n + 1} } \\ \vdots \\ {\text{d}z_{2n} } \\ \end{array} } \right) = \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}\user2{.} $$

(A.4)

Let

$$ {\varvec{z}}_{t} = \frac{{\text{d}{\varvec{z}}}}{{\text{d}t}}, $$

(A.5)

then the differentiation of $\omega$ to $t$ is

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{{\text{d}\left[ {\frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}} \right]}}{{\text{d}t}}. $$

(A.6)

Based on the property of the differential form, Eq. (A.6) can be rewritten as

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{1}{2}\left( {\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}} + \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}_{t} . $$

(A.7)

Considering the right multiplication of matrix ${\varvec{J}}$ only turns the position as well as the symbols of vector elements and the product of two negative unities make one positive unity, we can have

$$ \left( {\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}} = \left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge {\varvec{J}} \cdot {\varvec{J}}\text{d}{\varvec{z}}{.} $$

(A.8)

Substituting Eq. (A.8) into Eq. (A.7) yields

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{1}{2}\left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge {\varvec{J}} \cdot {\varvec{J}}\text{d}{\varvec{z}} + \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}_{t} . $$

(A.9)

Considering ${\varvec{J}} \cdot {\varvec{J}} = - {\varvec{J}}^{ - 1} \cdot \user2{J = } - {\varvec{I}}$, we have

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{1}{2}\left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge \left( { - \text{d}{\varvec{z}}} \right) + \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}_{t} . $$

(A.10)

As $\left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right)^{\text{T}} \wedge \left( { - \text{d}{\varvec{z}}} \right) = \left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge \left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right)$, Eq. (A.10) can be transformed as

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge \left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right) + \frac{1}{2}\left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge {\varvec{J}}\text{d}{\varvec{z}}_{t} = \left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge \left( {{\varvec{J}}\text{d}{\varvec{z}}_{t} } \right). $$

(A.11)

For a linear Hamiltonian system, we can obtain

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \left( {\text{d}{\varvec{z}}} \right)^{\text{T}} \wedge \left[ {{\varvec{J}}\text{d}\left( {{\varvec{Bz}}} \right)} \right] = \left( {\text{d}{\varvec{z}}} \right)^{{\text{T}}} \wedge {\varvec{JB}}\text{d}{\varvec{z}} = \left( {\text{d}{\varvec{z}}} \right)^{{\text{T}}} \wedge {\varvec{C}}\text{d}{\varvec{z}}{.} $$

(A.12)

Let $C = \left( {C_{jk} } \right)_{2n \times 2n}$, then Eq. (A.12) can be determined by

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \sum\limits_{1 \le j,k \le 2n} {C_{jk} {\text{d}}z^{j} \wedge {\text{d}}z^{k} } . $$

(A.13)

Considering ${\text{d}}z^{j} \wedge {\text{d}}z^{k} = 0 \,(j = k)$, Eq. (A.13) can be rewritten as

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \sum\limits_{1 \le j,k \le 2n,j \ne k} {C_{jk} \text{d}z^{j} \wedge \text{d}z^{k} } . $$

(A.14)

As $C_{jk} = C_{kj} \,(k \ne j)$, we have

$$ C_{jk} \text{d}z^{j} \wedge \text{d}z^{k} + C_{kj} \text{d}z^{k} \wedge \text{d}z^{j} = C_{jk} \text{d}z^{j} \wedge \text{d}z^{k} - C_{kj} \text{d}z^{j} \wedge \text{d}z^{k} = 0 \,(k \ne j). $$

(A.15)

Substituting Eq. (A.15) into Eq. (A.14) yields

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \sum\limits_{1 \le j,k \le 2n} {C_{jk} \text{d}z^{j} \wedge \text{d}z^{k} } = 0. $$

(A.16)

Consequently, the conservation law of the linear Hamiltonian system is proved.

Appendix B: Detailed proof about that a non-infinitesimal symplectic matrix can be approximately denoted by a linear combination of an infinite series of infinitesimal symplectic matrices

Firstly, we introduce a linear function, namely

$$ f(x) = \left( {{\text{e}}^{{\alpha_{1} - \varepsilon x}} - \beta_{1} } \right){\varvec{B}}^{*} + \left( {{\text{e}}^{{\varepsilon x/n - \alpha_{2} }} - \beta_{2} } \right)\Delta \user2{B,} $$

(B.1)

where ${\varvec{B}}^{*}$ is an infinitesimal symplectic matrix while $\Delta {\varvec{B}}$ is a non infinitesimal symplectic matrix. The parameters $\alpha_{1} ,\beta_{1} ,\alpha_{2} ,\beta_{2}$ satisfy the following condition:

$$ \begin{gathered} {\text{e}}^{{\alpha_{1} }} - \beta_{1} = 1,{\text{e}}^{{\alpha_{1} - \varepsilon }} - \beta_{1} = 0 \Rightarrow \alpha_{1} = - \ln \left( {1 - {\text{e}}^{ - \varepsilon } } \right),\beta_{1} = {{{\text{e}}^{ - \varepsilon } } \mathord{\left/ {\vphantom {{{\text{e}}^{ - \varepsilon } } {(1 - {\text{e}}^{ - \varepsilon } )}}} \right. \kern-\nulldelimiterspace} {(1 - {\text{e}}^{ - \varepsilon } )}}, \hfill \\ {\text{e}}^{{ - \alpha_{2} }} - \beta_{2} = 0,{\text{e}}^{{\varepsilon /n - \alpha_{2} }} - \beta_{2} = 1 \Rightarrow \alpha_{2} = \ln \left( {{\text{e}}^{\varepsilon /n} - 1} \right),\beta_{2} = {1 \mathord{\left/ {\vphantom {1 {\left( {{\text{e}}^{\varepsilon /n} - 1} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {{\text{e}}^{\varepsilon /n} - 1} \right)}}. \hfill \\ \end{gathered} $$

(B.2)

Hence, we can obtain

$$ f(0) = {\varvec{B}}^{*},\;f\;(1) = \Delta {\varvec{B}}\user2{.} $$

(B.3)

Based on the Taylor series expansion theorem, we can obtain

$$ \begin{gathered} f(1) = f(0) + f^{(1)} (0)\left( {1 - 0} \right) + \frac{1}{2}f^{(2)} (0)\left( {1 - 0} \right)^{2} + \cdots + \frac{{f^{(k)} (0)}}{k!}\left( {1 - 0} \right)^{k} + \cdots \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} = f(0) + f^{(1)} (0) + \frac{1}{2}f^{(2)} (0) + \cdots + \frac{{f^{(k)} (0)}}{k!} + \cdots, \hfill \\ \end{gathered} $$

(B.4)

where $f^{(k)} (0)$ represents the k-th derivative of function $f$. Calculating $f^{(k)} (0)$ yields:

$$ f^{(k)} (0) = \left( { - \varepsilon } \right)^{k} {\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \left( {\frac{\varepsilon }{n}} \right)^{k} {\text{e}}^{{ - \alpha_{2} }} \Delta {\varvec{B}}\text{ = }\varepsilon^{k} \left[ {\left( { - 1} \right)^{k} {\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \left( \frac{1}{n} \right)^{k} {\text{e}}^{{ - \alpha_{2} }} \Delta {\varvec{B}}} \right]. $$

(B.5)

By choosing a large value of parameter $n$ we can obtain

$$ \left( { - 1} \right)^{k} {\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \left( \frac{1}{n} \right)^{k} {\text{e}}^{{ - \alpha_{2} }} \Delta {\varvec{B}} \approx \left( { - 1} \right)^{k} {\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} . $$

(B.6)

Therefore, we can obtain

$$ f^{(k)} (0) \approx \varepsilon^{k} \left( { - 1} \right)^{k} {\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} . $$

(B.7)

Substituting Eq. (B.7) into Eq. (B.4) yields

$$ f(1) = \Delta {\varvec{B}} \approx {\varvec{B}}^{*} + ( - \varepsilon ){\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \frac{{\varepsilon^{2} }}{2!}{\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \cdots + \frac{{\left( { - \varepsilon } \right)^{k} }}{k!}{\text{e}}^{{\alpha_{1} }} {\varvec{B}}^{*} + \cdots . $$

(B.8)

Consequently, a non-infinitesimal symplectic matrix is approximately denoted by a linear combination of an infinite series of infinitesimal symplectic matrices.

Appendix C: Conservation law of non-conservative linear Hamiltonian system

The symplectic structure of the non-conservative Hamiltonian system is expressed by

$$ \omega = \frac{1}{2}\text{d}\left( {{\varvec{z}}_{0} + \varepsilon {\varvec{z}}_{1} + \varepsilon^{2} {\varvec{z}}_{2} + \cdots + \varepsilon^{n} {\varvec{z}}_{n} + \cdots } \right)^{\text{T}} \wedge {\varvec{J}}\text{d}\left( {{\varvec{z}}_{0} + \varepsilon {\varvec{z}}_{1} + \varepsilon^{2} {\varvec{z}}_{2} + \cdots + \varepsilon^{n} {\varvec{z}}_{n} + \cdots } \right). $$

(C.1)

Expanding Eq. (C.1) yields

$$ \omega = \frac{1}{2}\sum\limits_{k,l = 0}^{\infty } {\varepsilon^{k + l} \left( {{\text{d}}{\varvec{z}}_{k} } \right)^{{\text{T}}} \wedge {\varvec{J}}\text{d}{\varvec{z}}_{l} } . $$

(C.2)

Expanding $\omega$ as power series yields

$$ \omega = \sum\limits_{i = 0}^{\infty } {\varepsilon^{i} \omega_{i} } . $$

(C.3)

Based on Eq. (C.2) and Eq. (C.3) we can have

$$ \begin{gathered} \varepsilon^{0} :\omega_{0} = \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} {\varvec{J}}{\text{d}}{\varvec{z}}_{0}, \hfill \\ \varepsilon^{1} :\omega_{1} = \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{1} + \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{1} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{0}, \hfill \\ \varepsilon^{2} :\omega_{2} = \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{2} + \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{1} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{1} + \frac{1}{2}\left( {{\text{d}}{\varvec{z}}_{2} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{0}, \hfill \\ \ldots \hfill \\ \varepsilon^{n} :\omega_{n} = \frac{1}{2}\sum\limits_{i = 0}^{n} {\left( {{\text{d}}{\varvec{z}}_{i} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{n - i} }, \hfill \\ \cdots \hfill \\ \end{gathered} $$

(C.4)

The differentiation of $\omega$ to $t$ is expressed by

$$ \frac{{\text{d}\omega }}{{\text{d}t}} = \frac{{\text{d}\left( {\sum\limits_{i = 0}^{\infty } {\varepsilon^{i} \omega_{i} } } \right)}}{{\text{d}t}} = \sum\limits_{i = 0}^{\infty } {\varepsilon^{i} \frac{{\text{d}\omega_{i} }}{{\text{d}t}}} , $$

(C.5)

where

$$ \frac{{\text{d}\omega_{i} }}{{\text{d}t}} = \frac{1}{2}\frac{{\text{d}\left[ {\sum\limits_{j = 0}^{i} {\left( {{\text{d}}{\varvec{z}}_{j} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{i - j} } } \right]}}{{\text{d}t}}. $$

(C.6)

Let

$$ {\varvec{z}}_{t}^{i} = \frac{{\text{d}{\varvec{z}}^{i} }}{{\text{d}t}}, $$

(C.7)

then Eq. (C.6) can be transformed as follows:

$$ \frac{{\text{d}\omega_{i} }}{{\text{d}t}} = \sum\limits_{j = 0}^{i} {\left( {{\text{d}}{\varvec{z}}_{j} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{t}^{{_{i - j} }} } . $$

(C.8)

For $i = 0,$ it gives

$$ \frac{{\text{d}\omega_{0} }}{{\text{d}t}} = \left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{t}^{{_{0} }} . $$

(C.9)

As ${\varvec{z}}_{0}$ corresponds to a linear Hamiltonian system, its conservation law is proved in Sect. 2.2, namely

$$ \frac{{\text{d}\omega_{0} }}{{\text{d}t}} = 0. $$

(C.10)

For $i = 1,$ we can have

$$ \frac{{\text{d}\omega_{1} }}{{\text{d}t}} = \left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{t}^{{_{1} }} + \left( {{\text{d}}{\varvec{z}}_{1} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}{\varvec{z}}_{t}^{{_{0} }} . $$

(C.11)

Substituting Eq. (18) into Eq. (C.11) yields

$$ \frac{{\text{d}\omega_{1} }}{{\text{d}t}} = \left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}\left( {{\varvec{B}}_{0} {\varvec{z}}_{1} + {\varvec{B}}_{1} {\varvec{z}}_{0} } \right) + \left( {{\text{d}}{\varvec{z}}_{1} } \right)^{{\text{T}}} \wedge {\varvec{J}}{\text{d}}\left( {{\varvec{B}}_{0} {\varvec{z}}_{0} } \right). $$

(C.12)

Simplifying Eq. (C.12) we can have

$$ \frac{{\text{d}\omega_{1} }}{{\text{d}t}} = \left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{JB}}_{0} {\text{d}}{\varvec{z}}_{1} { + }\left( {{\text{d}}{\varvec{z}}_{0} } \right)^{{\text{T}}} \wedge {\varvec{JB}}_{1} {\text{d}}{\varvec{z}}_{0} + \left( {{\text{d}}{\varvec{z}}_{1} } \right)^{{\text{T}}} \wedge {\varvec{JB}}_{0} {\text{d}}{\varvec{z}}_{0} . $$

(C.13)

As ${\varvec{B}}_{0}$ and ${\varvec{B}}_{1}$ are infinitesimal symplectic matrices, we can have

$$ \begin{aligned} {\varvec{JB}}_{0} = &- {\varvec{B}}_{0}^{\text{T}} {\varvec{J}} = {\varvec{B}}_{0}^{\text{T}} {\varvec{J}}^{\text{T}} = \left( {\varvec{JB}}_{0} \right)^{\text{T}}, \\ {\varvec{JB}}_{1} =& - {\varvec{B}}_{1}^{\text{T}}{\varvec{JT}} = {\varvec{B}}_{1}^{\text{T}} {\varvec{J}}^{\text{T}} = \left( {\varvec{JB}}_{1} \right)^{\text{T}}. \end{aligned} $$

(C.14)

Let ${\varvec{C}}^{0} = \left( {C_{jk}^{0} } \right)_{2n \times 2n} = {\varvec{JB}}_{0} ,{\varvec{C}}^{1} = \left( {C_{jk}^{1} } \right)_{2n \times 2n} = {\varvec{JB}}_{1}$, then ${\varvec{C}}^{0}$ and ${\varvec{C}}^{1}$ are symmetric matrices. Simplifying Eq. (C.13) yields

$$ \frac{{\text{d}\omega_{1} }}{{\text{d}t}} = \sum\limits_{1 \le j,k \le 2n} {C_{jk}^{0} {\text{d}}z_{0}^{j} \wedge {\text{d}}z_{1}^{k} } + \sum\limits_{1 \le j,k \le 2n} {C_{jk}^{1} {\text{d}}z_{0}^{j} \wedge {\text{d}}z_{0}^{k} } + \sum\limits_{1 \le j,k \le 2n} {C_{jk}^{0} {\text{d}}z_{1}^{j} \wedge {\text{d}}z_{0}^{k} } . $$

(C.15)

As ${\text{d}}z_{0}^{j} \wedge {\text{d}}z_{1}^{k} = - {\text{d}}z_{1}^{k} \wedge {\text{d}}z_{0}^{j}$ and $C_{jk}^{0} = C_{kj}^{0}$ we can have

$$ \sum\limits_{1 \le j,k \le 2n} {C_{jk}^{0} {\text{d}}z_{0}^{j} \wedge {\text{d}}z_{1}^{k} } + \sum\limits_{1 \le j,k \le 2n} {C_{kj}^{0} {\text{d}}z_{1}^{k} \wedge {\text{d}}z_{0}^{j} } = 0. $$

(C.16)

Based on Eq. (A.16) in Appendix A, we can obtain

$$ \sum\limits_{1 \le j,k \le 2n} {C_{jk}^{1} {\text{d}}z_{0}^{j} \wedge {\text{d}}z_{0}^{k} } = 0. $$

(C.17)

Substituting Eqs. (C.16) and (C.17) into Eq. (C.15) yields

$$ \frac{{\text{d}\omega_{1} }}{{\text{d}t}} = 0. $$

(C.18)

For $i = l$, we can have

$$ \frac{{\text{d}\omega_{l} }}{{\text{d}t}} = \sum\limits_{m = 0}^{l} {\sum\limits_{n = m}^{l} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} } } \wedge {\varvec{JB}}_{n - m} {\text{d}}{\varvec{z}}_{l - n} = \sum\limits_{m = 0}^{l} {\sum\limits_{n = m}^{l} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} } } \wedge C^{n - m} {\text{d}}{\varvec{z}}_{l - n} .$$

(C.19)

Simplifying Eq. (C.19) yields

$$ \frac{{\text{d}\omega_{l} }}{{\text{d}t}} = \sum\limits_{m = 0}^{l} {\sum\limits_{n = m}^{l} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} } } \wedge C^{n - m} {\text{d}}{\varvec{z}}_{l - n} = \sum\limits_{0 \le m \le n \le l}^{{}} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} \wedge C^{n - m} {\text{d}}{\varvec{z}}_{l - n} } . $$

(C.20)

Based on Eq. (C.16), we can similarly have

$$ \left( {{\text{d}}{\varvec{z}}_{m} } \right)^{\text{T}} \wedge C^{n - m} {\text{d}}{\varvec{z}}_{l - n} + \left( {{\text{d}}{\varvec{z}}_{l - n} } \right)^{{\text{T}}} \wedge C^{n - m} {\text{d}}{\varvec{z}}_{m} = 0{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} (m \ne l - n). $$

(C.21)

Substituting Eq. (C.21) into Eq. (C.20) yields

$$ \frac{{\text{d}\omega_{l} }}{{\text{d}t}} = \sum\limits_{m \le l/2}^{{}} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} \wedge C^{l - 2m} {\text{d}}{\varvec{z}}_{m} } . $$

(C.22)

Based on Eq. (A.16) in Appendix A, we can similarly have

$$ \sum\limits_{m \le l/2}^{{}} {\left( {{\text{d}}{\varvec{z}}_{m} } \right)^{{\text{T}}} \wedge C^{l - 2m} {\text{d}}{\varvec{z}}_{m} } = 0. $$

(C.23)

Therefore, we can have

$$ \frac{{{\text{d}}\omega_{2} }}{{{\text{d}}t}} = 0, \cdots ,\frac{{{\text{d}}\omega_{n} }}{{{\text{d}}t}} = 0, \cdots . $$

(C.24)

Consequently, the conservation law of the non-conservative linear Hamiltonian system is proved.