Skip to main content
SpringerLink
Log in

New methods to find solutions and analyze stability of equilibrium of nonholonomic mechanical systems

  • Research Paper
  • Published:
Acta Mechanica Sinica Aims and scope Submit manuscript

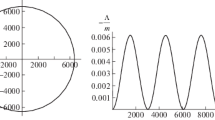
Abstract

A large proportion of constrained mechanical systems result in nonlinear ordinary differential equations, for which it is quite difficult to find analytical solutions. The initial motions method proposed by Whittaker is effective to deal with such problems for various constrained mechanical systems, including the nonholonomic systems discussed in the first part of this paper, where in addition to differential equations of motion, nonholonomic constraints apply. The final equations of motion for these systems are obtained in the form of corresponding power series. Also, an alternative, direct method to determine the initial values of higher-order derivatives \({\ddot{q}}_0 ,{{\dddot{q}{} }}_{\!0} ,\ldots \) is proposed, being different from that of Whittaker. The second part of this work analyzes the stability of equilibrium of less complex, nonholonomic mechanical systems represented by gradient systems. We discuss the stability of equilibrium of such systems based on the properties of the gradient system. The advantage of this novel method is its avoidance of the difficulty of directly establishing Lyapunov functions aimed at such unsteady nonlinear systems. Finally, these theoretical considerations are illustrated through four examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Neimark, J.I., Fufaev, N.A.: Dynamics of Nonholonomic Systems. American Mathematical Society, Providence (1972)

    MATH  Google Scholar 

  2. Hamel, G.: Die Lagrange–Eulersche Gleichungen der mechanik. Z. Math. Phys. Ser. 50, 1–57 (1904)

    MATH  Google Scholar 

  3. Voronets, P.V.: About motion equations of nonholonomic systems. J. Math. 22, 4 (1901). (in Russian)

    Google Scholar 

  4. Dobronravov, V.: Elements of Nonholonomic Systems Mechanics. Vysshaya Shkola, Moscow (1970). (in Russian)

    MATH  Google Scholar 

  5. Chaplygin, S.A.: Investigations of Nonholonomic Systems Dynamics. GIITL, Gorakhpur (1949). (in Russian)

    Google Scholar 

  6. Kane, T.R.: Discussion on the paper: dynamics of nonholonomic systems. Trans. ASME 29, 31 (1962)

    Google Scholar 

  7. Pars, L.A.: A Treatise on Analytical Dynamics. Heinemann, London (1965)

    MATH  Google Scholar 

  8. Gantmacher, F.: Lectures in Analytical Mechanics. Mir, Moscow (1990)

    Google Scholar 

  9. Papastavridis, J.G.: Analytical Mechanics. Oxford University, New York (2002)

    MATH  Google Scholar 

  10. Novoselov, V.S.: Variational Methods in Mechanics. Leningrad University, Leningrad (1966). (in Russian)

    Google Scholar 

  11. Mei, F.X.: Nonholonomic mechanics. ASME Appl. Mech. Rev. 53, 283–305 (2000)

    Article  Google Scholar 

  12. Appell, P.: Traité de Mécanique Rationлelle, 6th edn. Gauthier-Villars, Paris (1953). (in French)

    Google Scholar 

  13. Guo, Y.X., Shang, M., Mei, F.X.: Poincaré-Cartan integral invariants of nonconservative dynamical systems. Int. J. Theor. Phys. 38, 1017–1027 (1999)

    Article  Google Scholar 

  14. Mušicki, D., Zeković, D.: Energy integral for the systems with nonholonomic constraints of arbitrary form and original. Acta. Mech. 227, 467–493 (2016)

    Article  MathSciNet  Google Scholar 

  15. Dragomir, N.Z.: Dynamics of mechanical systems with nonlinear nonholonomic constraints—analysis of motion. Z. Angew. Math. Mech. 93, 550–574 (2013)

    Article  MathSciNet  Google Scholar 

  16. Zhang, Y., Mei, F.X.: Form invariance for systems of generalized classical mechanics. Chin. Phys. B 12, 1058 (2003)

    Article  Google Scholar 

  17. Block, A.M.: Stability of nonholonomic control systems. Automatica 28, 431–435 (1992)

    Article  Google Scholar 

  18. Maggi, G.A.: Prineipii della Teoria Matematical del Movimento dei corpi: Corso di Meccanica, Razionale edn. Ukico Hoepli, Milano (1896). (in Italian)

    Google Scholar 

  19. Maggi, G.A.: Di Aleune nuove forme delle equazioni della dinamica applicabili ai sistemi anolonomL. Rendiconti della Regia Accademia dei Lincei Serie X, 287–291 (1901). (in Italian)

  20. Levi-Civita, T., Amaldi, U.: Lezioni di Meccanica Razionale. Zanichelli Editore, Bologna (1926). (in Italian)

    MATH  Google Scholar 

  21. Kurdil, A.A., Papastavridis, J.G., Kamat, M.P.: Role of Maggi’s equations in computational methods for constrained multibody systems. J. Guid. 13, 113–120 (1990)

    Article  MathSciNet  Google Scholar 

  22. de Jalon, J.G., Callejo, A., Hidalgo, A.F.: Efficient solution of Maggi’s equations. J. Comput. Nonlinear Dyn. 3, 011004 (2012)

    Google Scholar 

  23. Borri, M., Bottasso, C., Mantegazza, P.: Equivalence of Kane’s and Maggi’s equations. Meccanica 25, 272–274 (1990)

    Article  MathSciNet  Google Scholar 

  24. Finzi, B.: Meccanica Razionale. Zanichelli Editore, Bologna (1946). (in Italian)

    MATH  Google Scholar 

  25. Zegzhda, S.A., Soltakhanov, S.K., Yushkov, M.P.: Equations of Motion of Nonholonomic Systems and Variational Principle of Mechanics. New Class Problems of Control. FIMATLIT, Moscow (2005). (in Russian)

    MATH  Google Scholar 

  26. Whittaker, E.T.: A Treatise on the Analytical Dynamics of Particle and Rigid Bodies, 4th edn. Cambridge University Press, Cambridge (1952)

    MATH  Google Scholar 

  27. Novoselov, V.S., Korolev, V.S.: Analytical Mechanics of Controllable Systems. S Petr University Press, Cambridge (2005). (in Russian)

    Google Scholar 

  28. Chen, J., Guo, Y.X., Mei, F.X.: The initial motions of holonomic and nonholonomic mechanical systems. Acta. Mech. Sin. 228, 11 (2017)

    MathSciNet  MATH  Google Scholar 

  29. Matveev, M.V.: Lyapunov stability of equilibrium sates of reversible systems. Math. Notes 57, 1–2 (1995)

    Article  Google Scholar 

  30. Ghaffari, A., Lasemi, N.: New method to examine the stability of equilibrium points for a class of nonlinear dynamical systems. Nonlinear Dyn. 79, 2271–2271 (2015)

    Article  MathSciNet  Google Scholar 

  31. Čović, V., Vesković, M., et al.: On the stability of equilibria of nonholonomic systems with nonlinear constraints. Appl. Math. Mech. Engl. Ed. 31, 751–760 (2010)

    Article  MathSciNet  Google Scholar 

  32. Zhu, H.P., Mei, F.X.: On the stability of nonholonomic mechanical systems with respect to partial variables. Appl. Math. Mech. Engl. Ed. 16, 237–245 (1995)

    Article  MathSciNet  Google Scholar 

  33. Cantarelli, G.: On the stability of the equilibrium of mechanical systems. Period. Math. Hung. 44, 157–167 (2002)

    Article  MathSciNet  Google Scholar 

  34. Hirsch, M.W., Smale, S., Devaney, R.L.: Differential Equations, Dynamical Systems, and Introduction to Chaos. Elsevier, Singapore (2008)

    MATH  Google Scholar 

  35. McLachlan, R.I., Quispel, G.R.W., Robidoux, N.: Geometric integration using discrete gradients. Philos. Trans. R. Soc. Lond. A 357, 1021–1045 (1999)

    Article  MathSciNet  Google Scholar 

  36. Mei, F.X., Wu, H.B.: Gradient Representations of Constrained Mechanical Systems. Science Press, Beijing (2016). (in Chinese)

    Google Scholar 

  37. Mei, F.X., Wu, H.B.: Gradient systems and mechanical systems. Acta. Mech. Sin. 32, 935–940 (2016)

    Article  MathSciNet  Google Scholar 

  38. Mei, F.X., Wu, H.B.: A gradient representation for first-order Lagrange system. Acta. Phys. Sin. 62, 214501 (2013)

    Google Scholar 

  39. Lou, Z.M., Mei, F.X.: A second order gradient representation of mechanics system. Acta. Phys. Sin. 61, 024502 (2012). (in Chinese)

    Google Scholar 

  40. Chen, X.W., Zhao, G.L., Mei, F.X.: A fractional gradient representation of the Poincaré equations. Nonlinear Dyn. 73, 579–582 (2013)

    Article  Google Scholar 

  41. Mei, F.X., Wu, H.B.: Bifurcation for the generalized Birkhoffian system. Chin. Phys. B. 24, 054501 (2015)

    Article  Google Scholar 

  42. Appell, P.: Example de movement dun point assujetti à une liaison exprimée par une relation non-lineaire entre les composantes de la vitesse. Rend Circ. Math. Palermo. 32, 48–50 (1911). (in French)

    Article  Google Scholar 

  43. Hamel, G.: Theoretische Mechanik. Springer, Berlin (1949). (in German)

    Book  Google Scholar 

Download references

Acknowledgements

This project was supported by the National Natural Science Foundation of China (Grants 11572145, 11472124, and 11572034).

Author information

Authors and Affiliations

  1. School of Aerospace Engineering, Beijing Institute of Technology, Beijing, 100081, China

    J. Chen & F. X. Mei

  2. School of Physics, Liaoning University, Shenyang, 110000, China

    Y. X. Guo

Authors
  1. J. Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Y. X. Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. F. X. Mei
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to F. X. Mei.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, J., Guo, Y.X. & Mei, F.X. New methods to find solutions and analyze stability of equilibrium of nonholonomic mechanical systems. Acta Mech. Sin. 34, 1136–1144 (2018). https://doi.org/10.1007/s10409-018-0768-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10409-018-0768-x

Keywords

Search

Navigation