Introduction

Problem solving is the main goal of mathematics teaching. To reach that goal, students need to face different types of mathematical problems, from routine exercises aimed at providing practice on a particular mathematical technique to the most difficult or perplexing problems (Schoenfeld, 1992). Arithmetic word problems (AWPs) are a specific type of mathematical problem. Solving AWPs is essential for mathematical learning since it provides students with the opportunity to apply mathematics to everyday situations and gain essential heuristic and metacognitive abilities necessary for solving different types of problems (Verschaffel et al., 2020). AWPs can be defined as verbal descriptions of problem situations that give rise to one or more questions whose answers can be obtained by performing mathematical operations with the problem data (Verschaffel et al., 2020).

Different theoretical models have been proposed to explain the cognitive mechanisms needed for solving AWPs. For example, Verschaffel et al. (2000) proposed a model that identifies multiple types of problems with different levels of complexity that require the solver to use specific types of reasoning. According to this model, students need to encounter a wide range of AWPs, varying in difficulty levels, throughout their school years to learn how to solve them (Caldwell et al., 2011; Parmar et al., 1996; Xin et al., 2011). Additionally, certain types of illustrations serve as reasoning aids and facilitate better AWP solving capabilities among students (Chan & Kwan, 2021). These two ideas underlie two evidence-based approaches to teaching math: cognitively guided instruction (CGI) and schema-based instruction (SBI). These approaches have underpinned numerous intervention programs (Carpenter et al., 1999; Marshall, 2012) and have influenced textbook development in some countries.

Textbooks play a critical role in shaping student learning, as they are often used as a teaching tool for solving AWPs (Depaepe et al., 2009; Hiebert et al., 2003), and their influence extends to the content taught and the depth of coverage (Morris & Adamson, 2010; Stein & Smith, 2010). They also have an impact on the development of mathematical skills (Sievert et al., 2019, 2021; Törnroos, 2005). In this regard, the inclusion of sound theoretical and methodological approaches to mathematics education in the US (CGI) and Singapore (SBI) curricula has played a key role in the emergence of high-quality textbooks designed in accordance with these approaches (Oates, 2014; Schoen et al., 2020). In contrast, the mathematics curricula of other countries, such as Spain, have not specified any approach for teaching resolutions to AWPs. This may explain why Spanish textbooks show deficiencies in the choice of AWPs and teaching tools, such as a limited variety of problems, superficial models of resolution, and the limited usefulness of the illustrations included (Orrantia et al., 2005; Tárraga et al., 2021; Vicente et al., 2018, 2020). However, although these approaches are not yet part of the Spanish mathematics curriculum, some textbooks recently released by major publishers in the country were developed using guidelines from theoretical and methodological approaches based on CGI and SBI. This raises two questions regarding AWPs.: first, without a prior change in the country’s math curriculum, is it possible for a publisher to improve its textbooks by adopting a particular theoretical-methodological approach? Second, would different theoretical and methodological frameworks lead to different publications emphasizing different facets of teaching AWP solving?

Problem solving: theoretical models and theoretical-methodological approaches

The resolution of AWPs is a complex task that is the result of the implementation of several cognitive processes. Various theoretical frameworks have been proposed to describe these cognitive processes. One such model proposed by Verschaffel et al. (2000) identifies two modes of problem solving: genuine and superficial. The genuine mode requires the solver to understand the mathematical structure of the problem and apply mathematical reasoning. This allows for the resolution of AWPs of any level of complexity. In contrast, the superficial mode relies on the solver selecting textual or contextual information to deduce the appropriate mathematical operation to solve the problem without applying mathematical reasoning. This superficial mode involves strategies such as modeling the actions described in the problem using concrete materials (Riley & Greeno, 1988) or the “key word strategy,” namely, the use of certain words such as “lose” as cues for selecting a specific arithmetic operation (e.g., subtraction) (Hegarty et al., 1995; Verschaffel et al., 1992). The superficial approach is solely effective in resolving simple problems that can be solved in a straightforward manner.

Based on the postulates of models such as those in Verschaffel et al. (2000), certain theoretical and methodological approaches encourage specific strategies for teaching mathematics and solving AWPs. Two of these approaches are cognitively guided instruction (CGI) and schema-based instruction (SBI). Based on these approaches, numerous instructional programs have been developed to improve students’ mathematical skills. These programs contain specifically designed interventions that target key elements of the teaching–learning process, such as instructors’ knowledge, instructional procedures, students’ cognitive development, and task type, and have demonstrable success (Carpenter et al., 1999). In relation to solving AWPs, the first of these approaches (CGI) emphasizes the need to teach students to solve problems starting from their intuitive knowledge of mathematics and the rudimentary solving procedures that such knowledge allows them (e.g., initially following the language of the problems with direct modeling, that is, following the language of the problem to model the action of the problem, Riley & Greeno, 1988). Since these strategies only allow them to solve the easiest problems, students are provided with problems of different types and levels of difficulty, allowing them to replace direct modeling with more efficient and genuine solving strategies based on the understanding of the relationships between the sets of the problem, as well as the relationships between the numbers (Carpenter et al., 1999). To this end, CGI provides a taxonomy of problem types that provide an expanded perspective on what it means to add, subtract, multiply, and divide (Carpenter & Frankle, 2004). As a result, this approach indicates that students can enhance their problem-solving skills through ample opportunities to solve all types of problems listed in the taxonomy. This is consistent with learning variation theory (Marton, 2015), which states that for solvers to adequately develop their ability to solve AWPs, they should be confronted with problems of the widest possible variety. By solving a wide variety of problems, solvers learn to discern and focus on the fundamental aspects of the solving process, which allows them to develop appropriate strategies and avoid the generalized application of certain AWP solving strategies, such as keyword identification (see Riley & Greeno, 1988).

A second approach, schema-based instruction (SBI), aims to improve students’ performance in solving word problems by emphasizing both the semantic and mathematical structures of the problem (Marshall, 2012). SBI is grounded in schema theory and cognitive models that prioritize the knowledge of procedures, such as problem representation and planning, for a specific class of problems. This approach guides teachers to use instructional practices, such as having students actively compare, reflect on, and discuss multiple resolution methods or use “think-alouds” to develop metacognitive strategy knowledge, to (a) help students recognize common underlying semantic structures in problems (those described in the CGI taxonomy); (b) represent problems using visual-schematic diagrams; (c) plan how to solve problems; and (d) solve and check the reasonableness of answers (Alghamdi et al., 2019). The utilization of schematic illustrations depicting the mathematical structure of problems is a fundamental component of SBI for instructing students to solve problems genuinely, in the mode described by Verschaffel et al. (2000). SBI relies on schematic representations, rather than other forms of representations, as not all types of representations facilitate problem solving among students. For instance, Elia and Philippou (2004) identified four types of illustrations that can accompany a problem statement: (a) decorative images that provide information unrelated to the problem resolution; (b) representational images that reproduce a part (or all) of the situation in which the problem arises; (c) informative images that supply the problem’s main information source; and (d) organizational images that facilitate problem resolution through schematic representation of its structure. Empirical evidence shows that providing students with representational illustrations has no effect on their success in solving AWPs (Hegarty & Kozhevnikov, 1999; Vicente et al, 2008). However, learners solve AWPs more effectively when presented with organizational illustrations in the form of diagrams that represent the problem’s mathematical structure, such as those used in SBI (Múñez et al., 2013; Xin, 2019). An example of this type of representation is the “Model” method used for teaching problem solving in Singapore. In this method, students are instructed to draw rectangular “bars” representing the quantitative parts or whole as given in the story context. The drawings, which are schematic in nature, can be of three different types: the part-whole model, the comparison model, and the change model (see Kaur, 2019). By utilizing these three models, almost all additive and multiplicative problems described in the CGI taxonomy can be represented. These structures are described below.

Types of arithmetic word problems

Exposing students to a significant number and variety of AWPs is crucial for the acquisition of problem-solving skills, as per the theoretical models and theoretical-methodological approaches described above. In addition, exposing students to a variety of AWPs should enhance the acquisition of arithmetic skills rather than the opposite (Nunes et al., 2016). In this sense, different types of AWPs, implying different levels of difficulty, can be established according to linguistic, mathematical, social, or pedagogical criteria (Daroczy et al., 2015). Among the factors that determine the difficulty of AWPs, semantic-mathematical structure is of great importance (Carpenter & Moser, 1984; Greer, 1992; Vergnaud, 1991).

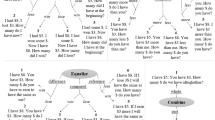
For example, additive AWPs, which can be solved by addition or subtraction, can be categorized into four types: change, compare, combine, and equalize. These problems are classified based on their semantic-mathematical structure (Carpenter & Moser, 1984; Heller & Greeno, 1978). Depending on the unknown set and the relationships (additive or subtractive) that exist between the sets involved in each AWP, the categories shown in Fig. 1 can be established.

Fig. 1
figure 1

Subtypes of additive structure problems (adapted from Vicente et al., 2022b). Note: CH = change; CP = compare; CB = combine; EQ = equalize

Full size image

Some of these problems can be solved simply. For example, problems such as “I had $5. I won $3. How many do I have now?” can be solved using the “keyword strategy” (Hegarty et al., 1995), taking the numerical data provided in the problem and using the word “won” as a clue to add 5 and 3. Alternatively, the problem can be addressed through direct modeling by adding the $3 earned to the initial amount. However, solving other problems requires an understanding of the relationships between the quantities involved (Verschaffel et al., 2000). For example, the problem “I have $8. I have $3 more than you. How many $ do you have?” requires that students reason that if I have more money than you, then you will have less than me, and therefore a subtraction is necessary.

Likewise, within multiplicative AWPs, four types can be distinguished (Greer, 1992; Vergnaud, 1991): rates (or equal groups), multiplicative comparison (or scalars), Cartesian products, and rectangular matrices (see Fig. 2).

Fig. 2
figure 2

Subtypes of multiplicative structure problems (adapted from Vicente et al., 2022b)

Full size image

Thus, 34 types of AWPs of additive and multiplicative semantic-mathematical structures have been described. To check whether students have ample opportunity to solve a wide variety of these problems and whether they are provided with certain aids for this purpose, an analysis of the textbooks is advisable.

Textbooks and AWPs

Textbooks have a significant impact on educational practice and student learning. They form a crucial component of the school curriculum and largely determine what is taught in the classroom (Apple, 1992; Oates, 2014). Moreover, teachers in most countries frequently use textbooks (Depaepe et al., 2009; Hiebert et al., 2003). Empirical evidence indicates a link between the design of textbooks, particularly the quantity and quality of mathematical content, and the development of mathematical skills (Fagginger Auer et al., 2016; Schmidt et al., 2001; Siegler & Oppenzato, 2021; Sievert et al., 2019, 2021; Törnroos, 2005).

Textbooks are complex instructional resources, so there are many aspects that can be analyzed (see Fuchs & Bock, 2018). Regarding AWP solving, different studies have focused on diverse aspects, such as resolution models (Sánchez & Vicente, 2015; Vicente et al., 2020), the design of teachers’ instructional guides (Tárraga & Tarín, 2022), the level of authenticity of AWPs (Depaepe et al., 2010; Vicente et al., 2021), the aids provided to support the resolution process (Vicente et al., 2022b) or the semantic-mathematical variety and the level of difficulty of the problems included in books (Tárraga et al., 2021; Vicente et al., 2008, 2018, 2022b).

It is these last two groups of studies that are a direct antecedent for our work. Building on previous research connecting poor performance with limited exposure to AWPs (Parmar et al., 1996; Xin et al., 2011), these studies suggest that low scores on international student assessments in certain countries may result from inadequate textbook design when compared to textbook designs in countries such as Japan, China, or Singapore. For example, books from the aforementioned Asian countries show a more diversified distribution of additive and multiplicative AWPs than books from the USA (Schoenfeld, 1991; Stigler et al., 1986; Xin, 2007), Greece (Despina & Harikleia, 2014) and Spain (Tárraga et al., 2021; Vicente et al., 2008, 2018, 2022b). Additionally, Singaporean books included a larger proportion of AWP-solving activities and more schematic representations of their mathematical structures than Spanish textbooks (Vicente et al., 2022b).

Although certain current books in some countries may include components that facilitate a student’s ability to learn to solve AWPs through reasoning, it is important to acknowledge that this has not always been the case. In certain countries, the instructional design of AWP resolution in textbooks has seen advancements over the years associated with changes in the official curriculum. One paradigmatic example is the USA, where the establishment of the Common Core State Standards for Mathematics in 2010 changed the landscape across many aspects of mathematics education in that country (Schoen et al., 2020). In relation to the resolution of AWPs, the standards for 1st- and 2nd-grade students in primary schools explicitly require students to solve all types of problems included in the taxonomy of additive problems proposed in the theoretical framework of CGI, which encompasses all types of AWPs illustrated in Fig. 1 (Carpenter et al., 1999; National Governors Association Center for Best Practices & Council of Chief State School Officers, 2010). Similarly, the standards for the 3rd and 4th graders cover rate, scalar, and rectangular matrix problems, as outlined in Fig. 2. Consequently, several American publishers have revised their textbooks to incorporate a broader range of additive AWPs in 1st-grade books than in books published prior to the establishment of these standards (Schoen et al., 2020).

Another example is the textbooks created after Singapore’s New Education System was established and implemented in 1981, thereby expanding on its key curricular aspects such as detailed curricula or textbooks (see Kaur, 2019.) A fundamental aspect of this curriculum was the implementation of the C-P-A (Concrete-Pictorial-Abstract) approach to mathematics education, which was first introduced by Bruner (1973). The Singapore Ministry of Education developed the Model Method (Kho, 1987) to implement an approach that utilizes schematic representations to enable students to visualize the mathematical structure of problems prior to selecting the necessary operation(s) to solve them (see Ferrucci et al., 2008; Kaur, 2019). The Model Method is an example of SBI (Kaur, 2019). Notably, Singaporean textbooks prioritize teaching problem solving through bar modeling over the provision of AWPs with diverse structures and difficulty levels, in contrast to the recent US textbooks (Schoen et al., 2020; Vicente et al., 2022b).

In contrast, mathematics textbooks in certain countries, such as Spain (Tárraga et al., 2021; Vicente et al., 2022b), have seen minimal updates over time regarding the resolution of AWPs, despite various adjustments to educational legislation. Although these laws introduced modifications to previous laws, none of them followed a specific theoretical-methodological approach but rather focused on aspects such as the implementation of teaching and the assessment of learning by competencies (Molina et al., 2016). Notably, other countries, such as the Netherlands, seem to have a similar situation (see Van Zanten & Van den Heuvel-Panhuizen, 2018).

Finally, in 2019, two major Spanish publishers released mathematics textbooks following specific theoretical and methodological approaches, without any modification of the official curriculum. One such textbook was based on the Open Algorithm Based on Numbers Method (ABN, Martínez-Montero & Sánchez, 2013), proposed as an alternative to traditional methods of teaching calculus and problem solving. Problem solving is based on the semantic categories model, which provides students with a diverse range of experiences related to the semantic-mathematical structure of AWPs. This model explicitly considers all the structures described in Figs. 1 and 2 (see Martínez-Montero & Sánchez, 2013). As a result, the ABN approach to solving AWPs is similar to that of CGI. The second textbook was developed in accordance with the aforementioned Singaporean curriculum, particularly utilizing the C-P-A approach. In addition, the model method is used as a teaching strategy for problem solving.

This study

The goal of this research is to assess whether a mathematics textbook publisher can enhance certain elements of AWP solving using a particular theoretical-methodological approach in textbooks without prior modifications in the mathematics curriculum. For this purpose, some characteristics of the AWPs included in the 2019 textbooks of Spanish publishers Anaya and SM will be compared with those of their previous books published between 2008 and 2018, which lacked any explicit theoretical or methodological framework. To ensure a focused study, our analysis of the textbooks is limited to the problems themselves. Out of all the features present in AWPs included in the book, we concentrated on the three variables used by Vicente et al. (2022b) to compare Spanish and Singaporean books. These measures are the percentage of AWPs included in each textbook in relation to the total number of activities, the semantic-mathematical structures of the AWPs (including their variety and level of difficulty), and the function of the illustrations included with the AWPs.

Method

Sample: publishers and books analyzed

We analyzed all the activities included in the books and complementary booklets for the 3rd grade of primary schoolFootnote 1 that were released between 2008 and 2019 by Spanish publishers that, having a wide presence in the classrooms, explicitly adopted a theoretical and methodological approach for the development of the textbooks published in 2019. Two publishers met both requirements: Anaya (hereafter, ED1), which based its books on the ABN method, and SM (hereafter ED2), which based its books on the Singaporean curriculum. Between 2008 and 2019, ED1 published 5 different textbooks, which we will refer to in our study as ED1-2008, ED1-2014, ED1-2015, ED1-2018, and ED1-2019, in reference to the years in which they were published. In the same period, ED2 published 4 different textbooks, which we will refer to as ED2-2008, ED2-2014, ED2-2018, and ED2-2019. Notably, textbooks are the primary resource for more than 80% of teachers in Spain (ANELE, 2014; Mullis et al., 2014). Therefore, the analysis of the AWPs contained in these books can provide a reliable measure of the actual teaching activities related to the resolution of AWPs. Finally, Vicente et al. (2018) reported that ED1 and ED2 textbooks were used in 21.30% and 25.76% of primary schools, respectively, in Spain.

Procedure

Categories of analysis: AWPs vs. other mathematical activities (OMA)

An “activity” was defined as each task or group of tasks included under the same heading or label, in which the student had to provide answers to one or more questions that usually needed calculations or the application of mathematical knowledge. Each activity presented in the textbooks was assigned to one of the following categories: (1) AWP-solving activities and (2) other mathematical activities (hereafter OMAs). The AWP-solving activities included one or more AWPs (therefore, the number of AWP-solving activities was lower than the number of AWPs). AWPs were those problems that could be solved by applying at least one of the four basic arithmetic operations and whose semantic-mathematical structure can be categorized as one of those described in the literature (see Figs. 1 and 2). The rest of the activities were considered OMAs, including solving other types of problems (i.e., geometry or statistics). The first measure analyzed in the study was the percentage of activities in each book devoted to solving AWPs and OMAs, each. Given the purpose of our study, we did not perform any further analysis of OMAs.

Categories of analysis: semantic-mathematical structure

The number of different semantic-mathematical structures included in each of the books analyzed was the second measure of the study. Subsequently, AWPs with an additive structure and those with a multiplicative structure were categorized separately. For problems with two or more structures, it was decided to decompose these problems into individual structures for categorization due to the complexity of establishing a single level of difficulty for a problem with multiple structures (see Vicente et al, 2022a).

Additive AWPs were classified as change, compare, combine, and equalize problems (Carpenter & Moser, 1984; Heller & Greeno, 1978), with 20 different subcategories established based on the unknown set and the relationships (additive or subtractive) between the sets involved (see Fig. 1). Each subcategory was classified according to its level of difficulty, following the model of resolution strategies proposed by Riley and Greeno (1988). The first level, easy AWPs, included those whose resolution did not require the creation of a mental representation (change 1 and 2, compare 1 and 2, equalize 1 and 2, and combine 1, see Fig. 1). The second level—AWPs of medium difficulty—included those whose resolution needed students to generate a mental representation of the problem before performing the actions necessary to solve it (change 3 and 4, compare 3 and 4, equalize 5 and 6 problems). Combine 2 problems were also considered of medium difficulty (see Rathmell, 1986; Riley & Greeno, 1988). The third level—difficult AWPs—includes those that require the application of conceptual knowledge (e.g., part-whole) to transform the student’s initial mental representation of the problem (change 5 and 6, comparison 5 and 6, and equalize 3 and 4). The percentage of AWP structures belonging to each level of semantic-mathematical difficulty in each textbook from each publisher was the third measure included in the study.

Regarding multiplicative problems, and according to the categories described in Fig. 2, simple rate AWPs—those closest to additive structure problems—were considered easy. AWPs of medium difficulty were considered to be (a) multiple rate, since the rate is not as evident as in simple rate problems (Vergnaud, 1991); and (b) scalars, as long as they were consistent—when the expression “times more” or “times less” coincided with the operation to be performed (multiplication and division, respectively). It should be noted that scalar AWPs are more difficult to solve than rate AWPs (Xin, 2007). Finally, both inconsistent scalar AWPs and rectangular matrix and Cartesian product AWPs were considered difficult (Vergnaud, 1991). The fourth measure considered in the study was the percentage of multiplicative structures at each level of semantic-mathematical difficulty in each textbook from each publisher.

Category of analysis: types of illustrations

Only those illustrations provided together with the AWPs in the textbooks were analyzed. To check the role of these illustrations in the problem-solving process, an adaptation of the classification made by Elia and Philippou (2004) was used. Three types of illustrations were considered (see Fig. 3).

Fig. 3
figure 3

Types of illustrations considered in the study. Note. Figurative illustration taken from ED1-2019, p. 38; informative illustration taken from ED2-2018, p. 139; schematic illustration taken from ED2-2019, p. 72

Full size image

  • Figurative: pictorial illustrations that do not contribute to the resolution, either because they are unrelated to the situation described in the problem or because, even though they represent an element, part or all of the problem situation, they do not present information relevant to the resolution (e.g., they do not contain numerical data or references to the mathematical structure of the problem).

  • Informative: pictorial illustrations, tables, and graphs containing data necessary to solve the problem (i.e., these illustrations replace the text of the problem as a source of information).

  • Schematic: illustrations that represent part or all of the mathematical structure of the problem, helping students to understand the mathematical relationships between the quantities in the problem. These illustrations may include the numerical data from the AWPs. Singapore’s “bar modeling” would fall into this category.

The fifth measure of the study was the percentage of AWPs in each book that were accompanied by illustrations of each of the types described (figurative, informative, and schematic).

Data analysis

General comparisons were made between textbooks from the same publisher. To test the statistical significance of the overall differences between books from the same publisher, the nonparametric chi-square test was used through planned tables, and Fisher’s exact test was used when the chi-square test was not appropriate. To quantify the effect of these differences, we used Cramer’s V, which, according to Cohen (1988), indicates whether the effect of the differences is small (0.1), medium (0.3), or large (0.5). To compare the differences between two specific textbooks, z tests with a significance level of 0.05 were used, using pairwise comparisons of column proportions from the planned tables.

Predictions

Based on the theoretical and methodological approaches used by ED1 and ED2 for the design of the books published in 2019, our general prediction is that compared to books published between 2008 and 2018, 2019 books will contain:

  • A higher percentage of activities aimed at solving AWPs.

  • A greater variety of semantic-mathematical structures.

  • A higher percentage of semantic-mathematical structures of high difficulty, both additive (prediction 3a) and multiplicative (prediction 3b).

  • A higher percentage of AWPs accompanied by schematic illustrations in ED2 books (prediction 4a) but not in ED1 books (prediction 4b), since ED2-2019 is based on the Singapore curriculum, which follows the C-P-A approach.

Results

AWPs vs. other mathematical activities

A total of 9879 activities were categorized (5857 in ED1 and 4022 in ED2), of which 2829 were AWP-solving activities (1838 in ED1 and 991 in ED2). Significant differences between previous and most recent textbooks were found for each publisher, ED1: χ2 (4, n = 5857) = 141.26, p < 0.001; ED2: χ2 (3, n = 4022) = 34.62, p < 0.001. The effects were small (0.16 and 0.09, respectively).

Of the ED1 books, ED1-2019 included a higher percentage of AWP-solving activities than the rest of the books. Of the ED2 books, ED2-2014, ED2-2018, and ED2-2019 included a higher percentage of AWP-solving activities than ED2-2008 (see Fig. 4). These results confirm prediction 1 for the ED1 books but not for the ED2 books.

Fig. 4
figure 4

Percentage of AWP resolution activities in each textbook of each publisher. Note. An asterisk indicates the book that showed significant differences from the most recent book

Full size image

Variety of problems

As shown in Fig. 5, the most recent book from ED1 included the greatest variety of structures; in fact, it included AWPs of all possible structures. No differences were found in the number of structures included between the different books from ED2. These results confirm prediction 2 for the ED1 books but not for the ED2 books.

Fig. 5
figure 5

Number of semantic-mathematical structures included in each textbook of each publisher

Full size image

Semantic-mathematical difficulty

In the AWP-solving activities, 3840 AWPs were identified (2465 in ED1 and 1375 in ED2 books). A total of 4927 semantic-mathematical structures were identified (2448 additive, 1502 in ED1 and 946 in ED2; and 2479 multiplicative, 1635 in ED1 and 844 in ED2).

Additive structures

Fisher’s exact test showed significant differences between the two publishers’ textbooks (p < 0.001 in both cases), with small effect sizes (0.14 and. 17, respectively). ED1-2019 included significantly fewer easy problems, and the most recent books from both publishers included significantly more difficult structures than the rest. Additionally, ED2-2014 included more problems of medium difficulty than ED2-2019. These results, shown in Fig. 6, confirm prediction 3a.

Fig. 6
figure 6

Percentage of additive structures by difficulty level in each textbook from each publisher. Note. The asterisk indicates the book that showed significant differences from the 2019 books

Full size image

Multiplicative structures

Fisher’s exact test showed significant differences between ED1 books (p < 0.001), with a small effect (0.18), but not between those of ED2. The most recent textbooks of the two publishers included the fewest easy structures and the most difficult structures. However, only a few differences were significant. ED1-2019 included more difficult structures than the rest of the ED1 books, fewer easy structures than ED1-2008, ED1-2014, and ED1-2015, and more structures of medium difficulty than ED1-2015 (also ED1-2018 included more medium structures than ED1-2015). On the other hand, although ED2-2019 included fewer easy structures and more medium- and high-difficulty structures than the rest of the ED2 books, these differences did not reach the level of significance. These results, shown in Fig. 7, confirm prediction 3b.

Fig. 7
figure 7

Percentage of multiplicative structures by level of difficulty in each textbook from each publisher. Note. The asterisk indicates the book that showed significant differences from the 2019 books

Full size image

Illustrations

With regard to the types of illustrations included by each publisher, significant differences were found between the books of ED1, χ2 (8, n = 1732) = 297.43, p < 0.001, and ED2, χ2 (6, n = 1008) = 136.42, p < 0.001, the effect being small-medium in both cases (0.29 and. 26, respectively).

More figurative illustrations and fewer informational illustrations were found in ED1-2019 compared to the rest of the ED1 books (ED1-2008, ED1-2014, and ED1-2015 also presented more figurative illustrations than ED1-2018). Finally, it is striking that ED1-2018 included a significantly higher proportion of schematic illustrations than the rest of the ED1 books, despite being the textbook with the lowest percentage of problems accompanied by illustrations. In ED2 books, ED2-2019 included more schematic illustrations and fewer figurative illustrations than the rest of the publisher’s books. In addition, ED2-2018 contained more figurative and fewer informational illustrations than the rest. These results, shown in Fig. 8, confirm prediction 4a for ED2 books and prediction 4b for ED1 books.

Fig. 8
figure 8

Percentage of each type of illustrations included in each textbook for each publisher. Note. The asterisk indicates the book that showed significant differences from the 2019 books

Full size image

Discussion

In some countries, such as the USA or Singapore, the official mathematics curricula were modified according to certain theoretical-methodological frameworks (CGI and SBI, respectively, see Carpenter et al., 1999; Kaur, 2019; National Governors Association Center for Best Practices & Council of Chief State School Officers, 2010). These changes led to the appearance of new textbooks, with certain improvements observed over previous books, both in the variety of problems (Schoen et al., 2020) and in other related aspects, such as the inclusion of graphical aids for reasoning (Kaur, 2019). However, in Spain, successive changes to the mathematics curriculum were not explicitly supported by a theoretical framework on AWP solving (Orrantia et al., 2005; Tárraga et al., 2021; Vicente et al., 2018). Nevertheless, two of the most widely used Spanish publishers released new versions of their books based on specific theoretical-methodological frameworks: ABN (itself based on the taxonomy of problems proposed by the CGI, Martínez-Montero & Sánchez, 2013) and the Singapore curriculum (based on the SBI approach). Therefore, the aim of the present work was to determine whether the mathematics books of these two Spanish publishers have improved over previous books in terms of the number and variety of AWPs and the inclusion of graphical aids in resolution.

As in the case of the American and Singaporean books, in our study, substantial improvements were observed in the Spanish textbooks that were designed according to specific theoretical-methodological approaches. Moreover, these improvements are in line with the theoretical-methodological framework adopted by each book. Starting with ED1-2019, in line with the ABN theoretical framework and the AWP taxonomy from CGI on which it is based, a higher percentage of tasks dedicated to solving AWPs was included, along with a greater variety of semantic-mathematical structures and a higher number of difficult semantic-mathematical structures than the previous textbooks. However, this book did not contain more schematic illustrations since the ABN approach (similar to the CGI on which it is based) emphasizes problem variety and the use of a specific algorithm for computation over the use of schematic illustrations. An exception to these results was ED1-2018: this book did not contain a significantly higher percentage of easy multiplicative AWPs but did contain a higher percentage of schematic illustrations than ED1-2019. This was because ED1-2018 included an additional booklet entitled “Problem Solving Workshop,” which contained more multiplicative problems of medium difficulty and in which virtually all the schematic illustrations from this book were found. One may wonder why these illustrations were not included in ED1-2019; a possible answer is that, in accordance with the ABN approach on which the textbook is based, it was decided to include other types of aids to teach the execution of the algorithms, more specifically to demonstrate calculation by the decomposition of quantities, which is the basis of the ABN calculation method. An example of this aid is the “grid” for the calculation by decomposition of quantities shown in Fig. 9.

Fig. 9
figure 9

Whole pages dedicated to solving AWPs, taken from ED1-2019, booklet 1, p. 16 (left) and ED2-2019, booklet A, p. 39 (right)

Full size image

In line with Singapore’s C-P-A approach (and the SBI on which it is based), ED2-2019 contained more schematic illustrations than the rest of the publisher’s books. It also included semantic-mathematical structures of a higher level of difficulty than the previous textbooks, both additive and multiplicative; it should be noted that the differences in multiplicative structures did not reach the level of significance, probably because the sample of this type of structure was much smaller than that of the ED1 books. However, the textbook did not contain a higher percentage of activities dedicated to solving AWPs or a greater variety of structures, thus it resembled the most widely used books in Singapore (Vicente et al., 2022b). According to these authors, the Singapore books appear to be designed to place more emphasis on how to solve problems using bar modeling than on providing AWPs of a variety of structures. It is important to keep in mind that reasoning aids such as bar modeling will only be helpful if they are accessible within their cognitive resources of the student. This is consistent with the theory of cognitive load (Sweller, 1988) and emphasizes the importance of considering the cognitive load associated with the task and its comprehension. The design of ED2-2019, like the texbooks of Singapore, seems to take this into account, since although an increase in the difficulty of the problems is observed over previous books, this increase is moderate.

On the other hand, the fact that we did not find a higher proportion of AWP solving activities in ED2-2019 compared to previous editions may be a consequence of both the application of the C-P-A approach and the characteristics of the SBI approach on which it is based. Many of the problem-solving pages in the ED2-2019 books were devoted to solving one or two problems, with few pages solving more than four problems. Sometimes the problems were accompanied by a bar model and other aids to other parts of the resolution process (e.g., representations of manipulatives to illustrate carrying addition or borrowing subtraction), while at other times, they left room for the student to elaborate on this representation. Given this distribution, it is logical that the ED2-2019 books left no room for a very high percentage of problems. Figure 9 illustrates this.

In short, the results of our study show that the new books, designed on the basis of specific theoretical-methodological frameworks, can be considered more appropriate tools for students for introducing a wider variety of problems and for learning to solve problems in a genuine way through reasoning, reducing the probability that students adopt only superficial solving strategies, such as the keyword strategy or direct modeling of the statement (Verschaffel et al., 2000). In this sense, and as seen in Fig. 9, each publisher emphasizes a specific aspect: while ED1-2019 focuses on presenting students with problems of all types and levels of difficulty, ED2-2019 focuses on bar modeling.

Finally, the incorporation of these theoretical-methodological frameworks by two leading Spanish publishers and the subsequent enhancement of their books was not a result of changes in the official curriculum, as was the case in the USA and Singapore (see Baker et al., 2010). In contrast, the publishers took the initiative themselves. Spanish publishers commonly revise their books every four years to accommodate new educational laws or update their products. However, ED1-2019 and ED2-2019 were released just a year after the previous editions. Consequently, it is worth asking why these books were published simultaneously at this time. One possible explanation posits that some publisher behaviors trigger responses from others; this would justify, for example, the tendency for resources from different publishers to become more similar over time as they follow the market leader (Oates, 2014). Our research suggests that major publishers tend to follow each other’s actions over time, even when these actions are risky and involve the production of resources that differ significantly from those teachers are accustomed to using. This suggests that even without legislative changes, one publisher’s positive action could indirectly lead to the improvement of other publishers’ materials. However, negative actions by one publisher could also result in lower quality books from other publishers.

Educational implications

The study’s primary educational implication is that the design of AWPs for student problem-solving abilities should be based on theoretical and methodological approaches. This general implication can be applied to three actors in the educational system: those responsible for the design of the official curriculum, textbook designers, and teachers.

Those in charge of the official curriculum must clearly state the theoretical and methodological frameworks that direct modifications to the curriculum. Moreover, they ought to offer precise guidance to textbook designers on the types of arithmetic word problems children need to learn to address, when to introduce them during the learning process, and what specific aids to use, including schematic illustrations. This could help publishers align with theoretical and methodological frameworks rather than leaving the enhancement of books solely to their own initiative.

Publishers can enhance the problem-solving design of their textbooks by utilizing an appropriate theoretical-methodological framework. This can be achieved without waiting for modifications to the mathematics curriculum. Cognitively guided instruction and schema-based instruction approaches offer valuable perspectives to guide learning task design. The Spanish publishers analyzed in the study may serve as a model for publishers in other countries to enhance their textbook design.

Teachers must acquire essential skills during their initial training (Leavy & Hourigan, 2022; Piñeiro et al., 2022) and ongoing professional development (Stylianou et al., 2019) to select and utilize pedagogically sound books to teach mathematical problem-solving and other content areas. Approaches such as cognitively guided instruction and schema-based instruction offer valuable guidance in assisting teachers in acquiring these skills. Ultimately, books should not be seen as the “silver bullet” that will solve the problem of teaching AWPs all by itself (Blazar et al., 2020) but rather as a tool that teachers should use wisely to effectively guide students in developing their mathematical thinking and AWP resolution strategies.

Limitations and future studies

This study has several limitations. First, the sample of books was reduced to 3rd-grade textbooks, so the representativeness of the sample is limited. Second, the analysis of the books is limited to only the AWPs, which leaves other tasks unanalyzed. These tasks could provide valuable information on the degree of adjustment across textbook topics to the theoretical-methodological framework the textbooks are based on. Additionally, the inclusion of some features common to both CGI and SBI, such as the promotion of multiple resolution strategies, is not analyzed in the books. Furthermore, an analysis of how teachers utilize the new books has not been conducted, making it impossible to determine the potential effects on both the educational practices of teachers and the learning efficacy of students. Finally, these findings are restricted to books from only one country, so they cannot be generalized to other countries.

Given these limitations, there are several issues that future studies should address. First, it is recommended that the analysis be extended to all primary grades, especially 1st and 2nd, where word problems are needed to understand basic operations. Second, the analysis of additional features of the ED1 and ED2 textbooks, such as resolution models for teaching AWP solving to students (Vicente et al., 2020), may provide insight into whether these books are consistent with their respective theoretical and methodological frameworks. The mere quantity or variety of AWPs provided does not necessarily initiate the same quality of procedures and learning paths. It is recommended that researchers assess the extent to which the books incorporate key concepts of the implemented theoretical and methodological frameworks, such as promoting multiple problem-solving strategies or enhancing metacognitive skills. Third, it would be valuable to investigate the extent to which Spanish primary school educators have included these materials in their instructional plans and to analyze the pedagogical practices that accompany them. It would also be instructive to examine how teachers use the ED1 and ED2 books when teaching students to solve AWPs in their classrooms, including their adherence to the guidelines provided by the theoretical and methodological approaches used in designing the books. Evidence suggests that well-designed instructional materials, such as the ED1-2019 and ED2-2019 textbooks, can help teachers improve their teaching practices (see Charambolous et al., 2012), especially those with high pedagogical knowledge for teaching AWP solving (Ramos et al., 2024). Finally, it would be beneficial to investigate the adoption of alternative theoretical approaches by publishers in different countries and to examine the unique features of those publications.