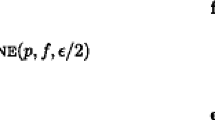Abstract
For single node flow sets with fixed costs and constant capacities on the inflow and outflow arcs, a family of constant capacity flow covers are known to provide the convex hull in different special cases and are conjectured to provide it in the general case. Here we study more general mixed integer sets for which such single node flow cover inequalities suffice to give the convex hull. In particular we consider the case of a path in which each node has one (or several) incoming and outgoing arcs with constant capacities and fixed costs. This can be seen as a lot-sizing set with production and sales decisions driven by costs and prices and by the lower and upper bounds on stocks instead of being driven by demands as in the standard lot-sizing model. The approach we take is classical: we characterize the extreme points, derive tight extended formulations and project out the additional variables. Specifically we show that Fourier–Motzkin elimination, though far from elegant, can be used to carry out the non-trivial projections. The validity of the conjecture for the single node flow set follows from our results.

Similar content being viewed by others
References
Aksen, D., Altinkemer, K., Chand, S.: The single-item lot-sizing problem with immediate lost sales. Eur. J. Oper. Res. 147, 558–566 (2003)
Atamtürk, A.: Flow pack facets of the single node fixed-charge flow polytope. Oper. Res. Lett. 29, 107–114 (2001)
Atamtürk, A.: On capacitated network design cut-set polyhedra. Math. Program. 92, 425–437 (2002)
Atamtürk, A., Gomez, A., Küçükyavuz, S.: Three-partition inequalities for constant capacity fixed-charge network flow problems. Networks 67, 299–315 (2016)
Atamtürk, A., Küçükyavuz, S.: Lot-sizing with inventory bounds and fixed costs: polyhedral study and computation. Oper. Res. 53, 711–730 (2005)
Atamtürk, A., Küçükyavuz, S., Tezel, B.: Path cover and path pack inequalities for the capacitated fixed-charge network flow problem. SIAM J. Optim. 27, 194–1976 (2017)
Barany, I., Van Roy, T.J., Wolsey, L.A.: Uncapacitated lot-sizing: the convex hull of solutions. Math. Program. 22, 32–43 (1984)
Bitran, G.R., Yanasse, H.H.: Computational complexity of the capacitated lot size problem. Manag. Sci. 28, 1174–1186 (1982)
Conforti, M., Di Summa, M., Eisenbrand, F., Wolsey, L.A.: Network formulations of mixed-integer programs. Math. Oper. Res. 34, 194–209 (2009)
Florian, M., Klein, M.: Deterministic production planning with concave costs and capacity constraints. Manag. Sci. 18, 12–20 (1971)
Florian, M., Lenstra, J.K., Rinnooy Kan, H.G.: Deterministic production planning: algorithms and complexity. Manag. Sci. 26, 669–679 (1980)
Ghouila-Houri, A.: Caractérisation des matrices totalement unimodulaire. C.R. Acad. Sci. Paris 254, 1192–1194 (1962)
Gu, Z., Nemhauser, G.L., Savelsbergh, M.W.P.: Lifted flow cover inequalities for mixed 0–1 integer programs. Math. Program. 85, 439–467 (1999)
Loparic, M., Pochet, Y., Wolsey, L.A.: The uncapacitated lot-sizing problem with sales and safety stocks. Math. Program. 89, 487–504 (2001)
Louveaux, Q., Wolsey, L.A.: Lifting, superadditivity, mixed integer rounding and single node flow sets revisited. 4OR 1, 173–207 (2003)
Love, S.F.: Bounded production and inventory models with piece- wise concave costs. Manag. Sci. 20, 313–318 (1973)
Padberg, M.W., Van Roy, T.J., Wolsey, L.A.: Valid inequalities for fixed charge problems. Math. Program. 33, 842–861 (1985)
Pochet, Y., Wolsey, L.A.: Lot-sizing with constant batches: formulation and valid inequalities. Math. Oper. Res. 18, 767–785 (1993)
Pochet, Y., Wolsey, L.A.: Production Planning by Mixed Integer Programming. Springer, New York (2006)
Stallaert, J.I.A.: The complementary class of generalized flow cover inequalities. Discrete Appl. Math. 77, 73–80 (1997)
van den Heuvel, W., Wagelmans, A.P.M.: Four equivalent lot-sizing models. Oper. Res. Lett. 36, 465–470 (2008)
Van Roy, T.J., Wolsey, L.A.: Valid inequalities for mixed 0–1 Programs. Discrete Appl. Math. 14, 199–213 (1986)
Wagner, H.M., Whitin, T.M.: Dynamic version of the economic lot size model. Manag. Sci. 5, 89–96 (1958)
Wolsey, L.A.: Lot-sizing with production and delivery time windows. Math. Program. 107, 471–489 (2006)
Wolsey, L.A.: Erratum: A tight formulation for uncapacitated lot-sizing with stock upper bounds. Math. Program. 161, 603–609 (2017)
Wolsey, L.A., Yaman, H.: Convex hull results for the warehouse problem. Discrete Optim. 30, 108–120 (2018)
Acknowledgements
Part of the research of the second author was carried out in the Department of Industrial Engineering, Bilkent University, Turkey.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1: Proof of Theorem 3
The proof is by induction. The result holds for \(k=n\). We show that if it holds for k, then it holds for \(k-1\).
For ease of presentation, we introduce \(\pi _u=\min \{x_u, (c-\lambda )z_u\}\) for \(u\in [1,n]\).
1.1 Elimination of \(\alpha ^2_k\) and \(\sigma ^2_k\)
The first step is to use the equations (73) and (74) for \(t=k\) to eliminate \(\alpha ^2_k\) and \(\sigma ^2_k\) by substitution.
Inequality (77) for \(t=k\) becomes
Inequality (78) for \(t=k\) becomes
Inequality (79) for \(t=k\) becomes
Inequality (81) for \(t=k\) becomes
Inequality (82) for \(t=k\) becomes
Inequalities (72) for \(t \in [k+1,n]\) become
1.2 Elimination of \(\alpha ^1_k\)
Now we use Fourier–Motzkin to eliminate \(\alpha ^1_k\). We have to combine the inequalities (108) and (112) with the inequalities (76), (83), (109) and (110).
Inequality (76) with (108) gives
Inequality (76) with (112) gives
Inequality (83) with (112) gives
Inequality (83) with (108) gives
Inequality (109) with (108) gives
These inequalities (115) and (116) together give
Inequality (109) with (112) gives
Inequality (110) with (108) gives
Inequality (110) with (112) gives
Note that inequality (118) is redundant as it is dominated by (114).
1.3 Elimination of \(\sigma ^1_k\)
\(\sigma ^1_k\) occurs in the inequalities (80), (113), (114), (119) and with the opposite sign in (82), (111), (117). We treat inequalities (80) and (113) together as (80) is the same as (113) for \(t=k\).
Combining (82) with (119) gives
Combining (111) with (113) for \(t\in [k,n]\) gives
For \(t=k\), we obtain
For \(t\in [k+1,n]\), these inequalities are redundant. To see this, we take inequality \(s_u -s_{u-1} \le x_u\) for \([u \in k+1,t]\) with weight \((c-\lambda )\) and \(s_t \le b_t\) with weight \(\lambda \). We obtain \(cs_t-(c-\lambda )s_k \le \lambda b_t +(c-\lambda )x_{k+1,t}\). Now \((c-\lambda )x_u \le cx_u\) and as \(x_u \le cz_u\), \((c-\lambda )x_u \le c(c-\lambda )z_u\). Thus \((c-\lambda )x_u \le c \pi _u\) for \(u\in [k+1,t]\) and the inequality is dominated. Combining (117) with (113) for \(t\in [k,n]\) gives
Note that these are inequalities (72) of \(R^{k-1}{\setminus } R^k\).
Combining (82) with (113) for \(t\in [k,n]\) gives
The inequality is redundant for \(t=k\). For \(t\in [k+1, n]\), we obtain the inequalities (71) for \(j=k\) of \(R^{k-1}{\setminus } R^k\).
Note that we have obtained all the inequalities describing \(R^{k-1}\).
It now suffices to show that the remaining inequalities are redundant for \(Q^{k-1} \cap R^{k-1}\).
Combining (82) with (80) and (114) give \( \left\lfloor \frac{b_k}{c} \right\rfloor \ge 0\) and \(x_k +c\sigma ^1_{k-1} \ge 0\), respectively. These are both redundant.
Combining (111) with (119) gives \(c-\lambda \ge 0\), which is redundant.
Combining (111) with (114) gives
Combining (117) with (119) gives
Combining (117) with (114) gives
The last three inequalities are all dominated using (114), \(s_k \le x_k+s_{k-1}\) and (118), \(x_k \le cz_k\) together with \(\pi _k=\min \{x_k, (c-\lambda )z_k\}\) and \(s_{k-1}=c\sigma ^1_{k-1}+(c-\lambda )\sigma ^2_{k-1}\).
Now the result follows by induction as \(R^0 \cap Q^0\) is as claimed in the Theorem. \(\square \)
Appendix 2: Proof of Theorem 4
Suppose that after the elimination of variables for \(t \in [k+1,n]\), we obtain \(P_k\cap R_k\) plus inequalities (106) and (107) for \(t\in [k+1,n]\). We will show that after eliminating \(\mu ^0_k\), \(\mu ^1_k\) and \(\mu ^2_k\), we obtain \(P_{k-1}\cap R_{k-1}\) plus inequalities (106) and (107) for \(t\in [k,n]\).
1.1 Elimination of \(\mu ^0_k\)
The first step is the elimination of \(\mu ^0_k\) by substitution. The inequalities \(\mu ^0_k \le \mu ^0_{k-1} \), \(\mu ^0_k \le \mu ^1_k\), \(\mu ^0_k-\zeta _k \le \left\lfloor \frac{b}{c} \right\rfloor \) and \(\mu ^2_k-\mu ^0_k \le 1\) give
respectively.
1.2 Elimination of \(\mu ^1_k\)
Now we eliminate \(\mu ^1_k\) using Fourier–Motzkin elimination. Overall, after eliminating \(\mu ^0_k\), we are left with \(P_{k-1}\cap R_k\) plus (97), (98), (101), (106), (107), (120), (121), (122) and (123).
The constraints involving \(\mu ^1_k\) are all these inequalities except (97) for \(i=2\) and (101).
As (122) is the same as (106) for \(t=k\), we treat them together.
The resulting inequalities are: (97) and (106) for \(t\in [k,n]\) give
(97) and (107) for \(t\in [k+1,n]\) give
(97) and (121) give \((c-\alpha )\mu ^1_{k-1} \ge \sigma _k-\alpha \mu ^2_k\). This is redundant since adding (126) and \((c-\beta )\) times \(\mu ^1_{k-1} \ge \mu ^0_{k-1}\) gives this inequality.
(98) and (106) for \(t\in [k,n]\) give
(98) and (107) give \((\beta -\alpha )\mu ^2_{k} \ge \sigma _t-(c-\beta +\alpha )(\zeta _t+ \left\lfloor \frac{b}{c} \right\rfloor ) -\alpha \ \ t \in [k+1,n]\). This is redundant as it can be obtained by taking \(b \ge \sigma _t-c\zeta _t \) with weight \(\frac{\alpha }{\beta }\) and (127) for t with weight \(\frac{\beta -\alpha }{\beta }\).
(123) and (106) for \(t\in [k,n]\) give
For \(t\in [k+1,n]\), the inequality is redundant since adding \(\frac{c-\beta }{c-\beta +\alpha }\) times (131) (obtained from (123) and (107) below) and \(\frac{\alpha }{c-\beta +\alpha }\) times \(\sigma _k\ge \sigma _t\) gives this inequality. For \(t=k\), we obtain
(123) and (120) give \(\mu ^2_k \le \mu ^0_{k-1} +1 \). This is redundant since \(\mu ^2_k \le \mu ^2_{k-1} \le \mu ^0_{k-1}+1\).
The resulting formulation is \(P_{k-1}\cap R_k\) plus inequalities
that do not involve \(\mu ^2_k\) and the following families of inequalities that involve \(\mu ^2_k\): (97) for \(i=2\), (130), (132), (131), (129), (101), (128), (126), (127) and (124).
1.3 Elimination of \(\mu ^2_k\)
Here we need to combine the four inequalities (97) for \(i=2\), (130), (132) and (131) with the six inequalities (129), (101), (128), (126), (127) and (124) to eliminate \(\mu ^2_k\).
Combining (97) and (126) gives (90) for \(t=k\):
Combining (97) and (124) gives for \(t\in [k,n]\):
These are inequalities (106) for \(t\in [k,n]\).
Combining (130)and (127) for \(t=k\) gives:
which is (91) for \(t=k\).
Combining (130) and (124) for \(t=k\) gives
This is (107) for \(t=k\). Together with (125), we have (107) for \(t\in [k,n]\).
Combining (132) and (101) gives (92) for \(t=k\):
Combining (131) and (101) gives for \(t\in [k+1,n]\)
These are inequalities (106) since \(\left\lfloor \frac{b-a}{c} \right\rfloor = \left\lfloor \frac{b}{c} \right\rfloor - \left\lfloor \frac{a}{c} \right\rfloor \).
The remaining combinations are redundant:
Combining (97) and (129) gives
It is redundant taking \(\sigma _{k-1}-(c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1} -\alpha \mu ^2_{k-1}=0\) with weight 1, \(\mu ^0_{k-1}-\mu ^1_{k-1} \le 0\) with weight \((c-\beta )\), \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((c-\alpha )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight 1.
Combining (97) and (101) gives
It is redundant taking \(-\zeta _{k-1}+\zeta _k \le 0\) with weight 1 and \(\zeta _{k-1}-\mu ^2_{k-1} \le -\left\lfloor \frac{a}{c} \right\rfloor -1\) with weight 1.
Combining (97) and (128) gives
It is redundant taking \(\sigma _{k-1}-(c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1} -\alpha \mu ^2_{k-1}=0\) with weight 1, \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((\beta -\alpha )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight 1.
Combining (97) and (127) for \(t \in [k,n]\) gives
It is redundant taking (106) \(\sigma _t-(c-\beta )\zeta _t-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1} \le (c-\beta ) \left\lfloor \frac{b}{c} \right\rfloor \) with weight 1 and \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((\beta - \alpha )\).
Combining (130) and (129) gives
This is redundant taking \(\sigma _k-c\zeta _k \le b.\)
Combining (130) and (101) gives
This is redundant as \(a \le b\).
Combining (130) and (128) gives
This is redundant taking \(\sigma _{k-1}-(c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1} -\alpha \mu ^2_{k-1}=0\) with weight \(\frac{c-\beta }{c}\), \(\sigma _k-c\zeta _k \le b\) with weight \(\frac{\beta }{c}\), \( \mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \(\frac{c-\beta }{c}(\beta -\alpha )\), \( \mu ^2_{k-1}-\mu ^0_{k-1} \le 1\) with weight \(\frac{\beta }{c}(c-\beta )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \(\frac{c-\beta }{c}\).
Combining (130) and (126) gives
This is redundant taking \(-(\beta -\alpha )\mu ^1_{k-1}+\sigma _k-(c-\beta +\alpha ) \zeta _k \le (c-\beta +\alpha ) \left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\frac{\alpha }{c-\beta +\alpha }\), \(\sigma _{k-1}-(c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1}=0\) with weight \(\frac{c-\beta }{c-\beta +\alpha }\), \( \mu ^2_{k-1}-\mu ^0_{k-1} \le 1\) with weight \(\frac{\alpha (c-\beta )}{c-\beta +\alpha }\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \(\frac{c-\beta }{c-\beta +\alpha }\).
Combining (130) and (127) for \(t\in [k+1,n]\) gives
This is redundant taking \(\sigma _k-c\zeta _k \le b\) with weight \(\frac{\beta }{c}\), \(\sigma _t-c\zeta _t \le b\) with weight \(\frac{c-\beta }{c}\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\frac{\beta }{c}\) for \(j \in [k+1,t]\).
Combining (130) and (124) for \(t\in [k+1,n]\) gives
This is redundant taking ((107) for k) \(-(\beta -\alpha )\mu ^1_{k-1}+\sigma _k -(c-\beta +\alpha )\zeta _k \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\frac{\alpha }{c-\beta +\alpha }\), ((107) for t) \(-(\beta -\alpha )\mu ^1_{k-1}+\sigma _t -(c-\beta +\alpha )\zeta _t \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(1-\frac{\alpha }{c-\beta +\alpha }\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight\(\frac{\alpha }{c-\beta +\alpha }\) for \(j \in [k+1,t]\).
Combining (132) and (129) gives
which is redundant.
Combining (132) and (128) gives
This is redundant taking \(\sigma _{k-1}- (c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1}=0\) with weight \((c-\beta )\), \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((c-\beta )(\beta -\alpha )\), \(\mu ^2_{k-1}-\mu ^0_{k-1} \le 1 \) with weight \((c-\beta )\beta \), \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \((c-\beta )\)and \( (c-\beta )\beta \le (c-\alpha )\beta \) with weight 1.
Combining (132) and (126) gives
This is redundant taking \(\sigma _{k-1}- (c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1}=0\) with weight \((c-\alpha )\), \(\mu ^0_{k-1}-\mu ^1_{k-1} \le 0\) with weight \(\alpha (\beta -\alpha )\), \(\mu ^2_{k-1}-\mu ^0_{k-1} \le 1 \) with weight \(\alpha (c-\alpha )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \((c-\alpha )\).
Combining (132) and (127) for \(t\in [k,n]\) gives
This is redundant taking \(\sigma _t-c\zeta _t \le b\) with weight \((c-\beta )\), \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\beta \) for \(j \in [k+1,t]\), \(b(c-\beta )= \beta (c-\beta )+(c-\beta )c\left\lfloor \frac{b}{c} \right\rfloor \) with weight 1 and \(\beta (c-\beta ) \le \beta (c-\alpha )\) with weight 1.
Combining (132) and (124) for \(t\in [k,n]\) gives
This is redundant taking ((107) for t) \(\sigma _t-(c-\beta +\alpha )\zeta _t - (\beta -\alpha )\mu ^1_{k-1} \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\frac{c(c-\beta )}{c-\beta +\alpha }\), \(\sigma _{k-1}- (c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1}=0\) with weight \(\frac{\alpha (\beta -\alpha )}{c-\beta +\alpha }\), \(\mu ^0_{k-1}-\mu ^1_{k-1} \le 0\) with weight \(\alpha (\beta -\alpha )\), \(\mu ^2_{k-1}-\mu ^0_{k-1} \le 1 \) with weight \(\frac{\alpha ^2(\beta -\alpha )}{c-\beta +\alpha }\), \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \(\frac{\alpha (\beta -\alpha )}{c-\beta +\alpha }\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\frac{\alpha c}{c-\beta +\alpha }\) for \(j \in [k+1,t]\).
Combining (131) for \(t\in [k+1,n]\) and (129) gives
This is redundant taking \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \((\beta -\alpha )\) for \(j \in [k+1,t]\), \(\sigma _t-c\zeta _t \le b\) with weight \((c-\beta +\alpha )\) and \(\beta \le c\) with weight \(c-\beta +\alpha \).
Combining (131) for \(t\in [k+1,n]\) and (128) gives
Case 1. \(c-2\beta +\alpha \ge 0\)
This is redundant taking ((107) for t) \(\sigma _t-(c-\beta +\alpha )\zeta _t-(\beta -\alpha )\mu ^1_{k-1} \le (c-\beta +\alpha ) \left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\beta \), \(\sigma _{k-1}-(c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1} -\alpha \mu ^2_{k-1} =0 \) with weight \(c-2\beta +\alpha \), \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((\beta -\alpha )(c-\beta +\alpha )\), \(-\mu ^0_{k-1}+\mu ^2_{k-1} \le 1\) with weight \(\beta (c-\beta )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \(c-2\beta +\alpha \).
Case 2. \(c-2\beta +\alpha < 0\)
This is redundant taking \(\sigma _t-c \zeta _t \le b\) with weight \(\frac{(c-\beta +\alpha )(2 \beta -c-\alpha )}{\beta -\alpha }\), \(\sigma _t-(c-\beta +\alpha )\zeta _t-(\beta -\alpha )\mu ^1_{k-1} \le (c-\beta +\alpha ) \left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\frac{(c-\beta +\alpha )(c-\beta )}{\beta -\alpha }\), \(\mu ^1_{k-1}-\mu ^2_{k-1} \le 0\) with weight \((c-\beta )(c-\beta +\alpha )\), \(-\mu ^0_{k-1}+\mu ^2_{k-1} \le 1\) with weight \((c-\beta )(c-\beta +\alpha )\)and \(-\sigma _{j-1}+\sigma _j \le 0\) for \(j \in [k+1,t]\) with weight \(2 \beta -c-\alpha \).
Combining (131) for \(t\in [k+1,n]\) and (126) gives
This is redundant taking ((107) for t) \(-(\beta -\alpha )\mu ^1_{k-1}+\sigma _t-(c-\beta +\alpha )\zeta _t \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\alpha \), \(\sigma _{k-1}- (c-\beta )\mu ^0_{k-1}-(\beta -\alpha )\mu ^1_{k-1}-\alpha \mu ^2_{k-1}=0\) with weight \((c-\beta )\), \(\mu ^2_{k-1}-\mu ^0_{k-1} \le 1 \) with weight \(\alpha (c-\beta )\) and \(-\sigma _{k-1}+\sigma _k \le 0\) with weight \((c-\beta )\).
Combining (131) for \(t\in [k+1,n]\) and (127) for \(q\in [k,n]\) gives
Case 1. \(t>q\)
This is redundant taking \(\sigma _t-c\zeta _t \le b\) with weight \((c-\beta +\alpha )\beta /c\), \(\sigma _q-c\zeta _q \le b\) with weight \((c-\beta +\alpha )(c-\beta )/c\), \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\beta \) for \(j \in [k+1,q]\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\beta (\beta -\alpha )/c\) for \(j \in [q+1,t]\).
Case 2. \(t\le q\)
This is redundant taking \(\sigma _t-c\zeta _t \le b\) with weight \((c-\beta +\alpha )\beta /c\), \(\sigma _q-c\zeta _q \le b\) with weight \((c-\beta +\alpha )(c-\beta )/c\), \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\beta \) for \(j \in [k+1,t]\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\beta (c-\beta +\alpha )/c\) for \(j \in [t+1,q]\).
Combining (131) for \(t \in [k+1,n]\) and (124) for \(q \in [k,n]\) gives
This is redundant taking ((107) for t) \(-(\beta -\alpha ) \mu ^1_{k-1}+\sigma _t-(c-\beta +\alpha )\zeta _t \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \(\alpha \), ((107) for q) \(-(\beta -\alpha ) \mu ^1_{k-1}+\sigma _q-(c-\beta +\alpha )\zeta _q \le (c-\beta +\alpha )\left\lfloor \frac{b}{c} \right\rfloor +\alpha \) with weight \((c-\beta )\) and \(-\sigma _{j-1}+\sigma _j \le 0\) with weight \(\alpha \) for \(j \in [k+1,q]\).
The iteration is complete and we obtain the formulation with k replaced by \(k-1\).
\(\square \)
Rights and permissions
About this article
Cite this article
Wolsey, L.A., Yaman, H. Convex hull results for generalizations of the constant capacity single node flow set. Math. Program. 187, 351–382 (2021). https://doi.org/10.1007/s10107-020-01481-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10107-020-01481-6
Keywords
- Single node flow set
- Flow cover inequalities
- Lot-sizing with sales
- Convex hull
- Extended formulation
- Fourier–Motzkin elimination