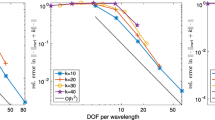
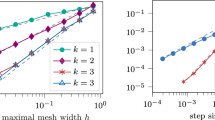
Abstract
We consider time-harmonic Maxwell’s equations set in a heterogeneous medium with perfectly conducting boundary conditions. Given a divergence-free right-hand side lying in \(L^2\), we provide a frequency-explicit approximability estimate measuring the difference between the corresponding solution and its best approximation by high-order Nédélec finite elements. Such an approximability estimate is crucial in both the a priori and a posteriori error analysis of finite element discretizations of Maxwell’s equations, but the derivation is not trivial. Indeed, it is hard to take advantage of high-order polynomials given that the right-hand side only exhibits \(L^2\) regularity. We proceed in line with previously obtained results for the simpler setting of the scalar Helmholtz equation and propose a regularity splitting of the solution. In turn, this splitting yields sharp approximability estimates generalizing known results for the scalar Helmholtz equation and showing the interest of high-order methods.
Similar content being viewed by others
Notes
The authors believe it is of interest to explicitly mention \(c_s\) proofs, since at least in principle, the regularity splitting results may apply in cases where \(c_s\) is not obtain via Theorem 1.
References
Adams, R., Fournier, J.: Sobolev Spaces. Academic Press, New York (2003)
Assous, F., Ciarlet, P., Jr., Labrunie, S.: Mathematical Foundations of Computational Electromagnetism, Applied Mathematical Sciences, vol. 198. Springer, Cham (2018)
Bériot, H., Prinn, A., Gabard, G.: Efficient implementation of high-order finite elements for Helmholtz problems. Int. J. Numer. Methods Eng. 106, 213–240 (2016)
Chaumont-Frelet, T.: Mixed finite element discretization of acoustic Helmholtz problems with high wavenumbers. Calcolo 56, 1–27 (2019)
Chaumont-Frelet, T., Ern, A., Vohralík, M.: On the derivation of guaranteed and \(p\)-robust a posteriori error estimates for the Helmholtz equation. Numer. Math. 148, 525–573 (2021)
Chaumont-Frelet, T., Nicaise, S.: Wavenumber explicit convergence analysis for finite element discretizations of general wave propagation problems. IMA J. Numer. Anal. 40, 1503–1543 (2020)
Chaumont-Frelet, T., Nicaise, S., Pardo, D.: Finite element approximation of electromagnetic fields using nonfitting meshes for Geophysics. SIAM J. Numer. Anal. 56, 2288–2321 (2018)
Dörfler, W., Sauter, S.: A posteriori error estimation for highly indefinite Helmholtz problems. Comput. Methods Appl. Math. 13, 333–347 (2013)
Ern, A., Guermond, J.-L.: Semiclassical Analysis, Graduate Studies in Mathematics, vol. 138. American Mathematical Society (2012)
Ern, A., Guermond, J.-L.: Analysis of the edge finite element approximation of the Maxwell equations with low regularity solutions. Comput. Math. Appl. 75, 918–932 (2018)
Ern,A., Guermond, J.-L.: Finite Elements I. Approximation and Interpolation, Texts in Applied Mathematics, vol. 72. Springer Nature Switzerland (2021)
Fernandes, P., Gilardi, G.: Magnetostatic and electrostatic problems in inhomogeneous anisotropic media with irregular boundary and mixed boundary conditions. Math. Methods Appl. Sci. 47, 2872–2896 (1997)
Gallistl, D., Olkhovskiy, V.: Computational lower bounds for the Maxwell eigenvalues (2021). arXiv:2110.02605
Girault, V., Raviart, P.A.: Finite Element Methods for Navier-Stokes Equations: Theory and Algorithms, Springer (1986)
Ihlenburg, F., Babuška, I.: Finite element solution of the Helmholtz equation with high wave number. Part II: the \(h\)-\(p\)-version of the FEM. SIAM J. Numer. Anal. 34, 315–358 (1997)
Lafontaine, D., Spence, E.A., Wunsch, J.: Wavenumber-explicit convergence of the \(hp\)-FEM for the full-space heterogeneous Helmholtz equation with smooth coefficients (2020). arXiv:2010.00585
Melenk, J.M., Sauter, S.: Wavenumber explicit convergence analysis for Galerkin discretizations of the Helmholtz equation. SIAM J. Numer. Anal. 49, 1210–1243 (2011)
Melenk, J.M., Sauter, S.A.: Wavenumber-explicit \(hp\)-FEM analysis for Maxwell’s equations with transparent boundary conditions. Found. Comput. Math. 21, 125–241 (2021)
Sauter, S., Zech, J.: A posteriori error estimation of \(hp\)-dG finite element methods for highly indefinite Helmholtz problems. SIAM J. Numer. Anal. 53, 2414–2440 (2015)
Schatz, A.H.: An observation concerning Ritz–Galerkin methods with indefinite bilinear forms. Math. Comput. 28, 959–962 (1974)
Taus, M., Zepeda-Núñez, L., Hewett, R., Demanet, L.: Pollution-free and fast hybridizable discontinuous Galerkin solvers for the high-frequency Helmholtz equation. In: Proc, SEG annual meeting (Houston) (2017)
Weber, C.: A local compactness theorem for Maxwell’s equations. Math. Methods Appl. Sci. 2, 12–25 (1980)
Weber, C.: Regularity theorems for Maxwell’s equations. Math. Methods Appl. Sci. 3, 523–536 (1981)
Zhong, L., Shu, S., Wittum, G., Xu, J.: Optimal error estimates for Nédélec edge elements for time-harmonic Maxwell’s equations. J. Comput. Math. 27, 563–572 (2009)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Chaumont-Frelet, T., Vega, P. Frequency-explicit approximability estimates for time-harmonic Maxwell’s equations. Calcolo 59, 22 (2022). https://doi.org/10.1007/s10092-022-00464-7
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10092-022-00464-7