Abstract
We investigate whether and how an individual giving decision is affected in risky environments in which the recipient’s wealth is random. We demonstrate that, under risk neutrality, the donation of dictators with a purely ex post view of fairness should, in general, be affected by the riskiness of the recipient’s payoff, while dictators with a purely ex ante view should not be. Furthermore, we observe that some influential inequality aversion preferences functions yield opposite predictions when we consider ex post view of fairness. Hence, we report on dictator games laboratory experiments in which the recipient’s wealth is exposed to an actuarially neutral and additive background risk. Our experimental data show no statistically significant impact of the recipient’s risk exposure on dictators’ giving decisions. This result appears robust to both the experimental design (within subjects or between subjects) and the origin of the recipient’s risk exposure (chosen by the recipient or imposed on the recipient). Although we cannot sharply validate or invalidate alternative fairness theories, the whole pattern of our experimental data can be simply explained by assuming ex ante view of fairness and risk neutrality.


Similar content being viewed by others
Notes
Precisely, in Engel and Goerg (2018)’s “baseline” risk-free treatment, the dictator is endowed with €10 (against €15 in T1) and the recipient is endowed with €5 (as in T1). In their “symmetry” risky treatment, the dictator is endowed with €10 (against €15 in T2), and the recipient has a risky endowment taking either value €0 or value €10 with equal probability (as in T2).
Dictators with spiteful preferences would, of course, also give zero in all our treatments.
The certainty equivalent of \({\widetilde{r}}(g)\) is \(u^{-1}\left( Eu\left( {\widetilde{r}}(g)\right) \right) \).
The proof is given in the appendix.
The proof is given in appendix.
The proof is given in the appendix.
The instructions of both designs are presented in the appendix.
Random incentives have sometimes been noted for undermining their saliency. Subjects would calculate the expected value of each single decision, which may reduce the significance of any incentives provided. However, several studies do not find a difference between random and deterministic incentives (Starmer and Sugden 1991; Beattie and Loomes 1997; Cubitt et al. 1998).
Given a sample size of 57 subjects per treatment and a common standard deviation of 2, the difference required to detect it as significantly different from zero at a 5% level (Type I error) and with a power of the test at 80% (Type II error) is 1.05. The power of finding a difference of 0.44, as between T1 and T2 in Table 1 is approximately 25%, which is rather low.
Except for the proportion of those equally splitting the endowment, the differences between treatments are not significant.
We only present the main results in Table 2, but we also performed a series of additional regressions in which we interact our treatments variables with risk preferences. These regressions do not add qualitative results. All the results are also robust to the use of other specifications such as OLS, robust estimators and sessions clustered standard errors.
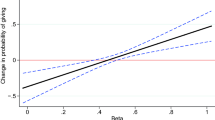
The correlation between the two variables is 0.301 and significantly different than zero at 5%. A linear regression explaining the belief about the recipientï¿\(\frac{1}{2}\) s risk preference by choice of the endowment by the recipient gives a very significant positive coefficient (p-value<1%). These results raise the question of multicollinearity if we use both variables in the same specification.
We did not introduce the possibility for the recipient to choose between a certain and a risky endowment since we did not observe a significant difference with the two first treatments in the between-subject design. It allows us also to control for order effects more easily.
For details, see the instructions in the appendix.
There is no significant difference between T1 and T2 when both are played first (MS: \(p=0.579\); ES: \(p=0.110\)) or when both are played second (MS: \(p=0.569\); ES: \(p=0.501\)).
Although we do not observe differences between our two experimental designs, we must acknowledge that the decision making is not fully comparable since in the within-subjects design, participants had to make three decisions, instead of two in the between-subject design, with only one being actually paid.
References
Beattie J, Loomes G (1997) The impact of incentives upon risky choice experiments. J Risk Uncertain 14(2):155–68
Beaud M, Willinger M (2015) Are people risk vulnerable? Manage Sci 61(3):624–636
Bolton GE, Ockenfels A (2000) ERC: a theory of equity, reciprocity, and competition. Am Econom Rev 90(1):166–193
Bolton GE, Ockenfels A (2010) Betrayal aversion: evidence from Brazil, China, Oman, Switzerland, Turkey, and the United States: comment. Am Econom Rev 100(1):628–33
Brock JM, Lange A, Ozbay EY (2013) Dictating the risk: experimental evidence on giving in risky environments. Am Econ Rev 103(1):415–37
Cappelen AW, Konow J, Sorensen E, Tungodden B (2013) Just luck: an experimental study of risk-taking and fairness. Am Econom Rev 103(4):1398–1413
Cettolin E, Riedl A, Tran G (2017) Giving in the face of risk. J Risk Uncertain 55(2–3):95–118
Chowdhury SM, Jeon JY (2014) Impure altruism or inequality aversion?: An experimental investigation based on income effects. J Public Econ 118(C):143–150
Cubitt R, Starmer C, Sugden R (1998) On the validity of the random lottery incentive system. Exp Econ 1(2):115–131
Eckel CC, Grossman PJ (1996) Altruism in anonymous dictator games. Games Econom Behav 16(2):181–191
Engel C (2011) Dictator games: a meta study. Exp Econ 14(4):583–610
Engel C, Goerg SJ (2018) If the worst comes to the worst: dictator giving when recipient’s endowments are risky. Eur Econ Rev 105:51–70
Falk A, Fehr E, Fischbacher U (2008) Testing theories of fairness-intentions matter. Games Econom Behav 62(1):287–303
Fehr E, Schmidt KM (1999) A theory of fairness, competition, and cooperation. Q J Econ 114(3):817–868
Fong M, Oberholzer-Gee F (2011) Truth in giving: experimental evidence on the welfare effects of informed giving to the poor. J Public Econ 95(5):436–444
Frechette G (2012) Session-effects in the laboratory. Exp Econ 15(3):485–498
Fudenberg D, Levine D (2012) Fairness, risk preferences and independence: impossibility theorems. J Econom Behavior Organiz 81(2):606–612
Gneezy U, Potters J (1997) An experiment on risk taking and evaluation periods. Q J Econ 112(2):631–645
Gächter S, Renner E (2010) The effects of (incentivized) belief elicitation in public goods experiments. Exp Econ 13(3):364–377
Kahneman D, Knetsch JL, Thaler RH (1986) Fairness and the assumptions of economics. J Business 59(4):285–300
Korenok O, Millner EL, Razzolini L (2012) Are dictators averse to inequality? J Econom Behavior Organiz 82(2):543–547
Krawczyk M, Lec FL (2010) ‘Give me a chance!’ an experiment in social decision under risk. Exp Econ 13(4):500–511
Palfrey T, Wang S (2009) On eliciting beliefs in strategic games. J Econom Behavior Organiz 71(2):98–109
Saito K (2013) Social preferences under risk: equality of opportunity versus equality of outcome. Am Econom Rev 103(7):3084–3101
Starmer C, Sugden R (1991) Does the random-lottery incentive system elicit true preferences? an experimental investigation. Am Econom Rev 81(4):971–78
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This paper forms part of the research project RISKADAPT (Contract ANR-17-CE02-0011) of the French National Agency for Research (ANR) whose financial support is gratefully acknowledged. This work was also supported by the French National Research Agency Grant ANR-17-EURE-0020, and by the Excellence Initiative of Aix-Marseille University - A*MIDEX. It has also been supported by LabEx Entrepreneurship (Contract ANR-10-LABX-11-01). The authors would like to thank the editor and two referees for their helpful comments. They also thank participants of seminars at PSE (Paris), GATE (Lyon-Saint-Etienne) and CREM (Rennes) and the ASFEE conference 2017 (Rennes).
Appendix
Appendix
1.1 Proof of Proposition 2
We first consider Fehr and Schmidt (1999)’s preferences function. Substituting \( d(g)=15-g\) and \(r(g)=5+g-\pi \) in (5), the dictator’s preferences function is
or, equivalently,
where \(\alpha \ge \beta \) and \(0\le \beta <1\). Observe that \(u_{3}^{\prime }=u_{1}^{\prime }\). Therefore, if (i) \(\frac{1}{2}<\beta <1\), \(u_{3}\) is first increasing and then decreasing above the kink point at \(5+\frac{1}{2} \pi \), where \(u_{3}(5+\frac{1}{2}\pi )=10-\frac{1}{2}\pi \). The optimal donations is \(g_{3}^{*}=5+\frac{1}{2}\pi \). If (ii) \(\beta =\frac{1}{2}\) , \(u_{3}\) is first flat, then decreasing above the kink point. The optimal donation is between 0 and \(5+\frac{1}{2}\pi \) in this very special case. Let us assume that the optimal donation is \(g_{1}^{*}=5+\frac{1}{2}\pi \) . If (iii) \(0\le \beta <\frac{1}{2}\), \(u_{3}\) is everywhere decreasing. The optimal donations is \(g_{3}^{*}=0\).
Under risk aversion (\(\pi >0\)), we have shown that \(g_{1}^{*}=5<5+\frac{1 }{2}\pi =g_{3}^{*}\) in case (i), \(g_{1}^{*}=5<5+\frac{1}{2}\pi =g_{3}^{*}\) in case (ii), and \(g_{1}^{*}=g_{3}^{*}=0\) in case (iii). Therefore we have shown that \(g_{1}^{*}\le g_{3}^{*}\) everywhere, that is, for any parameter value of the Fehr and Schmidt (1999)’s preferences function. The opposite result obviously holds under risk seeking (\(\pi <0\)).
We now consider Bolton and Ockenfels (2000)’s preferences function. Substituting \( d(g)=15-g\) and \(r(g)=5+g-\pi \) in (6), the dictator’s preferences function is
where \(a\ge 0\) and \(b>0\). The Kuhn-Tucker first order conditions are:
Because \(v_{3}^{\prime }\left( 10\right) \ge 0\) is equivalent to \(a\le \frac{\frac{1}{2}\pi -5}{\left[ 20-\pi \right] ^{2}}b\), where \(\pi <5\), we conclude that \(g_{1}=10\) cannot be an optimal choice. On the other hand, we could have \(v_{3}^{\prime }\left( 0\right) \le 0\), which is equivalent to \( \frac{a}{b}\ge \frac{5+\frac{1}{2}\pi }{\left[ 20-\pi \right] ^{2}}\). The optimal donation is
We observe that \(g_{3}^{*}\le 5+\frac{1}{2}\pi \), and that the treshold \(\frac{5+\frac{1}{2}\pi }{\left[ 20-\pi \right] ^{2}}\) increases with \(\pi \).
Under risk aversion, with \(\pi \in \left( 0,5\right) \), we have shown that: (i) \(g_{1}^{*}=g_{3}^{*}=0\) if \(\frac{a}{b}\ge \frac{5+\frac{1}{2} \pi }{\left[ 20-\pi \right] ^{2}}\), (ii) \(g_{1}^{*}=0<g_{3}^{*}\) if \( \frac{1}{80}\le \frac{a}{b}<\frac{5+\frac{1}{2}\pi }{\left[ 20-\pi \right] ^{2}}\), and (iii) \(g_{1}^{*}<g_{3}^{*}\) if \(0\le \frac{a}{b}<\frac{ 1}{80}\). Therefore we have shown that \(g_{1}^{*}\le g_{3}^{*}\) everywhere, that is, for any parameter value of the Bolton and Ockenfels (2000)’s preferences function. The opposite result obviously holds under risk seeking , with \(\pi \in \left( -5,0\right) \). This concludes the proof.
1.2 Proof of proposition 3
We first consider the case where the recipient’s endowment is certain, as in T1. Substituting \(d(g)=15-g\) and \(r(g)=5+g\) in (4), the dictator’s preferences function is
or, equivalently,
where \(\alpha \ge \beta \) and \(0\le \beta <1\). Observe that \(u_{1}^{\prime }=2\beta -1\) for \(g<5\) and \(u_{1}^{\prime }=-2\alpha -1\) for \(g>5\). Therefore, if (i) \(\frac{1}{2}<\beta <1\), \(u_{1}\) is first increasing, then decreasing above a kink point at \(g=5\) (where \(u_{1}(5)=10\)). The optimal donation is \(g_{1}^{*}=5\). If (ii) \(\beta =\frac{1}{2}\), \(u_{1}\) is first flat, then decreasing above the kink point at \(g=5\). The optimal donation is between 0 and 5 in this very special case. Let us assume that the optimal donation is \(g_{1}^{*}=5\). If (iii) \(0\le \beta <\frac{ 1}{2}\), \(u_{1}\) is everywhere decreasing. The optimal donation is \( g_{1}^{*}=0\).
Now suppose that the recipient’s endowment is risky, as in T2. Substituting \( d(g)=15-g\) and \({\widetilde{r}}(g)=(10+g,\frac{1}{2};g,\frac{1}{2})\) in (5), the dictator’s preferences function is
or, equivalently,
where \(\alpha \ge \beta \) and \(0\le \beta <1\). Observe that \(u_{2}^{\prime }=2\beta -1\) for \(g<\frac{5}{2}\), \(u_{2}^{\prime }=-\alpha +\beta -1\) for \( \frac{5}{2}<g<\frac{15}{2}\) and \(u_{2}^{\prime }=-2\alpha -1\) for \(g>\frac{15 }{2}\). Therefore, if (i) \(\frac{1}{2}<\beta <1\), \(u_{2}\) is first increasing and then decreasing above the kink point at \(g=\frac{5}{2}\) (where \( u_{2}\left( \frac{5}{2}\right) =-5\beta +\frac{25}{2}\)). The optimal donation is \(g_{2}^{*}=2\), with \(u_{2}\left( 2\right) =-6\beta +13>- \frac{1}{2}\alpha -\frac{9}{2}\beta +12=u_{2}\left( 3\right) \)). If (ii) \( \beta =\frac{1}{2}\), \(u_{2}\) is first flat and then decreases above the kink point. The optimal donation is between 0 and 2 in this very special case. Let us assume that the optimal donation is \(g_{1}^{*}=2\). If (iii) \(0\le \beta <\frac{1}{2}\), \(u_{2}\) is everywhere decreasing. The optimal donation is \(g_{2}^{*}=0\).
We have shown that \(g_{1}^{*}=5>2=g_{2}^{*}\) in case (i), \( g_{1}^{*}=5>2=g_{2}^{*}\) in case (ii), and \(g_{1}^{*}=g_{2}^{*}=0\) in case (iii). Therefore we have shown that \(g_{1}^{*}\ge g_{2}^{*}\) everywhere, that is, for any parameter value of the Fehr and Schmidt (1999)’s preferences function. This concludes the proof.
1.3 Proof of Proposition 4
We first consider the case where the recipient’s endowment is certain, as in T1. Substituting \(d(g)=15-g\) and \(r(g)=5+g\) in (6), the dictator’s preferences function is
where \(a\ge 0\) and \(b>0\). The Kuhn and Tucker’s first-order conditions are
Because \(v_{1}^{\prime }\left( 10\right) \ge 0\) is equivalent to \(80a\le -b \), we conclude that \(g_{1}=10\) cannot be an optimal choice. On the other hand we could have \(v_{1}^{\prime }\left( 0\right) \le 0\), which is equivalent to \(b\le 80a\). The optimal donation is
We observe that \(g_{1}^{*}\le 5\).
Now suppose that the recipient’s endowment is risky, as in T2. Substituting \( d(g)=15-g\) and \({\widetilde{r}}(g)=(10+g,\frac{1}{2};g,\frac{1}{2})\) in (7), the dictator’s preferences function is
The Kuhn and Tucker’s first-order conditions are
Because \(v_{2}^{\prime }\left( 10\right) \ge 0\ \)is equivalent to \( 1125a\le -13b\), we conclude that \(g_{2}^{*}=10\) cannot be an optimal choice. On the other hand we could have \(v_{2}^{\prime }\left( 0\right) \le 0\), which is equivalent to \(7b\le 375a\). The optimal donation is
We observe that \(g_{2}^{*}\le \frac{105}{17}\simeq 6,2\).
We have shown that: (i) \(g_{1}^{*}=g_{2}^{*}=0\) if \(\frac{a}{b}\ge \frac{7}{375}\), (ii) \(g_{1}^{*}=0<g_{2}^{*}\) if \(\frac{1}{80}\le \frac{a}{b}<\frac{7}{375}\), and (iii) \(g_{1}^{*}<g_{2}^{*}\) if \( 0\le \frac{a}{b}<\frac{1}{80}\). Therefore we have shown that \(g_{1}^{*}\le g_{2}^{*}\) everywhere, that is, for any parameter value of the Bolton and Ockenfels (2000)’s preferences function. This concludes the proof.
1.4 Between-subject design: instructions for the benchmark riskless treatment T1
This is a translation of the original French version.
You are about to participate in an experiment to study decision making. Please carefully read the instructions, they should help you understand the experiment. All your decisions are anonymous. You will enter your choices in the computer in front of you.
The present experiment consists of two parts: “Part 1” and “Part 2”. The instructions for Part 1 are included hereafter. The instructions for Part 2 will be distributed once everybody has completed Part 1. At the end of the experiment, one of the two parts will be randomly drawn for real pay. You will then be paid in cash your earnings in euros.
During the experiment, you are not allowed to communicate. If you have questions, then please raise your hand, and one experimenter will come to you and answer your question in private.
In this experiment, there were two types of subjects (in equal number): player A and player B. You are randomly assigned a type for the entire experiment. It will be revealed privately before starting the experiment. You will be randomly paired to a player of another type than yours such that one player A is matched with one player B.
Part 1
At the beginning of the game, each player, whatever his/her type, receives an endowment of 5 euros.
Additionally, players A have to share 10 euros between them, and the players B they are paired with. Players A are free to send player B any amount between 0 and 10. The only compulsory limitation is that the amounts are integers (0, 1, 2, etc.). Playersï¿\(\frac{1}{2}\) B have no decision to make.
The earnings for each type of player are as follows:
-
Player A’s earnings: 5 euros + (10 euros - the amount sent to player B).
-
Player B’s earnings: 5 euros + the amount received from player A.
Example 1: If player A sent 3 euros to the player B.
-
Player A’s earnings: 5 + (10 - 3) = 12 euros.
-
Player B’s earnings: 5 + 3 = 8 euros.
Example 2: If player A sent 5 euros to the player B.
-
Player A’s earnings: 5 + (10 - 5) = 10 euros.
-
Player B’s earnings: 5 + 5 = 10 euros.
Example 3: If player A sent 7 euros to the player B.
-
Player A’s earnings: 5 + (10 - 7) = 8 euros.
-
Player B’s earnings: 5 + 7 = 12 euros.
Part 2 (given at the end of Part 1, identical for all treatments)
Part 2 is independent from Part 1.
In this part of the experiment, you receive an endowment of 10 euros. You must decide which part of this amount you wish to invest in a risky option. You can choose any amount (in integer only) between 0 and 10.
The risky option consists of a coin toss. The risky option will bring you 3 times the invested amount if heads are drawn and 0 if tails are drawn.
Your final earnings are equal to the amount kept + the earnings of your investment.
Examples:
-
1.
If you decide to invest 5 euros, your earnings will be 20 euros if Heads is drawn (5 euros + 3*5 euros invested in the risky option) and 5 euros if tails are drawn (5 euros + 0*5 euros from your investment in the risky option).
-
2.
If you decide to invest 0 euro, your final earnings will be 10 euros whatever the result of the coin toss.
-
3.
If you decide to invest 10 euros. Your final earnings will be 30 euros if Heads is drawn (0 euro + 3*10 euros invested in the risky option) and 0 euro if Tails is drawn (0 euro kept + 0*10 euros from your investment in the risky option).
To avoid calculations, the table below displays the earnings according to the amount invested in the risky option and the coin toss result.
Investment | Earnings | |
|---|---|---|
If Heads | If Tails | |
0 | 10 | 10 |
1 | 12 | 9 |
2 | 14 | 8 |
3 | 16 | 7 |
4 | 18 | 6 |
5 | 20 | 5 |
6 | 22 | 4 |
7 | 24 | 3 |
8 | 26 | 2 |
9 | 28 | 1 |
10 | 30 | 0 |
1.5 Between-subject design: instructions of Part 1 for the risky treatment T2
Part 1
At the beginning of the game, player A receives an endowment of 5 euros.
The endowment of player B is risky and depends on coin toss result. If the result of the coin toss is Tails, player B has an endowment of 0 euro. If the result is Heads, player B receives an endowment of 10 euros. The coin toss result will only be known at the end of the experiment.
Additionally, players A have to share 10 euros between them, and the players B they are paired with. The players A are free to send player B any amount between 0 and 10. The only compulsory limitation is that the amounts are integers (0, 1, 2, etc.). Players B have no decision to make.
The earnings for each type of player are as follows:
-
Player A’s earnings: 5 euros + (10 euros - the amount sent to player B).
-
Player B’s earnings depend on the result of the coin toss:
-
If Tails, player B’s earnings: 0 euros + the amount received from the player A.
-
If Heads, player B’s earnings: 10 euros + the amount received from the player A.
-
Example 1: If player A sent 3 euros to the player B:
-
Player A’s earnings: 5 + (10 - 3) = 12 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 3 = 3 euros.
-
If Heads, player B’s earnings: 10 + 3 = 13 euros.
-
Example 2: If player A sent 5 euros to player B:
-
Player A’s earnings: 5 + (10 - 5) = 10 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 5 = 5 euros.
-
If Heads, player B’s earnings: 10 + 5 = 15 euros.
-
Example 3: If player A sent 7 euros to player B:
-
Player A’s earnings: 5 + (10 - 7) = 8 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 7 = 7 euros.
-
If Heads, player B’s earnings: 10 + 7 = 17 euros.
-
1.6 Between-subject design: instructions of part 1 for the choice treatment T3
Part 1
At the beginning of the game, player A receives an endowment of 5 euros.
Player B must make a choice between two alternatives:
-
Choice 1: an endowment of 5 euros
-
Choice 2: a risky endowment that depends on the result of a coin toss. If the result of the coin toss is Tails, player B has an endowment of 0 euro. If the result is Heads, player B receives an endowment of 10 euros. The coin toss results will only be known at the end of the experiment.
Player A and player B are informed about the choice (1 or 2) made by player B.
Additionally, players A have to share 10 euros between them, and the players B they are paired with. The players A are free to send player B any amount between 0 and 10. The only compulsory limitation is that the amounts are integers (0, 1, 2, etc. ).
The earnings for each type of player are as follows:
-
Player A’s earnings: 5 euros + (10 euros - the amount sent to player B).
-
Player B’s earnings depend on the choice:
-
If player B made choice 1, player B’s earnings: 5 euros + the amount received from the player A.
-
If player B made choice 2, player B’s earnings depend on the result of the coin toss:
-
*If Tails, player B’s earnings: 0 euros + the amount received from the player A.
-
*If Heads, player B’s earnings: 10 euros + the amount received from the player A.
-
-
Example 1: If player A sent 3 euros to the player B:
-
Player A’s earnings: 5 + (10 - 3) = 12 euros.
-
Player B’s earnings depend on the choice:
-
If player B made the choice 1: 5 + 3 = 8 euros.
-
If player B made choice 2, player B’s earnings depend on the result of the coin toss:
-
*If Tails, player B’s earnings: 0 + 3 = 3 euros.
-
*If Heads, player B’s earnings: 10 + 3 = 13 euros.
-
-
Example 2: If player A sent 5 euros to player B:
-
Player A’s earnings: 5 + (10 - 5) = 10 euros.
-
Player B’s earnings depend on the choice:
-
If player B made the choice 1: 5 + 5 = 10 euros.
-
If player B made choice 2, player B’s earnings depend on the result of the coin toss:
-
*If Tails, player B’s earnings: 0 + 5 = 5 euros.
-
*If Heads, player B’s earnings: 10 + 5 = 15 euros.
-
-
Example 3: If player A sent 7 euros to player B:
-
Player A’s earnings: 5 + (10 - 7) = 8 euros.
-
Player B’s earnings depend on the choice:
-
If player B made the choice 1: 5 + 7 = 12 euros
-
If player B made choice 2, player B’s earnings depend on the result of the coin toss:
-
*If Tails, player B’s earnings: 0 + 7 = 7 euros.
-
*If Heads, player B’s earnings: 10 + 7 = 17 euros.
-
-
1.7 Within-subject design: instructions for \(T_{1-2}\)
You are about to participate in an experiment to study decision making. Please carefully read the instructions, they should help you understand the experiment. All your decisions are anonymous. You will enter your choices on the computer in front of you.
The present experiment consists of three parts: “Part 1” “Part 2” and “Part 3”. The instructions for Part 1 are included hereafter. The instructions for Part 2 and Part 3 will be distributed once everybody has completed the previous part. At the end of the experiment, one of the three parts will be randomly drawn for real pay. You will then be paid in cash your earnings in euros.
During the experiment, you are not allowed to communicate. If you have questions, then please raise your hand, and an experimenter will come to you and answer your question in private.
In this experiment, there are two types of subjects (in equal number): player A and player B. You are randomly assigned a type for the entire experiment. It will be revealed privately before starting the experiment.
Part 1
At the beginning of the experiment, you are randomly paired with a player of type other than yours such that one player A is matched with one player B.
Each player, whatever his/her type, receives an endowment of 5 euros.
Additionally, players A have to share 10 euros between them, and the players B they are paired with. The players A are free to send player B any amount between 0 and 10. The only compulsory limitation is that the amounts are integers (0, 1, 2, etc. ).
Players B have an independent decision to take. They must give their opinion on the amount shared by player A of their pair. This prediction has no impact on their payment.
The earnings for each type of player are as follows:
-
Player A’s earnings: 5 euros + (10 euros - the amount sent to player B).
-
Player B’s earnings: 5 euros + the amount received from player A.
Example 1 : If player A sent 3 euros to the player B.
-
Player A’s earnings: 5 + (10 - 3) = 12 euros.
-
Player B’s earnings: 5 + 3 = 8 euros.
Example 2 : If player A sent 5 euros to the player B.
-
Player A’s earnings: 5 + (10 - 5) = 10 euros.
-
Player B’s earnings: 5 + 5 = 10 euros.
Example 3 : If player A sent 7 euros to the player B.
-
Player A’s earnings: 5 + (10 - 7) = 8 euros.
-
Player B’s earnings: 5 + 7 = 12 euros.
Part 2 (given at the end of Part 1)
Part 2 is independent of Part 1. At the beginning of this part, you are randomly paired to a player of another type than yours such that one player A is matched with one player B. The pair is different than the one you belonged to in Part 1.
Player A receives an endowment of 5 euros.
The endowment of player B is risky and depends on coin toss result. If the result of the coin toss is Tails, player B has an endowment of 0 euro. If the result is Heads, player B receives an endowment of 10 euros. The coin toss result will only be known at the end of the experiment.
Additionally, players A have to share 10 euros between them, and the players B they are paired with. The players A are free to send player B any amount between 0 and 10. The only compulsory limitation is that the amounts are integers (0, 1, 2, etc. ).
Players B have an additional independent decision to make. They must give their opinion on the amount shared by player A of their pair. This prediction has no impact on their payment.
The earnings for each type of player are as follows:
-
Player A’s earnings: 5 euros + (10 euros - the amount sent to player B).
-
Player B’s earnings depend on the result of the coin toss:
-
If Tails, player B’s earnings: 0 euros + the amount received from the player A.
-
If Heads, player B’s earnings: 10 euros + the amount received from the player A.
-
Example 1: If player A sent 3 euros to the player B:
-
Player A’s earnings: 5 + (10 - 3) = 12 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 3 = 3 euros.
-
If Heads, player B’s earnings: 10 + 3 = 13 euros.
-
Example 2: If player A sent 5 euros to player B:
-
Player A’s earnings: 5 + (10 - 5) = 10 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 5 = 5 euros.
-
If Heads, player B’s earnings: 10 + 5 = 15 euros.
-
Example 3: If player A sent 7 euros to player B:
-
Player A’s earnings: 5 + (10 - 7) = 8 euros.
-
Player B’s earnings:
-
If Tails, player B’s earnings: 0 + 7 = 7 euros.
-
If Heads, player B’s earnings: 10 + 7 = 17 euros.
-
Part 3 (given at the end of Part 2)
Part 3 is independent of parts 1 and 2.
In this part of the experiment, you receive an endowment of 10 euros. You must decide which part of this amount you wish to invest in a risky option. You can choose any amount (an integer only) between 0 and 10.
The risky option consists of a coin toss. The risky option will bring you 3 times the invested amount if heads are drawn and 0 if tails are drawn.
Your final earnings are equal to the amount kept + the earnings of your investment. Examples:
-
1.
If you decide to invest 5 euros, your earnings will be 20 euros if Heads is drawn (5 euros + 3*5 euros invested in the risky option) and 5 euros if tails are drawn (5 euros + 0*5 euros from your investment in the risky option).
-
2.
If you decide to invest 0 euro, your final earnings will be 10 euros whatever the result of the coin toss.
-
3.
If you decide to invest 10 euros. Your final earnings will be 30 euros if Heads is drawn (0 euro + 3*10 euros invested in the risky option) and 0 euro if Tails is drawn (0 euro kept + 0*10 euros from your investment in the risky option).
To avoid calculations, the table below displays the earnings according to the amount invested in the risky option and the the coin toss result.
Investment | Earnings | |
|---|---|---|
If Heads | If Tails | |
0 | 10 | 10 |
1 | 12 | 9 |
2 | 14 | 8 |
3 | 16 | 7 |
4 | 18 | 6 |
5 | 20 | 5 |
6 | 22 | 4 |
7 | 24 | 3 |
8 | 26 | 2 |
9 | 28 | 1 |
10 | 30 | 0 |
1.8 Within-subject design: instructions for \(T_{2-1}\)
The instructions are the same as for \(T_{1-2}\) except for the order of Part 1 and Part 2.
Rights and permissions
About this article
Cite this article
Beaud, M., Lefebvre, M. & Rosaz, J. Other-regarding preferences and giving decision in a risky environment: experimental evidence. Rev Econ Design 27, 359–385 (2023). https://doi.org/10.1007/s10058-022-00296-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10058-022-00296-5