Abstract
For decades, ecosystem scientists have used global warming potentials (GWPs) to compare the radiative forcing of various greenhouse gases to determine if ecosystems have a net warming or cooling effect on climate. On a conceptual basis, the continued use of GWPs by the ecological community may be untenable because the use of GWPs requires the implicit assumption that greenhouse gas emissions occur as a single pulse; this assumption is rarely justified in ecosystem studies. We present two alternate metrics—the sustained-flux global warming potential (SGWP, for gas emissions) and the sustained-flux global cooling potential (SGCP, for gas uptake)—for use when gas fluxes persist over time. The SGWP is generally larger than the GWP (by up to ~40%) for both methane and nitrous oxide emissions, creating situations where the GWP and SGWP metrics could provide opposing interpretations about the climatic role of an ecosystem. Further, there is an asymmetry in methane and nitrous oxide dynamics between persistent emission and uptake situations, producing very different values for the SGWP vs. SGCP and leading to the conclusion that ecosystems that take up these gases are very effective at reducing radiative forcing. Although the new metrics are more realistic than the GWP for ecosystem fluxes, we further argue that even these metrics may be insufficient in the context of trying to understand the lifetime climatic role of an ecosystem. A dynamic modeling approach that has the flexibility to account for temporally variable rates of greenhouse gas exchange, and is not limited by a fixed time frame, may be more informative than the SGWP, SGCP, or GWP. Ultimately, we hope this article will stimulate discussion within the ecosystem science community about the most appropriate way(s) of assessing the role of ecosystems as regulators of global climate.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Avoid common mistakes on your manuscript.
Introduction
Ecosystems are a fundamental component of the earth system that must be understood in order to model and manage atmospheric greenhouse gas concentrations and therefore global climate. Carbon dioxide (CO2) removed from the atmosphere by primary producers can be stored for decades to thousands of years in woody biomass and soil organic matter (Torn and others 1997; Gough and others 2008; Hakkenberg and others 2008). Further, ecosystems either add or remove the greenhouse gases methane (CH4) and nitrous oxide (N2O) from the atmosphere (Syakila and Kroeze 2011; Serrano-Silva and others 2014), which can either offset (gas additions) or reinforce (gas removals) the climatic benefits of carbon sequestration. Each greenhouse gas differs in its atmospheric lifetime and radiative efficiency (that is, how effectively a gas absorbs and re-radiates infrared radiation). The global warming potential (GWP) is the most commonly used metric for comparing the relative radiative forcing of gases with different lifetimes, radiative characteristics, and cycling. Using the GWP, greenhouse gas fluxes are converted to a common unit—CO2 equivalents—and then compared directly to one another to determine whether an ecosystem has a net warming or cooling effect on global climate. This approach of using GWPs to examine greenhouse gas balances has been used in the ecological community for over two decades (for example, Gorham 1991; Robertson and others 2000; Whiting and Chanton 2001; Shrestha and others 2009; Poffenbarger and others 2011; van Groenigen and others 2011; Weston and others 2014). Far less common in the ecological community is the approach of directly modeling the atmospheric dynamics of greenhouse gases (Frolking and others 2006; Frolking and Roulet 2007; Neubauer 2014).
By definition, the GWP is “the time-integrated radiative forcing due to a pulse emission of a given component, relative to a pulse emission of an equal mass of CO2” (Sect. 8.7.1.2 of Myhre and others 2013a). This definition contains several important points. Firstly, the GWP is a time-integrated index and thus its value changes depending on the time scale of interest. Because ecosystem ecologists are generally interested in the effects of a change in emissions on near-term climate change, most greenhouse gas source/sink calculations use a 100-year time frame. However, it should be noted that all time scales are arbitrary, and both shorter and longer time scales are reported in the literature. Secondly, to use GWPs as they are reported in the literature, gas emissions should be in gas mass units (for example, kg CO2), not carbon mass (kg CO2–C) or molar units (mol C). Thirdly—this is one key issue motivating this communication—the GWP is a metric for dealing with the radiative forcing due to a pulse (that is, one-time) emission of a greenhouse gas. Is a metric designed for pulse emissions appropriate for ecosystems where greenhouse gas fluxes are sustained over time? Fourthly—this is another key issue we address—the definition is directed at greenhouse gas emissions. Is the GWP metric appropriate for ecosystems that are greenhouse gas sinks?
Given the frequency with which GWPs are applied to determine whether ecosystems are net sources or sinks of greenhouse gases (that is, have a positive or negative effect on radiative forcing), we feel that it is informative to consider how the use of GWPs affects the conclusions that ecologists draw from greenhouse gas studies. We use a modeling approach to demonstrate that applying a GWP calculated from a single greenhouse gas pulse may provide misleading conclusions about the climatic role of different ecosystems because greenhouse gas fluxes from all natural ecosystems are, in reality, sustained over time. As an alternate metric, we (re)introduce the ecological community to the sustained-flux global warming potential (SGWP), a metric that has also been called the step-change global warming potential (Fuglestvedt and others 1996; Johnson and Derwent 1996; Berntsen and others 2005) and that specifically treats gas emissions as persistent—not one-time—events. Our analyses indicated a large difference in greenhouse gas dynamics depending on whether fluxes are directed into or out of the atmosphere, so we also present a new metric, the sustained-flux global cooling potential (SGCP), for situations where ecosystems remove greenhouse gases from the atmosphere. Both the SGWP and SGCP are similar to the GWP in that they are metrics for comparing the radiative forcing of different greenhouse gases, they apply over a defined time period, and they are mathematically easy to apply. However, we suggest that the SGWP and SGCP are more appropriate than the GWP when greenhouse gas fluxes are persistent and not one-time events, and that using GWPs can yield quantitatively (and sometimes qualitatively) different conclusions about the effects of an ecosystem on global radiative forcing over defined time periods. We hope that this article will stimulate discussion within the ecological community about the wide variety of greenhouse gas metrics, and lead to more appropriate ways of assessing the role of ecosystems as sources/sinks of greenhouse gases and regulators of global climate.
Methods
The atmospheric perturbation model described below follows the fate of atmospheric CO2, CH4, and N2O as these greenhouse gases are emitted from, or sequestered by, ecosystems (Figure 1). The model considers how an ecosystem changes (either by adding or removing) atmospheric greenhouse gases, but does not consider background levels of atmospheric CO2, CH4, and N2O. Further, the model assumes equal inputs and outputs to the actively cycling pools of soil organic C and CO2/CH4/N2O in soil pores (that is, these pools are in steady state). The model is based on one that was originally presented in Frolking and others (2006), as modified by Neubauer (2014) and described below. After Neubauer (2014) went to press, the Intergovernmental Panel on Climate Change (IPCC) released a new synthesis report. Consequently, we have updated the model with revised radiative efficiencies, atmospheric lifetime numbers, and indirect radiative forcing effects (Myhre and others 2013a). Additionally, we updated the CO2 portion of the model to use the impulse response parameters from Joos and others (2013) instead of an earlier parameterization.
Conceptual structure of the atmospheric perturbation model used for calculating the radiative forcing due to ecosystem C sequestration, and exchanges of CH4 and N2O between an ecosystem and the atmosphere. The atmospheric inventories of CO2, CH4, and N2O were calculated using equations (1)–(3). The soil inventories of actively cycled organic C (that is, that which is not sequestered), dissolved CO2 (not shown), dissolved CH4, and dissolved N2O were assumed to be at steady state (inputs = outputs). When CH4 or N2O fluxes are directed into the ecosystem, there is no CH4 oxidation (flux ⑥) or N2O destruction (flux ⑧), respectively.
The inventories of ecosystem-derived atmospheric CH4 and N2O (\( M_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \), g C m−2; and \( M_{{{\text{N}}_{2} {\text{O}} {-} {\text{N}}}} \), g N m−2) are calculated similarly, where the atmospheric inventory at time t is a function of the emission (or uptake) of CH4 or N2O (\( F_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \), g C m−2 y−1; and \( F_{{{\text{N}}_{2} {\text{O}} {-} {\text{N}}}} \), g N m−2 y−1), the existing inventories of ecosystem-derived CH4 and N2O (\( M_{{{\text{CH}}_{ 4} {{ {-} \text{C}, (}}t - 1)}} \), g C m−2; and \( M_{{{\text{N}}_{2} {\text{O}} {-} {\text{N, (}}t - 1)}} \), g N m−2), and the first-order removal of these gases from the atmosphere through processes such as CH4 oxidation and the photolysis of N2O with ultraviolet light:
where \( \tau_{{{\text{CH}}_{ 4} }} \) and \( \tau_{{{\text{N}}_{ 2} {\text{O}}}} \) are the atmospheric perturbation lifetimes for CH4 and N2O (12.4 and 121 years, respectively; Myhre and others 2013a), and dt is the time step of the model (0.2 years). When the \( M_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \) and/or \( M_{{{\text{N}}_{2} {\text{O}} - {\text{N}}}} \) terms are negative (that is, there has been net removal of CH4 and/or N2O from the atmosphere), the \( e^{{\left( { - dt/\tau } \right)}} \) terms in equations (1) or (2) are removed to reflect the fact that the atmospheric processes of CH4 oxidation and N2O destruction cannot affect gases that are no longer in the atmosphere. Dropping this term results in model formulations that differ for emissions and uptake.
Similar to the calculation of atmospheric inventories of ecosystem-derived CH4 and N2O, the inventory of atmospheric CO2 (\( M_{{{\text{CO}}_{2} - {\text{C}}}} \), g C m−2) depends on the rate of ecosystem CO2 flux (\( F_{{{\text{CO}}_{2} - {\text{C}}}} \), g C m−2 y−1); this flux will have a negative sign when the ecosystem is a net sink for atmospheric CO2. The net flux of CO2 between atmosphere and ecosystem will depend on the gross rate of CO2 fixation (gross primary production), the rate of autotrophic and heterotrophic CO2 emissions (ecosystem respiration), and the rate of CH4 emissions (\( F_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \)). If we make the simplifying assumption that non-atmospheric sources and sinks of ecosystem C (for example, dissolved organic and inorganic C, allochthonous carbon) are unimportant, then \( F_{{{\text{CO}}_{2} {-} {\text{C}}}} \) can be approximated as \( F_{{{\text{CO}}_{2} {-} {\text{C}}}} \) = F seq–C − \( F_{{{\text{CH}}_{ 4} {{ {-} {\text{C}} }}}} \) (g C m−2 y−1), or the balance between rates of ecosystem C sequestration (F seq–C; typically a negative number, representing CO2 removal from the atmosphere) and the C required to support CH4 fluxes (\( F_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \); a positive number for CH4 emissions, negative for CH4 uptake). Atmospheric CO2 equilibrates with various non-atmospheric reservoirs over a variety of time scales (for example, short-term exchange with the surface ocean, weathering of continental rocks over geological scales; Walker 1991; Siegenthaler and Sarmiento 1993). These feedback processes result in an exchange of CO2 between external biological, hydrological, and geological reservoirs and the atmosphere whenever CO2 is added (or removed) from the atmosphere, and thus buffer perturbations in atmospheric CO2 inventories. Mathematically, this can be modeled as though the atmosphere consists of four non-interacting reservoirs of CO2 (Joos and others 2013). Carbon dioxide can also be added to the atmosphere due to the oxidation of atmospheric CH4 emitted from the ecosystem (\( M_{{{\text{CH}_{ 4} {-} {\text{ox}}}}} \); g C m−2). Thus, the inventory of ecosystem-derived atmospheric CO2 at any point t can be calculated as follows:
where \( \tau_{{{\text{CO}}_{2} }} \) is the atmospheric perturbation lifetime for each of the four CO2 pools (4.3–394 years, with one pool that stays permanently in the atmosphere) and f i is the relative fractional size of pool i (0.217–0.282; see Joos and others 2013). \( M_{{{\text{CH}_{ 4} {-} {\text{ox}}}}} \) is the \( \left[ {M_{{{\text{CH}}_{4} - {\text{C}},(t - 1)}} \times e^{{\left( { - dt/\tau_{{{\text{CH}}_{4} }} } \right)}} } \right] \) term from equation (1); this entire term is removed when there has been a net removal of CH4 from the atmosphere (that is, \( M_{{{\text{CH}}_{ 4} {{ {-} {\text{C}}}}}} \) < 0).
The model was run for periods of 500 years. At each time step, the instantaneous radiative forcing due to each gas n was calculated by multiplying atmospheric inventories converted to kg CO2, CH4, or N2O (M n,(t) ) by the appropriate radiative efficiency (1.75 × 10−15 W m−2 (kg CO2)−1, 1.28 × 10−13 W m−2 (kg CH4)−1, or 3.83 × 10−13 W m−2 (kg N2O)−1) (Myhre and others 2013a). Following accepted practices, the radiative efficiencies for CH4 and N2O were multiplied by factors of 1.65 and 0.93, respectively, to account for the indirect effects of these gases on the global radiation balance (Myhre and others 2013b). For each gas, the time-integrated (that is, cumulative) radiative forcing over the model period was calculated as the sum of the instantaneous radiative forcing values.
The model was run as follows to track the fate of ecosystem-derived greenhouse gases under scenarios of (1) a one-time pulse emission of each gas, and (2) continuous fluxes between ecosystem and atmosphere, modeled as repeated pulses at 0.2-year intervals. Scenario 1: In separate model runs, the initial (time 0) atmospheric inventory of each greenhouse gas was set at 1 kg m−2 (representing a one-time pulse), with inventories of the other gases set at 0 kg m−2. There were no new inputs or removal by the ecosystem (that is, F n = 0 g C or N m−2 y−1). Scenario 2: The initial atmospheric inventory of each gas was set at 0 kg m−2. Individually for each gas, emission or uptake rates of CO2, CH4, or N2O were set at 1 kg CO2, CH4, or N2O m−2 y−1; the flux of one gas was sustained over the entirety of each 500-year model run and all other fluxes were set at zero. When running the model, each annual emission rate was divided into five equal increments (corresponding to the 0.2 year time step of the model). We also ran a series of model runs where 80–100% of the fluxes were concentrated during the middle three time points of each year in order to approximate higher biological activity during the growing season. The cumulative radiative forcing over a range of time frames, from 20 to 500 years, differed by trivial amounts (<0.03%) relative to the baseline runs where fluxes were constant throughout the entire year.
In each scenario, the cumulative radiative forcing of gas n was calculated over periods of 20, 100, and 500 years. For CH4, the total radiative forcing reflects contributions from ecosystem-derived atmospheric CH4, CO2 that was produced from CH4 oxidation in the atmosphere, and fluxes of CO2 between the ecosystem and atmosphere that either support CH4 emissions or result from soil CH4 oxidation (Figure 1). The cumulative radiative forcing of each gas over the time period of interest was divided by the cumulative radiative forcing of CO2 over the same period to yield GWP (Scenario 1), SGWP (Scenario 2—emission), or SGCP (Scenario 2—uptake) values. By definition, the GWP, SGWP, and SGCP for CO2 are 1.
Results and Discussion
The global warming potential (GWP) has become a profoundly important tool for quantifying the role of ecosystems in regulating climate and for informing land use policies that mitigate climate change. GWPs have motivated a great deal of contemporary research on ecosystem sources and sinks of greenhouse gases, including the plant and microbial processes that regulate emissions of CH4 and N2O, gases that are otherwise a trivial component of their respective elemental cycles (Ciais and others 2013). Adoption by the Kyoto Protocol in 1997 elevated GWPs from a useful research tool to a legal instrument, a trend that has continued through subsequent adoption by voluntary carbon markets such as the Verified Carbon Standard (Couwenberg and others 2011; VCS 2013), regulatory markets such as California’s cap and trade program (Deshpande and others 2014), and offset programs such as Reducing Emissions from Deforestation and forest Degradation (REDD; Canadell and Schulze 2014; UNFCCC 2014). This paper is motivated by the fact that GWPs are being used to translate science into economic and regulatory policy, and by our perception that GWPs may not be the best way for ecologists to communicate research findings related to greenhouse gas fluxes.
Pulse Greenhouse Gas Emissions
The Scenario 1 calculations followed the fates of individual 1 kg pulses of CO2, CH4, and N2O that were emitted into the atmosphere at the beginning of separate 500-year model runs. Because of the different radiative efficiencies of each greenhouse gases, the radiative forcing at the beginning of the simulation ranged from 1.8 fW m−2 for CO2 to 355.8 fW m−2 for N2O (Figure 2A). The equilibration of atmospheric CO2 with non-atmospheric reservoirs happens over a variety of time scales so the decay dynamics of a CO2 pulse are complex. As modeled, over 50% of the added CO2 has an effective perturbation lifetime of no more than 37 years, whereas 22% will effectively remain in the atmosphere forever. Over the course of the 500-year model simulation, the concentration and radiative forcing of CO2 decrease to 28% of their initial values. In contrast, the atmospheric removal of N2O is modeled as a first-order decay process (lifetime = 121 years), with concentrations and radiative forcing decreasing exponentially; the instantaneous radiative forcing of 5.7 fW m−2 after 500 years represents approximately 1.6% of the initial forcing from this gas. With its short atmospheric perturbation lifetime (12.4 years), the pulse of CH4 decayed rapidly such that 90% of the CH4 was removed within the first 30 years of the simulation and only 1% of the CH4 remained after 100 years. Despite the rapid atmospheric disappearance of the CH4, the radiative forcing due to the initial CH4 pulse never reached zero because the oxidation of atmospheric CH4 produces CO2 that contributes radiative forcing and is governed by the complex (and slower) CO2 equilibrium dynamics described above. After 100 years, 97% of the instantaneous radiative forcing attributable to the initial CH4 pulse was due to this CO2. Remembering that GWP is a cumulative value, nearly 8% of methane’s cumulative radiative forcing at the 100-year point (and 24% after 500 years) was attributable to CO2 that was produced by atmospheric CH4 oxidation.
(A) Instantaneous radiative forcing of CO2, CH4, and N2O following a 1 kg pulse addition of each gas at time 0 and the decay of each gas over a 500-year period. Inset shows the fraction of each gas remaining over time. The remainder of the figure shows radiative forcing due to sustained emissions (B) or sustained uptake (C) of CO2, CH4, and N2O throughout the model period. All fluxes were 1 kg m−2 y−1. In all panels, the “CH4” curves include any radiative forcing by CO2 that was produced from the oxidation of atmospheric CH4. Note the logarithmic scale on the y axes. fW = 10−15 W.
The GWP values calculated from the Scenario 1 model simulations show that GWP varies as a non-linear function of time (Figure 3), with these temporal patterns due to the differing atmospheric dynamics of each gas. Notwithstanding the complexities associated with atmospheric CH4 oxidation, CH4 decays faster than CO2 equilibrates with external reservoirs so the GWP of CH4 steadily decreases from 120 at time 0 to 87 at 20 years, 32 at 100 years, and 11 at 500 years (Table 1). Recall that the value of 120 at time 0 is the ratio of the radiative efficiencies of CH4 and CO2 after accounting for the indirect forcings associated with CH4. In contrast, the GWP for N2O increases from 203 at time 0 (the ratio of the radiative efficiencies of N2O and CO2, after indirect effects) to 260 at 20 years and a maximum of 273 at roughly 50 years before decreasing to 263 at 100 years, and 132 at 500 years (Figure 3; Table 1). These temporal dynamics reflect the rapid initial disappearance of atmospheric CO2 (relative to N2O; Figure 2A inset) followed by more rapid N2O decay (relative to CO2) during later stages.
Temporal variations in the global warming potential (GWP) and sustained-flux global warming potential (SGWP) of CH4 and N2O, calculated as the cumulative (time-integrated) radiative forcing of each gas (that is, the area under each curve in Figure 2) relative to the cumulative radiative forcing of CO2 over the same time period. The GWP values were calculated following the emission of a 1 kg pulse of each gas at the start of a model run. The SGWP values assumed continuous emissions of CH4 and N2O, thus representing the amount of CO2 (in kg CO2 m−2 y−1) that must be sequestered to offset the emissions of 1 kg m−2 y−1 of CH4 or N2O. The GWPs and SGWPs for the commonly reported 20-, 100-, and 500-year periods are summarized in Table 1. Black symbols indicate the GWP values as reported in Myhre and others (2013a), that latest IPCC report does not include 500-year GWPs.
For CH4, the modeled 20-year GWP of 87 is similar to the value of 85 reported in the 2013 IPCC report (value for “fossil CH4,” which includes CO2 from CH4 oxidation; Myhre and others 2013a). Similarly, the GWP at 100 years is also somewhat larger than that from the latest IPCC report (32 vs. 30). For N2O, there is even better agreement (within 2%) between our GWPs and those of the IPCC. Given that GWPs summarized in the IPCC reports are calculated with more advanced models than used here and have an estimated uncertainty of ±30–40% (Myhre and others 2013b), we consider that our simple atmospheric perturbation model is sufficiently describing the atmospheric dynamics of single pulses of CO2, CH4, and N2O and thus turn our attention to the behavior of the system when fluxes are sustained over time.
Sustained Ecosystem Greenhouse Gas Fluxes
The second set of scenarios involved situations where greenhouse gas fluxes (either uptake or emission to the atmosphere) were sustained over time; these scenarios produced very different time series of radiative forcing than those following a single pulse emission. When emissions are continuous from year-to-year, the atmospheric concentration and instantaneous radiative forcing of ecosystem-derived CH4 or N2O will reach steady state when the rates of atmospheric CH4 oxidation or N2O destruction are balanced by new inputs from the ecosystem. This steady state is reached after roughly four atmospheric lifetimes (~50 years for CH4 and ~480 years for N2O; Figure 2B). In contrast, the atmospheric reservoir of CO2 never reaches steady state because one of the modeled CO2 pools remains in the atmosphere over the time scales of the simulation; this is associated with the geological scale weathering of continental rocks. Thus, sustained ecosystem CO2 emissions will cause radiative forcing to steadily increase over time, with climatic effects continuing long after the impacts of CH4 or N2O emissions have reached steady state.
Many studies addressing the question of whether an ecosystem is a net source or sink of greenhouse gases have examined the balance between ecosystem CO2 sequestration (which contributes negative radiative forcing) and emissions of CH4 and/or N2O to the atmosphere (positive radiative forcing). Under these conditions, the GWPs and SGWPs for CH4 and N2O follow similar temporal trajectories but the SGWP is generally larger than the GWP; the major exception to this pattern is for N2O at time scales <70 years (Table 1; Figure 3). At the commonly used 100-year time horizon, the SGWP for CH4 is approximately 40% higher than the corresponding GWP. Thus, when fluxes are sustained over time, the emission of 1 kg CH4 m−2 y−1 would be offset by the persistent sequestration of 45 kg CO2 m−2 y−1 (Table 1). An ecosystem with fluxes in the ratio of 32 kg CO2 sequestered per 1 kg CH4 emitted would not be greenhouse gas neutral, as would be inferred if one used the 100-year GWP value calculated herein, but would actually contribute positive radiative forcing (that is, would have a net warming effect). The situation is similar for N2O, except that the 100-year SGWP for N2O emissions is only a few percent greater than the 100-year GWP (Table 1).
It should be reiterated that the SGWP derived from these sustained flux scenarios is conceptually similar to the step-change global warming potential that has been calculated for a variety of gases, including CH4, although we have not yet found a previously calculated step-change GWP for N2O (Harvey 1993; Fuglestvedt and others 1996; Johnson and Derwent 1996; Berntsen and others 2005). The SGWP values that we report (Table 1) were calculated using the latest estimates of atmospheric lifetimes, radiative efficiencies, and indirect effects of greenhouse gases on radiative forcing, so our SGWP numbers are not directly comparable to those from previous studies. Even so, our calculated GWP:SGWP ratios for emissions of CH4 (lifetime 12.4 years) and N2O (121 years) were similar to those for hypothetical gases with lifetimes of 10 and 100 years, respectively (Berntsen and others 2005). One important difference with previous work is that our calculations explicitly consider the uptake of greenhouse gases, and not emissions only. This seemingly trivial difference has important consequences for the application of greenhouse gas metrics to ecosystems.
Greenhouse gas metrics like GWP typically deal with emissions of gases to the atmosphere because these metrics were developed in the context of understanding, managing, and limiting the climatic effects of anthropogenic greenhouse gas production (Fuglestvedt and others 2003). However, ecosystems can either emit greenhouse gases or remove them from the atmosphere. Because the atmospheric dynamics of CO2 are controlled by first-order equilibration with non-atmospheric reservoirs and not by the chemical destruction of CO2 (compare with chemical loss mechanisms for CH4 and N2O), the sustained removal (sequestration) of atmospheric CO2 produces a time course of radiative forcing that is equal in magnitude, but opposite in sign, to that produced by ecosystem CO2 emissions (compare Figure 2B and C). As noted above, scenarios with CH4 or N2O emissions to the atmosphere will approach steady state (Figure 2B) as emissions of these gases are counterbalanced by chemical destruction in the atmosphere. In contrast to the atmosphere where concentrations are the balance of two competing processes, the ecosystem uptake of atmospheric CH4 and N2O produces a linear decrease in radiative forcing from these gases (Figure 2C). Applying an emissions model to uptake fluxes would mathematically imply that removal from the atmosphere would trigger new inputs to the atmosphere, but there are no important processes that add these gases to the atmosphere from other reservoirs once the gases have been taken up by an ecosystem. Thus, atmospheric CH4 and N2O exhibit very different trajectories over time depending on whether the gas fluxes are directed into or out of the ecosystem. This asymmetrical behavior has implications because it means that greenhouse gas uptake has a larger impact than emissions on net radiative forcing. These profound differences in greenhouse gas behavior as a function of flux direction lead to our suggestion that it would be more appropriate to use different metrics when fluxes are directed into vs. out of ecosystems (the SGCP and SGWP, respectively) than to use a single metric for all fluxes.
The net uptake of CH4 and/or N2O is certainly not a universal characteristic of ecosystems, but sites that can remove these gases from the atmosphere would be very effective at reducing radiative forcing. A synthesis of carbon fluxes in North American wetlands reported a net annual CH4 uptake for only 5 of 113 sites (Bridgham and others 2006). In contrast, upland ecosystems including forests, agricultural lands, and grasslands are considered global CH4 sinks (Le Mer and Roger 2001; Dutaur and Verchot 2007). On a global basis, terrestrial and aquatic ecosystems are net sources of N2O, with higher N2O emissions in systems that have higher availability of reactive nitrogen (Ciais and others 2013). However, a number of studies have measured N2O uptake in ecosystems ranging from forests to pastures, with N2O uptake being most common in soils with a high water content and low inorganic N availability (Chapuis-Lardy and others 2007). A recent synthesis of wetland N2O fluxes supports these findings, showing net N2O uptake primarily in low-nutrient freshwater and coastal wetlands, and high emissions in wetlands exposed to high nitrogen loading (Moseman-Valtierra 2012). The removal of 1 kg CH4 m−2 y−1 or 1 kg N2O m−2 y−1, sustained over a 100-year period, would be equivalent to removing 203 or 349 kg CO2 m−2 y−1, respectively (Table 1); these SGCPs are 350 and 30% greater (for CH4 and N2O, respectively) than the SGWPs for the same time frame. Because of differences in the trajectories of gases over time between emissions and uptake (Figure 2B vs. C), there is a greater difference between the SGWP and SGCP at longer time frames (Table 1).
The SGWP and SGCP values, like GWPs, are additive when multiple greenhouse gases are considered. For example, over a 100-year time period, the radiative forcing due to the continuous emission of 1 kg CH4 m−2 y−1 and 1 kg N2O m−2 y−1 would be offset if CO2 sequestration rates were 315 kg CO2 m−2 y−1 (\( = {\text{SGWP}}_{{{\text{CH}}_{ 4} , 100 {\text{y}}}} + {\text{SGWP}}_{{{\text{N}}_{ 2} {\text{O}}, 100 {\text{y}}}} \); Table 1). Any reader who wishes to apply these SGWP and SGCP values to determine whether a site is a source or sink of greenhouse gases should be aware that these ratios are based on rates of ecosystem CO2 sequestration, not net rates of CO2 uptake. This decision was based on practicality, as our impression is that more ecosystem scientists measure C sequestration (for example, by quantifying wood accumulation and/or using 137Cs and 210Pb radiodating techniques in accreting soils) than develop robust annual estimates of net ecosystem CO2 exchange (for example, by using flux chambers or eddy covariance methods).
Implications of Using GWP versus SGWP and SGCP
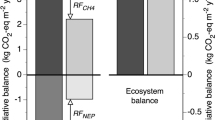
The SGWP and SGCP are alternates to the commonly used GWP that can provide a more robust estimate of the greenhouse gas source/sink status of ecosystems over a defined time period (for example, 100 years) because the calculations are based on greenhouse gas fluxes that are sustained over the entire period of interest. In contrast, the use of GWPs requires the implicit assumption that these fluxes occur as a single pulse and are not sustained over time. For ecosystems, this assumption is rarely, if ever, justified. An important question then is as follows: How does the use of GWPs instead of SGWPs or SGCPs affect interpretations of whether an ecosystem is a net source or sink of greenhouse gases (that is, radiative balance), or how a management activity changes the radiative balance of an ecosystem (that is, radiative forcing)? At the commonly used 100-year time horizon, using the GWP instead of the SGWP when considering CH4 and N2O emissions would cause one to quantitatively overestimate an ecosystem’s role as a greenhouse gas sink or, conversely, underestimate its role as a greenhouse gas source (Figure 3). If the ecosystem ratio of CO2 sequestration to CH4/N2O emission rates was greater than both GWP and SGWP, the qualitative conclusion that a site is a net greenhouse gas sink would not change regardless of whether GWP or SGWP were used (Figure 4). However, the quantitative assessment of the strength of the site’s source or sink status would differ as a function of the metric used. Similarly, if the ratio was less than both the GWP and SGWP, the qualitative conclusion that the site is a net greenhouse gas source would not change. A problem exists, however, if the ecosystem sequestration:emission ratio lies between the GWP and SGWP. In this case, one metric would indicate that the site or activity had a net cooling effect on the climate, whereas the other would indicate a net warming effect over the same time period. The potential for conflicting conclusions from the two metrics changes with time horizon; for the three time horizons shown in Table 1, the proportional difference between GWP and SGWP is greatest at 100 years for CH4 and at 500 years for N2O. The same issue applies to comparisons of GWP with other alternative metrics, illustrating that the choice of a metric has the potential to influence conclusions about how changes in ecosystem processes will affect climate.
Interpretation of an ecosystem’s status as a source or sink of greenhouse gases can depend on whether one uses the GWP or SGWP. When a site’s ratio of CO2 sequestration to CH4 or N2O emissions lies between the GWP and SGWP, these indices provide conflicting information about the greenhouse gas status of the site. Conversely, when the ratio of sequestration to emissions is either greater than both indices (greenhouse gas sink) or less than both (greenhouse gas source) one would reach the same qualitative determination about the source/sink status of a particular site regardless of which index was used, but the GWP and SGWP would give different quantitative assessments of the site’s strength as a source or sink. GHG greenhouse gas.
Using two separate global data compilations, we illustrate how the choice of a greenhouse gas metric can affect the calculated greenhouse gas source/sink status of an ecosystem, with potential management implications. First, we consider the global exchanges of CH4 and N2O between upland and wetland ecosystems and the atmosphere (Table 2; Ciais and others 2013; GCP 2013). Collectively, the fluxes of CH4 and N2O from upland and wetland systems contribute 10.2–14.7 Pg CO2-equivalents y−1 to the atmosphere, with the range reflecting use of the GWP, SGWP, and SGCP gas metrics that have been discussed in this article (there is considerable additional variability due to scientific uncertainty in the flux estimates themselves). The effect of the different greenhouse gas metrics is most apparent when examining upland soils, where the uptake of CH4 has the same cooling effect as the sequestration of 0.9–5.7 Pg CO2 y−1 (calculated using the 100-year GWPs and SGCPs, respectively). This six-fold difference also reflects the asymmetry in greenhouse gas behavior for uptake and emissions (Figure 2B vs. 2C; Table 1), and indicates how the estimate of the net cooling effect of upland systems would be significantly affected by the choice of metric. Note that applying the SGWP values for both emissions and uptake (the ‘SGWP’ column of Table 2) produces a flux value that is more similar to that calculated using GWPs and consequently underestimates the significant cooling that can result from greenhouse gas uptake by ecosystems. The fact that greenhouse gas metrics are markedly different depending on the direction of gas transport (that is, emissions versus uptake) has not been previously recognized in the calculation or criticism of existing greenhouse gas metrics. For ecosystems that emit CH4 and N2O to the atmosphere, the CO2-equivalent fluxes vary by factors of 1.41 for CH4 and 1.03 for N2O (Table 2), which are the ratios of the 100-year SGWPs:GWPs for these gases (Table 1). In some cases, the effect of using different greenhouse gas metrics can be greater than the scientific uncertainty in the flux estimates themselves. For example, estimates of CH4 emissions from rice paddies range from 33 to 40 Tg CH4 y−1 (Table 2; GCP 2013). These emissions are equivalent to 1.5–1.8 Pg CO2-eq y−1 (calculated using 100-year SGWP) or 1.1–1.3 Pg CO2-eq y−1 (using 100-year GWP); note that these ranges do not overlap. When considering total fluxes to/from upland and wetland ecosystems, there is a difference of 4.4 Pg CO2-eq y−1 between the low and high best estimates of the ecosystem CH4 and N2O fluxes (Table 2). For context, this is larger than the annual emissions of CO2 due to land use change (4.0 Pg CO2 y−1; Ciais and others 2013), further illustrating how the choice of a metric can have significant effects on how one interprets the importance of ecosystems as contributors to global greenhouse gas budgets.
As a second example, we consider tidal marshes where there is a general trend of decreasing CH4 emissions as one moves from freshwater toward saline (oceanic) systems (Bartlett and others 1987; Poffenbarger and others 2011). Using the GWP to compare CH4 emission rates with soil carbon sequestration rates, Poffenbarger and others (2011) estimated that there was at least a 95% chance that tidal marshes at salinities of at least 18 sequester enough carbon to offset CH4 emissions. More precisely, above a salinity of 18, the CO2-equivalent CH4 emissions of the 5% of marshes with the highest CH4 emissions were less than the CO2-equivalent sequestration in the 5% of marshes with the lowest soil carbon accumulation rates, making 18 a conservative delineation. Using the SGWP to make the same comparison, a marsh would require a higher carbon sequestration rate in order for the system to be conservatively climate neutral over a 100-year period; at a salinity of about 18, the probability that C sequestration would offset CH4 emissions drops from 95 to 85%. To keep a 95% level of certainty, the salinity threshold would need to increase by roughly 4–5 salinity units. Thus, although both the GWP and SGWP indicate that the cooling effect of carbon sequestration in high-salinity tidal salt marshes exceeds the warming effects from CH4 emissions, using GWP instead of SGWP to make this comparison adds a degree of uncertainty to the design and implementation of wetland creation, restoration, and protection activities (for example, “blue carbon” projects) that can affect project viability or the value of carbon credits issued to the project.
Alternative Metrics and Other Approaches
One of our main goals in this article is to begin a discussion about whether the use of GWPs as practiced by the ecological community is appropriate for determining whether natural or management-driven changes in greenhouse gas sources or sinks have a net warming or cooling effect on climate. Although we suggest that the SGWP and SGCP are more appropriate than the GWP, it is not clear that any single metric is appropriate to apply across the full spectrum of ecological research because different study goals may require different metrics. An advantage of the GWP, SGWP, and SGCP is that they are relatively straightforward to apply; one only needs to know rates of greenhouse gas uptake/emission to assess the relative radiative forcing due to each gas. This simplicity is one reason why the GWP has been widely adopted (Myhre and others 2013a). However, there are fundamental questions and issues associated with the usage and interpretation of GWPs; this topic has been covered extensively in the climate literature (Harvey 1993; O’Neill 2000; Smith and Wigley 2000; Fuglestvedt and others 2003; Shine 2009; Pierrehumbert 2014). At the risk of being redundant, we briefly highlight some of the issues and note that these concerns are generally applicable to the SGWP and SGCP metrics as well. Although ecosystem scientists and policy makers generally apply GWPs over a 100-year time frame, it is not clear what the correct time frame should be, or even that there is a “correct” time frame (Rodhe 1990; Shine and others 1990). Implicitly, neither the GWP nor SGWP considers any gas that remains in the atmosphere after the time period of interest (O’Neill 2000), which can result in policies that favor abatement of short-lived gases while delaying mitigation of longer-lived gases whose climatic effects persist beyond the time frame of the greenhouse gas metric (Pierrehumbert 2014). Because these metrics were calculated over a defined time period, they cannot deal with temporally variable fluxes (Frolking and others 2006). Finally, these metrics have “warming” in their name (or “cooling” in the case of the SGCP) but deal only with cumulative radiative forcing and not with temperature change (Fuglestvedt and others 2003).
Despite the many shortcomings, the GWP has been widely adopted as a policy, regulatory, management, and research tool because it captures a key driver of climate (radiative forcing) with minimal uncertainty. The global temperature change potential (GTP) is conceptually more useful as a metric of ecosystem-climate feedbacks because it computes global temperature as the product of radiative forcing and a climate sensitivity parameter (Shine and others 2005). However, for a given change in greenhouse gas emissions, there is far more certainty about the processes that govern radiative forcing than the processes that govern climate sensitivity. Thus, GTP calculations have the potential to produce a wider range of outcomes than GWP depending on model structure and assumptions, making GTP more value-laden, less certain, and perhaps less appealing as an accounting and policy tool. A number of other metrics such as the climate change impact potential (Kirschbaum 2014), cost-effective temperature potential (Johansson 2012), global cost potential (Manne and Richels 2001), and global damage potential (Kandlikar 1995) go well beyond GTP by modeling the impacts of greenhouse gas emissions on environmental, economic, and social outcomes, all of which are of immediate importance to policy makers. The uncertainty of measurements and metrics increases as one moves from considering emissions (reasonably well constrained) to quantifying radiative forcing (minimal uncertainty) to calculating temperature change (greater uncertainty) to forecasting actual impacts on social and economic systems (highest uncertainty) (Fuglestvedt and others 2003; Prather and others 2009). In other words, the level of uncertainty increases as the metric encompasses processes with increasing relevance to ecological, social, or economic outcomes. To date, ecosystem ecologists have not debated the balance between certainty, relevance, and time frame that is most appropriate for advancing science as opposed to policy.
The effect any ecosystem has on global radiative forcing (and by extension, climate) will be the balance between cooling due to long-term C sequestration plus any CH4 or N2O uptake that has taken place over the lifetime of the site, and the warming due to greenhouse gas emissions over recent decades (for CH4) to centuries (for N2O). Because of varying time frames over which ecosystems affect climate, using a defined period metric such as SGWP, GWP, GTP, or one of the others mentioned above can yield misleading conclusions about the long-term climatic role of ecosystems. Indeed, a site that has a warming effect on climate over a defined 100 (or 20, or 500)-year period may actually have a net cooling effect when greenhouse gas uptake and emission are integrated over the lifetime of the system (Frolking and Roulet 2007). Depending on the ratio of greenhouse gas uptake to emissions, it can take decades to thousands of years before cumulative radiative forcing from a site switches from positive (net warming) to negative (net cooling), a point known as the radiative forcing switchover time (Frolking and others 2006; Neubauer 2014). When the ratio of CO2 sequestration to either CH4 or N2O emissions is the same as the SGWP, then the switchover time will be the time frame over which the SGWP was calculated. For example, an ecosystem that sequesters 45 kg CO2 per kg CH4 emitted (that is, a ratio equivalent to the 100-year SGWP for CH4, Table 1) has a cumulative radiative forcing switchover time of 100 years.
Unlike metrics such as the GWP, SGWP, or SGCP, the modeling of switchover times does not require one to use a fixed time frame (for example, 20 or 100 years). Using this approach on the previously discussed Poffenbarger and others (2011) CH4 emission dataset (assuming a conservative carbon accumulation rate of 44 g C m−2 y−1, which is the 5th percentile of the Chmura and others 2003 dataset), we calculate that 9 of 10 marshes with salinity at least 18 had an immediate and a persistent net cooling effect (switchover time = 0 years), whereas one site had a switchover time of 195 years. In contrast, the switchover times for tidal freshwater and brackish marshes (salinity <18) were considerably higher (median = 663 years; 5th–95th percentile range = 26–17,000 years). Modeling switchover times also offers flexibility, as it is not necessary to assume a constant flux over time (an implicit assumption behind SGWP and SGCP values). Instead, one could, for example, take into account temporal changes in carbon sequestration as a forest matures (Gough and others 2008) or in methanogenesis as a created wetland develops (Liikanen and others 2006; Cornell and others 2007). This type of modeling approach, therefore, has high potential to provide valuable scientific insights into the climatic role of ecosystems and is an example of a new approach that the ecological community could adopt independently of the needs of policy makers.
Concluding Remarks
Although the GWP has been in use for decades, the climate community is still developing new metrics (for example, Kirschbaum 2014) and discussing which metrics are most appropriate for addressing and mitigating the various environmental, social, and economic impacts of climate change (Myhre and others 2013a). There is no single “perfect” metric. However, the analyses and arguments presented herein indicate that the use of GWPs for assessing the climatic impacts of ecosystems is fundamentally untenable. We suggest that use of the SGWP and SGCP is a more appropriate way of understanding the greenhouse gas source/sink status of ecosystems, but conclude that these metrics share many of the shortcomings of the GWP. We propose that the ecological research community should adopt a dynamic modeling approach that provides insights into the lifetime climatic role of an ecosystem (for example, by calculating switchover times). From a research perspective, we question whether the ecosystem science community should be using metrics that were developed for policy applications when other approaches (modeling) are likely to provide more relevant scientific insights. Ultimately, we hope that this article will motivate the ecological community to consider whether GWPs are being used correctly and to begin a larger discussion about the most appropriate way(s) of assessing and quantifying the role of ecosystems as regulators of global climate.
Change history
24 July 2019
In our 2015 paper, we dropped the e(������dt/��) term from Equations (1) and (2) when an ecosystem removed CH4 or N2O from the atmosphere, reasoning that greenhouse gases that are not in the atmosphere cannot undergo atmospheric oxidation or photolysis. The flaw in this logic is that the uptake of greenhouse gases by an ecosystem has the effect of reducing the pool of bulk atmospheric greenhouse gases and, consequently, lowering the decay rate of those gases. This violates our implicit assumption that the pool of bulk atmospheric greenhouse gases is in steady state and not affected by the ecosystem. To account for this, the e(������dt/��) term needs to remain in the model, with the result that the exact same equation is used when an ecosystem exhibits sustained greenhouse gas emissions or uptake. We thank Paavo Ojanen for bringing this error to our attention.
24 July 2019
In our 2015 paper, we dropped the e(������dt/��) term from Equations (1) and (2) when an ecosystem removed CH4 or N2O from the atmosphere, reasoning that greenhouse gases that are not in the atmosphere cannot undergo atmospheric oxidation or photolysis. The flaw in this logic is that the uptake of greenhouse gases by an ecosystem has the effect of reducing the pool of bulk atmospheric greenhouse gases and, consequently, lowering the decay rate of those gases. This violates our implicit assumption that the pool of bulk atmospheric greenhouse gases is in steady state and not affected by the ecosystem. To account for this, the e(������dt/��) term needs to remain in the model, with the result that the exact same equation is used when an ecosystem exhibits sustained greenhouse gas emissions or uptake. We thank Paavo Ojanen for bringing this error to our attention.
References
Bartlett KB, Bartlett DS, Harriss RC, Sebacher DI. 1987. Methane emissions along a salt marsh salinity gradient. Biogeochemistry 4:183–202.
Berntsen T, Fuglestvedt J, Joshi M, Shine KP, Stuber N, Ponater M, Sausen R, Hauglustaine DA, Li L. 2005. Response of climate to regional emissions of ozone precursors: sensitivities and warming potentials. Tellus B 57B:283–304. doi:10.1111/j.1600-0889.2005.00152.x.
Bridgham SD, Megonigal JP, Keller JK, Bliss NB, Trettin CC. 2006. The carbon balance of North American wetlands. Wetlands 26:889–916.
Canadell JG, Schulze ED. 2014. Global potential of biospheric carbon management for climate mitigation. Nat Commun 5:5282. doi:10.1038/ncomms6282.
Chapuis-Lardy L, Wrage N, Metay A, Chotte J-L, Bernoux M. 2007. Soils, a sink for N2O? A review. Glob Change Biol 13:1–17. doi:10.1111/j.1365-2486.2006.01280.x.
Chmura GL, Anisfeld SC, Cahoon DR, Lynch JC. 2003. Global carbon sequestration in tidal, saline wetland soils. Global Biogeochemical Cycles 17:Article 1111.
Ciais P, Sabine CL, Bala G, Bopp L, Brovkin V, Canadell JG, Chhabra A, Defries R, Galloway J, Heimann M, Jones C, Le Quéré C, Myneni RB, Piao S, Thornton P. 2013. Carbon and other biogeochemical cycles. In: Stocker TF, Qin D, Plattner G-K, Tignor M, Allen SK, Boschung J, Nauels A, Xia Y, Bex V, Midgley PM, Eds. Climate change 2013: The physical science basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change. Cambridge: Cambridge University Press, p. 465–570.
Cornell JA, Craft CB, Megonigal JP. 2007. Ecosystem gas exchange across a created salt marsh chronosequence. Wetlands 27:240–50.
Couwenberg J, Thiele A, Tanneberger F, Augustin J, Bärisch S, Dubovik D, Liashchynskaya N, Michaelis D, Minke M, Skuratovich A, Joosten H. 2011. Assessing greenhouse gas emissions from peatlands using vegetation as a proxy. Hydrobiologia 674:67–89. doi:10.1007/s10750-011-0729-x.
Deshpande B, Hunsaker L, Vayssières M, Lutter K, Eslinger K, Scott K, Huang A. 2014. California’s 2000–2012 greenhouse gas emissions: Technical support document. Accessed 06 January 2015 from http://www.arb.ca.gov/cc/inventory/doc/doc.htm.
Dutaur L, Verchot LV. 2007. A global inventory of the soil CH4 sink. Global Biogeochemical Cycles 21:GB4013. doi:10.1029/2006GB002734.
Frolking S, Roulet NT. 2007. Holocene radiative forcing impact of northern peatland carbon accumulation and methane emissions. Glob Change Biol 13:1079–88. doi:10.1111/j.1365-2486.2007.01339.x.
Frolking S, Roulet N, Fuglestvedt J. 2006. How northern peatlands influence the Earth’s radiative budget: Sustained methane emission versus sustained carbon sequestration. J Geophys Res 111:G01008. doi:10.1029/2005JG000091.
Fuglestvedt JS, Isaksen ISA, Wang W-C. 1996. Estimates of indirect global warming potentials for CH4, CO and NOX. Clim Change 34:405–37. doi:10.1007/BF00139300.
Fuglestvedt JS, Berntsen TK, Godal O, Sausen R, Shine KP, Skodvin T. 2003. Metrics of climate change: Assessing radiative forcing and emission indices. Clim Change 58:267–331. doi:10.1023/A:1023905326842.
GCP (Global Carbon Project). 2013. Methane budget and trends 2013. Released 23 September 2013, accessed 21 February 2015 from http://www.globalcarbonproject.org/methanebudget.
Gorham E. 1991. Northern peatlands: Role in the carbon cycle and probable responses to climatic warming. Ecol Appl 1:182–95.
Gough CM, Vogel CS, Schmid HP, Curtis PS. 2008. Controls on annual forest carbon storage: Lessons from the past and predictions for the future. Bioscience 58:609–22. doi:10.1641/B580708.
Hakkenberg R, Churkina G, Rodeghiero M, Börner A, Steinhof A, Cescatti A. 2008. Temperature sensitivity of the turnover times of soil organic matter in forests. Ecol Appl 18:119–31.
Harvey LDD. 1993. A guide to global warming potentials (GWPs). Energy Policy 21:24–34. doi:10.1016/0301-4215(93)90205-T.
Johansson DJA. 2012. Economics- and physical-based metrics for comparing greenhouse gases. Clim Change 110:123–41. doi:10.1007/s10584-011-0072-2.
Johnson C, Derwent R. 1996. Relative radiative forcing consequences of global emissions of hydrocarbons, carbon monoxide and NOx from human activities estimated with a zonally-averaged two-dimensional model. Clim Change 34:439–62.
Joos F, Roth R, Fuglestvedt JS, Peters GP, Enting IG, von Bloh W, Brovkin V, Burke EJ, Eby M, Edwards NR, Friedrich T, Frölicher TL, Halloran PR, Holden PB, Jones C, Kleinen T, Mackenzie FT, Matsumoto K, Meinshausen M, Plattner G-K, Reisinger A, Segschneider J, Shaffer G, Steinacher M, Strassmann K, Tanaka K, Timmermann A, Weaver AJ. 2013. Carbon dioxide and climate impulse response functions for the computation of greenhouse gas metrics: A multi-model analysis. Atmos Chem Phys 13:2793–825. doi:10.5194/acp-13-2793-2013.
Kandlikar M. 1995. The relative role of trace gas emissions in greenhouse abatement policies. Energy Policy 23:879–83. doi:10.1016/0301-4215(95)00108-U.
Kirschbaum MUF. 2014. Climate-change impact potentials as an alternative to global warming potentials. Environ Res Lett 9:034014. doi:10.1088/1748-9326/9/3/034014.
Le Mer J, Roger P. 2001. Production, oxidation, emission and consumption of methane by soils: a review. Eur J Soil Biol 37:25–50.
Liikanen A, Huttunen JT, Karjalainen SM, Heikkinen K, Väisänen TS, Nykänen H, Martikainen PJ. 2006. Temporal and seasonal changes in greenhouse gas emissions from a constructed wetland purifying peat mining runoff waters. Ecol Eng 26:241–51. doi:10.1016/j.ecoleng.2005.10.005.
Manne AS, Richels RG. 2001. An alternative approach to establishing trade-offs among greenhouse gases. Nature 371:675–7. doi:10.1038/35070541.
Moseman-Valtierra S. 2012. Reconsidering the climatic role of marshes: Are they sinks or sources of greenhouse gases? In: Abreu DC, de Borbón SL, Eds. Marshes: ecology, management and conservation. Hauppauge: Nova Scientific Publishers. p 1–48.
Myhre G, Shindell D, Bréon F-M, Collins W, Fuglestvedt JS, Huang J, Koch D, Lamarque J-F, Lee D, Mendoza B, Nakajima T, Robock A, Stephens G, Takemura T, Zhang H. 2013a. Anthropogenic and natural radiative forcing. In: Stocker TF, Qin D, Plattner G-K, Tignor M, Allen SK, Boschung J, Nauels A, Xia Y, Bex V, Midgley PM, editors. Climate change 2013: The physical science basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change. Cambridge, UK: Cambridge University Press. p. 659–740.
Myhre G, Shindell D, Bréon F-M, Collins W, Fuglestvedt JS, Huang J, Koch D, Lamarque J-F, Lee D, Mendoza B, Nakajima T, Robock A, Stephens G, Takemura T, Zhang H. 2013b. Anthropogenic and natural radiative forcing, Supplementary Material. In: Stocker TF, Qin D, Plattner G-K, Tignor M, Allen SK, Boschung J, Nauels A, Xia Y, Bex V, Midgley PM, Eds. Climate change 2013: The physical science basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change. Available from www.climatechange2013.org and www.ipcc.ch. p. 8SM1–44.
Neubauer SC. 2014. On the challenges of modeling the net radiative forcing of wetlands: Reconsidering Mitsch and others (2013). Landscape Ecol 29:571–7. doi:10.1007/s10980-014-9986-1.
O’Neill BC. 2000. The jury is still out on Global Warming Potentials. Clim Change 44:427–43. doi:10.1023/A:1005582929198.
Pierrehumbert RT. 2014. Short-lived climate pollution. Annu Rev Earth Planet Sci 42:341–79. doi:10.1146/annurev-earth-060313-054843.
Poffenbarger HJ, Needelman BA, Megonigal JP. 2011. Salinity influence on methane emissions from tidal marshes. Wetlands 31:831–42. doi:10.1007/s13157-011-0197-0.
Prather MJ, Penner JE, Fuglestvedt JS, Kurosawa A, Lowe JA, Höhne N, Jain AK, Andronova N, Pinguelli L, Pires de Campos C, Raper SCB, Skeie RB, Stott PA, van Aardenne J, Wagner F. 2009. Tracking uncertainties in the causal chain from human activities to climate. Geophys Res Lett 36:L05707. doi:10.1029/2008GL036474.
Robertson GP, Paul EA, Harwood RR. 2000. Greenhouse gases in intensive agriculture: Contributions of individual gases to the radiative forcing of the atmosphere. Science 289:1922–5. doi:10.1126/science.289.5486.1922.
Rodhe H. 1990. A comparison of the contribution of various gases to the greenhouse effect. Science 248:1217–19. doi:10.1126/science.248.4960.1217.
Serrano-Silva N, Sarria-Guzmán Y, Dendooven L, Luna-Guido M. 2014. Methanogenesis and methanotrophy in soil: A review. Pedosphere 24:291–307. doi:10.1016/S1002-0160(14)60016-3.
Shine KP. 2009. The global warming potential—the need for an interdisciplinary retrial. Clim Change 96:467–72. doi:10.1007/s10584-009-9647-6.
Shine KP, Derwent RG, Wuebbles DJ, Morcrette J-J. 1990. Radiative forcing of climate. In: Houghton JT, Jenkins GJ, Ephraums JJ, Eds. Climate change: The IPCC scientific assessment. Working Group I. Cambridge, UK: Cambridge University Press. p. 41–68.
Shine KP, Fuglestvedt JS, Hailemariam K, Stuber N. 2005. Alternatives to the global warming potential for comparing climate impacts of emissions of greenhouse gases. Clim Change 68:281–302. doi:10.1007/s10584-005-1146-9.
Shrestha R, Lal R, Penrose C. 2009. Greenhouse gas emissions and global warming potential of reclaimed forest and grassland Soils. J Environ Qual 38:426–37. doi:10.2134/jeq2008.0283.
Siegenthaler U, Sarmiento J. 1993. Atmospheric carbon dioxide and the ocean. Nature 365:119–25.
Smith S, Wigley T. 2000. Global warming potentials: 2. Accuracy. Clim Change 44:459–69. doi:10.1023/A:1005537014987.
Syakila A, Kroeze C. 2011. The global nitrous oxide budget revisited. Greenhouse Gas Meas Manage 1:17–26. doi:10.3763/ghgmm.2010.0007.
Torn MS, Trumbore SE, Chadwick OA, Vitousek PM, Hendricks DM. 1997. Mineral control of soil organic carbon storage and turnover. Nature 389:170–3. doi:10.1038/38260.
UNFCCC (United Nations Framework Convention on Climate Change). 2014. Report of the Conference of the Parties on its nineteenth session, held in Warsaw from 11 to 23 November 2013. Decision 14/CP.19 Modalities for measuring, reporting, and verifying. Accessed 06 January 2015 from http://unfccc.int/redd
van Groenigen KJ, Osenberg CW, Hungate BA. 2011. Increased soil emissions of potent greenhouse gases under increased atmospheric CO2. Nature 475:214–16. doi:10.1038/nature10176.
VCS (Verified Carbon Standard). 2013. VCS standard: Requirements document, v3.4. Accessed 06 January 2015 from http://www.v-c-s.org/program-documents
Walker JCG. 1991. Biogeochemical cycles of carbon on a hierarchy of time scales. In: Oremland RS, Ed. Biogeochemistry of global change. New York: Chapman and Hall. p 3–28.
Weston NB, Neubauer SC, Velinsky DJ, Vile MA. 2014. Net ecosystem carbon exchange and the greenhouse gas balance of tidal marshes along an estuarine salinity gradient. Biogeochemistry 120:163–89. doi:10.1007/s10533-014-9989-7.
Whiting GJ, Chanton JP. 2001. Greenhouse carbon balance of wetlands: Methane emission versus carbon sequestration. Tellus B 53:521–8. doi:10.1034/j.1600-0889.2001.530501.x.
Author information
Authors and Affiliations
Corresponding author
Additional information
Author contributions
SCN designed the study, developed and ran the model, and analyzed the data. JPM provided input on data interpretation/presentation. Both SCN and JPM wrote and edited the manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Neubauer, S.C., Megonigal, J.P. Moving Beyond Global Warming Potentials to Quantify the Climatic Role of Ecosystems. Ecosystems 18, 1000–1013 (2015). https://doi.org/10.1007/s10021-015-9879-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10021-015-9879-4