Skip to main content
SpringerLink
Log in

Effects of excitation angle and coupled heave–surge–sway motion on fluid sloshing in a three-dimensional tank

  • Original Article
  • Published:
Journal of Marine Science and Technology Aims and scope Submit manuscript

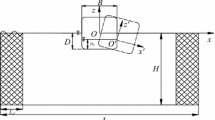
Abstract

Sloshing waves in moving tanks is an important engineering problem, and most studies of this phenomenon have focused on tanks that are excited by forcing motion in a limited number of directions and with fixed excitation frequencies throughout the forcing. In practice, the excitation comprises multiple degree of freedom motion that potentially couples surge, sway, heave, pitch, roll, and yaw motions. In the present study, a time-independent finite difference method is used to simulate fluid sloshing in three-dimensional tanks filled to an arbitrary depth for various excitation frequencies and multiple degree of freedom motion. The numerical scheme developed here was verified by rigorous benchmark tests. The coupled motions of surge and sway are simulated for various excitation angles, frequencies and water depths. Five kinds of sloshing waves found under coupled surge–sway motions: diagonal, single-directional, square-like, swirling, and irregular waves. The effect of excitation angle on the frequency responses of different sloshing waves is analyzed and discussed in the present study. Further, the components of horizontal force of various sloshing waves are also presented. The coupled effect of surge, sway and heave motions is also discussed, and the results show that unstable sloshing occurs when the excitation frequency of the heave motion is twice the fundamental natural frequency. Moreover, the effects of heave motion on the different types of sloshing waves are explored. It is found that heave motion causes all of the sloshing waves to change type.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30
Fig. 31
Fig. 32
Fig. 33
Fig. 34
Fig. 35
Fig. 36
Fig. 37
Fig. 38
Fig. 39

Similar content being viewed by others

References

  1. Abramson HN (1966) The dynamics of liquids in moving containers (NASA report SP 106). GPO, Washington, DC

  2. Nakayama T, Washizu K (1980) Nonlinear analysis of liquid motion in a container subjected to forced pitching oscillation. Int J Numer Methods Eng 15:1207–1220

    Article  MathSciNet  MATH  Google Scholar 

  3. Ockendon JR, Ockendon H, Waterhouse DD (1996) Multi-mode resonances in fluids. J Fluid Mech 315:317–344

    Article  MathSciNet  MATH  Google Scholar 

  4. Faltinsen OM (1974) A nonlinear theory of sloshing in rectangular tanks. J Ship Res 18:224–241

    Google Scholar 

  5. Chen BF, Chiang SW (1999) Complete 2D and fully nonlinear analysis of ideal fluid in tank. J Eng Mech 125(1):70–78

    Article  Google Scholar 

  6. Aliabadi S, Johnson A, Abedi J (2003) Comparison of finite element and pendulum models for simulation of sloshing. Comput Fluids 23:535–545

    Article  Google Scholar 

  7. Frandsen JB (2004) Sloshing motions in excited tanks. J Comp Phys 196:53–87

    Article  MATH  Google Scholar 

  8. Chen BF, Nokes R (2005) Time-independent finite difference analysis of fully non-linear and viscous fluid sloshing in a rectangular tank. J Comp Phys 209:47–81

    Google Scholar 

  9. Ibrahim RA, Pilipchuk VN, Ikeda T (2001) Recent advances in liquid sloshing dynamics. Appl Mech Res 54(2):133–199

    Article  Google Scholar 

  10. Cariou A, Casella G (1999) Liquid sloshing in ship tanks: a comparative study of numerical simulation. Marine Struct 12:183–189

    Article  Google Scholar 

  11. ISSC (1997) Report of Committee I.2 Loads. In: Moan T, Berge S (eds) Proceedings of 13th International Ship and Offshore Structures Congress. Pergamon–Elsevier, Oxford, 1:59–122

  12. Wu GX (2007) Second-order resonance of sloshing in a tank. Ocean Eng. 34:2345–2349

    Article  Google Scholar 

  13. Feng GC (1973) Dynamic loads due to moving liquid (AIAA paper no. 73–409). AIAA, Reston

  14. Wu GX, Ma QW, Eatock Taylor R (2008) Numerical simulation of sloshing waves in a 3D tank based on a finite element methods. Appl Ocean Res 20:337–355

    Article  Google Scholar 

  15. Akyildiz H, Ünal NE (2005) Experimental investigation of pressure distribution on a rectangular tank due to the liquid sloshing. Ocean Eng. 32:1503–1516

    Article  Google Scholar 

  16. Akyildiz H, Ünal NE (2006) Sloshing in a three-dimensional rectangular tank: numerical simulation and experimental validation. Ocean Eng 33:2135–2149

    Article  Google Scholar 

  17. Liu D, Lin P (2008) A numerical study of three-dimensional liquid sloshing in tanks. J Comp Phys 227:3921–3939

    MathSciNet  MATH  Google Scholar 

  18. Hung TK, Wang MH (1987) Nonlinear hydrodynamic pressure on rigid dam motion. J Eng Mech ASCE 113(4):482–499

    Article  Google Scholar 

  19. Hung TK, Chen BF (1990) Nonlinear hydrodynamic pressure on dams during earthquake. J Eng Mech ASCE 116(6):1372–1391

    Article  Google Scholar 

  20. Kim Y (2001) Numerical simulation of sloshing flows with impact loads. Appl Ocean Res 23:53–62

    Article  Google Scholar 

  21. Faltinsen OM, Rognebakke OF, Timokha AN (2005) Classification of three-dimensional nonlinear sloshing in a square-base tank with finite depth. J Fluids Struct 20:81–103

    Article  Google Scholar 

  22. Faltinsen OM, Rognebakke OF, Timokha AN (2005) Resonant three-dimensional nonlinear sloshing in a square-base basin. Part 2. Effect of higher modes. J Fluid Mech 523:199–218

    Google Scholar 

  23. Faltinsen OM, Rognebakke OF, Timokha AN (2006) Resonant three-dimensional nonlinear sloshing in a square-base basin. Part 3. Base ratio perturbations. J Fluid Mech 551:93–116

    Article  MathSciNet  MATH  Google Scholar 

  24. Benjamin TB, Ursell F (1954) The stability of the plane free surface of a liquid in a vertical periodic motion. Proc R Soc Lond Ser A 225:505–515

    Article  MathSciNet  MATH  Google Scholar 

  25. Wu CH, Chen BF (2009) Sloshing waves and resonant modes of fluid in a 3D tank by a time-independent finite different method. Oecan Eng 36:500–510

    Article  Google Scholar 

  26. Bredmose H, Brocchini M, Peregrine DH, Thais L (2003) Experimental investigation and numerical modeling of steep forced water waves. J Fluid Mech 490:217–249

    Article  MATH  Google Scholar 

Download references

Acknowledgments

This study is partially supported by the National Science Council under the grants NSC 96-2221-E-110-107-MY3 and NSC 98-3114-P-110-001, and is also funded by the Ministry of Education under the grant Aim for the Top University Plan. The second author also would like to thank Prof. Faltinsen for providing valued supervision during his visit to the Norwegian University of Science and Technology, Norway.

Author information

Authors and Affiliations

  1. Department of Marine Environment and Engineering, National Sun Yat-Sen University, Kaohsiung, Taiwan

    Bang-Fuh Chen & Chih-Hua Wu

Authors
  1. Bang-Fuh Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chih-Hua Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Bang-Fuh Chen.

Appendices

Appendix 1

The dimensionless momentum equations can be written as

$$ {\frac{\partial V}{\partial T}} + C_{11} C_{14} {\frac{\partial V}{\partial Y}} + C_{1} C_{13} U{\frac{\partial V}{\partial X}} + C_{2} C_{14} U{\frac{\partial V}{\partial Y}} + C_{5} C_{14} V{\frac{\partial V}{\partial Y}} + C_{8} C_{14} W{\frac{\partial V}{\partial Y}} + C_{9} C_{15} W{\frac{\partial V}{\partial Z}} = - g/g - \left( {C_{5} C_{14} {\frac{\partial P}{\partial Y}}} \right) - \ddot{Y}_{CTT} $$
(25)
$$ \begin{aligned} & {\frac{\partial W}{\partial T}} + \left( {C_{11} C_{14} {\frac{\partial W}{\partial Y}}} \right) + C_{1} C_{13} U{\frac{\partial W}{\partial X}} + C_{2} C_{14} U{\frac{\partial W}{\partial Y}} + C_{5} C_{14} V{\frac{\partial W}{\partial Y}} + C_{8} C_{14} W{\frac{\partial W}{\partial Y}} + C_{9} C_{15} W{\frac{\partial W}{\partial Z}} \\ & \quad = - \left( {C_{8} C_{14} {\frac{\partial P}{\partial Y}} + C_{9} C_{15} {\frac{\partial P}{\partial Z}}} \right) - \ddot{Z}_{CTT} . \\ \end{aligned} $$
(26)

Continuity equation:

$$ C_{1} C_{13} {\frac{\partial U}{\partial X}} + C_{2} C_{14} {\frac{\partial U}{\partial Y}} + C_{5} C_{14} {\frac{\partial V}{\partial Y}} + C_{8} C_{14} {\frac{\partial W}{\partial Y}} + C_{9} C_{15} {\frac{\partial W}{\partial Z}} = 0. $$
(27)

Kinematic free surface condition:

$$ {\frac{\partial H}{\partial T}} + UC_{1} C_{13} {\frac{\partial H}{\partial X}} + WC_{9} C_{15} {\frac{\partial H}{\partial Z}} = V. $$
(28)

Poisson equation:

$$ \begin{aligned} & {(C_{1}^{2} C_{13}^{2} ){\frac{{\partial^{2} P}}{{\partial X^{2} }}} + \left( {C_{2}^{2} C_{13}^{2} + C_{5}^{2} C_{14}^{2} + C_{8}^{2} C_{15}^{2} } \right){\frac{{\partial^{2} P}}{{\partial Y^{2} }}}} \\ & \quad + (C_{9}^{2} C_{15}^{2} ){\frac{{\partial^{2} P}}{{\partial Z^{2} }}} + 2(C_{1} C_{2} C_{13} C_{14} ){\frac{{\partial^{2} P}}{\partial X\partial Y}} + 2(C_{8} C_{9} C_{14} C_{15} ){\frac{{\partial^{2} P}}{\partial Y\partial Z}} = - \Upomega \\ \end{aligned} $$
(29)

where

$$ \begin{aligned} \Upomega & = \left [ U(C_{1}^{2} C_{13}^{2} U_{XX} + C_{2}^{2} C_{14}^{2} U_{YY} + 2C_{1} C_{2} C_{13} C_{14} U_{XY} \right. \\ & \quad + C_{2} C_{5} C_{14}^{2} V_{YY} + C_{1} C_{5} C_{13} C_{14} V_{XY} + C_{2} C_{8} C_{14}^{2} W_{YY} + C_{1} C_{8} C_{13} C_{14} W_{XY} \\ & \quad + C_{1} C_{9} C_{13} C_{15} W_{XZ} + C_{2} C_{9} C_{14} C_{15} W_{YZ} ) + V(C_{2} C_{5} C_{14}^{2} U_{YY} U_{ZZ} + C_{1} C_{5} C_{13} C_{14} U_{XY} \\ & \quad + C_{5}^{2} C_{14}^{2} V_{YY} + C_{5} C_{8} C_{14}^{2} W_{YY} + C_{5} C_{9} C_{14} C_{15} W_{YZ} ) + W(C_{2} C_{8} C_{14}^{2} U_{YY} + C_{1} C_{8} C_{13} C_{14} U_{XY} + C_{1} C_{9} \\ & \quad C_{13} C_{15} U_{XZ} + C_{2} C_{9} C_{14} C_{15} U_{YZ} + C_{5} C_{8} C_{14}^{2} V_{YY} + C_{5} C_{9} C_{14} C_{15} V_{YZ} + C_{8}^{2} C_{14}^{2} W_{YY} + C_{9}^{2} C_{15}^{2} W_{ZZ} \\ & \quad + 2C_{8} C_{9} C_{14} C_{15} W_{YZ} )] + [ (C_{1} C_{13} U_{X} + C_{2} C_{14} U_{Y} )^{2} + (C_{5} C_{14} V_{Y} )^{2} \\ & \quad + (C_{8} C_{14} W_{Y} + C_{9} C_{15} W_{Z} )^{2} + 2(C_{1} C_{13} V_{X} + C_{2} C_{14} V_{Y} ) \times (C_{5} C_{14} U_{Y} ) + 2(C_{1} C_{13} W_{X} + C_{2} C_{14} W_{Y} ) \times \\ & \quad \left. (C_{8} C_{14} U_{Y} + C_{9} C_{15} U_{Z} ) + 2(C_{5} C_{14} W_{Y} ) \times (C_{8} C_{14} V_{Y} + C_{9} C_{15} V_{Z} ) \right ] . \\ \end{aligned} $$
(30)

The finite-difference expressions of \( \wp_{i,j,k} ,\Re_{i,j,k} ,\;\aleph_{i,j,k} \) and \( \Im_{i,j,k} \) in Eqs. 17–20 are listed as follows:

$$ \begin{aligned} \wp_{i,j,k} & = - (C_{11} C_{14} U_{Y} + C_{1} C_{13} UU_{X} + C_{2} C_{14} UU_{Y} + C_{5} C_{14} VU_{Y} + C_{8} C_{14} WU_{Y} + C_{9} C_{15} WU_{z} ) - \ddot{X}_{CTT} \\ & = - \left( {C_{11} C_{14} {\frac{{U_{i,j + 1,k} - U_{i,j - 1,k} }}{2\Updelta Y}} + C_{1} C_{13} U{\frac{{U_{i + 1,j,k} - U_{i - 1,j,k} }}{2\Updelta X}} + C_{2} C_{14} U{\frac{{U_{i,j + 1,k} - U_{i,j - 1,k} }}{2\Updelta Y}}} \right. \\ & \quad \left. { + C_{5} C_{14} V{\frac{{U_{i,j + 1,k} - U_{i,j - 1,k} }}{2\Updelta Y}} + C_{8} C_{14} W{\frac{{U_{i,j + 1,k} - U_{i,j - 1,k} }}{2\Updelta Y}} + C_{9} C_{15} W{\frac{{U_{i,j,k + 1} - U_{i,j,k - 1} }}{2\Updelta Z}}} \right) - \ddot{X}_{CTT} \\ \end{aligned} $$
(31)
$$ \begin{aligned} \Re_{i,j,k} & = - \left( {C_{11} C_{14} {\frac{\partial V}{\partial Y}} + C_{1} C_{13} U{\frac{\partial V}{\partial X}} + C_{2} C_{14} U{\frac{\partial V}{\partial Y}} + C_{5} C_{14} V{\frac{\partial V}{\partial Y}} + C_{8} C_{14} W{\frac{\partial V}{\partial Y}} + C_{9} C_{15} W{\frac{\partial V}{\partial Z}}} \right) - \ddot{Y}_{CTT} \\ & = - \left( {C_{11} C_{14} {\frac{{V_{i,j + 1,k} - V_{i,j - 1,k} }}{2\Updelta Y}} + C_{1} C_{13} U{\frac{{V_{i + 1,j,k} - V_{i - 1,j,k} }}{2\Updelta X}} + C_{2} C_{14} U{\frac{{V_{i,j + 1,k} - V_{i,j - 1,k} }}{2\Updelta Y}}} \right. \\ & \quad \left. { + C_{5} C_{14} V{\frac{{V_{i,j + 1,k} - V_{i,j - 1,k} }}{2\Updelta Y}} + C_{8} C_{14} W{\frac{{V_{i,j + 1,k} - V_{i,j - 1,k} }}{2\Updelta Y}} + C_{9} C_{15} W{\frac{{V_{i,j,k + 1} - V_{i,j,k - 1} }}{2\Updelta Z}}} \right) - \ddot{Y}_{CTT} \\ \end{aligned} $$
(32)
$$ \begin{aligned} \aleph_{i,j,k} & = - \left( {C_{11} C_{14} {\frac{\partial W}{\partial Y}} + C_{1} C_{13} U{\frac{\partial W}{\partial X}} + C_{2} C_{14} U{\frac{\partial W}{\partial Y}} + C_{5} C_{14} V{\frac{\partial W}{\partial Y}} + C_{8} C_{14} W{\frac{\partial W}{\partial Y}} + C_{9} C_{15} W{\frac{\partial W}{\partial Z}}} \right) - \ddot{Z}_{CTT} \\ & = - \left( {C_{11} C_{14} {\frac{{W_{i,j + 1,k} - W_{i,j - 1,k} }}{2\Updelta Y}} + C_{1} C_{13} U{\frac{{W_{i + 1,j,k} - W_{i - 1,j,k} }}{2\Updelta X}} + C_{2} C_{14} U{\frac{{W_{i,j + 1,k} - W_{i,j - 1,k} }}{2\Updelta Y}}} \right. \\ & \quad \left. { + C_{5} C_{14} V{\frac{{W_{i,j + 1,k} - W_{i,j - 1,k} }}{2\Updelta Y}} + C_{8} C_{14} W{\frac{{W_{i,j + 1,k} - W_{i,j - 1,k} }}{2\Updelta Y}} + C_{9} C_{15} W{\frac{{W_{i,j,k + 1} - W_{i,j,k - 1} }}{2\Updelta Z}}} \right) - \ddot{Z}_{CTT} \\ \end{aligned} $$
(33)
$$ \Im_{i,j,k} = UC_{1} C_{13} {\frac{\partial H}{\partial X}} + WC_{9} C_{15} {\frac{\partial H}{\partial Z}} = UC_{1} C_{13} {\frac{{H_{i + 1,j,k} - H_{i - 1,j,k} }}{2\Updelta X}} + WC_{9} C_{15} {\frac{{H_{i,j,k + 1} - H_{i,j,k - 1} }}{2\Updelta Z}}. $$
(34)

Note that if the finite-difference expression is applied near the boundaries, the forward or backward finite-difference expression is implemented in Eqs. 31–34.

Appendix 2: The coefficients due to coordinate transformation

$$ \begin{aligned} C_{1} & = d_{0} {\frac{{\partial x^{*} }}{\partial x}} = {\frac{{d_{0} }}{{b_{2} - b_{1} }}} \\ C_{2} & = d_{0} {\frac{{\partial y^{*} }}{\partial x}} = {\frac{1}{{h^{2} }}}(y + d_{0} ){\frac{\partial h}{\partial x}} = {\frac{1}{{H^{2} }}}(y + d_{0} ){\frac{\partial H}{\partial x}} \\ C_{3} & = d_{0} {\frac{{\partial z^{*} }}{\partial x}} = 0 \\ C_{4} & = - d_{0} {\frac{{\partial x^{*} }}{\partial y}} = 0 \\ C_{5} & = - d_{0} {\frac{{\partial y^{*} }}{\partial y}} = {\frac{{d_{0} }}{h}} = \frac{1}{H} \\ C_{6} & = - d_{0} {\frac{{\partial z^{*} }}{\partial y}} = 0 \\ C_{7} & = d_{0} {\frac{{\partial x^{*} }}{\partial z}} = 0 \\ C_{8} & = d_{0} {\frac{{\partial y^{*} }}{\partial z}} = {\frac{1}{{h^{2} }}}(y + d_{0} ){\frac{\partial h}{\partial z}} = {\frac{1}{{H^{2} }}}(y + d_{0} ){\frac{\partial H}{\partial z}} \\ C_{9} & = d_{0} {\frac{{\partial z^{*} }}{\partial z}} = {\frac{{d_{0} }}{{b_{4} - b_{3} }}} \\ C_{10} & = \sqrt \frac{{d_{0} }}{g} \frac{{\partial x^{*} }}{\partial t} = 0 \\ C_{11} & = \sqrt \frac{{d_{0} }}{g} \frac{{\partial y^{*} }}{\partial t} = \sqrt \frac{{d_{0} }}{g} \frac{1}{{h^{2} }}(y + d_{0} )\frac{\partial h}{\partial t} = \sqrt \frac{{d_{0} }}{g} \frac{1}{{H^{2} }}(y + d_{0} )\frac{\partial H}{\partial t} \\ C_{12} & = \sqrt \frac{{d_{0} }}{g} \frac{{\partial z^{*} }}{\partial t} = 0 \\ C_{13} & = {\frac{\partial X}{{\partial x^{*} }}} = [1 + k_{1} (x^{*} - \lambda_{1} )(2x^{*} - 1)]{\text{e}}^{{k_{1} x^{*} (x^{*} - 1)}} \\ C_{14} & = {\frac{\partial Y}{{\partial y^{*} }}} = [1 + k_{2} (y^{*} - \lambda_{2} )(2y^{*} - 1)]{\text{e}}^{{k_{2} y^{*} (y^{*} - 1)}} \\ C_{15} & = {\frac{\partial Z}{{\partial z^{*} }}} = [1 + k_{3} (z^{*} - \lambda_{3} )(2z^{*} - 1)]{\text{e}}^{{k_{3} z^{*} (z^{*} - 1)}} \\ \end{aligned} $$

About this article

Cite this article

Chen, BF., Wu, CH. Effects of excitation angle and coupled heave–surge–sway motion on fluid sloshing in a three-dimensional tank. J Mar Sci Technol 16, 22–50 (2011). https://doi.org/10.1007/s00773-010-0111-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00773-010-0111-0

Keywords

Search

Navigation