Existence and uniqueness of price equilibria in location-based models of differentiation with full coverage

Abstract

In location-based models of price competition, traditional sufficient conditions for existence and uniqueness of an equilibrium (Caplin and Nalebuff in Econometrica 59(1):25–59) are not robust for the firm that serves the right-tail of the consumers’ distribution. Interestingly, as we relax these conditions, we observe only two new alternative cases. Moreover, we identify a novel, easily testable condition for uniqueness that is weaker than log-concavity and that can also apply to Mechanism Design. Thanks to this general framework, we can solve the equilibrium of general vertical differentiation models numerically and show that inequality has a U-shaped effect on profits and prices of a high-quality firm. Moreover, we prove that extreme levels of concentration can dissolve natural monopolies and restore competition, contrary to the Uniform case.

Proofs

Proof

of Proposition 1. First, we apply a change of variable to the profits, so that they can be both expressed in terms of the marginal consumer, instead of their respective individual prices. Then we show that the system of FOCs does not depend on qualities. Because every pure strategy equilibria must solve such system, it follows that also the number of solutions will not depend on qualities.

For $x,y \ge 0$ we define the (possibly multi-valued) functions:

$$\begin{aligned} \nu _H(x)&= \text { arg }\max _{\phi \in {\mathbb {R}}^+} \Delta s {\overline{F}}(\phi )(x+\phi ) = \text {arg}\max _{\phi \in {\mathbb {R}}^+} {\overline{F}}(\phi )(x+\phi ) \\ \nu _L(y)&= \text { arg }\max _{\phi \in {\mathbb {R}}^+} \Delta s F (\phi )(y-\phi ) = \text { arg }\max _{\phi \in {\mathbb {R}}^+} F (\phi )(y-\phi ). \end{aligned}$$

Then we can write the best response functions as:

$$\begin{aligned} \phi ^*&= \nu _H\left( \frac{p_L - MC}{\Delta s} \right) \quad \Rightarrow \quad p^*_H(p_L) = p_L + \Delta s \nu _H \left( \frac{p_L - MC}{\Delta s} \right) \\ \phi ^*&= \nu _L\left( \frac{p_H - MC}{\Delta s} \right) \quad \Rightarrow \quad p^*_L(p_H) = p_H - \Delta s \nu _L \left( \frac{p_H - MC}{\Delta s} \right) . \end{aligned}$$

Clearly, $(p^*_L, p^*_H)$ is a NE if and only if $(p^*_H- p^*_L)/\Delta s = \nu _H \left( (p^*_L - MC)/\Delta s \right) = \nu _L \left( (p^*_H - MC)/\Delta s \right)$.

If we can find a pair x, y that satisfies the system of equations

$$\begin{aligned} y - x \in \nu _H(x) \quad \text { and }\quad y - x \in \nu _L(y), \end{aligned}$$

(8)

then the relations $p^*_H = MC + \Delta s \times y$ and $p^*_L = MC + \Delta s \times x$ define a NE. Therefore, conditional on $\Delta s > 0$, the number of equilibrium points equals the number of solutions of (8), which is independent of $s_L$, $s_H$, and $\Delta s$. In particular, if (8) has no solutions, there are no equilibria for any $\Delta s \ne 0$. $\square$

Proof

of Lemma 2. First step) A finite expected value ${\mathbb {E}}[\theta ]<\infty$ implies $\lim _{b \rightarrow \infty } \int _0^b x f(x) \,dx$ $={\mathbb {E}}[\theta ]$ and $\lim _{b \rightarrow \infty }\int _b^\infty x f(x) \,dx =0$. Then:

$$\begin{aligned} \int _b^\infty x f(x) \,dx > b\int _b^\infty f(x) \,dx = b{\overline{F}}(b) \rightarrow 0 \text { as } b \rightarrow \infty . \end{aligned}$$

Therefore we have:

$$\begin{aligned} \lim _{x \rightarrow \infty } x {\overline{F}}(x) = 0. \end{aligned}$$

(9)

Moreover, a standard argument for improper integrals shows that a finite expected value forces $\lim _{x \rightarrow \infty } x f(x) = 0$. We will show a useful result:

$$\begin{aligned} \lim _{x \rightarrow 0} x f(x) = 0. \end{aligned}$$

(10)

Denote $L_0 := \lim _{x \rightarrow 0} x^2 f(x)$. We will first show that $L_0 = 0$, and leverage that fact to prove (10). Assumption 2 implies that the limit $L_0$ exists, and clearly $0 \le L_0 \le \xi ^2 f(\xi ) < \infty$. If $L_0 > 0$, then $L_0 \le x^2 f(x)$ on $x \in [0,\epsilon ]$ for some $\epsilon$. But $\infty> {\mathbb {E}}[\theta ] > \int _0^{\epsilon } x f(x) \,dx \ge \int _0^{\epsilon } L_0/x \,dx = \infty$ is a contradiction, therefore $L_0 = 0$.

To prove (10), first notice that if $z \le x \le y \le \xi$ then the Single-Peakedness implies $z^2 f(z) \le x^2 f(x) \Rightarrow z^2 f(z) / x^2 \le f(x)$. Integrating from z to y:

$$\begin{aligned} z f(z) - \frac{z^2 f(z)}{y} = z^2 f(z) \left[ \frac{1}{z} - \frac{1}{y} \right] = \int _z^y \frac{z^2 f(z)}{x^2} \,dx \le \int _z^y f(x) \,dx < F(y). \end{aligned}$$

Then $z f(z) < z^2 f(z)/y + F(y)$ for all $z \le y \le \xi$. Because F(y) decreases to 0 as y decreases to 0, for any $\epsilon > 0$ there exists a $y_\epsilon \le \xi$ such that $F(y_\epsilon ) < \epsilon /2$. Also, because $L_0 = 0$, there exists a $\delta _\epsilon > 0$ such that $z^2 f(z) < \epsilon y_\epsilon /2$ for all $0< z < \delta _\epsilon$. Therefore $z f(z)< z^2 f(z)/y_\epsilon + F(y_\epsilon ) < \epsilon$. That is, $z f(z) < \epsilon$ for all $z < \delta _\epsilon$, which proves (10).

Second step) Define the auxiliary function $m_H(x) := 1 - F(x) - x f(x)$ with derivative $m_H'(x) = - [2 f(x) + x f'(x)]$. Because $m'_H(x) \lessgtr 0$ if $x \lessgtr \xi$, then $\xi$ is the absolute minimum of $m_H$. By (9)–(10) we have that $m_H(0) = 1$ and $m_H(\infty ) = 0$. Because $m_H$ is strictly increasing over $(\xi , \infty )$, then $m_H(\xi ) < 0$. Hence, because $m_H(0)>0$, and $m_H$ is strictly decreasing in $[0,\xi ]$, it follows that it must have only one zero in $(0, \xi )$, which we label $\eta$. Notice that $m_H(x) = f(x) g_H(x)$, so we have the very useful relation (3).

Third step) Notice that $m_H(x) = m(x) + F(x) > m(x)$, and $m'(x) = - [3 f(x) + x f'(x)] = m_H'(x) - f(x) <m_H'(x)$, which imply that m is decreasing on $(0, \xi )$. Because $m(0) = 1$ and $m(\eta ) < m_H(\eta ) = 0$, m must have exactly one zero $\phi ^* \in (0, \eta )$. $\square$

Proof

of Propositions 3 and 4. In both Classes 2 and 3, there is a critical ${\overline{p}}_L$ at which the best response function $p^*_H(p_L)$ has a discontinuity. Denote by $p^1_H < p^2_H$ the two endpoints of this jump. The proof consists in finding conditions for which the best response of firm L can cross the one for firm H, avoiding the jump.

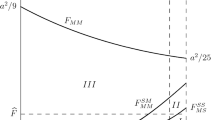
We define ${\overline{\phi }}_L := \frac{{\overline{p}}_L - MC}{\Delta s}$, $\phi ^1_H := \frac{p^1_H - MC}{\Delta s}$ and $\phi ^2_H := \frac{p^2_H - MC}{\Delta s}$, and also: $\phi ^1 := \frac{p^1_H - {\overline{p}}_L}{\Delta s} = \phi ^1_H - {\overline{\phi }}_L$ and $\phi ^2 := \frac{p^2_H - {\overline{p}}_L}{\Delta s} = \phi ^2_H - {\overline{\phi }}_L$. Then, at ${\overline{p}}_L$, firm H is indifferent between the two optimal prices $p^1_H,p^2_H$. That is, $\Pi _H(p^1_H) = \Pi _H(p^2_H)$. This is equivalent to $\phi ^1_H {\overline{F}}(\phi ^1) = (\phi ^1 + {\overline{\phi }}_L) {\overline{F}}(\phi ^1) = (\phi ^2 + {\overline{\phi }}_L) {\overline{F}}(\phi ^2) = \phi ^2_H {\overline{F}}(\phi ^2)$. The difference between the two classes is that, in Class 2, $p^1_H$ is a boundary solution in $[{\overline{p}}_L,\infty )$. That is, $\phi ^1 = 0$. On the other hand, in Class 3, both prices $p^1_H,p^2_H$ are interior. Therefore $0= \phi ^1< \eta _0< \phi ^2 < \eta$ in Class 2, and $0< \phi ^1< \eta _0< \phi ^2 < \eta$ in Class 3. Also, in Class 3 both optima satisfy the first and second order conditions: $g_H(\phi ^1) = g_H(\phi ^2) = {\overline{\phi }}_L$, $g'_H(\phi ^1)<0$ and $g'_H(\phi ^2)<0$. Plugging the FOCs into the indifference condition gives us $\big ( \phi ^1 + g_H(\phi ^1) \big ) {\overline{F}}(\phi ^1) = \big ( \phi ^2 + g_H(\phi ^2) \big ) {\overline{F}}(\phi ^2)$. With some algebraic manipulations we obtain the equations in Proposition 4.

However, in Class 2, the first and second order conditions only apply to $\phi ^2$ (in fact, for $\phi ^1 = 0$ we have that $g_H(0)<{\overline{\phi }}_L$ and $g'_H(0)>0$). The indifference condition in this class is simply ${\overline{\phi }}_L = (\phi ^2 + {\overline{\phi }}_L) {\overline{F}}(\phi ^2) = \phi ^2_H {\overline{F}}(\phi ^2)$. By the FOC $g_H(\phi ^2) = (\phi ^2 + g_H(\phi ^2)) {\overline{F}}(\phi ^2)$. After some algebra we obtain: $\phi ^2 f(\phi ^2) = F(\phi ^2) {\overline{F}}(\phi ^2)$. In Propositions 3 we rename $\phi ^2$ as ${\overline{\phi }}$.

By Theorem 1, if there exists a NE in classes 2 or 3, it must be the one described in (4). For this to be a NE, the two best response functions must cross. Therefore, there is no NE if and only if the best response of firm L passes through the gap created by the jump: $p^*_L(p^1_H)< {\overline{p}}_L < p^*_L(p^2_H)$. This is equivalent to: $\phi ^1 - g_L^{-1}({\overline{\phi }}_L + \phi ^1)< 0 < \phi ^2 - g_L^{-1}({\overline{\phi }}_L + \phi ^2)$. On the other hand, we can use the identity $g_L(x) = g_H(x) + x - (m/f)(x)$ to show that $g_L(\phi ^i) = {\overline{\phi }}_L + \phi ^i - \frac{m}{f}(\phi ^i)$ for $i=1,2$. Hence, there is no NE if and only if $m(\phi ^1)> 0 > m(\phi ^2)$. By the proof of Theorem 1, $\phi ^1< \phi ^* < \phi ^2$. For Class 2, we only need to apply the previous reasoning to $\phi ^2 = {\overline{\phi }}$. $\square$

Proof

of Theorem 3. The strategy of this proof is to transform the expected profits using a change of variable to show that the difference in qualities remains a scaling factor even when companies play mixed strategies. Then it follows that both firms will choose maximum differentiation.

First step) Denote by $\sigma _L(dp_L)$ and $\sigma _H(dp_H)$ possible mixed strategies in the second stage for each firm, that is, probability measures on ${\mathbb {R}}$, with support inside the interval $I=[MC,\infty )$. Both firms simultaneously choose $\sigma _i$ to maximize their expected profits $E_i$, for $\sigma _{-i}$ fixed:

$$\begin{aligned} E_H&= \int _{p_H \in I}\int _{p_L \in I} (p_H-MC){\overline{F}} \left( \frac{p_H-p_L}{\Delta s}\right) \,\sigma _L(dp_L)\sigma _H(dp_H) \\ E_L&= \int _{p_L \in I}\int _{p_H \in I} (p_L-MC) F \left( \frac{p_H-p_L}{\Delta s}\right) \,\sigma _H(dp_H)\sigma _L(dp_L). \end{aligned}$$

With the goal of making a simplifying a change of variable, we first rewrite these expected profits as:

$$\begin{aligned} E_H&= \Delta s \int _{p_H \in I}\int _{p_L \in I} \Phi (p_H) {\overline{F}} \big (\Phi (p_H) - \Phi (p_L) \big ) \,\sigma _L(dp_L)\sigma _H(dp_H) \\ E_L&= \Delta s \int _{p_L \in I}\int _{p_H \in I} \Phi (p_L) F \big (\Phi (p_H) - \Phi (p_L) \big ) \,\sigma _H(dp_H)\sigma _L(dp_L), \end{aligned}$$

where the function $\Phi : I \rightarrow [0,\infty )$ is defined as $\Phi (p)=(p-MC)/\Delta s$. Function $\Phi$ induces the push-forward measures $\mu _L,\mu _H$ on ${\mathbb {R}}$ defined for any Borel set $A \subset {\mathbb {R}}$ as: $\mu _H(A)=\sigma _H\left( \Phi ^{-1}(A)\right)$ and $\mu _L(A)=\sigma _L\left( \Phi ^{-1}(A)\right)$. Both are probability measures with supports in ${\mathbb {R}}^+=[0,\infty )$. Furthermore, for any function $G : {\mathbb {R}} \rightarrow {\mathbb {R}}$ we have:

$$\begin{aligned} \int _{p_H \in I} G\big ( \Phi (p_H) \big ) \,\sigma _H(dp_H)&= \int _{ y \in {\mathbb {R}}^+} G(y) \,\mu _H(dy) \\ \int _{p_L \in I} G\big ( \Phi (p_L) \big ) \,\sigma _L(dp_L)&= \int _{ x \in {\mathbb {R}}^+} G(x) \,\mu _L(dx). \end{aligned}$$

We can use the function $G(y) = \Phi (p_L) F\big (y- \Phi (p_L)\big )$, for fixed $p_L$, to rewrite the inner integral of $E_L$:

$$\begin{aligned} \int _{p_H \in I} \Phi (p_L) F\big (\Phi (p_H) - \Phi (p_L) \big ) \,\sigma _H(dp_H) = \int _{ y \in {\mathbb {R}}^+} \Phi (p_L) F\big (y - \Phi (p_L) \big ) \,\mu _H(dy). \end{aligned}$$

Next, we use the function $G(x)=\int _{y\in {\mathbb {R}}^+} x F(y-x)\,\mu _H(dy)$ to rewrite the outer integral of $E_L$:

$$\begin{aligned} E_L&= \Delta s \int _{p_L \in I} \left[ \int _{ y \in {\mathbb {R}}^+} \Phi (p_L) F\big (y - \Phi (p_L) \big ) \,\mu _H(dy) \right] \sigma _L(dp_L) \\&= \Delta s \int _{x\in {\mathbb {R}}^+}\int _{y\in {\mathbb {R}}^+} x F(y-x) \,\mu _H(dy)\mu _L(dx). \end{aligned}$$

We can follow the same procedure for $E_H$ to obtain:

$$\begin{aligned} E_H = \Delta s \int _{y\in {\mathbb {R}}^+}\int _{x\in {\mathbb {R}}^+} y {\overline{F}}(y-x) \,\mu _L(dx)\mu _H(dy). \end{aligned}$$

Because $\Phi$ is a bijection, there is a one-to-one relationship between the new measures $\mu _L,\mu _H$ and the original ones $\sigma _L,\sigma _H$, and also between any pair x, y and the corresponding prices: $p_H = MC+y\Delta s$ and $p_L = MC+x\Delta s$. In the second stage, $\Delta s$ is a given constant. Hence, solving the equilibrium problem for $E_L,E_H$ is equivalent to solving the new equilibrium problem:

$$\begin{aligned} \max _{\mu _H}&\iint y {\overline{F}}(y-x) \,\mu _L(dx)\mu _H(dy) \quad \hbox { for fixed}\ \mu _L, \\ \max _{\mu _L}&\iint x F (y-x) \,\mu _H(dy)\mu _L(dx) \quad \hbox { for fixed}\ \mu _H. \end{aligned}$$

The key observation is that this equivalent game is independent of the qualities $s_L,s_H$. By assumption, for some particular $\Delta s >0$ there exists a unique equilibrium $\sigma ^*_L,\sigma ^*_H$ for the original problem in $E_L,E_H$. This NE can be expressed in terms of $\mu ^*_L,\mu ^*_H$. Therefore, $\mu ^*_L,\mu ^*_H$ is the unique NE for the equivalent problem. From this equilibrium we can easily construct the unique equilibrium for any other pair of qualities. This argument generalizes Proposition 1 to mixed strategies.

Second step) Denote:

$$\begin{aligned} e^*_H&= \iint y {\overline{F}}(y-x) \,\mu ^*_L(dx)\mu ^*_H(dy), \\ e^*_L&= \iint x F (y-x) \,\mu ^*_H(dy)\mu ^*_L(dx). \end{aligned}$$

Then $e^*_L,e^*_H$ are constants independent of $s_L,s_H$. In the first stage both firms must solve $\max _{s_H}E^*_H(s_L,s_H) =\max _{s_H}\Delta s\times e^*_H$ for fixed $s_L$, and $\max _{s_L}E^*_L(s_L,s_H) =\max _{s_L}\Delta s\times e^*_L$ for fixed $s_H$. Therefore, the only possible equilibrium is maximum differentiation. $\square$

Proof

of Theorem 4. Denote the space of prices $(p_L,p_H)$ for which both profits are non-negative as $P = [MC,\infty )\times [MC,\infty )$. This space can be partitioned into two disjoint regions:

$$\begin{aligned} C =\left\{ (p_L,p_H) \in P: \frac{p_L}{s_L} < \frac{p_H}{s_H}\right\} \quad \text {and}\quad M =\left\{ (p_L,p_H) \in P: \frac{p_L}{s_L}\ge \frac{p_H}{s_H}\right\} . \end{aligned}$$

It is easy to see that in region C both demands are positive $D_H,D_L>0$, while in region M only the demand of firm H is positive, that is $D_H>0$ and $D_L=0$.

The proof will be divided in three steps. In a first step we show that Condition-$\eta$ implies that the best response of the firm H to any price of the firm L cannot be in region C, that is, Condition-$\eta$ is incompatible with positive market shares for firm L. However, this alone does not imply the existence of an equilibrium in region M. In a second step we will find the conditions for $p_H$ that forces the best response for firm L to be in region M. Here we will distinguish two cases. One case is when H can hold a monopoly only by setting a limit price, so as to force firm L’s price down to its marginal cost. The other case is where the exogenous parameters allow for an unconstrained monopoly price. In a third step will formally prove the existence of a monopolistic equilibrium. That is, we show that firm H can sustain an equilibrium in region M.

First step) For any fixed $p_L\ge MC$ we have:

$$\begin{aligned} \frac{\partial \Pi _H}{\partial p_H}= {\left\{ \begin{array}{ll} f\left( \frac{p_H}{s_H}\right) \left[ g_H\left( \frac{p_H}{s_H}\right) +\frac{MC}{s_H}\right] &{} \text {if }MC\le p_H\le p_L\frac{s_H}{s_L} \text { (region } M) \\ f\left( \frac{\Delta p}{\Delta s}\right) \left[ g_H\left( \frac{\Delta p}{\Delta s}\right) -\frac{p_L-MC}{\Delta s}\right] &{} \text {if }p_L\frac{s_H}{s_L}<p_H \text { (region } C). \end{array}\right. } \end{aligned}$$

In region C, $MC /s_L \le p_L / s_L< p_H /s_H < \Delta p/\Delta s$, which, together with Condition-$\eta$, implies that $\eta <\Delta p/\Delta s$, hence $g_H(\Delta p/\Delta s)<0$. This in turn implies that $\Pi _H$ is strictly decreasing in region C. Therefore, the best response $p_H^{*}(p_L)$ for any choice of $p_L$ must be in region M:

$$\begin{aligned} \frac{MC}{s_H}<\frac{p_H^{*}(p_L)}{s_H}\le \frac{p_L}{s_L}. \end{aligned}$$

Second step) Notice that, if the interval $(MC,p_Hs_L/s_H)$ is non-empty, then the best response $p_L^{*}(p_H)$ must be in region C, because $\Pi _L \le 0$ otherwise. Therefore an equilibrium in region M can exist if and only if that interval is empty, which is equivalent to:

$$\begin{aligned} \frac{p_H}{s_H} \le \frac{MC}{s_L}. \end{aligned}$$

(11)

If that is the case, then $\Pi _L(p_L,p_H)=0$ for all $p_L\ge MC$ and, therefore, the best response for firm L is the whole semi-line $p_L^{*}(p_H)=[MC,\infty )$.

On the other hand, in region M firm H faces the “constrained monopoly problem” (6) with solution $p_M = \min \{ p^*_M, p_L s_H/s_L \}$, where $p^*_M$ is the solution of the unconstrained problem. In our working paper we show that this must exist. With (11) in sight, it is useful to analyze the relationship between $p^*_M/s_H$ and $MC/s_L$. Because $g_H$ is strictly decreasing:

$$\begin{aligned} g_H^{-1}\left( -\frac{MC}{s_H}\right) =\frac{p^*_M}{s_H}\gtrless \frac{MC}{s_L}\quad \Longleftrightarrow \quad -\frac{MC}{s_H}\lessgtr g_H\left( \frac{MC}{s_L}\right) . \end{aligned}$$

Or equivalently:

$$\begin{aligned} \frac{p^*_M}{s_H}\gtrless \frac{MC}{s_L}\quad \Longleftrightarrow \quad -\frac{MC}{g_H\left( \frac{MC}{s_L}\right) }\gtrless s_H. \end{aligned}$$

Define the threshold $\sigma =\sigma (g_H,MC,s_L)=-MC/g_H(MC/s_L)$. Notice that, because $MC/s_L\ge \eta$ then $0<-g_H(MC/s_L)<MC/s_L$, which implies that $s_L<\sigma$. We have two cases:

Case i) If $s_H$ is sufficiently higher than $s_L$:

$$\begin{aligned} s_L<\sigma \le s_H\quad \Longleftrightarrow \quad \frac{p^*_M}{s_H}\le \frac{MC}{s_L}. \end{aligned}$$

Case ii) If $s_H$ is close enough to $s_L$:

$$\begin{aligned} s_L<s_H<\sigma \quad \Longleftrightarrow \quad \frac{p^*_M}{s_H}>\frac{MC}{s_L}. \end{aligned}$$

Third step) Now assume that $(p_L^{eq},p_H^{eq})$, which must be in region M by Condition-$\eta$, is an equilibrium. There are only two possible alternatives:

$$\begin{aligned} \frac{p_L^{eq}}{s_L}\ge \frac{p^*_M}{s_H}&\quad \text {and}\quad p_H^{eq}=p_H^{*}(p_L^{eq})=p^*_M,\text { or } \\ \frac{p_L^{eq}}{s_L}<\frac{p^*_M}{s_H}&\quad \text {and}\quad p_H^{eq}=p_H^{*}(p_L^{eq})=p_L^{eq}\frac{s_H}{s_L}. \end{aligned}$$

Because (11) must hold, in the first alternative we have

$$\begin{aligned} \frac{p_H^{eq}}{s_H}=\frac{p^*_M}{s_H}\le \frac{MC}{s_L}\le \frac{p_L^{eq}}{s_L}. \end{aligned}$$

This can only occur in Case i), and all the points $(p_L^{eq},p_H^{eq})=\left( p_L^{eq},p^*_M\right)$, for any $p_L^{eq}\ge MC$, are equilibria. In the second alternative we have that:

$$\begin{aligned} \frac{p_H^{eq}}{s_H}=\frac{p_L^{eq}}{s_L}\ge \frac{MC}{s_L}. \end{aligned}$$

Because (11) also holds here, then the only possibility is $p_L^{eq}=MC$. Then the point $(p_L^{eq},p_H^{eq})=(MC,MCs_H/s_L)$ is an equilibrium if and only if:

$$\begin{aligned} \frac{MC}{s_L}=\frac{p_L^{eq}}{s_L}<\frac{p^*_M}{s_H}. \end{aligned}$$

And this is exactly Case ii). $\square$