Strategic partial outsourcing in the presence of single-source components

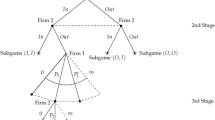
Abstract

We study the sourcing decision of a manufacturer in choosing between sole sourcing and multi-sourcing for a production component, when available sources are under different efficiency levels. In our model, the manufacturer can either produce the component in-house, or outsource to less efficient external suppliers. Without any demand uncertainty or ex-ante capacity constraint with in-house production, we find that the manufacturer may establish only limited in-house production capacity and eventually outsource to less efficient external suppliers. Such partial outsourcing is purely strategic and is due to the existence of single-source components, with which the manufacturer solely relies on key suppliers who possess great market power. Partial outsourcing enables the manufacturer to mitigate the pricing power of key suppliers, which is optimal to the manufacturer so long as the associated efficiency loss of outsourcing is not too pronounced. Moreover, cost increase in outsourcing may lead the manufacturer to outsource even more in order to boost its profitability.

Appendix

Proof of Lemma 1

First, we show that $q^{*}(w)$ and ${\bar{q}}(w)$ decrease in w. By (2), (3) and the implicit function theorem, it holds that

$$\begin{aligned} \frac{dq^{*}(w)}{dw}=\frac{d{\bar{q}}(w)}{dw}=\frac{1}{P^{\prime \prime }(q)q+2P^{\prime }(q)-C^{\prime \prime }(q)}<0. \end{aligned}$$

(11)

Second, we show that $q^{*}(w)>{\bar{q}}(w)$. By Assumption 1, $P^{\prime }(q)q+P(q)-C^{\prime }(q)$ decreases in q. By (2) and (3), $P^{\prime }( q^*(w))q^{*}(w)+P(q^{*}(w))-C^{\prime }(q^*(w))=w$, and $P^{\prime }({\bar{q}}(w)){\bar{q}}(w)+P( {\bar{q}}(w))-C^{\prime }({\bar{q}}(w))=w+m>w.$ Thus $q^{*}(w)>{\bar{q}}(w)$.

Third, note that $w_{1}(K)$ and $w_{2}(K)$ are well defined due to the monotonicity of ${\bar{q}}(w)$ and $q^{*}(w)$. By (4) and (11), it holds that $w_{1}(K)<w_{2}(K)$.

Forth, we derive firm M’s optimal quantity by discussing different cases. Since $w_{1}(K)<w_{2}(K)$, there exist three cases: (1) $w<w_{1}(K)$, (2) $w\ge w_{2}(K)$, and 3) $w\in [w_{1}(K),w_{2}(K))$. We discuss each of them below.

Case 1:

$w<w_{1}(K)$ By the definition of $w_{1}(K)$, ( 4) and (11), we have ${\bar{q}}(w)>K$ and $q^{*}(w)>K$. firm M maximizes $P(q)q-C(q)-wq-m(q-K)$ subject to $q>K$, and his equilibrium quantity is ${\bar{q}}(w)$ (given by the heavy part of ${\bar{q}}(w)$ in Fig. 1).

Case 2:

$w> w_{2}(K)$ By the definition of $w_{2}(K)$, ( 4) and (11), we have ${\bar{q}}(w)<K$ and $q^{*}(w)<K$. firm M maximizes $P(q)q-c(q)-wq$ subject to $q< K$. His equilibrium quantity is $q^{*}(w)$ (given by the heavy part of $q^{*}(w)$ in Fig. 1).

Case 3:

$w\in [w_{1}(K),w_{2}(K)]$ By the definitions of $w_{1}(K)$ and $w_{2}(K)$, (4) and (11), we have ${\bar{q}}(w)\le K\le q^{*}(w)$. The optimal quantity of firm M when he outsources component N shall never exceeds K. Thus, firm M maximizes $P(q)q-c(q)-wq$ subject to $q\le K$. The constraint is binding since $K\le q^{*}(w)$ and firm M’s equilibrium quantity is K. The lemma then follows.

$\square$

Proof of Lemma 2

First, note that ${\bar{w}}$ satisfies the first-order condition

$$\begin{aligned} {\bar{q}}(w)+(w-v){{\bar{q}}}^{\prime }(w)=0, \end{aligned}$$

(12)

and $w^{*}$ satisfies the first-order condition

$$\begin{aligned} q^{*}(w)+(w-v){q^{*}}^{\prime }(w)=0. \end{aligned}$$

(13)

Let the optimal quantity produced by firm M by q(w), suppressing $q(w)=q^{*}(w)$ or $q(w)={\bar{q}}(w)$. By (12) and (13), the second order condition of firm S’s profit maximization requires that

$$\begin{aligned} 2\frac{dq(w)}{dw}+(w-v)\frac{d^{2}q(w)}{dw^{2}}<0. \end{aligned}$$

(14)

By (11), we have

$$\begin{aligned} \frac{d^{2}q(w)}{dw^{2}}=-\frac{1}{H^{2}}[P^{\prime \prime \prime }(q)q+3P^{\prime \prime }(q)-C^{\prime \prime \prime }]\frac{dq(w)}{dw}\le 0, \end{aligned}$$

(15)

with $H\equiv P^{\prime \prime }(q)q+2P^{\prime }(q)-C^{\prime \prime }$. The above is guaranteed by Assumption 2. Thus (14) is satisfied, showing that $w^*$ and ${\bar{w}}$ exist and are unique to the profit maximization problem of firm S.

Second, we prove that $w^{*}>{\bar{w}}$. By (2) and (3), ${\bar{q}}(w)=q^{*}(w)$ at $m=0$. Therefore, ${\bar{w}}=w^{*}$ at $m=0$. To show that $w^{*}>{\bar{w}}$, it is sufficient to verify that $\frac{d{\bar{w}}}{dm}<0$ since $\frac{dw^*}{dm}=0$. Note that by the implicit function theorem, we have

$$\begin{aligned} \frac{d{\bar{q}}(w)}{dm}=\frac{1}{P^{\prime \prime }(q)+2P^{\prime }(q)-C^{\prime \prime }}<0. \end{aligned}$$

By (12) and the implicit function theorem, we have

$$\begin{aligned} \frac{d{\bar{w}}}{dm}=-\frac{\frac{d{\bar{q}}(w)}{dm}+(w-v)\frac{d^2{\bar{q}}(w)}{ dwdm}}{2\frac{d{\bar{q}}(w)}{dw}+(w-v)\frac{d^{2}{\bar{q}}(w)}{dw^{2}}}<0 \end{aligned}$$

(16)

by (14) and $\frac{d^2{\bar{q}}(w)}{dwdm}=0$ (by (11)). The continuity of ${\bar{w}}$ in m suggests that $w^{*}>{\bar{w}}$. $\square$

Proof of Lemma 3

We first establish some structural properties of $w^*$ and ${\bar{w}}$ before we proceed to prove the lemma.

• 1. Structural properties of firm S’s wholesale prices

  • (1) $(w^{*}-v)q^{*}(w^{*})>({\bar{w}}-v){\bar{q}}({\bar{w}}):$ observe that

    $$\begin{aligned} \begin{aligned} (w^*-v)q^*(w^*)&\ge ({\bar{w}}-v) q^*({\bar{w}})\qquad \text{ by } \text{ optimality } \text{ of } w^*\\&>({\bar{w}}-v){\bar{q}}({\bar{w}}) \qquad \text{ by } (4). \end{aligned} \end{aligned}$$

  • (2) ${\tilde{w}}$ exists and is unique: The slope of the iso-profit of firm S is $\frac{dq}{dw}=-\frac{q}{w-v}<0$ (since $w-v\ge 0$), which goes to zero when w goes to infinity. Since $q^*(w)$ strictly decreases in w, the iso-profit passing point A must intersect $q^*(w)$ from below, i.e., there exists ${\tilde{w}}>{\bar{w}}$ such that (6) holds. In addition, the iso-profit of firm S is convex since $\frac{d^2q}{dw^2}= \frac{q}{(w-v)^2}>0$, and $q^*(w)$ is concave since $\frac{d^2q^*(w)}{ dw^2}\le 0$ by (15). Thus the intersection of these two at which $w>{\bar{w}}$ is unique.

  • (3) ${\tilde{w}}>w^{*}:$ Suppose by negation that ${\tilde{w}}\le w^{*}$. Define $q^A(w)$ as the solution to $(w-v)q=({\bar{w}}-v){\bar{q}}( {\bar{w}})$ at given w. Thus $(w,q^A(w))$ is on the iso-profit of firm S which passes point A. Since point J is the intersection of this iso-profit and $q^*(w)$ when the iso-profit is getting flattened, it holds that for $w\ge {\tilde{w}}$, we have $q^*(w)\le q^A(w)$. Thus at $w^*$, $(w^*-v)q^A(w^*)=({\bar{w}}-v){\bar{q}}({\bar{w}})$. Since $(w^*-v)q^A(w^*)\ge (w^*-v)q^*(w^*)$, it follows that $(\bar{w }-v){\bar{q}}({\bar{w}})\ge (w^*-v)q^*(w^*)$. A contradiction to (i). We conclude that ${\tilde{w}}>w^{*}$.

• 2. Firm S’s optimal wholesale price decisions

At any given K, in Stage 2 firm S can modify w to induce firm M to produce any quantity along the kinked curve given by q(K, w). Since $q^{*}({\tilde{w}})<q^{*}(w^{*})$ by (11) and (iii), there can be three cases according to the established capacity K. We discuss each of them in what follows.

Case 1:

$K\le q^{*}({\tilde{w}})$. In this case, $w_{2}(K)\ge {\tilde{w}}$ by the definition of $w_{2}(K)$ and the fact that $q^{*}(w)$ decreases in w. firm M’s equilibrium quantity q(K, w) passes point A but not point B. Along ${\bar{q}}(w)$, point A maximizes firm S’s profit; it is also achievable for firm S at $w={\bar{w}}$. Along $q^{*}(w)$, the optimal point achievable for firm S is point V at $w=w_{2}(K)$ . Along UV,  firm S’s profit is $(w-v)K$, which is maximized at $w=w_{2}(K)$. Thus, firm S shall compare two prices: $w_{2}(K)$ and ${\bar{w}}$. Since firm S’s profit along $q^{*}(w)$, given by $(w-v)q^{*}(w)$ , decreases in w for $w>w^{*}$, we have $(w_{2}(K)-v)K=(w_{2}(K)-v)q^{ *}(w_{2}(K))\le ({\tilde{w}}-v)q^{*}({\tilde{w}})=({\bar{w}}-v){\bar{q}}( {\bar{w}})$, where equality holds only at $K= q^{*}({\tilde{w}})$. It follows that ${\bar{w}}$ is optimal for firm S.

Case 2:

$K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$. It holds that $w^{*}<w_{2}(K)< {\tilde{w}}$. There are two subcases: (a) $q^{*}(w^{*})\le {\bar{q}}({\bar{w}})$, and (b) $q^{*}(w^{*})> {\bar{q}}({\bar{w}})$.

2a:

$q^{*}(w^{*})\le {\bar{q}}({\bar{w}})$: When $q^{*}(w^{*})\le {\bar{q}}({\bar{w}})$, q(K, w) passes point A but not point B. Along $q^{*}(w)$ and segment UV on q(K, w), point V is optimal for firm S. Thus, firm S again compares two prices: $w_{2}(K)$ and ${\bar{w}}$. Since $(w-v)q^{*}(w)$ decreases in w for $w>w^{*}$, we have $(w_{2}(K)-v)K=(w_{2}(K)-v)q^{*}(w_{2}(K))> ({\tilde{w}}-v)q^{*}({\tilde{w}})=({\bar{w}}-v){\bar{q}}({\bar{w}})$. It follows that $w_{2}(K)$ is optimal for firm S.

2b:

$q^{*}(w^{*})>{\bar{q}}({\bar{w}})$: If $K\le {\bar{q}}({\bar{w}})$, q(K, w) passes point A but not point B. The proof is the same as in 2a). Consider the case when $K>{\bar{q}}({\bar{w}})$. Now neither point A nor point B is on q(K, w). Along ${\bar{q}}(w)$, it is optimal for firm S to set $w=w_{1}(K)$ at point U; along $q^{*}(w)$, setting $w=w_{2}(K)$ is optimal (at point V). As $w_{2}(K)>w_{1}(K)$, point V strictly dominates point U for firm S since $(w_{2}(K)-v)K>(w_{1}(K)-v)K$ . Thus, $w_{2}(K)$ is optimal for firm S.

Case 3 :

$K\ge q^{*}(w^{*})$: There are again two subcases: (a) $K\le {\bar{q}}({\bar{w}})$ and (b) $K>{\bar{q}}({\bar{w}})$.

3a:

$K\le {\bar{q}}({\bar{w}})$: Both points A and B are on q(K, w) . Firm S sets $w={\bar{w}}$ for her profit at point A along ${\bar{q}}(w)$ and $w=w^{*}$ for her profit at point B along $q^{*}(w)$. Since $(w^{*}-v)q^{*}(w^{*})>({\bar{w}}-v){\bar{q}}({\bar{w}})$, $w=w^{*}$ is optimal for firm S.

3b:

$K>{\bar{q}}({\bar{w}})$: Point B is on q(K, w) but not point A. Along ${\bar{q}}(w)$, firm S shall set $w=w_{1}(K)$ to maximize her profit (at point U); along $q^{*}(w)$, firm S then sets $w=w^{*}$ to arrive at point B. Since $(w^{*}-v)q^{*}(w^{*})>({\bar{w}}-v){\bar{q}}({\bar{w}})$, and point A dominates point U along ${\bar{q}}(w)$, $w=w^{*}$ is optimal for firm S.

Collectively, the optimal w for firm S is as expressed in the lemma. $\square$

Proof of Theorem 1

First, we show that firm M strictly prefers $K\ge q^{*}(w^{*})$ to $K\in (q^{*}({\tilde{w}} ),q^{*}(w^{*}))$. By Lemma 3, for $K\ge q^{*}(w^{*})$, $w=w^{*}$ in stage 2 and firm M will produce $q^{*}(w^{*})$ and get profit $\pi _{m}^{*}-F$. On the other hand, for $K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$, $w=w_{2}(K)$ in stage 2 and firm M produces $q=q^{*}(w_{2}(K))=K$. firm M’s profit is given by $\pi _m^V-F$, with

$$\begin{aligned} \pi _{m}^{V}\equiv \pi _{m}(w_2(K),q^{*}(w_2(K)))=P(K)K-C(K)-w_{2}(K)K. \end{aligned}$$

In either case, there is no outsourcing in stage 3. We use $\pi _{m}(w,q^{*}(w))-F$ to denote firm M’s profit when he produces $q^{*}(w)$ at given w without outsourcing, where

$$\begin{aligned} {\ \pi _{m}(w,q^{*}(w))=P(q^{*}(w))q^{*}(w)-C(q^{*}(w))-wq^{*}(w).\ } \end{aligned}$$

By the envelope theorem, we obtain that

$$\begin{aligned} \frac{d\pi _{m}(w,q^{*}(w))}{dw}=\frac{\partial \pi _{m}(w,q^{*}(w)) }{\partial w}=-q^{*}(w)<0. \end{aligned}$$

Since $w^{*}< w_{2}(K)$ for $K<q^{*}(w^{*})$, it follows that $\pi _{m}^{*}> \pi _{m}^{V}$. Thus $K\ge q^{*}(w^{*})$ dominates $K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$ for firm M.

Second, by Lemma 3, any $K\le q^{*}({\tilde{w}})$ leads firm M to produce ${\bar{q}}({\bar{w}})$ and firm M engages in partial outsourcing in the production stage. It is optimal for firm M to set $K=q^{*}({\tilde{w}})$ to minimize his outsourcing cost $m({\bar{q}}({\bar{w}} )-K)$. Accordingly, firm M’s equilibrium profit under partial outsourcing is at $K=q^{*}({\tilde{w}})$ and is given by

$$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})-F. \end{aligned}$$

Therefore, when firm M chooses K, he compares his profit at $K\ge q^{*}(w^{*})$ (with full in-house production) to his profit at $K=q^{*}({\tilde{w}})$ (with partial outsourcing). In equilibrium he engages in partial outsourcing whenever (8) is satisfied, which is reduced to

$$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})>\pi _{m}^{*}. \end{aligned}$$

(17)

Third, note that $\pi _{m}^{*}$ does not depend on m and at $m=0$, ${\bar{\pi }}_m+mq^{*}({\tilde{w}})=\pi _{m}^{*}$. To prove the theorem, it is sufficient to show that the left-hand-side of (17) strictly increases in m at $m=0$. We obtain that

$$\begin{aligned}&\frac{d}{dm}\left\{ {\bar{\pi }}_m+mq^{*}({\tilde{w}})\right\} |_{m=0} \\&\quad =\frac{\partial lhs}{\partial m}+\frac{\partial lhs}{\partial {\bar{w}}} \frac{d{\bar{w}}}{dm}+\frac{\partial lhs}{\partial {\tilde{w}}}\frac{d{\tilde{w}}}{ dm}\quad \text{ by } \text{ the } \text{ envelope } \text{ theorem } \\&\quad =-[{\bar{q}}({\bar{w}})-q^{*}({\tilde{w}})]-{\bar{q}}({\bar{w}})\frac{d{\bar{w}}}{ dm}+\frac{\partial lhs}{\partial q^{*}({\tilde{w}})}\frac{dq^{*}( {\tilde{w}})}{d{\tilde{w}}}\frac{d{\tilde{w}}}{dm} \\&\quad = -{\bar{q}}({\bar{w}})\frac{d{\bar{w}}}{dm}+m\frac{dq^{*}({\tilde{w}})}{d {\tilde{w}}}\frac{d{\tilde{w}}}{dm}\quad \text{ by } {\bar{q}}({\bar{w}})=q^*({\tilde{w}}) \text{ at } m=0 \\&\quad >0\quad \text{ since } \frac{d{\bar{w}}}{dm}<0 \text{ by } (16). \end{aligned}$$

We conclude that for m sufficiently close to 0, $\pi _{m}^{*}<\bar{ \pi }_m+mq^{*}({\tilde{w}})$. The rest of the theorem follows immediately. $\square$

Proof of Corollary 1

Under Assumption 3, Lemma 1 through Lemma 3 are preserved since capacity building cost is sunk cost in stage 3 and does not affect the quantity decision of firm M. We need to check the validity of the proof of Theorem  1. First, for $K\ge q^{*}(w^{*})$, firm M is optimal setting $K=q^{*}(w^{*})$ when $f^{\prime }(K)>0$. His profit is $\pi _{m}^{*}-f(q^{*}(w^{*}))$.

Second, for $K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$, firm M produces $q=q^{*}(w_{2}(K))=K$ and his profit is $\pi _{m}^{V}-f(K)$. Since

$$\begin{aligned} \frac{d[\pi _{m}(w,q^{*}(w))-f(K)]}{dw}=\frac{\partial \pi _{m}(w,q^{*}(w))}{\partial w}-f^{\prime }(K)\frac{dq^{*}(w)}{dw} =-q^{*}(w)-f^{\prime }(K)\frac{dq^{*}(w)}{dw}, \end{aligned}$$

the sign is ambiguous and it is not determined whether $K=q^{*}(w^{*})$ or $K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$ is optimal for firm M. In either case, firm M does not outsource.

Third, for $K\le q^{*}({\tilde{w}})$, firm M produces ${\bar{q}}({\bar{w}})$ and his profit is denoted as ${\bar{\pi }}_m+mK-f(K)$. It is still true that firm M is optimal setting $K=q^{*}({\tilde{w}})$ since $\frac{d[{\bar{\pi }} _m+mK-f(K)]}{dK}=m-f^{\prime }(K)>0$. Accordingly, firm M’s equilibrium profit under partial outsourcing is

$$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})-f(q^{*}({\tilde{w}})). \end{aligned}$$

Thus when firm M chooses K, he compares his profit with partial outsourcing to the profit with full in-house production. There can be two cases:

• Case 1 Firm M is optimal setting $K=q^{*}(w^{*})$ under full in-house production. If so, condition (8) is rewritten as

  $$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})-f(q^{*}({\tilde{w}}))>\pi _{m}^{*}-f(q^{*}(w^{*})). \end{aligned}$$

  (18)

  Condition (18) is weaker than condition (8) since $f(q^{*}({\tilde{w}}))<f(q^{*}(w^{*}))$.

• Case 2 Firm M is optimal setting $K\in (q^{*}({\tilde{w}}),q^{*}(w^{*}))$ under full in-house production. If so, condition (8) is rewritten as

  $$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})-f(q^{*}({\tilde{w}}))>\pi _{m}^V-f(K). \end{aligned}$$

  (19)

  Again, condition (19) is weaker than condition (8) since $\pi _m^V<\pi _m^{*}$ and $f(q^{*}({\tilde{w}}))<f(K)$ for $K> q^{*}({\tilde{w}})$.

We conclude that there is more partial outsourcing in equilibrium under Assumption 3. $\square$

Proof of Corollary 2

The corollary directly follows from the proof of Theorem 1. $\square$

Proof of Corollary 3

To prove the result, consider

$$\begin{aligned} P^{\prime }(q)q+P(q)-C^{\prime }(q)=\theta . \end{aligned}$$

(20)

Then $q^{*}(w^{*})$ solves (20) at $\theta =w^{*}$ and ${\bar{q}}({\bar{w}})$ solve (20) at $\theta ={\bar{w}}+m$. Let ${\tilde{q}}$ solve (20) at $\theta =v$. Thus ${\tilde{q}}$ is firm M’s monopoly quantity when he vertically integrate with firm S and produces the final good under full capacity. By Assumption 1, the left-hand-side of ( 20) decreases in q. Since $w^{*}>v,{\bar{w}}+m>v$, we have $q^{*}(w^{*})<{\tilde{q}},{\bar{q}}({\bar{w}})<{\tilde{q}}$.

At $m=0$, it holds that $w^{*}={\bar{w}}+m$. By (16) and $\frac{d^{2}{\bar{q}}(w)}{dwdm}=0$, we have

$$\begin{aligned} \frac{d({\bar{w}}+m)}{dm}=1-\frac{\frac{d{\bar{q}}(w)}{dm}}{2\frac{d{\bar{q}}(w)}{ dw}+(w-v)\frac{d^{2}{\bar{q}}(w)}{dw^{2}}}. \end{aligned}$$

Since $\frac{d{\bar{q}}(w)}{dw}=\frac{d{\bar{q}}(w)}{dm}=\frac{1}{P^{\prime \prime }(q)+2P^{\prime }(q)-C^{\prime \prime }}$, reorganizing gives

$$\begin{aligned} \frac{d({\bar{w}}+m)}{dm}=\frac{d{\bar{q}}(w)}{dw}\frac{1-(w-v)(P^{\prime \prime \prime }(q)q+3P^{\prime \prime }(q)-C^{\prime \prime \prime })/H^{2}}{2\frac{ d{\bar{q}}(w)}{dw}+(w-v)\frac{d^{2}{\bar{q}}(w)}{dw^{2}}}. \end{aligned}$$

By Assumption 2, $w-v\ge 0$, $\frac{d{\bar{q}}(w)}{dw}<0$ and $\frac{d^{2}{\bar{q}}(w)}{dw^{2}}\le 0$, it holds that $\frac{d({\bar{w}}+m)}{dm} >0$. Thus ${\bar{w}}+m>w^{*}$ for $m>0$, implying that ${\bar{q}}({\bar{w}} )<q^{*}(w^{*})$. We conclude that firm M produces a lower quantity for the final good under strategic partial outsourcing, leading to lower consumers’ surplus.

In addition, producers’ surplus under strategic partial outsourcing is

$$\begin{aligned} {\overline{PS}}=P({\bar{q}}({\bar{w}})){\bar{q}}({\bar{w}})-C({\bar{q}}({\bar{w}}))-v\bar{q }({\bar{w}})-m({\bar{q}}({\bar{w}})-q^{*}({\tilde{w}})), \end{aligned}$$

and producers’ surplus in status quo is

$$\begin{aligned} PS^{*}=P(q^{*}(w^{*}))q^{*}(w^{*})-C(q^{*}(w^{*}))-vq^{*}(w^{*}). \end{aligned}$$

By Assumption 1 and ${\tilde{q}}>q^{*}(w^{*})>{\bar{q}}({\bar{w}} )>q^{*}({\tilde{w}})$, it follows that $PS^{*}>{\overline{PS}}$. $\square$

Proof of Proposition 1

Since the proof is the same as for Theorem 1, we give only the sketch of the procedure of backward induction.

(1):

The production stage Without partial outsourcing, firm M maximizes his profit $(a-q)q-cq-wq$ and the optimal quantity is

$$\begin{aligned} q^{*}(w)=\frac{1}{2}(a-c-w). \end{aligned}$$

(21)

Instead, if firm M produces with partial outsourcing, his problem is to maximize profit $(a-q)q-cq-wq-m(q-K)$ and the optimal quantity is

$$\begin{aligned} {\bar{q}}(w)=\frac{1}{2}(a-c-w-m). \end{aligned}$$

(22)

(2):

The contracting stage In Stage 2, firm S chooses w to maximize her profit. When firm S anticipates that firm M fully uses in-house production for component N, she maximizes $(w-v)q^{*}(w)$ and the optimal wholesale price is

$$\begin{aligned} w^{*}=\frac{1}{2}(a-c+v). \end{aligned}$$

On the other hand, when firm S anticipates that firm M will produce with partial outsourcing, firm S maximizes $(w-v){\bar{q}}(w)$ and the optimal wholesale price is

$$\begin{aligned} {\bar{w}}=\frac{1}{2}(a-c-m+v). \end{aligned}$$

It is clear that $w^{*}>{\bar{w}}$. There exists a level of the in-house capacity such that in Stage 2, firm S is indifferent between setting $\bar{ w}$ while anticipating that firm M outsources his residual demand and setting ${\tilde{w}}$ while anticipating that firm M produces within his in-house capacity. Here, ${\tilde{w}}$ satisfies

$$\begin{aligned} ({\bar{w}}-v){\bar{q}}({\bar{w}})=({\tilde{w}}-v)q^{*}({\tilde{w}}) \end{aligned}$$

subject to ${\tilde{w}}>{\bar{w}}$. It is uniquely solved as

$$\begin{aligned} {\tilde{w}}=\frac{1}{2}(a-c+v+\sqrt{m[2(a-c-v)-m]}). \end{aligned}$$

The corresponding capacity which makes firm S to be indifferent between ${\bar{w}}$ and ${\tilde{w}}$ is

$$\begin{aligned} q^{*}({\tilde{w}})=\frac{1}{4}(a-c-v-\sqrt{m[2(a-c-v)-m]}). \end{aligned}$$

According to Lemma 3, if and only if $K\le q^{*}( {\tilde{w}})$, firm S finds it optimal to set $w={\bar{w}}$, a lower price of component SS.

(3):

The capacity building stage In Stage 1, by establishing $K\ge q^{*}(w^{*})$, firm M is able to produce without capacity constraint in the production stage and his profit is

$$\begin{aligned} \pi _{m}^{*}=\frac{1}{16}(a-c-v)^{2}. \end{aligned}$$

Whereas at $K=0$, firm M’s profit is

$$\begin{aligned} {\bar{\pi }}_{m}=\frac{1}{16}(a-c-v-m)^{2}. \end{aligned}$$

Instead, by establishing $K=q^{*}({\tilde{w}})$, firm M anticipates that firm S will set $w={\bar{w}}$ so that in Stage 3, firm M outsources quantity

$$\begin{aligned} {\bar{q}}({\bar{w}})-q^{*}({\tilde{w}})=\frac{1}{4}(\sqrt{m[2(a-c-v)-m]}-m). \end{aligned}$$

In this case, firm M’s profit is

$$\begin{aligned} {\bar{\pi }}_{m}+mq^{*}({\tilde{w}})=\frac{1}{16}(a-c-v-m)^{2}+mq^{*}( {\tilde{w}}). \end{aligned}$$

firm M’s problem in Stage 1 is to compare $\pi _{m}^{*}$ to ${\bar{\pi }} _{m}+mq^{*}({\tilde{w}})$ in order to determine the optimal capacity. We find that for $m<{\bar{m}}$, Condition (8) is satisfied. In this case, firm M finds it optimal setting $K=q^{*}({\tilde{w}})$ to induce firm S to charge ${\bar{w}}$, then outsourcing quantity ${\bar{q}}({\bar{w}} )-q^{*}({\tilde{w}})$ for component N. The rest of the proposition follows the fact that ${\bar{\pi }}_{m}+mq^{*}({\tilde{w}})$ is maximized at $m=m^{*}$.

$\square$

Proof of Corollaries 4–6

The results follow from straightforward calculations and thus the details are omitted. $\square$