1 Introduction

Eringen [1] introduced a theory known as nonlocal continuum mechanics to address issues related to small-scale structures. In traditional continuum elasticity, material particles are considered to be continuously distributed, and the stress tensor at a reference point is determined solely by the strain mechanics, which relies on a constitutive function that takes into account the deformation history of all material points in the body. To account for small length scale effects, the theory incorporates internal characteristic length, such as C–C bond lengths, into the constitutive relations. Various problems have led to solutions supporting this theory (Eringen [1, 2], Eringen and Edelen [3]). For example, the dispersion curve predicted by the nonlocal model is basically consistent with the dispersion curve derived from the Born-Karman theory of lattice dynamics. Recently, the nonlocal elasticity theory has been used to analyze the mechanical behavior of small-sized devices in micro-electro-mechanical systems, such as carbon nanotubes, single-layered graphene sheets and nanoswitches. Additionally, the nonlocal theory provides predictions for dislocation core and cohesive stress that align with established knowledge in solid-state physics. Duan and Wang [4] presented an analysis of bending in circular graphene sheets based on the theory of nonlocal elasticity. Zenkour and Abouelregal [5] discussed the influence of thermo-sensitive nanobeams using the thermoelasticity theory of the nonlocal solid with thermal relaxation time. Othman et al. [6] discussed the influence of rotation and memory-dependent derivative on a thermoelastic porous medium using GN (II, III) theory.

Fiber-reinforced composites are lightweight and extremely strong, making them useful in a wide range of constructions. A reinforced concrete member needs to be constructed using the laws of mechanics and intended to withstand all potential stresses. One characteristic that sets apart a reinforced concrete element is that, while both the concrete and the steel are in their elastic condition, they work as one cohesive unit; that is, they are connected to one another in a way that prevents any relative movement between them. The problem of stress on elastic plates reinforced by fibers arranged in concentric circles was examined by Belfield et al. [7]. Chattopadhyay and Choudhury [8] talked about how magneto-elastic shear waves are reflected and how it transmitted in fiber-reinforced media. Wave propagation in fiber-reinforced composite mediums was demonstrated by Singh [9]. The two-dimensional problem of a fiber-reinforced micro-polar thermoelastic half-space was developed by Othman et al. [10]. Said and Othman [11] studied the impact of a magnetic field on a thermoelastic medium with fiber reinforcement under two temperatures as a result of a thermal shock. There are a plenty of works on fiber-reinforced thermoelastic materials using fractional derivatives in the literature Refs. [12,13,14,15,16,17,18,19,20].

Several earlier studies have delved into diverse issues related to a rotating medium. Schoenberg and Censor [21] explored the propagation of plane harmonic vibrations within an elastic medium in rotation. Chand et al. [22] examined the distributions of magnetic fields, stress and deformation in a rotating elastic half-space. Sharma and co-researchers [23, 24] investigated how the presence of rotation influenced the propagation of waves in thermoelastic materials. Othman and Song [25] demonstrated the effects of rotation and magnetic fields on thermoelastic materials. Singh and Tomer [26] discussed the alterations in wave propagation within thermoelastic solids induced by rotation. An innovative model, created by Said [27], introduced a nonlocal thermoelastic half-space with a heat source in motion while subject to rotation. The influence of rotation on elastic solids has been addressed by various authors in references [28,29,30,31,32,33,34,35].

The two-dimensional problem of nonlocal fiber-reinforced thermo-visco-elastic materials impacted by rotation is solved in this paper using a modified Green–Lindsay (MGL), Green–Lindsay (G–L) and Lord–Shulman (L–S) theory. The analytical solution of a physics field is the exact formulation of the physics field obtained using normal model analysis. The impact of nonlocal factors, rotation and viscosity on a fiber-reinforced thermoelastic media was demonstrated by numerical simulation results. An appreciative effect of rotation, viscosity and the nonlocal parameter is shown on the physical variables.

2 The formulation of the study

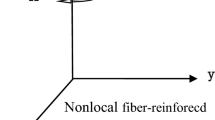
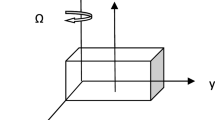
In the framework of the MGL model, the governing equations for a nonlocal fiber-reinforced thermo-visco-elastic medium subjected to rotation can be accurately represented (see Fig. 1), as discussed in the works of Belfield et al. [7], Said [36], Eringen [1, 2], Schoenberg and Censor [22] and Yu et al. [37].

Fig. 1
figure 1

Geometry of the problem

Full size image

The constitutive equations

$$\begin{aligned} & (1 - \varepsilon^{2} \nabla^{2} )\sigma_{ij} = \left( {1 + t_{0} \tau_{1} \frac{\partial }{\partial t}} \right)\left[ {\lambda^{*} e_{kk} \delta_{ij} } \right. \\ & \quad + 2\mu_{T}^{*} e_{ij} + \alpha^{*} (a_{k} a_{m} e_{km} \delta_{ij} + a_{i} a_{j} e_{kk} ) \\ & \quad \left. { + 2(\mu_{L}^{*} - \mu_{T}^{*} )(a_{i} a_{k} e_{kj} + \;a_{j} a_{k} e_{ki} ) + \beta^{*} a_{i} a_{j} a_{k} a_{m} e_{km} } \right] \\ & \quad - \gamma^{*} \left( {1 + t_{1} \tau_{1} \frac{\partial }{\partial t}} \right)\theta \delta_{ij} , \\ \end{aligned}$$
(1)
$$e_{ij} = \frac{1}{2}(u_{i,j} + u_{j,i} ),\quad e_{kk} = \frac{\partial u}{{\partial x}} + \frac{\partial v}{{\partial y}},\quad e = u_{i,i} ,$$
(2)
$$\sigma_{ji,j} = \rho \{ u_{i,tt} + [\Omega \times (\Omega \times u)]_{i} + 2(\Omega \times u_{,t} )_{i} \} ;\quad i,j = 1,2,3.$$
(3)
$$K\nabla^{2} \theta = \left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\rho C_{E} \frac{\partial \theta }{{\partial t}} + \left( {1 + t_{2} \tau_{0} \frac{\partial }{\partial t}} \right)\gamma^{*} T_{0} \frac{\partial e}{{\partial t}},$$
(4)

where \((\tau_{1} \ge \tau_{0} \ge 0)\). The parameters \(\lambda^{*} ,\alpha^{*} ,\mu_{T}^{*} ,\mu_{L}^{*} ,\beta^{*}\) and \(\gamma^{*}\) are defined as \(\lambda^{*} = \lambda (1 + \lambda_{0} \frac{\partial }{\partial t}),\) \(\alpha^{*} = \alpha (1 + \alpha_{0} \frac{\partial }{\partial t}),\)\(\mu_{T}^{*} = \mu_{T} (1 + \alpha_{1} \frac{\partial }{\partial t}),\;\,\,\mu_{L}^{*} = \mu_{L} (1 + \alpha_{2} \frac{\partial }{\partial t}),\)\(\beta^{*} = \beta (1 + \beta_{0} \frac{\partial }{\partial t}),\) \(\gamma^{*} = \gamma (1 + \gamma_{0} \frac{\partial }{\partial t}).\)

Introducing Eqs. (1) and (2) in Eqs. (3) becomes:

$$(1 - \varepsilon^{2} \nabla^{2} )\rho \,\left[ {\frac{{\partial^{2} u}}{{\partial t^{2} }} - \Omega^{2} u - 2\Omega \frac{\partial v}{{\partial t}}} \right] = A_{1} \frac{{\partial^{2} u}}{{\partial x^{2} }} + A_{2} \frac{{\partial^{2} v}}{\partial x\partial y} + A_{3} \frac{{\partial^{2} u}}{{\partial y^{2} }} - \gamma \left( {1 + \gamma_{0} \frac{\partial }{\partial t}} \right)\,\left( {1 + t_{1} \tau_{1} \frac{\partial }{\partial t}} \right)\frac{\partial \theta }{{\partial x}},$$
(5)
$$(1 - \varepsilon^{2} \nabla^{2} )\rho \,\left[ {\frac{{\partial^{2} v}}{{\partial t^{2} }} - \Omega^{2} v + 2\Omega \frac{\partial u}{{\partial t}}} \right] = A_{3} \frac{{\partial^{2} v}}{{\partial x^{2} }} + A_{2} \frac{{\partial^{2} u}}{\partial x\partial y} + A_{4} \frac{{\partial^{2} v}}{{\partial y^{2} }} - \gamma \left( {1 + \gamma_{0} \frac{\partial }{\partial t}} \right)\left( {1 + t_{1} \tau_{1} \frac{\partial }{\partial t}} \right)\frac{\partial \theta }{{\partial y}},$$
(6)

where \(A_{1} = (1 + t_{0} \tau_{1} \frac{\partial }{\partial t})[\lambda^{*} - 2\mu_{T}^{*} + 4\mu_{L}^{*} + 2\alpha^{*} + \beta^{*} ],\,\,\,\,\,\,A_{2} = (1 + t_{0} \tau_{1} \frac{\partial }{\partial t})[\lambda^{*} + \alpha^{*} + \mu_{L}^{*} ],\) \(A_{3} = \mu_{L}^{*} (1 + t_{0} \tau_{1} \frac{\partial }{\partial t}),\,\,\,\,A_{4} = (1 + t_{0} \tau_{1} \frac{\partial }{\partial t})[\lambda^{*} + 2\mu_{T}^{*} ]\).

Now, we present the non-dimensional quantities:

$$\begin{aligned}& (x^{\prime},y^{\prime},\varepsilon^{\prime},u^{\prime},v^{\prime}) = \frac{1}{{l_{0} }}(x,y,\varepsilon ,u,v), \hfill \\ &\theta^{\prime} = \frac{\gamma \theta }{{\lambda + 2\mu_{T} }},\quad \sigma^{\prime}_{ij} = \frac{{\sigma_{ij} }}{{\mu_{T} }}, \hfill \\ &\Omega^{\prime} = \frac{{l_{0} }}{{c_{0} }}\Omega ,\quad l_{0} = \sqrt {\frac{{K^{*} }}{{\rho C_{E} T_{0} }}} , \hfill \\ &(t^{\prime},\tau^{\prime}_{\upsilon } ,\tau^{\prime}_{\theta } ,\tau^{\prime}_{q} ,\lambda_{0}^{\prime } ,\alpha^{\prime}_{0} ,\alpha^{\prime}_{1} ,\alpha^{\prime}_{2} ,\beta^{\prime}_{0} ,\gamma^{\prime}_{0} ) = \frac{{c_{0} }}{{l_{0} }}(t,\tau_{\upsilon } ,\tau_{\theta } ,\tau_{q} ,\lambda_{0} ,\alpha_{0} ,\alpha_{1} ,\alpha_{2} ,\beta_{0} ,\gamma_{0} ), \hfill \\ &c_{0} = \sqrt {\frac{{\lambda + 2\mu_{T} }}{\rho }} . \hfill \\ \end{aligned}$$
(7)

From Eq. (7) in Eqs. (4)–(6), we get

$$(1 - \varepsilon^{2} \nabla^{2} )\left[ {\frac{{\partial^{2} u}}{{\partial t^{2} }} - \Omega^{2} u - 2\Omega \frac{\partial v}{{\partial t}}} \right] = h_{1} \frac{{\partial^{2} u}}{{\partial x^{2} }} + h_{2} \frac{{\partial^{2} v}}{\partial x\partial y} + h_{3} \frac{{\partial^{2} u}}{{\partial y^{2} }} - \left( {1 + \gamma_{0} \frac{\partial }{\partial t}} \right)\,\,\left( {1 + t_{1} \tau_{1} \frac{\partial }{\partial t}} \right)\frac{\partial \theta }{{\partial x}},$$
(8)
$$(1 - \varepsilon^{2} \nabla^{2} )\left[ {\frac{{\partial^{2} v}}{{\partial t^{2} }} - \Omega^{2} v + 2\Omega \frac{\partial u}{{\partial t}}} \right] = h_{3} \frac{{\partial^{2} v}}{{\partial x^{2} }} + h_{2} \frac{{\partial^{2} u}}{\partial x\partial y} + h_{4} \frac{{\partial^{2} v}}{{\partial y^{2} }} - \left( {1 + \gamma_{0} \frac{\partial }{\partial t}} \right)\left( {1 + t_{1} \tau_{1} \frac{\partial }{\partial t}} \right)\frac{\partial \theta }{{\partial y}},$$
(9)
$$\nabla^{2} \theta = d_{1} \left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\frac{\partial \theta }{{\partial t}} + d_{2} \left( {1 + t_{2} \tau_{0} \frac{\partial }{\partial t}} \right)\left( {1 + \gamma_{0} \frac{\partial }{\partial t}} \right)\frac{\partial e}{{\partial t}},$$
(10)

where \((h_{1} ,h_{2} ,h_{3} ,h_{4} ) = \left( {\frac{{A_{1} }}{{\rho c_{0}^{2} }},\frac{{A_{2} }}{{\rho c_{0}^{2} }},\frac{{A_{3} }}{{\rho c_{0}^{2} }},\frac{{A_{4} }}{{\rho c_{0}^{2} }}} \right),\, d_{1} = \frac{{\rho l_{0} c_{0} C_{E} }}{K},\, d_{2} = \frac{{\gamma^{2} l_{0} c_{0} T_{0} }}{{K(\lambda + 2\mu_{T} )}}.\)

3 Method of normal mode analysis

By employing a normal mode analysis, the problem of nonlocal fiber-reinforced rotating thermo-visco-elastic materials is addressed as follows:

$$(u,v,\theta ,\varepsilon ,\sigma_{ij} )(x,y,t) = (\overline{u} ,\overline{v} ,\overline{\theta } ,\overline{\varepsilon } ,\overline{\sigma }_{ij} )(x)e^{{{\text{i}}by - mt}} ,$$
(11)

where \([\overline{u},\overline{v},\overline{\theta } ,\overline{\sigma }_{ij} ]\) are the amplitudes of \([u,v,\theta ,\sigma_{ij} ]\).

Using Eq. (11) in Eqs. (8)–(10), we get

$$(B_{1} - B_{3} D^{2} )\overline{u} + (2\Omega m\varepsilon^{2} D^{2} + ib\overline{h}_{2} D - B_{5} )\overline{v} - B_{6} D\overline{\theta } = 0,$$
(12)
$$(2\Omega m\varepsilon^{2} D^{2} - ib\overline{h}_{2} D - B_{5} )\overline{u} + (B_{2} - B_{4} D^{2} )\overline{v} + ibB_{6} \overline{\theta } = 0,$$
(13)
$$B_{8} D\overline{u} + ibB_{8} \overline{v} + (D^{2} - B_{7} )\overline{\theta } = 0,$$
(14)

where \(D = \frac{{\text{d}}}{{{\text{d}}x}},\) \((\overline{h}_{1} ,\overline{h}_{2} ,\overline{h}_{3} ,\overline{h}_{4} ) = (\frac{{\overline{A}_{1} }}{{\rho c_{0}^{2} }},\frac{{\overline{A}_{2} }}{{\rho c_{0}^{2} }},\frac{{\overline{A}_{3} }}{{\rho c_{0}^{2} }},\frac{{\overline{A}_{4} }}{{\rho c_{0}^{2} }}),\) \(\overline{A}_{1} = (1 - t_{0} \tau_{1} m)[\lambda (1 - \lambda_{0} m) - 2\mu_{T} (1 - \alpha_{1} m) + 4\mu_{L} (1 - \alpha_{2} m) + 2\alpha (1 - \alpha_{0} m) + \beta (1 - \beta_{0} m)],\) \(\overline{A}_{2} = (1 - t_{0} \tau_{1} m)[\lambda (1 - \lambda_{0} m) + \alpha (1 - \alpha_{0} m) + \mu_{L} (1 - \alpha_{2} m)],\,\,\,\,\,\overline{A}_{3} = \mu_{L} (1 - \alpha_{2} m)(1 - t_{0} \tau_{1} m),\) \(\overline{A}_{4} = (1 - t_{0} \tau_{1} m)[\lambda (1 - \lambda_{0} m) + 2\mu_{T} (1 - \alpha_{1} m)],\,\,\,\,\,\,\overline{E} = \overline{{A_{2} }} - \overline{{A_{3} }} ,\,\,\,\,\,B_{1} = \Omega^{2} - m^{2} + \varepsilon^{2} b^{2} (\Omega^{2} - m^{2} ) - \overline{h}_{3} b^{2} ,\) \(B_{2} = - \Omega^{2} + m^{2} - \varepsilon^{2} b^{2} (\Omega^{2} - m^{2} ) + \overline{h}_{4} b^{2} ,\,\,\,\,\,B_{3} = \Omega^{2} \varepsilon^{2} - m^{2} \varepsilon^{2} - \overline{h}_{1} ,\,\,\,\,\,B_{4} = - \Omega^{2} \varepsilon^{2} + m^{2} \varepsilon^{2} + \overline{h}_{3} ,\) \(B_{5} = 2\Omega m(1 + \varepsilon^{2} b^{2} ),\,\,\,\,\,B_{6} = (1 - \gamma_{0} m)(1 - t_{1} \tau_{1} m),\,\,\,\,\,B_{7} = b^{2} - d_{1} m(1 - \tau_{0} m),\,\,\,\,\,B_{8} = d_{2} m(1 - t_{2} \tau_{0} m)(1 - \gamma_{0} m).\)

Eliminating \(\overline{u}(x),\,\;\overline{v}(x)\) and \(\overline{\theta } (x)\) between Eqs. (12)–(14), the following sixth order ordinary differential equations are satisfied by \(\overline{u}(x),\,\;\overline{v}(x)\) and \(\overline{\theta } (x)\) can be obtained

$$[D^{6} - C_{1} D^{4} + C_{2} D^{2} - C_{3} ]\overline{u} (x) = 0,$$
(15)

where \(C_{1} = \frac{{[B_{1} B_{4} + B_{2} B_{3} + b^{2} \overline{h}_{2}^{2} + B_{3} B_{4} B_{7} + B_{4} B_{6} B_{8} - 4B_{7} m^{2} \Omega^{2} \varepsilon^{4} - 4B_{5} m\Omega \varepsilon^{2} ]}}{{B_{3} B_{4} - 4m^{2} \Omega^{2} \varepsilon^{4} }},\) \(C_{2} = \frac{{[B_{1} B_{2} - B_{5}^{2} + B_{1} B_{4} B_{7} + B_{2} B_{3} B_{7} + B_{2} B_{6} B_{8} + B_{7} b^{2} \overline{h}_{2}^{2} - B_{3} B_{6} B_{8} b^{2} - 2B_{6} B_{8} b^{2} \overline{h}_{2} - 4B_{5} B_{7} \Omega m\varepsilon^{2} ]}}{{B_{3} B_{4} - 4m^{2} \Omega^{2} \varepsilon^{4} }},\) \(C_{3} = \frac{{[B_{1} B_{2} B_{7} - B_{5}^{2} B_{7} - B_{1} B_{6} B_{8} b^{2} ]}}{{B_{3} B_{4} - 4m^{2} \Omega^{2} \varepsilon^{4} }}.\)

The bounded solution (\(x \to \infty\)) of Eq. (11) is given as

$$\overline{u} (x) = \sum\limits_{i = 1}^{3} {M_{i} e^{{ - k_{i} x}} } ,$$
(16)
$$\overline{v} (x) = \sum\limits_{i = 1}^{3} {H_{1i} M_{i} e^{{ - k_{i} x}} } ,$$
(17)
$$\overline{\theta } (x) = \sum\limits_{i = 1}^{3} {H_{2i} M_{i} e^{{ - k_{i} x}} } .$$
(18)

Using Eqs. (7), (11) and (16)–(18) in Eq. (1), we obtain

$$\overline{\sigma }_{xx} = \sum\limits_{i = 1}^{3} {H_{3i} M_{i} e^{{ - k_{i} x}} } ,$$
(19)
$$\overline{\sigma }_{xy} = \sum\limits_{i = 1}^{3} {H_{4i} M_{i} e^{{ - k_{i} x}} } ,$$
(20)

where \(H_{1i} = \frac{{ibB_{3} k_{i}^{2} - iB_{1} b + 2\Omega m\varepsilon^{2} k_{i}^{3} + i\overline{h}_{2} bk_{i}^{2} - B_{5} k_{i} }}{{2ib\Omega m\varepsilon^{2} k_{i}^{2} + \overline{h}_{2} b^{2} k_{i} - ibB_{5} - k_{i} B_{2} + B_{4} k_{i}^{3} }},\) \(H_{2i} = \frac{{B_{3} k_{i}^{2} - B_{1} + (B_{5} + i\overline{h}_{2} bk_{i} - 2\Omega m\varepsilon^{2} k_{i}^{3} )H_{1i} }}{{B_{6} k_{i} }},\,\) \(H_{3i} = \frac{{ - \overline{{A_{1} }} k_{i} + ib\overline{E} H_{1i} - (\lambda + 2\mu_{T} )B_{6} H_{2i} }}{{[1 - \varepsilon^{2} (k_{i}^{2} - b^{2} )]\mu_{T} }},\) \(\,H_{4i} = \frac{{\overline{{A_{3} }} (ib - k_{i} H_{1i} )}}{{[1 - \varepsilon^{2} (k_{i}^{2} - b^{2} )]\mu_{T} }}.\)

4 Application

We consider the following initial and regularity conditions to find the constants \(M_{i} (i = 1,2,3)\), which we set for solving the problem at \(x = 0\) are:

  1. (i)

    A thermally insulated boundary condition

    $$\frac{\partial \theta }{{\partial x}} = 0.$$
    (21)
  2. (ii)

    The mechanical boundary conditions

    $$\sigma_{xx} = - f(y,t) = - f^{*} e^{iby - mt} ,\quad \sigma_{xy} = 0,$$
    (22)

    where \(f^{*}\) is the initial stress's magnitude.

By inserting Eqs. (18)–(20) in Eqs. (21) and (22), we have

$$\sum\limits_{i = 1}^{3} {k_{i} H_{2i} M_{i} } = 0,\quad \sum\limits_{i = 1}^{3} {H_{3i} M_{i} } = - f^{*} ,\quad \sum\limits_{i = 1}^{3} {H_{4i} M_{i} } = 0.$$
(23)

Upon solving aforementioned set of Eq. (23), by utilizing the inverse matrix method, we arrive at the expressions for displacements, temperature and stress components.

$$\left( {\begin{array}{*{20}c} {M_{1} } \\ {M_{2} } \\ {M_{3} } \\ \end{array} } \right) = \left( {\begin{array}{*{20}c} {k_{1} H_{21} } &\quad {k_{2} H_{22} } & \quad {k_{3} H_{23} } \\ {H_{31} } & \quad {H_{32} } &\quad {H_{33} } \\ {H_{41} } &\quad {H_{42} } & \quad {H_{43} } \\ \end{array} } \right)^{ - 1} \left( {\begin{array}{*{20}c} 0 \\ { - f^{*} } \\ 0 \\ \end{array} } \right).$$
(24)

5 Special cases

  1. (a)

    A rotating visco-thermoelastic solid with fiber reinforcement from the previous equation with \(\varepsilon = 0.\)

  2. (b)

    A nonlocal fiber-reinforced visco-thermoelastic solid from the above equation with \(\Omega = 0.\)

  3. (c)

    From the equation above, we obtain a rotating nonlocal fiber-reinforced thermoelastic solid with \(\alpha_{0} ,\alpha_{1} ,\alpha_{2} ,\beta_{0} ,\gamma_{0} = 0.\)

  4. (d)

    The modified Green–Lindsay (MGL) theory: \(t_{0} = t_{1} = t_{2} = 1\,.\)

  5. (e)

    \(t_{0} = t_{2} = 0,\) \(t_{1} = 1,\) according to the Green–Lindsay (GL) hypothesis.

  6. (f)

    \(t_{0} = t_{1} = 0,\) \(t_{2} = 1,\) according to the Lord and Shulman (LS) hypothesis.

6 Discussion and numerical findings of the problem

To explain the theoretical results for the study, we present the physical constants as (Othman et al. [38]):

\(\Omega = 0.2,\,\,\,\varepsilon = 0.8,\,\,\,\tau_{0} = 7 \times 10^{ - 4} \,{\text{s}},\,\,\,\tau_{1} = 9 \times 10^{ - 1} {\text{s}},\,\,\,K = 386\,{\text{w}}\,{\text{m}}^{ - 1} \,{\text{K}}^{ - 1} ,\,\,\,\,k^{*} = 386\,{\text{w}}\,{\text{m}}^{ - 1} \,{\text{K}}^{ - 1} ,\) \(f^{*} = 1.4,\,\,\,C_{E} = 383.1\,{\text{J}}\,{\text{kg}}^{ - 1} \,{\text{K}}^{ - 1} ,\,\,\,\,\,\lambda = 3.76 \times 10^{9} {\rm N}\,{\rm m}^{ - 2} ,\,\,\,\alpha = - 1.78 \times 10^{9} {\text{K}}^{ - 1} ,\,\,\,T_{0} = 293\,{\text{K}},\) \(\beta = 2 \times 10^{9} {\rm N}\,{\rm m}^{ - 2} ,\,\,\,\mu_{T} = 2.86 \times 10^{9} {\rm N}\,{\rm m}^{ - 2} ,\,\,\mu_{L} = 7.86 \times 10^{9} {\rm N}\,{\rm m}^{ - 2} ,\,\,\,\mu = 3.86 \times 10^{9} {\rm N}\,{\rm m}^{ - 2} ,\,\) \(\alpha_{T} = 2.78 \times 10^{ - 4} {\text{K}}^{ - 1} ,\,\,\lambda \,_{0} = 1.97\,{\text{s}}^{ - 1} ,\,\,\,\alpha_{0} = 1.98\,{\text{s}}^{ - 1} ,\,\,\,\alpha_{1} = 1.93\,{\text{s}}^{ - 1} ,\,\,\,\alpha_{2} = 1.95\,{\text{s}}^{ - 1} ,\,\,\beta_{0} = 1.96\,{\text{s}}^{ - 1} ,\,\,\) \(\,\gamma_{0} = 1.25\,s^{ - 1} ,\,\,\,\rho = 7800\,{\text{kg}}\,{\text{m}}^{{ - {3}}} ,\,\,\,m_{0} = - \;0.8,\,\,\,m = m_{0} + {\text{i}}\zeta ,\,\,\,b = 0.6,\,\,\,\zeta = 0.5,\,\,\,t = 0.2\,{\text{s}},\,\,\,y = 0.4\,{\text{m}}{.}\)

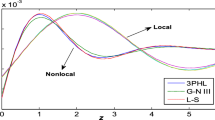
Figure 2 shows that the horizontal displacement \(u\) distribution always begging from positive value. In the context of the three theories (MGL, GL, LS), \(u\) decrease in the range \(0 \le x \le 10\) and converges to zero with increase in the distance \(x\) at \(x \ge 10\). Figure 3 explains that the thermal temperature \(\theta\) distribution always begging from positive value. However, \(\theta\) decreases in the range \(0 \le x \le 10\) in the context of the three theories, and then converges to zero with increase in the distance \(x\) at \(x \ge 10\). Figure 4 depicts that the stress component \(\sigma_{xy}\) begins from zero and satisfies the boundary conditions at \(x = 0\) in the context of the three theories. The values of \(\sigma_{xy}\) in the presence and absence of rotation \((\Omega = 0.2,0)\) decrease to a minimum value in the range \(0 \le x \le 2.3,\), then increase in the range \(2.3 \le x \le 10,\) and also move in wave propagation. It is clear from this figure that the values of \(\sigma_{xy}\) with rotation are higher than that without rotation. Figure 5 shows the distribution of the stress component \(\sigma_{xx}\) and demonstrates that it reaches a zero value and satisfies the boundary conditions at \(x = 0\). In the context of the three theories, the values of \(\sigma_{xx}\) increase in the range \(0 \le x \le 10,\) and converge to zero with increase in the distance \(x\) at \(x \ge 10.\)

Fig. 2
figure 2

Horizontal displacement distribution \(u\) in the absence and presence of rotation

Full size image
Fig. 3
figure 3

Thermal temperature distribution \(\theta\) in the absence and presence of rotation

Full size image
Fig. 4
figure 4

Distribution of stress component \(\sigma_{xy}\) in the absence and presence of rotation

Full size image
Fig. 5
figure 5

Distribution of stress component \(\sigma_{xx}\) in the absence and presence of rotation

Full size image

Figures 6, 7, 8 and 9 display the displacement component \(u\), thermal temperature \(\theta\) and stress component \(\sigma_{xy} ,\) \(\sigma_{xx}\) for different values of the nonlocal parameter \((\varepsilon = 0.8,0)\). Figure 6 shows that based on the three theories (MGL, GL, LS), the values of the horizontal displacement \(u\) decrease in the range \(0 \le x \le 10\) and converge to zero with increase in the distance \(x\) at \(x \ge 10\) for \((\varepsilon = 0.8,0)\). Figure 7 shows that the distribution of the thermal temperature \(\theta\) decreases in the range \(0 \le x \le 10\) with and without nonlocal parameter. Figure 8 depicts that the distribution of the stress component \(\sigma_{xy} ,\) in the context of the three theories, begins from zero point and satisfies the boundary conditions at \(x = 0.\) The value of the component stress \(\sigma_{xy} ,\) with and without nonlocal parameter \((\varepsilon = 0.8,0),\) attains its minimum values, then increases and takes a wave behavior. Figure 9 shows the distribution of the stress component \(\sigma_{xx}\) in the presence and absence of nonlocal parameter. It starts from a negative point and increases until it approaches zero. We also note that the boundary conditions are fulfilled at \(x = 0.\)

Fig. 6
figure 6

Horizontal displacement distribution \(u\) for local and nonlocal parameter

Full size image
Fig. 7
figure 7

Thermal temperature distribution \(\theta\) for local and nonlocal parameter

Full size image
Fig. 8
figure 8

Distribution of stress component \(\sigma_{xy}\) for local and nonlocal parameter

Full size image
Fig. 9
figure 9

Distribution of stress component \(\sigma_{xx}\) for local and nonlocal parameter

Full size image

Figures 10, 11, 12 and 13 show the comparisons between \(u,\)\(\theta ,\) and \(\sigma_{xy} ,\) \(\sigma_{xx}\) in the presence and absence of the viscosity. Figure 10 shows the variation of \(u\), it starts from positive values and reaches the minimum magnitude. It has the same behavior with and without viscosity. Figure 11 shows the variation of the thermal temperature \(\theta\) with and without viscosity, the values of \(\theta\) decrease in the range \(0 \le x \le 10,\) also observe that the values of \(\theta\) under the G–L theory are larger than that under L–S theory while they are lower than those under MGL model. Figure 12 depicts that the distribution of \(\sigma_{xy} ,\) begins from zero and satisfies the boundary condition at \(x = 0.\) In the presence of the viscosity, the values of \(\sigma_{xy}\) start with decrease to a minimum value in the range \(0 \le x \le 2.4\) then increase in the range \(2.4 \le x \le 10,\) but the values of \(\sigma_{xy}\) in the absence of the viscosity start with decrease to a minimum value in the range \(0 \le x \le 2\) then increase in the range \(2 \le x \le 10.\) Figure 13 explains that the variation of \(\sigma_{xx}\) starts from a negative value and reaches its maximum value. It has the same behavior with and without viscosity.

Fig. 10
figure 10

Vertical displacement distribution \(u\) with and without viscosity

Full size image
Fig. 11
figure 11

Thermal temperature distribution \(\theta\) with and without viscosity

Full size image
Fig. 12
figure 12

Distribution of stress component \(\sigma_{xy}\) with and without viscosity

Full size image
Fig. 13
figure 13

Distribution of stress component \(\sigma_{xx}\) with and without viscosity

Full size image

Figures 14, 15, 16 and 17 depict the comparisons between \(u\), \(\theta\) and \(\sigma_{xy} ,\sigma_{xx}\) with and without the reinforcement. Figures 14 and 15 show that the reinforcement parameters in the context three theories lead to a decrease in the values of \(u\) and \(\theta\) in the range \(0 \le x \le 10.\) Figure 16 shows that the changes in \(\sigma_{xy}\) originate from a zero value and adhere to the boundary condition (refer to Eq. (22)) at \(x = 0\). It subsequently reaches its minimum values, then progresses to its maximum values, displaying a wave-like behavior. Figure 17 indicates that the values of \(\,\sigma_{xx}\) increase in the range \(0 \le x \le 10\) and then tend to zero with and without reinforcement. This figure makes it evident that the values of \(\,\sigma_{xx}\) are greater when the reinforcement parameter is absent compared to when it is present.

Fig. 14
figure 14

Horizontal displacement distribution \(u\) in the absence and presence of reinforcement

Full size image
Fig. 15
figure 15

Thermal temperature distribution \(\theta\) in the absence and presence of reinforcement

Full size image
Fig. 16
figure 16

Distribution of stress component \(\sigma_{xy}\) in the absence and presence of reinforcement

Full size image
Fig. 17
figure 17

Distribution of stress component \(\sigma_{xx}\) in the absence and presence of reinforcement

Full size image

Figures 18 and 19 present 3D surface plots illustrating the stress components \(\sigma_{xy} ,\) \(\,\sigma_{xx}\) providing insights into the behavior of a nonlocal fiber-reinforced thermoelastic solid subjected to rotation within the MGL model. These figures are of utmost significance in examining how these field quantities depend on the vertical distance.

Fig. 18
figure 18

Distribution of stress component \(\sigma_{xy}\) in the context of MGL theory

Full size image
Fig. 19
figure 19

Distribution of stress component \(\sigma_{xx}\) in the context of MGL theory

Full size image

7 Conclusion

This study attempts to provide a mathematical model for determining the behavior of displacement, temperature and stress components in nonlocally rotating fiber-reinforced visco-thermoelastic media based on the modified Green–Lindsay theory using normal mode techniques. Display theoretical and numerical results, the nonlocal parameters rotation, viscosity parameters and gain parameters have a significant impact on the physics considered. The important conclusions of this study can be expressed as follows:

  • The local parameter significantly affects the changes in physical quantities.

  • The values of all physical fields converge to zero with an increase in the distance \(x\) and all functions are continuous.

  • The rotation and the viscosity parameters have significantly effects on the variations in physical quantities.

  • The reinforcement has settings that significantly affect the physics quantities.

  • The deformation of an object depends on the forces and boundary conditions.

This work can be used for theoretical modeling of fiber-reinforced visco-thermoelastic analysis under extreme conditions. Finally, the research results derived here can be applied in various fields due to their useful aspects in engineering, geophysics, earthquake estimation, etc.