1 Introduction

Hyperthermia is a therapeutic approach that utilizes physical methods to heat a specific organ or tissues to temperatures ranging from 40 to 44 °C for over 30 min. Typically, cancer cells have a higher susceptibility to death when exposed to temperatures above 42.5 °C, and this effect becomes more pronounced as the temperature increases Moroz et al. [1]. Ideally, hyperthermia treatment should selectively eliminate cancer cells without harming adjacent healthy tissues. Magnetic fluid hyperthermia is a modality of hyperthermia used for tumor treatments, and comprehending the temperature elevation process occurring in biological tissues during this treatment is crucial. It is essential to know the temperature distribution both inside and outside the target region as a function of exposure time to attain a therapeutic temperature and prevent overheating and damage to surrounding healthy tissue. The subject of hyperthermia in medicine is fascinating, particularly in the context of using lasers as external heat sources for thermal treatment. This approach offers several advantages over conventional therapies, including reduced procedure time, the ability to sculpt tissue, less bleeding resulting in less trauma, decreased postoperative pain, and other benefits [2]. Researchers have conducted various studies on the application of thermal transmission to living tissue in order to enhance treatment protocols, develop more advanced and precise temperature forecasting technologies, and ultimately cure cancer [3]. Thermal cell death happens through two mechanisms: necrosis (fast and strong heating for a short period of time) and apoptosis (moderate heating for a prolonged length of time) [4]. Nonetheless, there are just a few studies reports on real-time experimental hyperthermia therapy of pigs [5] and artificial tissue phantoms [6].

Pennes [7] discussed the temperature distribution in the forearm. One can often explore a variety of approaches to solve the time-dependent heat transfer equation in order to model infinite heat propagation based on classical Fourier heat conduction. This is done in order to get a solution. Eringen [8] was the first to propose the concept of nonlocal elastic theory, and after two years, he further investigated nonlocal thermoelasticity in his work [9]. He conducted a review of the constitutive relationships, basic formulations, equilibrium laws in continuum mechanics, and temperature under the nonlocal thermoelastic theory. Wang and Dhaliwal [10] elaborated on the distinctiveness of the non-local thermoelasticity theory, while Eringen [11] examined non-local electromagnetic solids and superconductivity under the framework of elasticity theory.

Despite the highly nonhomogeneous internal structure of living tissues, heat still propagates at a finite rate. To address the issue with Penne's bioheat equation, a thermal waves constitutive relation, as presented in [12, 13], is used to establish the heat wave model of bio-heat transmission. Ahmadikia et al. [14] provided an analytical solution for the parabolic-hyperbolic bio-heat equation in skin tissue subjected to continuous and transient heat flux. To accurately capture the temperature sensitivity of living tissue in therapeutic scenarios under both Fourier and non-Fourier heat transfer conditions, Kundu [15] employed the variable separation method. Kumar et al. [16] investigated the application of a dual-phase lag model for nonlocal heat conduction in a bi-layer tissue during magnetic fluid hyperthermia. A study by Abbas et al. [17] explored the use of nonlocal heat conduction to model the heat transfer in biological tissue caused by laser irradiation. Ghanmi and Abbas [18] conducted an analytical study on the transient heating of skin tissue during thermal therapy. Alzahrani and Abbas [19] utilized experimental data to conduct an analytical study estimating the temperature in living tissue resulting from laser irradiation. Hobiny and Abbas [20] investigated the analytical solutions of the fractional bioheat model in a tissue sphere.

Due to their small size, nanoparticles have the capability to detect even minor changes in a small portion of cells, making them useful for distinguishing between healthy and malignant cells. This can lead to earlier detection and treatment of cancer, particularly in its early stages when cancer cells are just starting to divide. Nanotechnology may also have potential applications in cancer imaging tests. A key benefit of nanotechnology in cancer therapy is its ability to target tumors accurately. This requires selectively eliminating healthy cells while identifying and targeting cancerous ones, which can be achieved through either passive or active targeting methods [21, 22].

Nanoparticles coated with antibodies or chemicals are more likely to bind to cancer cells. It is possible to coat the particles with chemicals that produce a signal upon contact with cancer cells [23]. Both aggressive and passive strategies are focused on targeting cancer cells that are particularly sensitive to nanomaterials [24]. Nanotechnology has the potential to improve the safety and effectiveness of cancer therapies. Nanoparticles with specific shapes and sizes can be utilized to deliver chemotherapy directly to cancer cells. Based on the literature review on bioheat modeling that was done in the preceding paragraphs, a small number of research studies [25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50] have concentrated on using various external heat sources for thermal treatment.

This study aims to provide an overview of the analytical methods used to estimate the temperature increment in living tissue in cancer thermal therapy. Graphical representations and discussions are presented to illustrate the impacts of nonlocal parameter on the physical field distributions of biological tissues. The numerical findings can help to understand the interactions between biological tissues and tools. The numerical results are verified by comparing them with the results reported in previous literature.

2 Mathematical model

Following Eringen [51, 52] and Cheng and Liu [53], the general forms of bioheat transfer in the tumor and normal tissues domains are given as [16, 17]:

$$ k_{1} \nabla^{2} T_{1} = \left( {1 - \alpha^{2} \nabla^{2} } \right)\left( {\rho_{1} c_{1} \frac{{\partial T_{1} }}{\partial t} - Q_{b1} - Q_{m1} - Q_{ext} } \right), \quad 0 < r \le R, $$
(1)
$$ k_{2} \nabla^{2} T_{2} = \left( {1 - \alpha^{2} \nabla^{2} } \right)\left( {\rho_{2} c_{2} \frac{{\partial T_{2} }}{\partial t} - Q_{b2} - Q_{m2} } \right), \quad R \le r \le b, $$
(2)

where \(\mathrm{\alpha }\) is the nonlocal parameter, \(t\) is the time, \({\rho }_{1}, {c}_{1}, {T}_{1}\) and \({k}_{1}\) refer to the density, specific heat, the temperature and thermal conductivity in the tumor tissues while \({\rho }_{2}, {c}_{2},{T}_{2}\) and \({k}_{2}\) refer to the density, specific heat, the temperature, and thermal conductivity in the healthy tissues, respectively, \({Q}_{m1},{Q}_{m2}\) denotes the generation of metabolic heat in tumor and normal tissues, \({Q}_{b1}, {Q}_{b2}\) refer to the heating sources of blood perfusions which are expressed by [7]

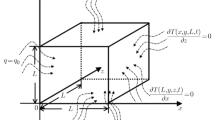
$$ Q_{b1} = \omega_{b} \rho_{b} c_{b} \left( {T_{b} - T_{1} } \right), Q_{b2} = \omega_{b} \rho_{b} c_{b} \left( {T_{b} - T_{2} } \right), $$
(3)

where \({T}_{b}\) refer to the blood temperature, \({\omega }_{b}\) is the blood perfusion rate, \({c}_{b}\) is the blood specific heat, \({\rho }_{b}\) point to the blood mass density and \({Q}_{ext}\) indicates the external heat production per unit of tissue volume. Let us assume that the heat source has a constant power density \(P\) and is constrained to a small sphere with a radius \(R\). This sphere is surrounded by a medium that has uniform heat conductivity. As a result, the temperature distribution in the tumor and nearby healthy tissue changed depending on both the time \(t\) and the distance \(r\) from the sphere's center as in Fig. 1.

Fig. 1
figure 1

Model of a spherical tumor implanted in healthy tissues with a radius of \(R\)

Full size image

The present study assumes that the physiological properties of tumor and normal tissue are constant, and the nonlocal bioheat equations in tumor and healthy tissues can be given by:

$$ k_{1} \left( {\frac{{\partial^{2} T_{1} }}{{\partial r^{2} }} + \frac{2}{r}\frac{{\partial T_{1} }}{\partial r}} \right) = \left( {1 - \alpha^{2} \left( {\frac{{\partial^{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{\partial }{\partial r}} \right)} \right)\left( {\rho_{1} c_{1} \frac{{\partial T_{1} }}{\partial t} + \omega_{b} \rho_{b} c_{b} \left( {T_{1} - T_{b} } \right) - Q_{m1} - P} \right), \quad 0 < r \le R, $$
(4)
$$ \begin{aligned} k_{2} \left( {\frac{{\partial^{2} T_{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{{\partial T_{2} }}{\partial r}} \right) = \left( {1 - \alpha^{2} \left( {\frac{{\partial^{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{\partial }{\partial r}} \right)} \right)\left( {\rho_{2} c_{2} \frac{{\partial T_{2} }}{\partial t} + \omega_{b} \rho_{b} c_{b} \left( {T_{2} - T_{b} } \right) - Q_{m2} } \right), \quad R \le r \le b.\end{aligned} $$
(5)

2.1 Initial and boundary conditions

Now, as in [54, 55], the initial temperature was considered to be the temperature at the boundary of the normal tissues, and the boundary conditions were defined by:

$$ T_{1} \left( {r,0} \right) = T_{b} , \;\frac{{\partial T_{1} \left( {r,0} \right)}}{\partial t} = 0, \;T_{2} \left( {r,0} \right) = T_{b} , \;\frac{{\partial T_{2} \left( {r,0} \right)}}{\partial t} = 0, $$
(6)
$$ \left. {\frac{{\partial T_{1} }}{\partial r}} \right|_{r \to 0} = 0,\quad T_{1} \left( {R,t} \right) = T_{2} \left( {R,t} \right),\quad - k_{1} \left. {\frac{{\partial T_{1} \left( {r,t} \right)}}{\partial r}} \right|_{r = R} = - k_{2} \left. {\frac{{\partial T_{2} \left( {r,t} \right)}}{\partial r}} \right|_{r = R} , \quad T_{2} \left( {b,t} \right) = 0. $$
(7)

The dimensionless variables may be stated appropriately by

$$ T_{1}^{\prime } = \frac{{T_{1} - T_{o} }}{{T_{o} }},\;T_{2}^{\prime } = \frac{{T_{2} - T_{o} }}{{T_{o} }},\;t^{\prime } = \frac{{k_{1} }}{{\rho_{1} c_{1} a^{2} }}t,\;r^{\prime } = \frac{r}{R},\;\alpha^{\prime } = \frac{\alpha }{R},\;k^{\prime } = \frac{{k_{2} }}{{k_{1} }} $$
(8)

Depending on the forms of these variables, the governing Eqs. (4), (5), with initial conditions (6) and boundary conditions (7), may be expressed in terms of these dimensionless form of variables in (8), (for appropriateness, the dash has been omitted)

$$ \frac{{\partial^{2} T_{1} }}{{\partial r^{2} }} + \frac{2}{r}\frac{{\partial T_{1} }}{\partial r} = \left( {1 - \alpha^{2} \left( {\frac{{\partial^{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{\partial }{\partial r}} \right)} \right)\left( {\frac{{\partial T_{1} }}{\partial t} + f_{b1} T_{1} - f_{m1} - f_{r1} } \right), \quad 0 < r \le R, $$
(9)
$$ \frac{{\partial^{2} T_{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{{\partial T_{2} }}{\partial r} = \left( {1 - \alpha^{2} \left( {\frac{{\partial^{2} }}{{\partial r^{2} }} + \frac{2}{r}\frac{\partial }{\partial r}} \right)} \right)\left( {f_{t} \frac{{\partial T_{2} }}{\partial t} + f_{b2} T_{2} - f_{m2} } \right),\quad R \le r \le b, $$
(10)
$$ T_{1} \left( {r,0} \right) = 0, \;\frac{{\partial T_{1} \left( {r,0} \right)}}{\partial t} = 0,\;T_{2} \left( {r,0} \right) = 0, \frac{{\partial T_{2} \left( {r,0} \right)}}{\partial t} = 0, $$
(11)
$$ \left. {\frac{{\partial T_{1} }}{\partial r}} \right|_{r \to 0} = 0,\;T_{1} \left( {R,t} \right) = T_{2} \left( {R,t} \right),\;\frac{{\partial T_{1} \left( {R,t} \right)}}{\partial r} = k\frac{{\partial T_{2} \left( {R,t} \right)}}{\partial r}, \;T_{2} \left( {b,t} \right) = 0, $$
(12)

where \({f}_{b1}=\frac{{\rho }_{b}{\omega }_{b}{c}_{b}{R}^{2}}{{k}_{1}}, {f}_{m1}=\frac{{R}^{2}{Q}_{m1}}{{k}_{1}{T}_{o}}\), \({f}_{r1}=\frac{{R}^{2}P}{{k}_{1}{T}_{o}},{f}_{t}=\frac{{k}_{1}{\rho }_{2}{c}_{2}}{{k}_{2}{\rho }_{1}{c}_{1}},{f}_{b2}=\frac{{\rho }_{b}{\omega }_{b}{c}_{b}{R}^{2}}{{k}_{2}}, {f}_{m2}=\frac{{R}^{2}{Q}_{m2}}{{k}_{2}{T}_{o}}\).

The following formula defines the Laplace transform for a function \(g(r,t)\):

$$ \overline{g}\left( {r,s} \right) = L\left[ {g\left( {r,t} \right)} \right] = \mathop \int \limits_{0}^{\infty } g\left( {r,t} \right)e^{ - st} {\text{d}}t,\; s > 0, $$
(13)

where \(s\) refer to the parameter of Laplace transforms. Hence, the basic equations may be reformulated using the initial conditions as follows:

$$ \frac{{{\text{d}}^{2} \overline{T}_{1} }}{{{\text{d}}r^{2} }} + \frac{2}{r}\frac{{{\text{d}}\overline{T}_{1} }}{{{\text{d}}r}} - a_{1}^{2} \overline{T}_{1} = - g_{1} , \;0 < r \le R, $$
(14)
$$ \frac{{{\text{d}}^{2} \overline{T}_{2} }}{{{\text{d}}r^{2} }} + \frac{2}{r}\frac{{{\text{d}}\overline{T}_{2} }}{{{\text{d}}r}} - a_{2}^{2} \overline{T}_{2} = - g_{2} , \;R \le r \le b, $$
(15)
$$ \left. {\frac{{{\text{d}}\overline{T}_{1} \left( {r,s} \right)}}{{{\text{d}}r}}} \right|_{r \to 0} = 0,\;\overline{T}_{1} \left( {R,s} \right) = \overline{T}_{2} \left( {R,s} \right),\;\frac{{{\text{d}}\overline{T}_{1} \left( {R,s} \right)}}{{{\text{d}}r}} = k\frac{{{\text{d}}\overline{T}_{2} \left( {R,s} \right)}}{{{\text{d}}r}}, \;\overline{T}_{2} \left( {b,s} \right) = 0, $$
(16)

where \({a}_{1}^{2}=\frac{\left(s+{f}_{b1}\right)}{\left(1+{\alpha }^{2}\left(s+{f}_{b1}\right)\right)}\), \({a}_{2}^{2}=\frac{\left(s{f}_{t}+{f}_{b2}\right)}{\left(1+{\alpha }^{2}\left(s{f}_{t}+{f}_{b2}\right)\right)}\), \({g}_{1}=\frac{\frac{{f}_{m1}+{f}_{r1}}{s}}{\left(1+{\alpha }^{2}\left(s+{f}_{b1}\right)\right)}\), \({g}_{2}=\frac{\frac{{f}_{m2}}{s}}{\left(1+{\alpha }^{2}\left(s{f}_{t}+{f}_{b2}\right)\right)}\).

The general solutions \({\overline{T} }_{1}\) and \({\overline{T} }_{2}\) of the nonhomogeneous Eqs. (14) and (15) can be given by

$$ \overline{T}_{1} \left( {r,s} \right) = \frac{{{\text{e}}^{{ - a_{1} r}} }}{r}A_{1} + \frac{{{\text{e}}^{{a_{1} r}} }}{{2ra_{1} }}A_{2} + \frac{{g_{1} }}{{a_{1}^{2} }}, $$
(17)
$$ \overline{T}_{2} \left( {r,s} \right) = \frac{{{\text{e}}^{{ - a_{2} r}} }}{r}A_{3} + \frac{{{\text{e}}^{{a_{2} r}} }}{{2ra_{2} }}A_{4} + \frac{{g_{2} }}{{a_{2}^{2} }}, $$
(18)

where \({A}_{1},{A}_{2}.{A}_{3}\) and \({A}_{4}\) can be determined from the boundary conditions (16) as:

$$ A_{1} = - \frac{{R^{2} {\text{e}}^{{Ra_{1} }} kg_{1} }}{{a_{1}^{2} \left( {1 + {\text{e}}^{{2Ra_{1} }} + Rk + R{\text{e}}^{{2Ra_{1} }} k + Ra_{1} - R{\text{e}}^{{2Ra_{1} }} a_{1} } \right)}}, $$
(19)
$$ A_{2} = - 2a_{1} A_{1} , $$
(20)
$$ A_{3} = \frac{{R{\text{e}}^{{\left( {R + 2b} \right)a_{2} }} \left( { - 1 + ba_{2} } \right)\left( {\left( { - 1 - Ra_{1} + {\text{e}}^{{2Ra_{1} }} \left( { - 1 + Ra_{1} } \right)} \right)a_{2}^{2} g_{1} + a_{1}^{2} \left( {1 + Rk + Ra_{1} + {\text{e}}^{{2Ra_{1} }} \left( {1 + Rk - Ra_{1} } \right)} \right)g_{2} } \right)}}{{a_{1}^{2} \left( { - \left( {\left( {1 + {\text{e}}^{{2Ra_{1} }} } \right)\left( {1 + Rk} \right)} \right) + R\left( { - 1 + {\text{e}}^{{2Ra_{1} }} } \right)a_{1} } \right)a_{2}^{2} \left( {{\text{e}}^{{2ba_{2} }} \left( { - 1 + ba_{2} } \right) + {\text{e}}^{{2Ra_{2} }} \left( {1 + ba_{2} } \right)} \right)}}, $$
(21)
$$ A_{4} = \frac{{2{\text{e}}^{{ - 2ba_{2} }} a_{2} \left( {1 + ba_{2} } \right)}}{{ - 1 + ba_{2} }}A_{3} . $$
(22)

Finally, we apply a numerical inversion approach to discover a general temperature solution that relies on both the t-domain (time) and the r-domain (redial spaces). The numerical results were derived using an approach based on work by Stehfest [56]. The Laplace transform \(\overline{f }\left(r,s\right)\) in this approach has an inverse function \(f\left(r,t\right)\), which is given by the formulation.

$$ f\left( {r,t} \right) = \frac{\ln 2}{t}\mathop \sum \limits_{j = 1}^{M} V_{j} \overline{f}\left( {r,j\frac{\ln 2}{t}} \right), $$
(23)

where \({V}_{j}\) can be given by

$$ V_{j} = \left( { - 1} \right)^{{\frac{M}{2} + 1}} \mathop \sum \limits_{{k = \frac{i + 1}{2}}}^{{\min \left( {i,\frac{M}{2}} \right)}} \frac{{k^{{\frac{M}{2} + 1}} \left( {2k} \right)!}}{{\left( {\frac{M}{2} - k} \right)!k!\left( {i - k} \right)!\left( {2k - 1} \right)!}}. $$
(24)

3 Results and discussion

This section uses the nonlocal bioheat model to explore the temperature estimation in both normal and tumor tissues during hyperthermia treatment. The properties of the blood, normal tissue, and tumor that are relevant to this study have been selected [57, 58].

For tumor:

$$ \begin{aligned} \rho_{1} & = 1660\;\left( {{\text{kg}}} \right)\;\left( {{\text{m}}^{ - 3} } \right),\;k_{1} = 0.778 \;\left( {\text{W}} \right)\;\left( {{\text{m}}^{ - 1} } \right)\left( {{\text{k}}^{ - 1} } \right),\quad \quad c_{1} = 2540\; \left( {\text{J}} \right)\;\left( {{\text{kg}}^{ - 1} } \right)\;\left( {{\text{k}}^{ - 1} } \right), \\ Q_{m1} & = 29 \times 10^{3}\; \left( {\text{W}} \right)\;\left( {{\text{m}}^{ - 3} } \right),\; \omega_{b1} = 0.009\;\left( {{\text{s}}^{ - 1} } \right)\;,\quad \quad R = 0.00315\,\left( {\text{m}} \right) \\ \end{aligned} $$

For normal tissue:

$$ \begin{aligned} \rho_{2} & = 1000\;\left( {{\text{kg}}} \right)\;\left( {{\text{m}}^{ - 3} } \right), \quad k_{2} = 0.642\; \left( {\text{W}} \right)\;\left( {{\text{m}}^{ - 1} } \right)\;\left( {{\text{k}}^{ - 1} } \right),\quad \quad c_{2} = 3720\; \left( {\text{J}} \right)\;\left( {{\text{kg}}^{ - 1} } \right)\;\left( {{\text{k}}^{ - 1} } \right), \\ Q_{m2} & = 450\;\left( {\text{W}} \right)\;\left( {{\text{m}}^{ - 3} } \right), \quad \omega_{b2} = 1.8 \times 10^{ - 4} \;\left( {{\text{s}}^{ - 1} } \right),\quad \quad b = 0.01\;\left( {\text{m}} \right), \\ \end{aligned} $$

Moreover, the other parameters are taken to be:

$$ \begin{aligned} \rho_{b} & = 1060\;\left( {{\text{kg}}} \right)\;\left( {{\text{m}}^{ - 3} } \right),\;c_{b} = 3860\; \left( {\text{J}} \right)\;\left( {{\text{kg}}^{ - 1} } \right)\;\left( {{\text{k}}^{ - 1} } \right),\quad \quad T_{b} = 37\,^{ \circ } {\text{C}},\;T_{o} = 37\,^{ \circ } {\text{C}}, \\ P & = 6.15 \times 10^{6} \;\left( {\text{W}} \right)\;\left( {{\text{m}}^{ - 3} } \right). \\ \end{aligned} $$

This mathematical model which is based on nonlocal bioheat transfer is studied in a wide range of tissue distance \(0<0.00315\le r\le 0.015\) m. MATLAB (R2022a) software was used to perform the calculations, and the results are presented graphically in Figs. 1, 2, 3, 4, 5, 6, 7, 8, 9. The calculations were conducted with \({T}_{o}=37\;^\circ{\rm C} \). As shown in Fig. 2, the effects of nonlocal parameter in the variation of temperature \(\Delta T\) with respect to the radial distance \(r\) under nonlocal bioheat model. Figure 2 displays the variations of temperature along the radial distances \(r\) at \(t=350 \;\mathrm{second}\) for four different values of nonlocal parameter (\(\alpha =\mathrm{0,0.002,0.004,0.006}\)). The plot demonstrates that as the nonlocal parameter value increases, the temperature numerical values decrease. This provides evidence that the nonlocal parameter \(\alpha \) has a decreasing impact on the variation of temperature. Figure 3 shows the temperature variations through the time at the center of the tumor with and without nonlocal parameter. In accordance with the results shown in Fig. 3, the temperature distribution has been steady after \(t=350 \mathrm{s}\). The temperature change of the center of the tumor has a maximum value for the Pennes model \((\alpha =0)\) and decreases for the increasing of nonlocal parameter \((\alpha =\mathrm{0.002,0.004,0.006})\) under nonlocal model. Figure 4 displays the variations of temperature through time on the surface of normal tissues with and without nonlocal parameter. Based on the findings presented in Fig. 4, the temperature distribution has remained constant since \(t=350 s\). For the Pennes and nonlocal models, the temperature change in the surface of normal tissue is greatest for the Pennes model \((\alpha =0)\) and decreases as the nonlocal parameter increases parameter \((\alpha =\mathrm{0.002,0.004,0.006})\). To have a clearer overview of all the results achieved, Figs. 5, 6, 7, and 8 show the temperatures of tumor and normal tissues obtained at the end of 350 s for each value of nonlocal parameter \(\alpha \). Figure 5 displays the contour of temperature through the tumor and normal tissues under Pennes model (\(\alpha =0\)). It observed that the temperature in tumor cells which have the range (\(57.9\;^\circ{\rm C} <T<68.8\;^\circ{\rm C} \)), therefore nonreversible thermal damages will happen for tumor cells. But the temperature in normal cells which have the range (\(38.2\;^\circ{\rm C} <T<57.9\;^\circ{\rm C} \)), which puts healthy tissue at serious risk in the range cells (\(0.00315<r<0.0066\)). Figure 6 shows the contour of temperature through the tumor and normal tissues under nonlocal model (\(\alpha =0.002\)). It is clear that the temperature in tumor cells which have the range (\(54\;^\circ{\rm C} <T<64\;^\circ{\rm C} \)), therefore nonreversible thermal damages will happen for tumor cells. But the temperature in normal cells, which have the range (\(38\;^\circ{\rm C} <T<54\;^\circ{\rm C} \)), which puts healthy tissue at serious risk on the range cells (\(0.00315<r\le 0.006\)). Figure 7 depicts the contour of temperature through the tumor and normal tissues under nonlocal model (\(\alpha =0.004\)). It is observed that the temperature in tumor cells which have the range (\(49\;^\circ{\rm C} <T<54\;^\circ{\rm C} \)), therefor nonreversible thermal damages will happen for tumor cells. But the temperature in normal cells which have the range (\(37.9\;^\circ{\rm C} <T<49\;^\circ{\rm C} \)), which puts healthy tissue at serious risk on the range cells (\(0.00315<r\le 0.0047\)). Figure 8 displays the contour of temperature through the tumor and normal tissues under nonlocal model (\(\alpha =0.006\)). It is clear that the temperature in tumor cells which have the range (\(45\;^\circ{\rm C} <T<49\;^\circ{\rm C} \)), therefore nonreversible thermal damages will happen for tumor cells. But the temperature in normal cells which have the range (\(37.8\;^\circ{\rm C} <T<44.5\;^\circ{\rm C} \)), which puts very minimal damage is observed in the healthy region (\(0.00315<r\le 0.0036\)).

Fig. 2
figure 2

Temperature profile in tumor and normal tissues with and without nonlocal parameter \(\alpha \)

Full size image
Fig. 3
figure 3

Temperature history at tumor center with and without nonlocal parameter \(\alpha \)

Full size image
Fig. 4
figure 4

Temperature history on the surface of normal tissue with and without nonlocal parameter \(\alpha \)

Full size image
Fig. 5
figure 5

The temperature variations in tumor and normal tissue for \(\alpha =0\)

Full size image
Fig. 6
figure 6

The temperature variations in tumor and normal tissue for \(\alpha =0.002\)

Full size image
Fig. 7
figure 7

The temperature variations in tumor and normal tissue for \(\alpha =0.004\)

Full size image
Fig. 8
figure 8

The temperature variations in tumor and normal tissue for \(\alpha =0.006\)

Full size image
Fig. 9
figure 9

a The variation of temperature as in Fig. 3 Cheng and Liu [53]. b The variation of temperature for our present results when \(\alpha =0\). c The variation of temperature after merge a with b using Xfig under Linux

Full size image

3.1 The verification of solutions

Figure 9 are used to compare the present results with those obtained by Cheng and Lui [53] to demonstrate the accuracy of the current findings. Figure 9a shows the results of Cheng and Lui [53] as shown in Fig. 3 which shows the variation of temperature in the center of the tumor \(r=0\) and the surface of normal tissues \(r=R\) through time with and without blood perfusion and metabolism under the Pennes model. Figure 9b displays our results under the Pennes model by using (\(\alpha =0\)). We can merge Fig. 9a with Fig. 9b by using the Xfig program under Linux as in Fig. 9c. The figures indicate a good agreement between the current results and those reported by Cheng and Lui [53] in the literature.

4 Conclusions

Based on the presented findings and results, the following conclusions can be drawn:

  • The numerical study of a nonlocal bioheat transfer model provides valuable new insights compared to the classic Pennes model. Introducing the nonlocal parameter α to account for microvascular architecture more realistically captures temperature variations in tissue.

  • As α increases from 0, temperatures decrease throughout the tumor and normal regions, indicating less diffusion of heat from the source. Higher α leads to more localized heating zones. This improved localization can allow safer delivery of higher temperatures for better therapeutic outcomes while protecting healthy tissue.

  • Across the range of α values studied, temperatures inside the tumor remain in the non-reversible damage range. However, increasing α significantly reduces temperatures in the bordering normal tissue, minimizing thermal damage risks.

  • For the highest α of 0.006, minimal overheating is observed in normal cells located near the tumor. This demonstrates that accounting for even small nonlocal effects helps optimize thermal therapy by improving treatment targeting and safety.

  • Verification with an independent published study showed excellent agreement, validating the accuracy of the current numerical approach.

Overall, the nonlocal bioheat model provides a more realistic representation of true tissue thermal transport compared to the classic local Pennes model. Consideration of nonlocal effects, even for small α, enhances therapeutic windows for treatments like hyperthermia.