An analytical stress–stretch relation for porous elastomeric materials with large deformation

Abstract

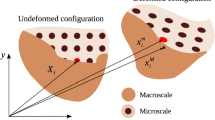
Porous elastomeric materials have wide applications in aerospace, electronics, biomedicine, and other fields. However, closed-form analytical solutions for macroscopic strain energy density of high-order porous elastomers have not been well resolved. In this work, we propose a new approach for constitutive modeling of porous elastomeric materials under large deformation, mainly relying on expressing the macroscopic strain energy density function of the composite material as a function of the strain energy of elastomer matrix via a strain-amplification relation. Such amplification relation, as constructed through a thick-walled sphere volume element model, is utilized in a mapping of the macroscopic deformation to the average deformation of elastomer matrix in the sense of the first invariant by a scaling coefficient–amplification factor, which depends on both the initial void volume fraction and the macroscopic volumetric deformation ratio. The analytical stress–stretch relation is then derived and given in simple form of only three material parameters. Discussions on how the factors affect the amplification factor are made and the behavior of the model is shown in several deformation simulations. The prediction results of this model are compared with those of existing models, and reasonable agreement is obtained.

Appendices

Appendix A: Danielsson–Parks–Boyce (DPB) model

According to Danielsson et al. [13], in order to obtain the macroscopic Cauchy stress tensor ${\overline{\mathbf{T}}}$, one first needs to calculate the macroscopic principal Cauchy stress tensor $\left[ {{\tilde{\overline{\mathbf{T}}}}} \right]_{i}$ through

$$\left[ {{\tilde{\overline{\mathbf{T}}}}} \right]_{i} = \frac{{\overline{\lambda }_{i} }}{{\overline{J}V_{{0}} }}\int_{{\left( {{{{3}f_{0} V_{0} } \mathord{\left/ {\vphantom {{{3}f_{0} V_{0} } {4\pi }}} \right. \kern-0pt} {4\pi }}} \right)^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}^{{\left( {{{{3}V_{0} } \mathord{\left/ {\vphantom {{{3}V_{0} } {4\pi }}} \right. \kern-0pt} {4\pi }}} \right)^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }} {\int_{0}^{2\pi } {\int_{0}^{\pi } {\left( {\frac{{\partial W^{\text{m}} }}{{\partial I_{1}^{\text{m}} }}\frac{{\partial I_{1}^{\text{m}} }}{{\partial \overline{\lambda }_{i} }}} \right)R^{2} \sin \Theta \text{d}\Theta \text{d}\Phi \text{d}R} } } \begin{array}{*{20}c} {} \\ \end{array} \begin{array}{*{20}c} {} \\ \end{array} \left( {i = 1,2,3} \right),$$

(15)

where ${{\partial W^{\text{m}} } \mathord{\left/ {\vphantom {{\partial W^{\text{m}} } {\partial I_{1}^{\text{m}} }}} \right. \kern-0pt} {\partial I_{1}^{\text{m}} }}$ is given by

$$\begin{aligned} \frac{{\partial W^{\text{m}} }}{{\partial I_{1}^{\text{m}} }} = & \frac{\mu }{{6}}\frac{\sqrt N }{{\lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\lambda_{{\text{chain}}} }}{\sqrt N }} \right), \\ \lambda_{{\text{chain}}} = & \sqrt {\frac{1}{{3\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} }}} \\ & \quad \times \sqrt {\left\{ {\overline{I}_{1} \psi^{2} + \left( {\overline{\lambda }_{1}^{2} \sin^{2} \Theta \sin^{2} \Phi + \overline{\lambda }_{2}^{2} \sin^{2} \Theta \cos^{2} \Phi + \overline{\lambda }_{3}^{2} \cos^{2} \Theta } \right)\left( {\psi^{ - 4} - \psi^{2} } \right)} \right\}} , \\ \end{aligned}$$

(16)

and ${{\partial I_{1}^{\text{m}} } \mathord{\left/ {\vphantom {{\partial I_{1}^{\text{m}} } {\partial \overline{\lambda }_{i} }}} \right. \kern-0pt} {\partial \overline{\lambda }_{i} }}$ is given by

$$\begin{aligned} \frac{{\partial I_{1}^{\text{m}} }}{{\partial \overline{\lambda }_{i} }}\text{ = } & - \frac{{2\overline{I}_{1} }}{{3\overline{\lambda }_{i} \overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} }}\psi^{2} \\ & \quad - \frac{2}{{3\overline{\lambda }_{i} \overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} }}\left( {\overline{\lambda }_{1}^{2} \sin^{2} \Theta \sin^{2} \Phi + \overline{\lambda }_{2}^{2} \sin^{2} \Theta \cos^{2} \Phi + \overline{\lambda }_{3}^{2} \cos^{2} \Theta } \right)\left( {\psi^{ - 4} - \psi^{2} } \right) \\ & \quad + \frac{{2\overline{\lambda }_{i} }}{{\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} }}\psi^{2} { + }\frac{{2\overline{I}_{1} \overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}{{3\overline{\lambda }_{i} }}\frac{{3V_{0} }}{{4\pi R^{3} }}\psi^{ - 1} + \frac{{2\overline{\lambda }_{i} }}{{\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} }}\left( {\frac{{X_{i} }}{R}} \right)^{2} \left( {\psi^{ - 4} - \psi^{2} } \right) \\ & \quad - \frac{{2\overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}{{3\overline{\lambda }_{i} }}\frac{{3V_{0} }}{{4\pi R^{3} }}\left( {\overline{\lambda }_{1}^{2} \sin^{2} \Theta \sin^{2} \Phi + \overline{\lambda }_{2}^{2} \sin^{2} \Theta \cos^{2} \Phi + \overline{\lambda }_{3}^{2} \cos^{2} \Theta } \right) \\ & \quad \times \left( {2\psi^{ - 7} + \psi^{ - 1} } \right). \\ \end{aligned}$$

(17)

After calculating $\left[ {{\tilde{\overline{\mathbf{T}}}}} \right]_{i}$, the macroscopic Cauchy stress tensor ${\overline{\mathbf{T}}}$ is then obtained through the rotation of ${\tilde{\overline{\mathbf{T}}}}$ by ${\overline{\mathbf{Q}}}$, i.e., ${\overline{\mathbf{T}}} = {\overline{\mathbf{Q}}}{\overline{\tilde{\mathbf{T}}}}\overline{Q}^{\text{T}}$, where ${\overline{\mathbf{Q}}}$ is the orthogonal rotation tensor that decomposes ${\overline{\mathbf{B}}}$ into a diagonal tensor ${\tilde{\overline{\mathbf{B}}}}$ via ${\overline{\mathbf{B}}} = \overline{Q}\overline{\tilde{B}}\overline{Q}^{\text{T}}$. For the DPB model, it is observed that the integrand of Eq. (15) is rather complex and $\left[ {{\tilde{\overline{\mathbf{T}}}}} \right]_{i}$ may only be solved through numerical integration methods. In addition, the lack of an explicit relationship between the macroscopic Cauchy stress tensor and deformation makes it inconvenient for application, especially in nonlinear problems (e.g., indentation). In this work, we use ten-order Gaussian quadrature for each of the integration variables $\left\{ {\Theta ,\Phi ,R} \right\}$ to generate data for comparison with the results obtained from our model (Fig. 2).

Appendix B: Jacobian matrix

The consistent, closed-form hyperelastic tangent operator (the Jacobian matrix), ${\overline{\mathbf{C}}}$, can be obtained by evaluating

$$\overline{C}_{ijkl} = \frac{1}{2}\overline{J}^{ - 1} \left( {\overline{F}_{lm} \frac{{\partial \left( {\overline{J}\overline{T}_{ij} } \right)}}{{\partial \overline{F}_{km} }} + \overline{F}_{km} \frac{{\partial \left( {\overline{J}\overline{T}_{ij} } \right)}}{{\partial \overline{F}_{lm} }}} \right),$$

(18)

where $\overline{C}_{ijkl}$ is the tangent operator. To simplify derivations, we first make some useful definitions, i.e.,

$$\begin{aligned} c_{1} \equiv & 2 - \frac{1}{{\overline{J}}} - \frac{{f_{0} + 2\left( {\overline{J} - 1} \right)}}{{\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} \eta^{{{{1} \mathord{\left/ {\vphantom {{1} 3}} \right. \kern-0pt} 3}}} }}, \\ c_{2} \equiv & \frac{{\partial c_{1} }}{{\partial \overline{J}}} = \frac{1}{{\overline{J}^{2} }} - \frac{1}{{3\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} \eta^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}\left( {\frac{{\left( {4 - f_{0} } \right)\eta + \left( {1 - f_{0} } \right)}}{{f_{0} \eta^{2} + \left( {1 - f_{0} } \right)\eta }}} \right), \\ c_{3} \equiv & \frac{{\partial c_{2} }}{{\partial \overline{J}}} = - \frac{1}{{\overline{J}^{2} }} - \frac{{\overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}{{9\eta^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}\left( {\frac{1}{{\overline{J}}} - \frac{1}{{\eta f_{0} }}} \right)\left( {\frac{{\left( {4 - f_{0} } \right)\eta + \left( {1 - f_{0} } \right)}}{{f_{0} \eta^{2} + \left( {1 - f_{0} } \right)\eta }}} \right) \\ & \quad + \frac{{\overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}{{3\eta^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}\left( {\frac{{\left( {4 - f_{0} } \right)\eta^{2} f_{0} + \left( {1 - f_{0} } \right)\left( {2\eta f_{0} + \left( {1 - f_{0} } \right)} \right)}}{{f_{0} \left( {f_{0} \eta^{2} + \left( {1 - f_{0} } \right)\eta } \right)^{2} }}} \right). \\ \end{aligned}$$

(19)

Then using the identities

$$\begin{aligned} & \frac{{\partial \overline{J}}}{{\partial \overline{F}_{km} }}{ = }\overline{J}\overline{F}_{mk}^{ - 1} ,\begin{array}{*{20}c} {} \\ \end{array} \begin{array}{*{20}c} {} \\ \end{array} \frac{{\partial \overline{B}_{ij} }}{{\partial \overline{F}_{km} }} = \delta_{ik} \overline{F}_{jm} + \delta_{jk} \overline{F}_{im} ,\begin{array}{*{20}c} {} \\ \end{array} \begin{array}{*{20}c} {} \\ \end{array} \frac{{\partial \overline{I}_{1} }}{{\partial \overline{F}_{km} }} = 2\overline{F}_{km} , \\ & \frac{{\partial \text{L}^{ - 1} \left( y \right)}}{\partial y} \approx \frac{\partial }{\partial y}\left( {y\frac{{3 - y^{2} }}{{1 - y^{2} }}} \right) = \frac{{3 + y^{4} }}{{\left( {1 - y^{2} } \right)^{2} }}, \\ \end{aligned}$$

(20)

we obtain

$$\begin{aligned} \frac{{\partial \Lambda_{{\text{chain}}} }}{{\partial \overline{F}_{km} }} = & \frac{\partial }{{\partial \overline{F}_{km} }}\left( {\sqrt {\frac{{c_{1} \overline{I}_{1} }}{{3\left( {1 - f_{0} } \right)}}} } \right) \\ = & \frac{1}{{2\sqrt {3\left( {1 - f_{0} } \right)c_{1} \overline{I}_{1} } }}\left( {c_{2} \overline{I}_{1} \frac{{\partial \overline{J}}}{{\partial \overline{F}_{km} }} + c_{1} \frac{{\partial \overline{I}_{1} }}{{\partial \overline{F}_{km} }}} \right) \\ = & \frac{1}{{2\sqrt {3\left( {1 - f_{0} } \right)c_{1} \overline{I}_{1} } }}\left( {c_{2} \overline{I}_{1} \overline{J}\overline{F}_{mk}^{ - 1} + {2}c_{1} \overline{F}_{km} } \right). \\ \end{aligned}$$

(21)

Considering $\overline{J}\overline{T}_{ij}$ can be expressed as

$$\overline{J}\overline{T}_{ij} = \frac{\mu }{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right){2}c_{1} \overline{B}_{ij} + \frac{{\mu \overline{I}_{1} \overline{J}}}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)c_{2} \delta_{ij} ,$$

(22)

it is straightforward to obtain the derivative of $\overline{J}\overline{T}_{ij}$ with respect to $\overline{F}_{km}$ as

$$\begin{aligned} \frac{{\partial \left( {\overline{J}\overline{T}_{ij} } \right)}}{{\partial \overline{F}_{km} }} \approx & - \frac{\mu }{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}}^{2} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)2c_{1} \overline{B}_{ij} \frac{{\partial \Lambda_{{\text{chain}}} }}{{\partial \overline{F}_{km} }} \\ & \quad + \frac{\mu }{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\frac{{3 + \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)^{4} }}{{\left( {1 - \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)^{2} } \right)^{2} }}2c_{1} \overline{B}_{ij} \frac{1}{\sqrt N }\frac{{\partial \Lambda_{{\text{chain}}} }}{{\partial \overline{F}_{km} }} \\ & \quad + \frac{\mu }{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)2c_{1} \frac{{\partial \overline{B}_{ij} }}{{\partial \overline{F}_{km} }} + \frac{\mu }{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)2c_{2} \overline{B}_{ij} \frac{{\partial \overline{J}}}{{\partial \overline{F}_{km} }} \\ & \quad + \frac{{\mu \overline{J}}}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)c_{2} \delta_{ij} \frac{{\partial \overline{I}_{1} }}{{\partial \overline{F}_{km} }} - \frac{{\mu \overline{I}_{1} \overline{J}}}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}}^{2} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)c_{2} \delta_{ij} \frac{{\partial \Lambda_{{\text{chain}}} }}{{\partial \overline{F}_{km} }} \\ & \quad + \frac{{\mu \overline{I}_{1} \overline{J}}}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\frac{{3 + \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)^{4} }}{{\left( {1 - \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)^{2} } \right)^{2} }}c_{2} \delta_{ij} \frac{1}{\sqrt N }\frac{{\partial \Lambda_{{\text{chain}}} }}{{\partial \overline{F}_{km} }} \\ & \quad + \frac{{\mu \overline{I}_{1} }}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)c_{2} \delta_{ij} \frac{{\partial \overline{J}}}{{\partial \overline{F}_{km} }} + \frac{{\mu \overline{I}_{1} \overline{J}}}{{6}}\frac{\sqrt N }{{\Lambda_{{\text{chain}}} }}\text{L}^{ - 1} \left( {\frac{{\Lambda_{{\text{chain}}} }}{\sqrt N }} \right)c_{3} \delta_{ij} \frac{{\partial \overline{J}}}{{\partial \overline{F}_{km} }}. \\ \end{aligned}$$

(23)

Substituting Eq. (20) and Eq. (21) in Eq. (23) gives the final expression of ${{\partial \left( {\overline{J}\overline{T}_{ij} } \right)} \mathord{\left/ {\vphantom {{\partial \left( {\overline{J}\overline{T}_{ij} } \right)} {\partial \overline{F}_{km} }}} \right. \kern-0pt} {\partial \overline{F}_{km} }}$; for brevity, we do not write it down here. Once the expression of ${{\partial \left( {\overline{J}\overline{T}_{ij} } \right)} \mathord{\left/ {\vphantom {{\partial \left( {\overline{J}\overline{T}_{ij} } \right)} {\partial \overline{F}_{km} }}} \right. \kern-0pt} {\partial \overline{F}_{km} }}$ is known, one can immediately obtain $\overline{C}_{ijkl}$ by using Eq. (18).

Appendix C: Shrimali–Lefèvre–Lopez-Pamies (SLLP) model

According to Shrimali et al. [21], the approximate solution for $\overline{W}$ for the general case of porous elastomers with any choice of stored-energy function $\psi_{\text{m}}$ for the elastomeric matrix is given by

$$\begin{aligned} & \overline{W}\left( {{\overline{\mathbf{F}}},f_{0} } \right) = \left( {1 - f_{0} } \right)\psi_{\text{m}} \left( {\frac{{I_{1} }}{{1 - f_{0} }} + 3} \right) \quad \text{with} \\ & I_{1} = \frac{{3\left( {1 - f_{0} } \right)}}{{3 + 2f_{0} }}\left[ {\overline{I}_{1} - 3} \right] + \frac{3}{{\overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}\left[ {2\overline{J} - 1 - \frac{{\left( {1 - f_{0} } \right)\overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} \left( {3\overline{J}^{{{2 \mathord{\left/ {\vphantom {2 3}} \right. \kern-0pt} 3}}} + 2f_{0} } \right)}}{{\left( {3 + 2f_{0} } \right)}} - \frac{{f_{0}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} \overline{J}^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} \left( {2\overline{J} + f_{0} - 2} \right)}}{{\left( {\overline{J} - 1 + f_{0} } \right)^{{{1 \mathord{\left/ {\vphantom {1 3}} \right. \kern-0pt} 3}}} }}} \right]. \\ \end{aligned}$$

(24)