Lamb waves in functionally graded magnetoelectric microplates with different boundary conditions

Abstract

Based on the modified couple stress theory, Legendre orthogonal polynomial method is used to solve the propagation characteristics of the Lamb wave in functionally graded magnetoelectric microplates. The correctness of the method is verified by comparisons with the previous literature and the global matrix method. Compared to the global matrix method, the Legendre orthogonal polynomial method provides an efficient numerical method for solving functionally graded magnetoelectric structures without layering and iterative root finding. In this paper, the influences of the couple stress, magnetoelectric coupling and inhomogeneity with different boundary conditions are discussed in detail. It is found that the couple stress effect and magnetoelectric effect are mutually inhibited. This mutual inhibition under the magnetoelectric short circuit boundary condition is stronger than that under the magnetoelectric open circuit boundary condition. The change of the magnetoelectric boundary has significant influence on the piezoelectric material side, but has little influence on the piezomagnetic material side.

Appendix 1

The expression of the displacement components, electric and magnetic potential can be expressed as follows:

$$\{ {\kern 1pt} u_{x}^{(i)} ,u_{z}^{(i)} ,\phi^{(i)} ,\psi^{\left( i \right)} \} = \{ A^{(i)} ,B^{(i)} ,C^{(i)} ,D^{(i)} \} \exp [ik(x + Q^{(i)} z) - i\omega t]{\kern 1pt} \quad \quad i = 1,2...N.$$

(36)

where the superscript (i) represents the i-th layer; A⁽ⁱ⁾, B⁽ⁱ⁾, C⁽ⁱ⁾ and D⁽ⁱ⁾ are the amplitude vectors of the mechanical displacements, electric and magnetic potential; and k and kQ⁽ⁱ⁾ are the wave vectors in x- and z-directions, respectively. The following matrix equation can be obtained by Substituting Eq. (1) into governing equations:

$$\left[ {\begin{array}{*{20}c} \begin{gathered} - C_{11}^{(i)} \, k^{2} - C_{55}^{(i)} \left( z \right)k^{2} (Q^{(i)} )^{2} \hfill \\ - 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \hfill \\ \, k^{4} l^{2} (Q^{(i)} )^{2} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} \hfill \\ - 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \, \hfill \\ k^{4} l^{2} (Q^{(i)} )^{4} \hfill \\ + \omega^{2} \rho^{(i)} \hfill \\ \end{gathered} & \begin{gathered} - C_{13}^{(i)} \, k^{2} Q^{(i)} - C_{55}^{(i)} \, k^{2} Q^{(i)} \hfill \\ + 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \hfill \\ \, k^{4} l^{2} (Q^{(i)} )^{2} \hfill \\ + 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \hfill \\ k^{4} l^{2} (Q^{(i)} )^{3} \hfill \\ \end{gathered} & {( - e_{15}^{(i)} - e_{31}^{(i)} ) \, k^{2} Q^{(i)} } & {( - q_{15}^{(i)} - q_{31}^{(i)} ) \, k^{2} Q^{(i)} } \\ {} & {} & {} & {} \\ \begin{gathered} - C_{13}^{(i)} \, k^{2} Q^{(i)} - C_{55}^{(i)} \, k^{2} Q^{(i)} \hfill \\ + 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \, \hfill \\ k^{4} l^{2} Q^{(i)} \hfill \\ + 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \, \hfill \\ k^{4} l^{2} (Q^{(i)} )^{3} \hfill \\ \end{gathered} & \begin{gathered} - C_{55}^{(i)} k^{2} - 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \, \hfill \\ k^{4} l^{2} \hfill \\ - C_{33}^{(i)} k^{2} (Q^{(i)} )^{2} - 1/12 \, \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right) \, \hfill \\ k^{4} l^{2} (Q^{(i)} )^{2} \hfill \\ + \omega^{2} \rho^{(i)} \hfill \\ \end{gathered} & { - e_{15}^{(i)} \, k^{2} - e_{33}^{(i)} \, k^{2} (Q^{(i)} )^{2} } & { - q_{15}^{(i)} \, k^{2} - q_{33}^{(i)} \, k^{2} (Q^{(i)} )^{2} } \\ \begin{gathered} \hfill \\ ( - e_{15}^{(i)} - e_{31}^{(i)} ) \, k^{2} Q^{(i)} \hfill \\ \hfill \\ ( - q_{15}^{(i)} - q_{31}^{(i)} ) \, k^{2} Q^{(i)} \hfill \\ \end{gathered} & \begin{gathered} \hfill \\ - e_{15}^{(i)} \, k^{2} - e_{33}^{(i)} \, k^{2} (Q^{(i)} )^{2} \hfill \\ \hfill \\ - q_{15}^{(i)} \, k^{2} - q_{33}^{(i)} \, k^{2} (Q^{(i)} )^{2} \hfill \\ \end{gathered} & \begin{gathered} \hfill \\ \varepsilon_{11}^{(i)} k^{2} + \varepsilon_{33}^{(i)} k^{2} (Q^{(i)} )^{2} \hfill \\ \hfill \\ g_{11}^{(i)} k^{2} + g_{33}^{(i)} k^{2} (Q^{(i)} )^{2} \hfill \\ \end{gathered} & \begin{gathered} \hfill \\ g_{11}^{(i)} k^{2} + g_{33}^{(i)} k^{2} (Q^{(i)} )^{2} \hfill \\ \hfill \\ \lambda_{11}^{(i)} k^{2} + \lambda_{33}^{(i)} k^{2} (Q^{(i)} )^{2} \hfill \\ \end{gathered} \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {A^{(i)} } \\ {B^{(i)} } \\ {C^{(i)} } \\ {D^{(i)} } \\ \end{array} } \right] = 0$$

(37)

To solve matrix equation Eq. (37), the determinant of the coefficient matrix must be equal to zero. The ten roots of Q (i) can be obtained by solving equation Eq. (37), so the mechanical displacements can be re-expressed as follows:

$$\{ u_{x}^{(i)} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} u_{z}^{(i)} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} \phi^{(i)} ,\psi^{\left( i \right)} \} = \sum\limits_{j = 1}^{10} {\{ 1,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} f_{j}^{(i)} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} t_{j}^{(i)} ,w_{j}^{\left( i \right)} \} A_{j}^{(i)} \exp (ik(x + Q_{j}^{(i)} z) - i\omega t)} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(38)

Substituting Eq. (38) into constitutive relations, the stress, couple stress, rotation, electrical displacement, magnetic induction, electric and magnetic potential can be re-expressed as linear combinations:

$$\sigma_{zz}^{(i)} = \sum\limits_{j = 1}^{10} {A_{j}^{(i)} \left( {ikC_{13}^{(i)} - ikf_{j}^{(i)} C_{33}^{(i)} Q_{j}^{(i)} - ikt_{j}^{(i)} e_{33}^{(i)} Q_{j}^{(i)} - ikq_{33}^{(i)} w_{j}^{(i)} Q_{j}^{(i)} } \right)\exp (ik(x + Q_{j}^{(i)} z) - i\omega t} .$$

(39)

$$\begin{gathered} \sigma_{zx}^{(i)} = \sum\limits_{j = 1}^{10} {A_{j}^{(i)} (C_{55}^{(i)} (ik - 0.25ik^{3} ly^{2} - 0.25ik^{3} ly^{2} (Q_{j}^{(i)} )^{2} )f_{j}^{(i)} } + A_{j}^{(i)} C_{55}^{(i)} ((ik + 0.25 \, ik^{3} ly^{2} )Q_{j}^{(i)} \hfill \\\qquad\qquad +\; 0.25 \, ik^{3} ly^{2} (Q_{j}^{(i)} )^{3} ) + A_{j}^{(i)} ike_{15}^{(i)} g_{j}^{(i)} )\exp (ik(x + Q_{j}^{(i)} z) - i\omega t). \hfill \\ \end{gathered}$$

(40)

$$\begin{gathered} \sigma_{zz}^{(i)} = \sum\limits_{j = 1}^{10} {A_{j}^{(i)} } (\left( { - ikC_{55}^{(i)} + 1/12ik^{3} l^{2} \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right)\left( {1 + (Q_{j}^{(i)} )^{2} } \right)} \right)f_{j}^{(i)}\\ - ik\left( {e_{15}^{(i)} t_{j}^{(i)} + q_{15}^{(i)} w_{j}^{(i)} - C_{15}^{(i)} Q_{j}^{(i)} } \right) \hfill \\ \qquad\qquad+ 1/12ik^{3} l^{2} \left( {C_{44}^{(i)} + C_{55}^{(i)} + C_{66}^{(i)} } \right)\left( {Q_{j}^{(i)} + (Q_{j}^{(i)} )^{3} } \right))\exp (ik(x + Q_{j}^{(i)} z) - i\omega t) \hfill \\ \end{gathered}$$

(41)

$$\mu_{zy}^{(i)} = \frac{1}{6}\sum\limits_{j = 1}^{10} {A_{j}^{(i)} \left( {C_{44}^{(i)} { + }C_{55}^{(i)} { + }C_{66}^{(i)} } \right)l^{2} ( - k^{2} f_{j}^{(i)} Q_{j}^{(i)} - k^{2} (Q_{j}^{(i)} )^{2} )} \exp (ik(x + Q_{j}^{(i)} z) - i\omega t).$$

(42)

$$\omega_{y}^{(i)} = \frac{1}{2}ik\sum\limits_{j = 1}^{10} {A_{j}^{(i)} (f_{j}^{(i)} + Q_{j}^{(i)} )} \exp (ik(x + Q_{j}^{(i)} z) - i\omega t).$$

(43)

$$D_{z}^{(i)} = ik\sum\limits_{j = 1}^{10} {A_{j}^{(i)} (e_{31}^{(i)} - e_{33}^{(i)} Q_{j}^{(i)} f_{j}^{(i)} { + }\varepsilon_{33}^{(i)} Q_{j}^{(i)} t_{j}^{(i)} { + }g_{33}^{(i)} Q_{j}^{(i)} w_{j}^{(i)} )} \exp (ik(x + Q_{j}^{(i)} z) - i\omega t)$$

(44)

$$B_{z}^{(i)} = ik\sum\limits_{j = 1}^{10} {A_{j}^{(i)} (q_{31}^{(i)} - q_{33}^{(i)} Q_{j}^{(i)} f_{j}^{(i)} { + }g_{33}^{(i)} Q_{j}^{(i)} t_{j}^{(i)} { + }\lambda_{33}^{(i)} Q_{j}^{(i)} w_{j}^{(i)} )} \exp (ik(x + Q_{j}^{(i)} z) - i\omega t)$$

(45)

$$\phi_{z}^{(i)} = - \sum\limits_{j = 1}^{10} {A_{j}^{(i)} t_{j}^{(i)} } \exp (ik(x + Q_{j}^{(i)} z) - i\omega t)$$

(46)

$$\psi_{z}^{(i)} = - \sum\limits_{j = 1}^{10} {A_{j}^{(i)} w_{j}^{(i)} } \exp (ik(x + Q_{j}^{(i)} z) - i\omega t)$$

(47)

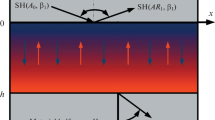
For TOBO, the boundary conditions can be written as:

$$\sigma_{zz}^{I} = 0,\sigma_{zx}^{I} = 0,\mu_{zy}^{I} = 0,D_{z}^{I} = 0,B_{z}^{I} = 0, z = \, 0$$

(48)

$$\sigma_{zz}^{II} = 0,\sigma_{zx}^{II} = 0,\mu_{zy}^{II} = 0,D_{z}^{II} = 0,B_{z}^{II} = 0. z = h$$

(49)

$$\begin{array}{*{20}c} \begin{gathered} u_{x}^{{{\text{i + }}1}} = u_{x}^{i} ,u_{z}^{{{\text{i + }}1}} = u_{z}^{{\text{i}}} ,\sigma_{zz}^{i + 1} = \sigma_{zz}^{i} ,\sigma_{zx}^{i + 1} = \sigma_{zx}^{i} ,\mu_{zy}^{{i{ + }1}} = \mu_{zy}^{i} ,\omega_{y}^{i + 1} = \omega_{y}^{i} , \hfill \\ D_{z}^{i + 1} = D_{z}^{i} ,\phi^{i + 1} = \phi^{i} ,B_{z}^{i + 1} = B_{z}^{i} ,\psi^{i + 1} = \psi^{i} . \hfill \\ \end{gathered} & {z = h_{i} ,i = {123} \ldots N - {1}} \\ \end{array}$$

(50)

The following equation can be obtained by substitution of Eqs. (39)–(47) into Eqs. (48)–(50):

$$\left[ {MM} \right]\{ A_{j}^{(i)} \}^{T} = \, 0 \, \left( {i = \, 1, \, 2 \ldots \, N,j = \, 1, \, 2 \ldots \, 8} \right).$$

(51)

where [MM] is a 10 N × 10 N matrix whose elements contain h, ω, k and material parameters. The dispersion relation can be obtained by solving |MM| = 0 numerically.