Homogenization approaches for the effective characteristics of fractional visco-piezoelastic fibrous composites

Abstract

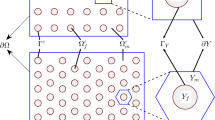
In this work, the effective coefficients for a two-phase visco-piezoelastic composite reinforced by cylindrical fibers embedded in a matrix are derived using the effective field and asymptotic homogenization approaches. The composite is characterized by an isotropic viscoelastic matrix reinforced by piezoelastic fibers, such that the fibers are oriented along the $x_3$-axis and their spatial distribution allows us to consider a hexagonal cell. Under these assumptions, closed formulas are obtained based on the Rabotnov’s fractional exponential kernel. Limit cases are reported as a validation of the model. Numerical implementation for the effective coefficients is carried out, and a comparison between both approaches is given. Electromechanical coupling coefficients for ultrasonic pulse-echo transducers in medical applications are computed.

Appendix A

Coefficients in Eqs. (38)–(46) are given as follows:

$$\begin{aligned} F_{33}^{\left( 1\right) }= & {} \left( 1-c\right) \left( K_0+\frac{4}{3} \mu _0\right) -c \left( \frac{a_3}{3\,b_2}-n\right) ,\nonumber \\ F_{33}^{\left( 2\right) }= & {} (1-c)\frac{4}{3} \mu _0\lambda +c\,\frac{\left( c_3-b_3\beta +a_3 \beta ^2\right) }{3\,b_2\left( \gamma _1-\beta \right) },\nonumber \\ F_{33}^{\left( 3\right) }= & {} c\,\frac{\left( c_3-b_3\ \gamma _1+a_3\ \gamma _1^2\right) }{3\ b_2\ \left( \beta -\gamma _1\right) }, \end{aligned}$$

(A1)

$$\begin{aligned} F_{11}^{\left( 1\right) }= & {} K_0+\frac{4}{3}\mu _0+\frac{a_7}{3\,b_2}-\frac{2\,c\,b_4\,b_5\,\mu _0^2}{a_6},\nonumber \\ F_{11}^{\left( 2\right) }= & {} \frac{4}{3}\mu _0 \lambda +\frac{c_7-b_7\,\beta +a_7\,\beta ^2}{3\,b_2 \left( \gamma _1-\beta \right) }-\frac{2\,c\,b_4\,b_5\, \mu _0\left( \gamma _2-\beta \right) \left( \gamma _3-\beta \right) \lambda }{a_6\left( x_1-\beta \right) \left( x_2-\beta \right) },\nonumber \\ F_{11}^{\left( 3\right) }= & {} \frac{c_7-b_7\,\gamma _1+a_7\,\gamma _1^2}{3\,b_2\left( \beta -\gamma _1\right) },\nonumber \\ F_{11}^{\left( 4\right) }= & {} -\frac{2\,c\,b_4\,b_5\,\mu _0\left( \gamma _2-x_1\right) \left( \gamma _3-x_1\right) \left( \lambda +\beta -x_1\right) }{a_6\left( \beta -x_1\right) \left( x_2-x_1\right) },\nonumber \\ F_{11}^{\left( 5\right) }= & {} -\frac{2\,c\,b_4\,b_5\,\mu _0\left( \gamma _2-x_2\right) \left( \gamma _3-x_2\right) \left( \lambda +\beta -x_2\right) }{a_6\left( \beta -x_2\right) \left( x_1-x_2\right) }, \end{aligned}$$

(A2)

$$\begin{aligned} F_{12}^{\left( 1\right) }= & {} K_0-\frac{2}{3}\mu _0+\frac{a_7}{3\,b_2}+\frac{2\,c\,b_4\,b_5\,\mu _0^2}{a_6},\nonumber \\ F_{12}^{\left( 2\right) }= & {} -\frac{2}{3}\mu _0 \lambda +\frac{c_7-b_7\,\beta +a_7\,\beta ^2}{3\,b_2\left( \gamma _1-\beta \right) } +\frac{2\,c\,b_4\,b_5\,\mu _0\left( \gamma _2-\beta \right) \left( \gamma _3-\beta \right) \lambda }{a_6\left( x_1-\beta \right) \left( x_2-\beta \right) },\nonumber \\ F_{12}^{\left( 3\right) }= & {} F_{11}^{\left( 3\right) }, F_{12}^{\left( 4\right) }={-F}_{11}^{\left( 4\right) }, F_{12}^{\left( 5\right) }={-F}_{11}^{\left( 5\right) }, \end{aligned}$$

(A3)

$$\begin{aligned} F_{66}^{\left( 1\right) }= & {} \mu _0\Big (1-\frac{2c\,b_4\,b_5\,\mu _0}{a_6}\Big ),\nonumber \\ F_{66}^{\left( 2\right) }= & {} -\frac{2c\,{b}_4\,b_5\,\mu _0\left( \gamma _2-\beta \right) \left( \gamma _3-\beta \right) \lambda }{a_6\left( x_1-\beta \right) \left( x_2-\beta \right) },\nonumber \\ F_{66}^{\left( 3\right) }= & {} F_{11}^{\left( 4\right) }, F_{66}^{\left( 4\right) }=F_{11}^{\left( 5\right) }, \end{aligned}$$

(A4)

$$\begin{aligned} F_{44}^{\left( 1\right) }= & {} \mu _0\Big (1-\frac{2\,c\, b_8}{b_9}\Big ), F_{44}^{\left( 2\right) }=\mu _0\,\lambda \left( 1-\frac{2\,c\left( c_8-b_8\,\beta \right) }{b_9\left( \gamma _4-\beta \right) }\right) ,\nonumber \\ F_{44}^{\left( 3\right) }= & {} -\frac{2\,c\,\mu _0\left( c_8-b_8\ \gamma _4\right) \left( \lambda +\beta -\gamma _4\right) }{b_9\left( \beta -\gamma _4\right) }, \end{aligned}$$

(A5)

$$\begin{aligned} E_{31}^{\left( 1\right) }= & {} \frac{c{\,b}_4\,e_{31}}{b_2},\quad E_{31}^{\left( 2\right) }=\frac{{c\,e}_{31} \left( c_4-b_4\,\gamma _1\right) }{b_2}, \end{aligned}$$

(A6)

$$\begin{aligned} E_{15}^{\left( 1\right) }= & {} \frac{4\,c\,\mu _0\,{e}_{15}\,\eta ^0}{b_9},\quad E_{15}^{\left( 2\right) }=\frac{4\,c\,{\mu }_0\,e_{15}\, \eta ^0\left( \lambda +\beta -\gamma _4\right) }{b_9} \end{aligned}$$

(A7)

$$\begin{aligned} E_{33}^{\left( 1\right) }= & {} \frac{b_{10}\,c}{b_2},\quad E_{33}^{\left( 2\right) }=\frac{c\left( c_{10}-b_{10}\,\gamma _1\right) }{b_2}, \end{aligned}$$

(A8)

$$\begin{aligned} D_{11}^{\left( 1\right) }= & {} \eta ^0-\frac{2\,b_{11}\,\eta ^0\,c}{b_9},\quad D_{11}^{\left( 2\right) }=-\frac{2\,\eta ^0\,c\left( c_{11}-b_{11}\,\gamma _4\right) }{b_2}, \end{aligned}$$

(A9)

$$\begin{aligned} D_{33}^{\left( 1\right) }= & {} \left( 1-c\right) \eta ^0-c\Big (-\eta _{33}+3\frac{\left( -1+c\right) }{b_2}e_{31}^2\Big ), \nonumber \\ D_{33}^{\left( 2\right) }= & {} \frac{3e_{31}^2\left( -\gamma _1+\beta \right) \left( -1+c\right) }{b_2}. \end{aligned}$$

(A10)

In Eqs. (A1)–(A10) are denoted

$$\begin{aligned} a= & {} 3\,K_0\,\mu _0\left( 1+c\right) +2\,\mu _0^2\left( 1+7\, c\right) +7\,\mu \,\mu _0\left( 1-c\right) ,\nonumber \\ b= & {} 3\,K_0\,\mu _0\left( 1+c\right) +\mu _0^2\left( 1+7\, c\right) +\mu \left( 3\,K_0+7\,\mu _0\right) \left( 1-c\right) , \end{aligned}$$

(A11)

$$\begin{aligned} a_3= & {} \left( 1-c\right) \left( -3 K_0+2\,\mu _0+3\,l\right) ^2, \nonumber \\ b_3= & {} 2\left( 1-c\right) \left( -3\,K_0+2\,\mu _0+3\,l\right) \left( 2\,\lambda \,\mu _0-3\,K_0\,\beta +2\,\beta \,\mu _0+3\,\beta \,l\right) ,\nonumber \\ c_3= & {} \left( 1-c\right) \left( 2\,\lambda \,\mu _0-3\,K_0\, \beta +2\,\beta \,\mu _0+3\,\beta \,l\right) ^2, \end{aligned}$$

(A12)

$$\begin{aligned} b_4= & {} 3\,K_0+4\,\mu _0,\quad c_4=4\,\lambda \,\mu _0+\beta \left( 3\,K_0+4\,\mu _0\right) , \end{aligned}$$

(A13)

$$\begin{aligned} b_5= & {} \mu _0-\mu ,\quad c_5=\lambda \,\mu _0+\beta \left( \mu _0-\mu \right) , \end{aligned}$$

(A14)

$$\begin{aligned} a_6= & {} \mu _0\,\left( 3\,K_0+\mu _0\right) +\left( \mu -c\left( \mu -\mu _0\right) \right) \left( 3\,K_0+7\,\mu _0\right) ,\nonumber \\ b_6= & {} a\,\lambda +2\,b\,\beta ,\quad c_6=\mu _0^2\,\left( 1+7\,c\right) \,\lambda ^2+a\,\lambda \,\beta +b\,\beta ^2, \end{aligned}$$

(A15)

$$\begin{aligned} a_7= & {} -c\,\left( 3\,K_0+4\mu _0\right) \left( 3\,K_0+\mu _0-3k\right) ,\nonumber \\ b_7= & {} -c\,\left( 3\,K_0+4\mu _0\right) \left( \lambda \,\mu _0+3\,K_0\,\beta +\beta \,\mu _0-3\,\beta \,k\right) \nonumber \\{} & {} \quad -c\,\left( 3\,K_0+\mu _0-3k\right) \left( 4\,\lambda \,\mu _0+3\,K_0\,\beta +4\,\beta \,\mu _0\right) ,\nonumber \\ c_7= & {} 4\,\lambda \,\mu _0+\beta \left( 3\,K_0+4\mu _0\right) \end{aligned}$$

(A16)

$$\begin{aligned} b_8= & {} \left( \left( \mu _0-\mu \right) \left( \eta ^0-\eta _{11}\right) +e_{15}^2\right) c+\left( \mu _0- \mu \right) \left( \eta ^0+\eta _{11}\right) -e_{15}^2,\nonumber \\ c_8= & {} \left[ \left( \left( \lambda +\beta \right) \mu _0-\beta \,\mu \right) \left( \eta ^0-\eta _{11}\right) +\beta e_{15}^2\right] c\nonumber \\{} & {} +\quad \left( \left( \lambda +\beta \right) \mu _0-\beta \ \mu \right) \left( \eta ^0+\eta _{11}\right) -\beta e_{15}^2, \end{aligned}$$

(A17)

$$\begin{aligned} b_9= & {} \left( \left( \mu _0-\mu \right) \left( \eta ^0-\eta _{11}\right) +e_{15}^2\right) c^2+2 \left( \eta ^0 \mu _0-\eta _{11}\mu -e_{15}^2\right) c\nonumber \\{} & {} +\quad \left( \mu _0+\mu \right) \left( \eta ^0+\eta _{11}\right) +e_{15}^2,\nonumber \\ c_9= & {} \left( \left( \left( \lambda +\beta \right) \mu _0-\beta \mu \right) \left( \eta ^0-\eta _{11}\right) +\beta e_{15}^2\right) c^2\nonumber \\{} & {} +\quad 2\left( \left( \lambda +\beta \right) \mu _0\,\eta ^0-\beta \left( \mu \,\eta _{11}+e_{15}^2\right) \right) c\nonumber \\{} & {} +\quad \left( \left( \lambda +\beta \right) \mu _0+\beta \mu \right) \left( \eta ^0+\eta _{11}\right) +\beta e_{15}^2, \end{aligned}$$

(A18)

$$\begin{aligned} b_{10}= & {} \left[ 3\,K_0\left( e_{33}-e_{31}\right) +\mu _0\left( e_{33}+2 e_{31}\right) -3\,e_{33}\,k+3\,e_{31} l\right] c\nonumber \\{} & {} +\quad 3\,K_0\,e_{31}+ \mu _0\left( 3\,e_{33}-2\,e_{31}\right) +3\,e_{33}\,k-3\,e_{31}\,l,\nonumber \\ c_{10}= & {} 3\,K_0\,\beta \left( e_{33}-e_{31}\right) c+\lambda \,\mu _0\left( e_{33}+2e_{31}\right) c +\beta \,\mu _0\left( e_{33}+2e_{31}\right) c\nonumber \\{} & {} \quad -3\,\beta \left( e_{33}\,k-e_{31}\,l\right) c+ 3\,K_0\,\beta \,e_{31}+\lambda \,\mu _0\left( 3\,e_{33}-2\,e_{31}\right) \nonumber \\{} & {} +\quad \beta \mu _0\left( 3e_{33}-2e_{31}\right) +3\,\beta \left( e_{33}\,k-e_{31}\,l\right) , \end{aligned}$$

(A19)

$$\begin{aligned} b_{11}= & {} \left( \left( \mu _0-\mu \right) \left( \eta ^0-\eta _{11}\right) +e_{15}^2\right) c +\left( \mu _0+\mu \right) \left( \eta ^0-\eta _{11}\right) -e_{15}^2,\nonumber \\ c_{11}= & {} \left( \left( \left( \lambda +\beta \right) \mu _0-\beta \,\mu \right) \left( \eta ^0-\eta _{11}\right) +\beta \,e_{15}^2\right) c\nonumber \\{} & {} +\quad \left( \left( \lambda +\beta \right) \mu _0+\beta \,\mu \right) \left( \eta ^0-\eta _{11}\right) -\beta \,e_{15}^2, \end{aligned}$$

(A20)

and

$$\begin{aligned} \gamma _1=\frac{c_2}{b_2},\quad \gamma _2=\frac{c_4}{b_4},\quad \gamma _3=\frac{c_5}{b_5},\quad \gamma _4=\frac{c_9}{b_9},\quad \begin{matrix}x_{1,2}=\frac{b_6\mp \sqrt{{b_6}^2-4\,a_6\,c_6}}{2\,a_6}.\ \ \ \\ \end{matrix} \end{aligned}$$

(A21)