Skip to main content
SpringerLink
Log in

Non-continuous curves phenomenon in stressed magneto-electro-elastic plates with negative magnetic permeability

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

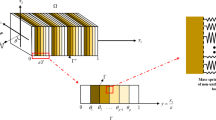
Abstract

Several numerical methods allow the prediction of guided dispersion curves. Due to some drawbacks of such methods, e.g., the challenge of divergence, which heavily depends on partial waves, the Legendre polynomial (LP) approach is applied to the magneto-electro-elastic (MEE) alloy. This approach is based on polynomial approximation, which replaces the problem of solving a transcendental dispersion equation with an eigenvalue problem. To apply the LP method for MEE alloy, this paper presents a numerical solution to calculate the waveguide dispersion curves. The roots prediction overlaps with the convergence character of this polynomial approach, leading to accurate phase velocities of guided waves. The results indicate the existence of the obvious physical phenomenon: non-continuous furcation in guided dispersion curves. It is thus now possible to confirm this physical phenomenon, differently reported in available data. However, with an incremental change of structure from bilayer plate to alloy, non-continuous furcation for SH modes appears more obviously, which was not discussed before. With such a phenomenon, the uniaxial residual stresses can affect these new trajectories, where these stress states could play a useful role in controlling the dispersion curves. Thereby, a comparison of the guided dispersion curves in stressed and unstressed 40%BaTiO3-60%CoFe2O4 alloy answers this need and sets forth to explore the effects of initial stress. Results show that the effects of initial stresses on phase velocity change are significant and give useful information, although their influence on the non-continuous furcation phenomenon is neglected.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

References

  1. Singh, S., Singh, A.K.: Anti-plane surface and interfacial waves influenced by layer reinforcement in piezo-electro-magnetic structures with surface energy. Eur. Phys. J. Plus 136, 312 (2021)

    Article  Google Scholar 

  2. Singh, A.K., Singh, S., Kumari, R., Ray, A.: Impact of point source and mass loading sensitivity on the propagation of an SH wave in an imperfectly bonded FGPPM layered structure. Acta Mech. 231, 2603–2627 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  3. Singh, A.K., Mahto, S., Guha, S.: Analysis of plane wave reflection and transmission phenomenon at the interface of two distinct micro-mechanically modeled rotating initially stressed piezomagnetic fiber-reinforced half-spaces. Mech. Adv. Mater. Struct. 1–17 (2021)

  4. Cao, X., Shi, J., Jin, F.: Lamb wave propagation in the functionally graded piezoelectric–piezomagnetic material plate. Acta Mech. 223, 1081–1091 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  5. Zhou, Y.Y., Lü, C.F., Chen, W.Q.: Bulk wave propagation in layered piezomagnetic/piezoelectric plates with initial stresses or interface imperfections. Compos. Struct. 94, 2736–2745 (2012)

    Article  Google Scholar 

  6. Xiao, D., Han, Q., Liu, Y., Li, C.: Guided wave propagation in an infinite functionally graded magneto-electro-elastic plate by the Chebyshev spectral element method. Compos. Struct. 153, 704–711 (2016)

    Article  Google Scholar 

  7. Pan, E.: Three-dimensional Green’s functions in anisotropic magneto-electro-elastic bimaterials. Z. Angew. Math. Phys. 53, 815–838 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  8. Pan, E., Chen, W.Q.: Static Green’s functions in anisotropic media. Cambridge University Press, New York (2015)

    Book  MATH  Google Scholar 

  9. Imano, K.: experimental study on the mode conversion of Lamb waves in a metal plate of stepped thickness using optical detection. Int. J. Soc. Mater. Eng. Resour. 17, 201–204 (2010)

    Article  Google Scholar 

  10. Othmani, C., Zhang, H.: Lamb wave propagation in anisotropic multilayered piezoelectric laminates made of PVDF-θ° with initial stresses. Compos. Struct. 240, 112085 (2020)

    Article  Google Scholar 

  11. Othmani, C., Njeh, A., Ben Ghozlen, M.H.: Influence of anisotropic fiber reinforced composite media properties on fundamental guided wave mode behavior: a Legendre polynomial approach. Aerosp. Sci. Technol. 78, 377–386 (2018)

    Article  Google Scholar 

  12. Pal, M.K., Singh, A.K.: Analysis of reflection and transmission phenomenon at distinct bonding interfaces in a rotating pre-stressed functionally graded piezoelectric-orthotropic structure. Appl. Math. Comput. 409, 126398 (2021)

    MathSciNet  MATH  Google Scholar 

  13. Othmani, C., Zhang, H., Lu, C.F., Takali, F.: Effects of initial stresses on the electromechanical coupling coefficient of SH wave propagation in multilayered PZT-5H structures. Eur. Phys. J. Plus 134, 551 (2019)

    Article  Google Scholar 

  14. Othmani, C., Labiadh, L., Lu, C.F., Kamali, A.R., Takali, F.: Influence of a piezoelectric ZnO intermediate layer on Rayleigh waves propagating in Sc43%AlN57%/ZnO/diamond hetero-structures subjected to uniaxial stress. Eur. Phys. J. Plus 135, 898 (2020)

    Article  Google Scholar 

  15. Kundu, S., Gupta, S., Manna, S.: Propagation of G-type seismic waves in heterogeneous layerlying over an initially stressed heterogeneous half-space. Appl. Math. Comput. 234, 1–12 (2014)

    MathSciNet  MATH  Google Scholar 

  16. Calas, H., Otero, J.A., Rodriguez-Ramos, R., Monsivais, G., Stern, C.: Dispersion relations for SH wave in magneto-electro-elastic heterostructures. Int. J. Solids Struct. 45, 5356–5367 (2008)

    Article  MATH  Google Scholar 

  17. Lefebvre, J.E., Zhang, V., Gazalet, J., Gryba, T.: Conceptual advantages and limitations of the Laguerre polynomial approach to analyze surface acoustic waves in semi-infinite substrates and multilayered structures. J. Appl. Phys. 83, 28–34 (1998)

    Article  Google Scholar 

  18. Yu, J.G., Ding, J., Ma, Z.: On dispersion relations of waves in multilayered magneto-electro-elastic plates. Appl. Math. Model. 36, 5780–5791 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  19. Yu, J.G., Lefebvre, J.E., Guo, Y.Q.: Free-ultrasonic waves in multilayered piezoelectric plates: an improvement of the Legendre polynomial approach for multilayered structures with very dissimilar materials. Compos. B Eng. 51, 260–269 (2013)

    Article  Google Scholar 

  20. Morse, M.P., Feshbach, H.: Methods of Theoretical Physics. Part I McGraw-Hill, New York (1953)

    MATH  Google Scholar 

  21. Guha, S., Singh, A.K.: Effects of initial stresses on reflection phenomenon of plane waves at the free surface of a rotating piezothermoelastic fiber-reinforced composite half-space. Int. J. Mech. Sci. 181, 105766 (2020)

    Article  Google Scholar 

  22. Guha, S., Singh, A.K.: Plane wave reflection/transmission in imperfectly bonded initially stressed rotating piezothermoelastic fiber-reinforced composite half-spaces. Eur. J. Mech. A/Solids 88, 104242 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  23. Guha, S., Singh, A.K.: Influence of varying fiber volume fractions on plane waves reflecting from the stress-free/rigid surface of a piezoelectric fiber-reinforced composite half-space. Mech. Adv. Mater. Struct. 1–15 (2021)

  24. Li, Ch., Han, Q., Wang, Z., Liu, Y.: Propagation and attenuation of guided waves in stressed viscoelastic waveguides. Math. Mech. Solids 24, 3957–3975 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  25. Sottos, N.R., Berfield, T., Ong, R., Payne, D.A.: Residual stress effects on ferroelectric thin film patterning, properties and performance. In: XXI ICTAM, Poland (2004)

  26. Tolstoy, I., Usdin, E.: Wave propagation in elastic plates: low and high mode dispersion. J. Acoust. Soc. Am. 29, 37–42 (1957)

    Article  Google Scholar 

  27. Othmani, C., Zhang, H., Kamali, A.R., Lü, C.F., Takali, F., Köhler, B.: Propagating, evanescent and ZGV Lamb modes in high-performance anisotropic Cu–Al–Ni alloy plates. Arch. Appl. Mech. 92, 21–43 (2021)

    Article  Google Scholar 

  28. Singh, A.K., Rajput, P., Guha, S., Singh, S.: Propagation characteristics of love-type wave at the electro mechanical imperfect interface of a piezoelectric fiber-reinforced composite layer overlying a piezoelectric half-space. Eur. J. Mech.-A/Solids 93, 104527 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  29. Maity, M., Kundu, S., Kumhar, R., Gupta, S.: An electromechanical based model for Love-type waves in anisotropic-porous-piezoelectric composite structure with interfacial imperfections. Appl. Math. Comput. 418, 126783 (2022)

    MathSciNet  MATH  Google Scholar 

  30. Li, Ch., Han, Q., Wang, Z., Wu, X.: Analysis of wave propagation in functionally graded piezoelectric composite plates reinforced with graphene platelets. Appl. Math. Model. 81, 487–505 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  31. Li, Ch., Han, Q.: Semi-analytical wave characteristics analysis of graphene-reinforced piezoelectric polymer nanocomposite cylindrical shells. Int. J. Mech. Sci. 186, 105890 (2020)

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by Tunisian Ministry of Higher Education, Scientific Research (LPM laboratory).

Author information

Authors and Affiliations

  1. Chair of Acoustics and Haptic Engineering, Dresden University of Technology, 01062, Dresden, Germany

    Cherif Othmani

  2. Department of Civil Engineering, Zhejiang University, Hangzhou, 310058, China

    Cherif Othmani

  3. Laboratory of Physics of Materials, Faculty of Sciences of Sfax, University of Sfax, PB 815, 3018, Sfax, Tunisia

    Samiha Karmi, Farid Takali & Anouar Njeh

  4. National School of Engineers of Sfax (ENIS), Street Soukra Km 4, 3018, Sfax, Tunisia

    Farid Takali

Authors
  1. Samiha Karmi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Cherif Othmani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Farid Takali
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Anouar Njeh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Cherif Othmani.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix: The elements of \({\varvec{A}}^{{{\varvec{jm}}}}\), \(\aleph^{{{\varvec{jm}}}}\) and \({\varvec{M}}^{{{\varvec{jm}}}}\)

Appendix: The elements of \({\varvec{A}}^{{{\varvec{jm}}}}\), \(\aleph^{{{\varvec{jm}}}}\) and \({\varvec{M}}^{{{\varvec{jm}}}}\)

The elements of \({\varvec{A}}^{{{\varvec{jm}}}}\), \(\aleph^{{{\varvec{jm}}}}\) and \({\varvec{M}}^{{{\varvec{jm}}}}\) in Eq. (22) are expressed as:

$$M^{jm} = \left[ {\vartheta_{1} \left( {m,j,0} \right) \times \rho \times \pi_{0 - kH} } \right]$$
(A.1)
$$A_{11}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \left[ {C_{11} + \sigma_{0}^{X} } \right] \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times C_{55} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times C_{55} \times \pi_{0 - kH} } \right)} \right]$$
(A.2)
$$A_{13}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {C_{55} + C_{13} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times C_{55} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.3)
$$A_{14}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {e_{15} + e_{31} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times e_{15} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.4)
$$A_{15}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {q_{15} + q_{31} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times q_{15} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.5)
$$A_{22}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \left[ {C_{66} + \sigma_{0}^{X} } \right] \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times C_{44} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times C_{44} \times \pi_{0 - kH} } \right)} \right]$$
(A.6)
$$A_{24}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times e_{16} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times e_{34} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times e_{34} \times \pi_{0 - kH} } \right)} \right]$$
(A.7)
$$A_{25}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times q_{16} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times q_{34} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times q_{34} \times \pi_{0 - kH} } \right)} \right]$$
(A.8)
$$A_{31}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {C_{55} + C_{31} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times C_{31} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.9)
$$A_{33}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \left[ {C_{55} + \sigma_{0}^{X} } \right] \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times C_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times C_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.10)
$$A_{34}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times e_{15} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times e_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times e_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.11)
$$A_{35}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times q_{15} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times q_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times q_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.12)
$$A_{41}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {e_{31} + e_{15} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times e_{31} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.13)
$$A_{42}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times e_{16} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times e_{34} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times e_{34} \times \pi_{0 - kH} } \right)} \right]$$
(A.14)
$$A_{43}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times e_{15} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times e_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times e_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.15)
$$A_{44}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \varepsilon_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times \varepsilon_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times \varepsilon_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A16)
$$AA_{44}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \varepsilon_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times \varepsilon_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times \varepsilon_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.17)
$$A_{45}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times g_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times g_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times g_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.18)
$$AA_{45}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times g_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times g_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times g_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.19)
$$A_{51}^{jm} = \left[ {\begin{array}{*{20}c} {\left( {i\vartheta_{1} \left( {m,j,1} \right) \times \left[ {q_{31} + q_{15} } \right] \times \pi_{0 - kH} } \right)} \\ { + \left( {i\vartheta_{2} \left( {m,j,0} \right) \times q_{31} \times \pi_{0 - kH} } \right)} \\ \end{array} } \right]$$
(A.20)
$$A_{52}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times q_{16} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times q_{34} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times q_{34} \times \pi_{0 - kH} } \right)} \right]$$
(A.21)
$$A_{53}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times q_{15} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times q_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times q_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.22)
$$A_{54}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times g_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times g_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times g_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.23)
$$AA_{54}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times g_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times g_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times g_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.24)
$$A_{55}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \mu_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times \mu_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times \mu_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.25)
$$AA_{55}^{jm} = \left[ {\left( { - \vartheta_{1} \left( {m,j,0} \right) \times \mu_{11} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{1} \left( {m,j,2} \right) \times \mu_{33} \times \pi_{0 - kH} } \right) + \left( {\vartheta_{2} \left( {m,j,1} \right) \times \mu_{33} \times \pi_{0 - kH} } \right)} \right]$$
(A.26)
$$\vartheta_{1} \left( {m,j,n} \right) = \mathop \int \limits_{0}^{kH} Q_{j}^{*} \left( \beta \right)\frac{{\partial^{n} Q_{m} \left( \beta \right)}}{\partial \beta }d\beta$$
(A.27)
$$\vartheta_{2} \left( {m,j,n} \right) = \mathop \int \limits_{0}^{kH} Q_{j}^{*} \left( \beta \right)\left( {\delta \left( {\beta = 0} \right) - \delta \left( {\beta = kH} \right)} \right)\frac{{\partial^{n} Q_{m} \left( \beta \right)}}{\partial \beta }d\beta$$
(A.28)

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Karmi, S., Othmani, C., Takali, F. et al. Non-continuous curves phenomenon in stressed magneto-electro-elastic plates with negative magnetic permeability. Acta Mech 234, 1599–1618 (2023). https://doi.org/10.1007/s00707-022-03460-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03460-8

Search

Navigation