Skip to main content
SpringerLink
Log in

On the role of mass distribution in free vibration of Hencky beam models: discrete and nonlocal continuous approaches

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

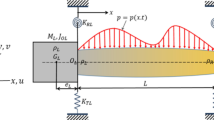
In this paper, we investigate the role of mass distribution on the vibration frequencies of various discrete Hencky-type beam models. These models are composed of rigid beam elements connected by concentrated rotational springs, and they all asymptotically converge towards the continuous Euler–Bernoulli beam models, for an infinite number of elements. However, the order of convergence and the upper bound or lower bound status of each discrete beam model as compared to the continuous counterpart depends on how the mass is assumed to be distributed along the discrete elements. Three mass distributions assumptions are considered herein: Hencky model with lumped masses at each joint, Hencky model with lumped masses at the middle of the rigid segment, and Hencky model with distributed masses along each rigid element. Exact eigenfrequency formulas are presented for the various Hencky beam models with simply supported ends. A nonlocal continuous approach for each model is also developed. A strong dependence with respect to the length scale effect is observed for each lattice beam model. Stiffening or softening length scale phenomena are shown to be controlled by the mass microstructure, namely concentrated or distributed mass inertia. The wave dispersive behaviour of each Hencky model is also discussed for each mass distribution (concentrated and distributed mass properties). The length scale of the associated nonlocal beam model is also fitted with respect to the exact dispersive curves of Hencky beams.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Hencky, H.: Über die angenaherte Lösung von Stabilitatsproblemen im Raum mittels der elastischen Gelenkkette. Der Eisenbau 11, 437–452 (1920)

    Google Scholar 

  2. Silverman, I.K.: Discussion on the paper of “Salvadori M.G., Numerical computation of buckling loads by finite differences. Trans. ASCE 116, 590–636 (1951)

    Google Scholar 

  3. Wang, C.M., Gao, R.P., Zhang, H., Challamel, N.: Treatment of elastically restrained ends for beam buckling in finite difference, microstructured and nonlocal beam models. Acta Mech. 226, 419–436 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Wang, C.M., Zhang, H., Challamel, N., Pan, W.: Hencky-Bar-Chain/Net for Structural Analysis. World Scientific, Singapore (2020).

  5. Wang, C.T.: Discussion on the paper of “Salvadori M.G., Numerical computation of buckling loads by finite differences". Trans. ASCE 116, 629–631 (1951)

    Google Scholar 

  6. Wang, C.T.: Applied Elasticity. McGraw-Hill, New-York (1953)

    MATH  Google Scholar 

  7. Livesley, R.K.: The equivalence of continuous and discrete mass distributions in certain vibration problems. Q. J. Mech. Appl. Math. 8(3), 353–360 (1955)

    Article  MathSciNet  MATH  Google Scholar 

  8. Leckie, F.A., Lindberg, G.M.: The effect of lumped parameters on beam frequencies. Aeronaut. Quart. 14, 224–240 (1963)

    Article  Google Scholar 

  9. Archer, J.S.: Consistent mass matrix for distributed mass systems. J. Struct. Div. ASCE 89(ST4), 161–178 (1963)

    Article  Google Scholar 

  10. Challamel, N., Picandet, V., Elishakoff, I., Wang, C.M., Collet, B., Michelitsch, T.: On nonlocal computation of eigenfrequencies of beams using finite difference and finite element methods. Int. J. Struct. Stab. Dyn., Special Issue in Honor of Prof. J.N. Reddy’s 70th birthday, 15, 7, 1540008 (2015).

  11. Banakh, L.Y., Kempner, M.L.: Vibrations of Mechanical Systems with Regular Structures. Springer, Berlin (2010)

    Book  MATH  Google Scholar 

  12. Chan, H.C., Cai, C.W., Cheung, Y.K.: Convergence studies of dynamical analysis by using the finite element method with lumped mass matrix. J. Sound Vib. 165(2), 193–207 (1993)

    Article  MATH  Google Scholar 

  13. Tong, P., Pian, T.H.H., Bucciarelli, L.L.: Mode shapes and frequencies by finite element method using consistent and lumped masses. Comput. Struct. 1, 623–638 (1971)

    Article  MathSciNet  Google Scholar 

  14. Belytschko, T., Mindle, W.L.: Flexural wave propagation behaviour of lumped mass approximation. Comput. Struct. 12, 805–812 (1980)

    Article  MATH  Google Scholar 

  15. Xie, Y.M., Steven, G.P.: Explicit formulas for correcting finite-element predictions of natural frequencies. Commun. Num. Meth. Eng. 9, 671–680 (1993)

    Article  MATH  Google Scholar 

  16. Andrianov, I.V., Awrejcewicz, J., Ivankov, O.: On an elastic dissipation model as continuous approximation for discrete media. Math. Probl. Eng. 27373, 1–8 (2006)

    MathSciNet  MATH  Google Scholar 

  17. Challamel, N., Wang, C.M., Elishakoff, I.: Discrete systems behave as nonlocal structural elements: bending, buckling and vibration analysis. Eur. J. Mech. A/Solids 44, 125–135 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  18. Zhang, Z., Wang, C.M., Challamel, N., Elishakoff, I.: Obtaining Eringen’s length scale coefficient for vibrating nonlocal beams via continualization method. J. Sound Vib. 333, 4977–4990 (2014)

    Article  Google Scholar 

  19. Chen, F.Y.: On modeling and direct solution of certain free vibration systems. J. Sound Vib. 14(1), 57–79 (1971)

    Article  MATH  Google Scholar 

  20. Deng, B., Zhang, Y., He, Q., Tournat, V., Wang, P., Bertoldi, K.: Propagation of elastic solitons in chains of pre-deformed beams. New J. Phys. 21, 073008 (2019)

    Article  Google Scholar 

  21. Bacigalupo, A., Gambarotta, L.: A dynamic high-frequency consistent continualization of beam-lattice materials. Compos. Struct. 272, 114146 (2021)

    Article  Google Scholar 

  22. Gomez-Silva, F., Zaera, R.: Analysis of low order non-standard continualization methods for enhanced prediction of the dispersive behaviour of a beam lattice. Int. J. Mech. Sci. 196, 106296 (2021)

    Article  Google Scholar 

  23. Turco, E., Barchiesi, E.: Kinematically triggered nonlinear vibrations of Hencky-type pantographic sheets. Math. Mech. Complex Syst. 9(3), 311–335 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  24. Duncan, W.J.: A critical examination of the representation of massive and elastic bodies by systems of rigid masses elastically connected. Quart. J. Mech. Appl. Math. 5, 97–108 (1952)

    Article  MathSciNet  MATH  Google Scholar 

  25. Wang, C.Y.: Free vibration of a linked rod. J. Sound Vib. 274, 455–459 (2004)

    Article  Google Scholar 

  26. Wang, C.Y., Zhang, H., Wang, C.M.: Vibration of a segmented rod. Int. J. Struct. Stab. Dyn. 2071011, 1–10 (2020)

    MathSciNet  Google Scholar 

  27. Challamel, N., Wang, C.M., Elishakoff, I.: Nonlocal or gradient elasticity macroscopic models: a question of concentrated or distributed microstructure. Mech. Res. Commun. 71, 25–31 (2016)

    Article  Google Scholar 

  28. De Domenico, D., Askes, H.: Stress gradient, strain gradient and inertia gradient beam theories for the simulation of flexural wave dispersion in carbon nanotubes. Compos. B 153, 285–294 (2018)

    Article  Google Scholar 

  29. Cauchy, A. : Sur les différences finies et les intégrales aux différences des fonctions entières d’une on de plusieurs variables. Exercices de mathématiques, pp. 155–159 (1828).

  30. Salvadori, M.G.: Numerical computation of buckling loads by finite differences. Trans. ASCE, 116, 590–624 (1951) (590–636 with the discussion).

  31. Andrianov, I.V., Awrejcewicz, J., Weichert, D.: Improved continuous models for discrete media. Math. Probl. Eng. 986242, 1–35 (2010)

    Article  MATH  Google Scholar 

  32. Andrianov, I.V., Awrejcewicz, J., Danishevskyy, V.: Linear and Nonlinear Waves in Microstructured Solids: Homogenization and Asymptotic Approaches. CRC Press, Boca Raton (2021)

    Book  Google Scholar 

  33. Eringen, A.C.: On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J. Appl. Phys. 54, 4703–4710 (1983)

    Article  Google Scholar 

  34. Reddy, J.N.: Nonlocal theories for bending, buckling and vibration of beams. Int. J. Eng. Sci. 45(2–8), 288–307 (2007)

    Article  MATH  Google Scholar 

  35. Papargyri-Beskou, S., Polyzos, D., Beskos, D.E.: Dynamic analysis of gradient elastic flexural beams. Struct. Eng. Mech. 15(6), 705–716 (2003)

    Article  MATH  Google Scholar 

  36. Born, M., von Kármán, T.: On fluctuations in spatial grids. Physikalische Zeitschrift 13, 297–309 (1912)

    MATH  Google Scholar 

  37. Gopalakrishnan, S., Narendar, S.: Wave Propagation in Nanostructures: Nonlocal Continuum Mechanics Formulations. Springer, Berlin (2013)

    Book  Google Scholar 

  38. Gopalakrishnan, S.: Elastic Wave Propagation in Structures and Materials. CRC Press, Boca Raton (2023)

    Google Scholar 

  39. Challamel, N., Atanackovic, T., Zhang, Y.P., Wang, C.M.: A fractional nonlocal elastic model for lattice wave analysis. Mech. Res. Commun. 126(103999), 1–9 (2022)

    Google Scholar 

  40. Kunin, I.A.: Elastic Media with Microstructure. Springer, Berlin (1983)

    Book  MATH  Google Scholar 

  41. Eringen, A.C.: Nonlocal Continuum Field Theories. Springer, New-York (2002)

    MATH  Google Scholar 

  42. Lazar, M., Maugin, G.A., Aifantis, E.C.: On a theory of nonlocal elasticity of bi-Helmholtz type and some applications. Int. J. Solids Struct. 43, 1404–1421 (2006)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. IRDL, UBS, Lorient, Centre de Recherche, Université de Bretagne Sud, Rue de Saint Maudé – BP 92116, 92116, Lorient Cedex, France

    Noël Challamel

  2. School of Mechatronical Engineering, Beijing Institute of Technology, Beijing, 100081, People’s Republic of China

    Hong Zhang

  3. School of Civil Engineering, University of Queensland, St Lucia, QLD, 4072, Australia

    C. M. Wang

Authors
  1. Noël Challamel
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hong Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. C. M. Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Noël Challamel.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Challamel, N., Zhang, H. & Wang, C.M. On the role of mass distribution in free vibration of Hencky beam models: discrete and nonlocal continuous approaches. Acta Mech 234, 807–823 (2023). https://doi.org/10.1007/s00707-022-03419-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03419-9

Search

Navigation