Analysis of Volterra dislocation in half-planes incorporating surface effects

Abstract

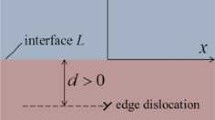
An isotropic elastic half-plane weakened by a Volterra dislocation, screw/edge is analyzed. The linear Gurtin–Murdoch surface elasticity theory is utilized to incorporate the surface effects. Therefore, the analysis is also valid for dislocations situated at a nanoscale distance from the boundary. The governing equations are derived in terms of displacement components. These equations are solved by means of the integral transform method. The resultant stress fields exhibit Cauchy as well as hyper-singular terms in the vicinity of dislocations. As the distance from dislocation to half-plane boundary increases, stress components tend to those of classical elasticity theory. Stress contours in the vicinity of screw and edge dislocations and plots of forces acting per unit length of dislocations in a thin elastic half-plane made up of glass substrate reinforced by an iron film are drawn. Stress contours reveal that surface effects magnify the stress field. Moreover, surface effects drastically change the behavior of force on a dislocation which is situated at a nanoscale distance from the stress-free half-plane boundary.

Appendix

The coefficients in Eq. (29) are

$$ \begin{aligned} A_{1} \left( \omega \right) & = - \frac{\omega }{\Lambda }\left( {4\left[ {\sinh \left( {2\omega h} \right) + 2\omega h} \right] + 2\left( {\kappa + 1} \right)\alpha_{2} \omega \cosh^{2} \left( {\omega h} \right)} \right. \\ & \quad \left. { + \alpha_{1} \omega \left\{ {2\left( {\kappa + 1} \right)\sinh^{2} \left( {\omega h} \right) + \alpha_{2} \omega \left[ {\kappa \sinh \left( {2\omega h} \right) - 2\omega h} \right]} \right\}} \right), \\ A_{2} \left( \omega \right) & = \frac{2}{\Lambda }\left\{ {\omega^{2} h\left[ {\alpha_{1} \left( {\alpha_{2} h\omega^{2} + \kappa + 1} \right) - \left( {\kappa + 1} \right)\alpha_{2} - 4h} \right] + \kappa^{2} - 1} \right\}, \\ A_{3} \left( \omega \right) & = - \frac{{2\omega^{2} }}{\Lambda }\left( {\alpha_{1} \alpha_{2} \omega^{2} - 4} \right), \\ A_{4} \left( \omega \right) & = - \frac{\omega }{\Lambda }\left\{ {4\left( {\sinh \left( {2\omega h} \right) - 2\omega h} \right) + 2\left( {\kappa + 1} \right)\alpha_{2} \omega \sinh^{2} \left( {\omega h} \right)} \right. \\ & \quad \left. { + \alpha_{1} \omega \left[ {2\left( {\kappa + 1} \right)\cosh^{2} \left( {\omega h} \right) + \alpha_{2} \omega \left( {\kappa \sinh \left( {2\omega h} \right) + 2\omega h} \right)} \right]} \right\}, \\ B_{1} \left( \omega \right) & = \frac{1}{\Delta }\left[ {\omega \left( {\alpha_{2} \omega + 2} \right)A_{2} + \left( {\kappa \alpha_{2} \omega + \kappa + 1} \right)\left( {A_{4} - 1} \right)} \right], \\ B_{2} \left( \omega \right) & = \frac{1}{\Delta }\left[ {\omega \left( {\alpha_{1} \omega + 2} \right)A_{2} + \left( {\kappa - 1} \right)\left( {A_{4} - 1} \right)} \right], \\ B_{3} \left( \omega \right) & = - \frac{1}{\Delta }\left[ {\omega \left( {\alpha_{2} \omega + 2} \right)A_{1} + \left( {\kappa \alpha_{2} \omega + \kappa + 1} \right)A_{3} - \omega \left( {\alpha_{2} \omega + 2} \right)} \right], \\ B_{4} \left( \omega \right) & = - \frac{1}{\Delta }\left[ {\omega \left( {\alpha_{1} \omega + 2} \right)\left( {A_{1} - 1} \right) + \left( {\kappa - 1} \right)A_{3} } \right], \\ B_{5} \left( \omega \right) & = \frac{1}{\Delta }\left\{ {\left[ {\omega \left( {\alpha_{2} \omega + 2} \right) - \left( {\kappa + 1} \right)A_{3} } \right],A_{2} + \left[ {\left( {\kappa + 1} \right)A_{1} + \kappa \alpha_{2} \omega } \right] \left( {A_{4} - 1} \right)} \right\}, \\ B_{6} \left( \omega \right) & = - \frac{1}{\Delta }\left\{ {\alpha_{2} \omega^{2} \left( {A_{1} A_{4} - A_{2} A_{3} } \right) + 2\omega \left( {A_{1} - 1} \right) + \left[ {\kappa \alpha_{2} \omega + \left( {\kappa + 1} \right)} \right] A_{3} - \alpha_{2} \omega^{2} A_{4} } \right\}, \\ B_{7} \left( \omega \right) & = \frac{1}{\Delta }\left[ {\left( {2\omega A_{2} - \kappa + 1} \right)A_{3} - \omega \left( {A_{1} - 1} \right)\left( {2A_{4} + \alpha_{1} \omega } \right)} \right] \\ \end{aligned} $$

(A.1)

where

$$ \begin{aligned} \Lambda \left( \omega \right) & = \omega \sinh \left( {2\omega h} \right)\left\{ {4\left[ {\cosh \left( {2\omega h} \right) + \kappa } \right]{\text{csch}} \left( {2\omega h} \right) + \left( {\kappa + 1} \right)\alpha_{2} \omega + \alpha_{1} \omega \left[ {\kappa \alpha_{2} \omega \tanh \left( {\omega h} \right) + \kappa + 1} \right]} \right\}, \\ \Delta \left( \omega \right) & = - \left( {\kappa + 1} \right)\omega \left[ {A_{1} A_{4} - A_{2} A_{3} - \left( {A_{1} + A_{4} } \right) + 1} \right]. \\ \end{aligned} $$

(A.2)