Nonlinear Viscoelastic Closure of Salt Cavities

Abstract

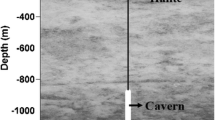
Time-dependent hole closure is a major problem for the many cavities present in rock salt. We use analytical and numerical methods to study how cylindrical holes close under pressure loads with time. We treat salt as a viscoelastic fluid and we use an incompressible nonlinear Maxwell constitutive law to model its mechanical behavior. The viscosity is described by either a power law or an Ellis model depending on whether dislocation creep is considered alone or in combination with pressure solution. The instantaneous closure rate of a circular hole in a power law-based viscoelastic salt is fully determined analytically. A proxy for the transient closure velocity at the rim is also proposed based on a modified version of the characteristic relaxation time θ proposed by Wang et al. (Rock Mech Rock Eng 48(6):2369–2382, 2015) and it has less than 3% inaccuracy for times smaller than 3θ, irrespective of the load or salt type. We derive an analytical expression describing the instantaneous closure rate in an Ellis-based viscoelastic salt. A load threshold determines whether steady state is approached initially. The time θ is also a characteristic relaxation time for this constitutive law, and a master curve can be used to describe the evolution of the closure velocity with time. Using these characteristic values in a typical application underlines the importance of considering pressure solution, in addition to dislocation creep, when studying hole closure in rock salt.

Appendix

1.1 Steady-State Closure Velocity for an Incompressible Ellis Fluid

From Eq. (15)

$$\frac{1}{2}\left[ \sigma \right]_{r}^{\infty }+\int\limits_{r}^{\infty } {\frac{\tau }{r}{\text{d}}r} =0.$$

(47)

Making the change of variable $D= - {v_R}{R \mathord{\left/ {\vphantom {R {{r^2}}}} \right. \kern-0pt} {{r^2}}}$, with

$$\frac{{{\text{d}}r}}{r}= - \frac{1}{2}\frac{{{\text{d}}D}}{D}.$$

(48)

We get

$$\left( { - \bar {p} - \sigma } \right)+\int\limits_{0}^{{D(r)}} {\frac{\tau }{D}} {\text{d}}D=0.$$

(49)

For an Ellis model

$$D=\frac{\tau }{{{A_{{\text{PS}}}}}}+\frac{{{\tau ^n}}}{{{A_{\text{D}}}}}.$$

(50)

So

$${\text{d}}D=\left( {\frac{1}{{{A_{{\text{PS}}}}}}+n\frac{{{\tau ^{n - 1}}}}{{{A_{\text{D}}}}}} \right){\text{d}}\tau .$$

(51)

Making another change of variables leads to:

$$\sigma = - \;\bar {p}+\int\limits_{0}^{{\tau \left( {D(r)} \right)}} {\frac{{\frac{\tau }{{{A_{{\text{PS}}}}}}+n\frac{{{\tau ^n}}}{{{A_{\text{D}}}}}}}{{\frac{\tau }{{{A_{{\text{PS}}}}}}+\frac{{{\tau ^n}}}{{{A_{\text{D}}}}}}}{\text{d}}\tau } ,$$

(52)

which can be solved as:

$$\sigma = - \bar {p}+\tau \left( {n - \left( {n - 1} \right){}_{2}{F_1}\left( {1,\frac{1}{{n - 1}};\frac{n}{{n - 1}}; - \frac{{{A_{{\text{PS}}}}}}{{{A_{\text{D}}}}}{\tau ^{n - 1}}} \right)} \right),$$

(53)

where ${}_{2}{F_1}$ is Gauss’s hypergeometric function. Using Euler’s hypergeometric transformation, and the relationship linking ${}_{2}{F_1}$ with the beta incomplete function B, we get:

$$\sigma = - \;\bar {p}+\tau \left( {n - \frac{1}{{{{\left( {\frac{{{A_{{\text{PS}}}}}}{{{A_{\text{D}}}}}} \right)}^{\frac{1}{{n - 1}}}}\tau }}B\left( {\frac{{\frac{{{A_{{\text{PS}}}}}}{{{A_{\text{D}}}}}{\tau ^{n - 1}}}}{{\frac{{{A_{{\text{PS}}}}}}{{{A_{\text{D}}}}}{\tau ^{n - 1}}+1}};\frac{1}{{n - 1}},\frac{{n - 2}}{{n - 1}}} \right)} \right),$$

(54)

which is valid for n > 2. Using the boundary condition at r = R and that $\tau _{{{\text{II}}}}^{*}={\left( {{{{A_{\text{D}}}} \mathord{\left/ {\vphantom {{{A_{\text{D}}}} {{A_{{\text{PS}}}}}}} \right. \kern-0pt} {{A_{{\text{PS}}}}}}} \right)^{{1 \mathord{\left/ {\vphantom {1 {n - 1}}} \right. \kern-0pt} {n - 1}}}}$:

$$\Delta p+\tau \left( {n - \frac{{\tau _{{{\text{II}}}}^{*}}}{\tau }B\left( {\frac{1}{{1+{{\left( {\frac{{\tau _{{{\text{II}}}}^{*}}}{\tau }} \right)}^{n - 1}}}};\frac{1}{{n - 1}},\frac{{n - 2}}{{n - 1}}} \right)} \right)=0.$$

(55)

1.2 Initial Closure Velocity for an Incompressible Nonlinearly Viscoelastic Material Having a Power Law Viscosity Model

As established in Eq. (26):

$$\frac{{{v_{\text{R}}}}}{R}= - \int\limits_{R}^{\infty } {\frac{1}{{{\mu _{{\text{app}}}}}}\frac{\tau }{r}{\text{d}}r} .$$

(56)

For a power law viscosity model this becomes:

$$\frac{{{v_{\text{R}}}}}{R}= - \;\frac{2}{{{{\rm A}_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{\operatorname{sgn} (\tau ){{\left| \tau \right|}^n}}}{r}{\text{d}}r} .$$

(57)

In $t=0+$, the stress is elastic: $\tau = - \;\Delta p{\left( {\frac{R}{r}} \right)^2},$

$$\frac{{{v_{\text{R}}}(t=0+)}}{R}=\operatorname{sgn} (\Delta p)\frac{{2{{\left| {\Delta p} \right|}^n}{R^{2n}}}}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{1}{{{r^{2n+1}}}}{\text{d}}r} ,$$

(58)

$$\frac{{{v_{\text{R}}}(t=0+)}}{R}=\frac{{\operatorname{sgn} (\Delta p)}}{{{A_{\text{D}}}}}\frac{{{{\left| {\Delta p} \right|}^n}}}{n}.$$

(59)

1.3 Initial Closure Acceleration for an Incompressible Nonlinearly Viscoelastic Material Having a Power Law Viscosity Model

Assuming closure is occurring, i.e., $\Delta p<0$, and deriving Eq. (56) according to time gives

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)= - \frac{2}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{n{\tau ^{n - 1}}\dot {\tau }}}{r}{\text{d}}r} ,$$

(60)

but from the constitutive law we know that:

$$\dot {\tau }=2GD - \frac{G}{{{\mu _{{\text{app}}}}}}\tau ,$$

(61)

so

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)= - \frac{2}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{n{\tau ^{n - 1}}}}{r}\left( {2GD - \frac{G}{{{\mu _{{\text{app}}}}}}\tau } \right){\text{d}}r} ,$$

(62)

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)=\frac{{4nGR{v_{\text{R}}}}}{{{A_{\text{D}}}}}\int\limits_{{\text{R}}}^{\infty } {\frac{1}{{{r^3}}}\frac{1}{{{\tau ^{1 - n}}}}{\text{d}}r} +\frac{{4nG}}{{A_{{\text{D}}}^{2}}}\int\limits_{R}^{\infty } {\frac{1}{r}\frac{1}{{{\tau ^{1 - 2n}}}}{\text{d}}r} .$$

(63)

In $t=0+$, the stress is elastic: $\tau = - \Delta p{\left( {\frac{R}{r}} \right)^2},$

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)(t=0+)=\frac{{2G}}{{{A_{\text{D}}}}}\left( {{{\left| {\Delta p} \right|}^{n - 1}}\frac{{{v_{\text{R}}}}}{R} - \frac{n}{{(2n - 1)}}\frac{{{{\left| {\Delta p} \right|}^{2n - 1}}}}{{{A_{\text{D}}}}}} \right).$$

(64)

Initially, $\frac{{{v_{\text{R}}}}}{R}= - \frac{1}{{{A_{\text{D}}}}}\frac{{{{\left| {\Delta p} \right|}^n}}}{n}$ so:

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)(t=0+)=\frac{{2G}}{{\left| {\Delta p} \right|}}\frac{{n{{\left( {n - 1} \right)}^2}}}{{2n - 1}}\frac{{v_{{\text{R}}}^{2}}}{{{R^2}}}.$$

(65)

1.4 Initial Closure Velocity for an Incompressible Nonlinearly Viscoelastic Material Having an Ellis Viscosity Model

As established in Eq. (26):

$$\frac{{{v_{\text{R}}}}}{R}= - \int\limits_{R}^{\infty } {\frac{1}{{{\mu _{{\text{app}}}}}}\frac{\tau }{r}{\text{d}}r,}$$

(66)

so for an Ellis viscosity model we have:

$$\frac{{{v_{\text{R}}}}}{R}= - \int\limits_{R}^{\infty } {2\left( {\frac{1}{{{A_{{\text{PS}}}}}}+\frac{{{{\left| \tau \right|}^{n - 1}}}}{{{A_{\text{D}}}}}} \right)\frac{\tau }{r}{\text{d}}r.}$$

(67)

At $t=0+$, $\tau = - \Delta p{\left( {\frac{R}{r}} \right)^2},$

$$\frac{{{v_{\text{R}}}(t=0+)}}{R}=\frac{1}{{{A_{{\text{PS}}}}}}\Delta p+\operatorname{sgn} (\Delta p)\frac{1}{{{A_{\text{D}}}}}\frac{{{{\left| {\Delta p} \right|}^n}}}{n}.$$

(68)

1.5 Initial Closure Acceleration for an Incompressible Nonlinearly Viscoelastic Material Having an Ellis Viscosity Model

Assuming closure is occurring, i.e., $\Delta p<0$, and deriving Eq. (67) according to time gives

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)= - \frac{2}{{{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{{\dot {\tau }}}{r}{\text{d}}r} - \frac{2}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{n{\tau ^{n - 1}}\dot {\tau }}}{r}{\text{d}}r} ,$$

(69)

$$\dot {\tau }=2GD - \frac{G}{{{\mu _{{\text{app}}}}}}\tau ,$$

(70)

$$\frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)= - \frac{2}{{{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{1}{r}\left( {2GD - \frac{G}{{{\mu _{{\text{app}}}}}}\tau } \right){\text{d}}r} - \frac{2}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{n{\tau ^{n - 1}}}}{r}\left( {2GD - \frac{G}{{{\mu _{{\text{app}}}}}}\tau } \right){\text{d}}r.}$$

(71)

Using that $D= - \;{v_{\text{R}}}{R \mathord{\left/ {\vphantom {R {{r^2}}}} \right. \kern-0pt} {{r^2}}}$ and Eq. (10)

$$\begin{aligned} \frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_{\text{R}}}}}{R}} \right)= & \frac{{4{v_{\text{R}}}RG}}{{{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{1}{{{r^3}}}{\text{d}}r} +\frac{{4n{v_{\text{R}}}RG}}{{{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{{\tau ^{n - 1}}}}{{{r^3}}}{\text{d}}r} \\ & \quad +\frac{{4G}}{{{A_{{\text{PS}}}}{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{\tau }{r}{\text{d}}r} +\frac{{4G}}{{{A_{{\text{PS}}}}{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{{\tau ^n}}}{r}{\text{d}}r} \\ & \quad +\frac{{4nG}}{{{A_{\text{D}}}{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{{{\tau ^n}}}{r}{\text{d}}r} +\frac{{4nG}}{{{A_{\text{D}}}{A_{\text{D}}}}}\int\limits_{R}^{\infty } {\frac{{{\tau ^{2n - 1}}}}{r}{\text{d}}r} . \\ \end{aligned}$$

(72)

At $t=0+,$ $\tau = - \Delta p{\left( {\frac{R}{r}} \right)^2},$

$$\begin{aligned} \frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_R}}}{R}} \right)(t=0+)= & \frac{{4{v_{\text{R}}}RG}}{{{A_{{\text{PS}}}}}}\int\limits_{R}^{\infty } {\frac{1}{{{r^3}}}{\text{d}}r} +\frac{{4n{v_{\text{R}}}RG}}{{{A_{\text{D}}}}}{\left( { - \Delta p} \right)^{n - 1}}{R^{2n - 2}}\int\limits_{R}^{\infty } {\frac{1}{{{r^{2n+1}}}}{\text{d}}r} \\ & \quad +\frac{{4G}}{{{A_{{\text{PS}}}}{A_{{\text{PS}}}}}}\left( { - \Delta p} \right){R^2}\int\limits_{R}^{\infty } {\frac{1}{{{r^3}}}{\text{d}}r} +\frac{{4G}}{{{A_{{\text{PS}}}}{A_{\text{D}}}}}{\left( { - \Delta p} \right)^n}{R^{2n}}\int\limits_{R}^{\infty } {\frac{1}{{{r^{2n+1}}}}{\text{d}}r} \\ & \quad +\frac{{4nG}}{{{A_{\text{D}}}{A_{{\text{PS}}}}}}{\left( { - \Delta p} \right)^n}{R^{2n}}\int\limits_{R}^{\infty } {\frac{1}{{{r^{2n+1}}}}{\text{d}}r} +\frac{{4nG}}{{{A_{\text{D}}}{A_{\text{D}}}}}{\left( { - \Delta p} \right)^{2n - 1}}{R^{4n - 2}}\int\limits_{R}^{\infty } {\frac{1}{{{r^{4n - 1}}}}{\text{d}}r} , \\ \end{aligned}$$

(73)

so, finally

$$\begin{aligned} \frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{{v_R}}}{R}} \right)(t=0+)&= 2G\left( {\frac{1}{{{A_{{\text{PS}}}}}}+\frac{1}{{{A_{\text{D}}}}}{{\left( { - \Delta p} \right)}^{n - 1}}} \right)\frac{{{v_R}}}{R} \\ & \quad +2G\left( { - \Delta p} \right)\left( {\frac{1}{{{A_{{\text{PS}}}}{A_{{\text{PS}}}}}}+\frac{1}{{{A_{{\text{PS}}}}{A_{\text{D}}}}}\frac{{{{\left( { - \Delta p} \right)}^{n - 1}}}}{n}+\frac{1}{{{A_{\text{D}}}{A_{{\text{PS}}}}}}{{\left( { - \Delta p} \right)}^{n - 1}}+\frac{n}{{2n - 1}}\frac{1}{{{A_{\text{D}}}{A_{\text{D}}}}}{{\left( { - \Delta p} \right)}^{2n - 2}}} \right). \\ \end{aligned}$$

(74)