Meson Exchange Currents in the Clothed-Particle Representation: Calculation of the Deuteron Magnetic Form Factor

Abstract

We show an original way to build up a new family of electromagnetic meson exchange current operators via the method of unitary clothing transformations. Being introduced in such a way they do not depend on the choice of states with which we calculate the matrix elements. The new expressions for meson exchange currents has been compared with ones derived within the previous explorations. Special attention is paid to the deuteron eigenvalue problem and finding the proper deuteron states in a moving frame. An effective technique of ensuring the gauge independence based upon generalization of Siegert’s theorem is proposed. Magnetic form factor of the deuteron is calculated with both one-body and two-body mechanisms. The influence of relativistic effects in the one-body calculation has been considered. Besides, separate contributions from the different meson exchange mechanisms are discussed.

Appendices

Appendix A: Several Technicalities

As was said in Sect. 1, we will show here how to obtain the formula (9) based on the in(out) formalism. At this point, we consider Lagrangian density

$$\begin{aligned} \mathcal {L} = \mathcal {L}_e + \mathcal {L}_h + \mathcal {L}_{\gamma } + \mathcal {L}_{\gamma e} + \mathcal {L}_{\gamma h} \end{aligned}$$

(A1)

for interacting electrons, hadrons and photons, where after Schwinger we have

$$\begin{aligned} \mathcal {L}_e= & {} \frac{i}{4} [\bar{\psi }_e, \gamma ^{\mu } \partial _{\mu } \psi _e] - \frac{i}{4} [\partial _{\mu } \bar{\psi }_e \gamma ^{\mu }, \psi _e] - \frac{m_0^e}{2}[\bar{\psi }_e, \psi _e], \end{aligned}$$

(A2)

$$\begin{aligned} \mathcal {L}_h= & {} \mathcal {L}_N + \mathcal {L}_M + \mathcal {L}_{\mathcal {M}N}, \end{aligned}$$

(A3)

$$\begin{aligned} \mathcal {L}_N= & {} \frac{i}{4} [\bar{\psi }, \gamma ^{\mu } \partial _{\mu } \psi ] - \frac{i}{4} [\partial _{\mu } \bar{\psi } \gamma ^{\mu }, \psi ] - \frac{{m}_0}{2}[\bar{\psi }, \psi ], \end{aligned}$$

(A4)

with the density $\mathcal {L}_M$ for mesons consists of the pionic (pseudoscalar) part

$$\begin{aligned} \mathcal {L}_M= & {} \frac{1}{2} [ \partial _{\mu } {\varvec{\mathbf {\varphi }}}_{ps} \partial ^{\mu } {\varvec{\mathbf {\varphi }}}_{ps} - \mu _0^2 {\varvec{\mathbf {\varphi }}}_{ps}^2] \end{aligned}$$

(A5)

as well as heavier meson contributions. $\mathcal {L}_{\mathcal {M}N} = \mathcal {L}_{s} + \mathcal {L}_{ps} + \mathcal {L}_{v} +\cdots$ is the density of the meson–nucleon interaction. In particular, in the case of pseudoscalar coupling

$$\begin{aligned} \mathcal {L}_{ps}= & {} - \frac{ig_0}{2} [\bar{\psi }, \gamma _5 {\varvec{\mathbf {\tau }}} \psi ] {\varvec{\mathbf {\varphi }}}_{ps} \end{aligned}$$

(A6)

where $\psi$ is a two-component isospinor, ${\varvec{\mathbf {\tau }}} = (\tau ^x,\tau ^y,\tau ^z)$ the Pauli matrices in the isospin space and ${\varvec{\mathbf {\varphi }}}_{ps} =({\varphi }_{ps}^x(x), {\varphi }_{ps}^y(x), {\varphi }_{ps}^z(x))$ is an isovector in the charge pion space. Here, we used $m_0^e$ and $m_0$ for the electron and nucleon bare (trial) masses, respectively, as well as $g_0$ is a trial coupling constant. Throughout this paper we use the definitions and notations of [22], so, e.g., $\gamma _\mu \gamma _\nu + \gamma _\nu \gamma _\mu = 2 g_{\mu \nu }$, $g_{00}=1, g_{11}=g_{22}=g_{33}=-1$, $\gamma _\mu ^\dag = \gamma _0 \gamma _\mu \gamma _0$ and $\gamma _5=i \gamma ^0 \gamma ^1 \gamma ^2 \gamma ^3$.

Electromagnetic interactions with the lepton current $J_e^{\mu } = \frac{e_0}{2} [\bar{\psi }_e, \gamma _{\mu } \psi _e]$ and the hadron current $J_h = J_N + J_M$, where $J^{\mu }_N = \frac{e_0}{2} [\bar{\psi }, \gamma ^{\mu } \frac{1 + \tau ^z}{2} \psi ]$ are given by

$$\begin{aligned} \mathcal {L}_{\gamma e}= & {} J_e^{\mu } A^{\mu } = J_e A \end{aligned}$$

(A7)

$$\begin{aligned} \mathcal {L}_{\gamma h}= & {} J_h A \end{aligned}$$

(A8)

At last, the Lagrangian density for the radiation field after Stückelberg is defined as

$$\begin{aligned} \mathcal {L}_{\gamma }= & {} -\frac{1}{4} F_{\mu \nu } F^{\mu \nu } - \frac{1}{2} (\partial A)^2 + \frac{\mu _{\gamma }^2}{2} A^2 \end{aligned}$$

(A9)

with infinitesimally small photon mass $\mu _{\gamma }$. $\partial A \equiv \partial _{\mu } A^{\mu }$ is the divergence of the electromagnetic field vector $A = (A^0(x), A^1(x), A^2(x), A^3(x))$.

Note also that these currents originate from the so-called minimal substitution $\partial ^{\mu } \rightarrow \partial ^{\mu } + ie_0 A^{\mu }$. Introduction of non-minimal currents is a separate problem, but their inclusion does not violate the gauge invariance of the theory.

The corresponding Euler–Lagrange equations

$$\begin{aligned} (i \partial \hspace{-0.45em} / - m_0) \psi _e= & {} e_0A \hspace{-0.45em} / \psi _e \end{aligned}$$

(A10)

$$\begin{aligned} \bar{\psi }_e (-i \partial \hspace{-0.45em} / - m_0)= & {} e_0 \bar{\psi }_eA \hspace{-0.45em} / \end{aligned}$$

(A11)

$$\begin{aligned} (i \partial \hspace{-0.45em} / - {m}^N_0) \psi= & {} \frac{\partial \mathcal {L}_{\mathcal {M}N}}{\partial \bar{\psi }} + e_0 A \hspace{-0.45em} / \frac{1 + \tau ^z}{2}\psi \end{aligned}$$

(A12)

$$\begin{aligned} \bar{\psi } (-i \partial \hspace{-0.45em} / - {m}^N_0)= & {} \frac{\partial \mathcal {L}_{\mathcal {M}N}}{\partial \psi } + e_0 \frac{1 + \tau ^z}{2} \bar{\psi } A \hspace{-0.45em} / \end{aligned}$$

(A13)

$$\begin{aligned} \Box A_{\mu }(x)= & {} J_e^{\mu }(x) + J_h^{\mu }(x) \equiv {J}_{\mu }(x) \end{aligned}$$

(A14)

where we introduced the symbol $O \hspace{-0.6em} / = \gamma _{\mu }O^{\mu }$ for any vector O and $\frac{1 + \tau ^z}{2}$ is the proton isospin projection operator.

A possible nonperturbative way of obtaining expressions similar to Eq. (9) is to apply some recipes of the reduction technique proposed within the in(out) formalism, in particular, the so-called asymptotic LSZ approach (see, e.g., monographs by Schweber (1961) [38], Goldberger and Watson (1964) [39], Bjorken and Drell (1962) [22]). In this context,

let us start from the typical in(out) form

$$\begin{aligned} \langle e'h'; out | eh; in \rangle= & {} - \frac{m_e}{\sqrt{EE'}} \int d^4x' \int d^4x \frac{e^{ik'x' -ikx}}{(2 \pi )^3}\bar{u}_{\alpha '}(k') [i \partial \hspace{-0.45em} /' -m_e]_{\alpha ' \beta '} \nonumber \\ {}{} & {} \times \langle h' | T\{\psi ^e_{\beta '}(x') \bar{\psi }^e_{\beta } (x) \} | h \rangle [-i \partial \hspace{-0.45em} / -m_e]_{\beta \alpha } u_{\alpha }(k) \end{aligned}$$

(A15)

$$\begin{aligned} T\{\psi ^e_{\beta '}(x') \bar{\psi }^e_{\beta } (x) \}= & {} \theta (x'_0 - x_0) \psi ^e_{\beta '}(x') \bar{\psi }^e_{\beta } (x) - \theta (x_0 - x'_0) \bar{\psi }^e_{\beta } (x) \psi ^e_{\beta '}(x') \end{aligned}$$

(A16)

Henceforth, the superscript “e” will be omitted. We find step by step

$$\begin{aligned}{} & {} [i \partial \hspace{-0.45em} /' -m_e]_{\alpha ' \beta '} T\{\psi _{\beta '}(x') \bar{\psi }_{\beta } (x) \} [-i \partial \hspace{-0.45em} / -m_e]_{ \beta \alpha } \nonumber \\{} & {} \quad =i \gamma ^0_{\alpha ' \beta '} \delta (x_0'-x_0) \{ \psi _{\beta '}(x') \bar{\psi } _{\beta }(x) + \bar{\psi } _{\beta }(x) \psi _{\beta '}(x')\} [i\partial _0 \gamma ^0 -i {\varvec{\mathbf {\gamma }}} {\varvec{\mathbf {\partial }}} - m]_{\beta \alpha } \nonumber \\{} & {} \qquad + \{ \theta (x_0'-x_0) [i \partial \hspace{-0.45em} /' -m]_{\alpha ' \beta '} \psi _{\beta '}(x') {\overline{\psi }}_{\beta }(x) \nonumber \\{} & {} \qquad -\theta (x_0-x_0') \bar{\psi }_{\beta }(x) [i \partial \hspace{-0.45em} /' -m]_{\alpha ' \beta '} \psi _{\beta '}(x') [-i \partial _0 \gamma ^0 - i {\varvec{\mathbf {\gamma }}} {\varvec{\mathbf {\partial }}} -m]_{\beta \alpha } \} \end{aligned}$$

(A17)

or with the help of Eqs. (A10)–(A11)

$$\begin{aligned}&= \partial _0 \delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \{ \psi _{\beta '}(x') , \bar{\psi } _{\beta }(x) \} \gamma ^0_{\beta \alpha } \end{aligned}$$

(A18)

$$\begin{aligned}&+ i e_0 \delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \{ \psi _{\beta '}(x') \bar{\psi } _{\beta }(x) A \hspace{-0.45em} / _{\beta \alpha }(x) + \bar{\psi } _{\beta }(x) A \hspace{-0.45em} / _{\beta \alpha }(x) \psi _{\beta '}(x') \} \end{aligned}$$

(A19)

$$\begin{aligned}&- ie_0 \delta (x_0'-x_0) \{ A \hspace{-0.45em} /_{\alpha ' \beta '}(x') \psi _{\beta '} (x') \bar{\psi }_{\beta }(x) + \bar{\psi }_{\beta }(x) A \hspace{-0.45em} /_{\alpha ' \beta '}(x') \psi _{\beta '} (x') \}\gamma ^0_{\beta \alpha } \end{aligned}$$

(A20)

$$\begin{aligned}&+ e_0^2 T \{ [A \hspace{-0.45em} /(x') + \delta m]_{\alpha ' \beta '} \psi _{\beta '}(x') \bar{\psi }_{\beta }(x) [A \hspace{-0.45em} /(x) + \delta m]_{\beta \alpha } \} \end{aligned}$$

(A21)

Owing to

$$\begin{aligned} \{ \psi _{\beta '}(x'), \bar{\psi } _{\beta }(x) \} = \delta ({\varvec{\textbf{x}}}' - {\varvec{\textbf{x}}}) \gamma ^0_{\beta ' \beta } \end{aligned}$$

(A22)

the mass counterterms in (A19) and (A20) are omitted giving zero contributions to the amplitude of interest. The contribution from the first term (A18) consists of an integral with the integrand

$$\begin{aligned} ik_0 \delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \langle h' | \{ \psi _{\beta '}(x'), \bar{\psi } _{\beta }(x) \} | h \rangle \gamma ^0_{\beta \alpha } \end{aligned}$$

(A23)

which is nonzero only for forward scattering, and an integral that contains

$$\begin{aligned}{} & {} -\delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \{ \psi _{\beta '}(x'), \partial _0 \bar{\psi } _{\beta }(x) \} \gamma ^0_{\beta \alpha } \nonumber \\{} & {} \quad = - \delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \{ \psi _{\beta '}(x'), \bar{\psi } _{\beta }(x) \} [{\varvec{\mathbf {\gamma }}} {\varvec{\mathbf {\partial }}} + i \, m_0]_{\beta \alpha } \nonumber \\{} & {} \qquad - ie_0 \delta (x_0'-x_0) \gamma ^0_{\alpha ' \beta '} \{ \psi _{\beta '}(x'), \bar{\psi } _{\beta }(x) A \hspace{-0.45em} /_{\beta \alpha } (x)\} \end{aligned}$$

(A24)

Again the first term gives a c-number contribution, while the second term cancels with (A19).

Thus, apart from the forward scattering, we find

$$\begin{aligned}{} & {} [i \partial \hspace{-0.45em} /' -m]_{\alpha ' \beta '} T \{ \psi _{\beta '}(x') \bar{\psi } _{\beta }(x) \} [-i \partial \hspace{-0.45em} /-m]_{\beta \alpha } \nonumber \\{} & {} \quad =ie_0\delta (x_0'-x_0) \{A \hspace{-0.45em} /_{\alpha ' \beta '}(x') \psi _{\beta '}(x') \bar{\psi }_{\beta }(x) + \bar{\psi }_{\beta }(x) A \hspace{-0.45em} /_{\alpha ' \beta '}(x') \psi _{\beta '}(x') \} \gamma ^0_{\beta \alpha } \nonumber \\{} & {} \quad + e_0^2 T\{ [A \hspace{-0.45em} /(x') + \delta m]_{\alpha ' \beta '} \psi _{\beta '}(x') \bar{\psi }_{\beta }(x) [A \hspace{-0.45em} /(x) + \delta m]_{\beta \alpha } \} \end{aligned}$$

(A25)

But at equal times $x_0'=x_0$

$$\begin{aligned} \{A \hspace{-0.45em} /_{\alpha ' \beta '} (x')\psi _{\beta '}(x') \bar{\psi }_{\beta }(x) + \bar{\psi }_{\beta }(x) A \hspace{-0.45em} /_{\alpha ' \beta '}(x') \psi _{\beta '}(x') \} \gamma ^0_{\beta \alpha } \nonumber \\ = A \hspace{-0.45em} /_{\alpha ' \beta '} \{\psi _{\beta '}(x'), \bar{\psi }_{\beta }(x) \} \gamma ^0_{\beta \alpha } = A \hspace{-0.45em} /_{\alpha ' \alpha } \delta ({\varvec{\textbf{x}}}' - {\varvec{\textbf{x}}}) \end{aligned}$$

(A26)

so, omitting for a moment the $e_0^2$ term on the right hand side of (A25) we arrive to

$$\begin{aligned} \langle e'h'; out | eh; in \rangle= & {} - \frac{im e_0}{\sqrt{EE'}} \bar{u}_{\alpha '}(k') \int d^4x \frac{e^{i(k' -k)x}}{(2 \pi )^3} \langle h' | A \hspace{-0.45em} /_{\alpha ' \alpha } (x) | h \rangle u_{\alpha }(k) \nonumber \\= & {} -2 \pi i \frac{m e_0}{\sqrt{EE'}} \bar{u}(k') \gamma _{\mu } u(k)\langle h' | A^{\mu } (0) | h \rangle \delta (p'+k'-p-k) \end{aligned}$$

(A27)

It is convenient to do the last step from (A27) to (9) via a little trick that is valid on the energy shell $p'+k'=p+k$, viz.,

$$\begin{aligned} q_{\nu } q^{\nu } \langle h' | A^{\mu } (0) | h \rangle = \langle h' | [{P}_{\nu },[{P}^{\nu }, A^{\mu } (0)]] | h \rangle \end{aligned}$$

(A28)

and taking into account the equation of motion (A14)

$$\begin{aligned}{}[{P}_{\nu },[{P}^{\nu }, A^{\mu } (0)]] = - \Box A^{\mu } (0) = - {J}^{\mu }(0), \end{aligned}$$

(A29)

where ${P}_{\nu }$ is the 4-momentum operator and the current density operator J(x) taken at the space-time point $x=(t,{\varvec{\textbf{x}}})=0$ consists of the hadronic part $J_h(0)$ and electronic one $J_e(0)$. The matrix elements of the last one

$$\begin{aligned} \langle h' | J_e^{\alpha }(0) | h \rangle = 0 \end{aligned}$$

(A30)

with accuracy up to the terms of the $e^1$-order, so $\langle h' | {J}^{\mu }(0) | h \rangle$ can be replaced by $\langle h' | J_h^{\mu } (0) | h \rangle$. In addition, assuming that the difference between $e_0$ and particle’s physical charge ${e_0 - e \sim O(e^3)}$ we can resort to the OPEA in order to eventually obtain the formula (9).

Appendix B: Relevant Model Definitions

In this article, we use the model from the Ref. [18]. Here we would like to highlight some definitions regarding our field-theoretical model.

A departure point is to use the so-called Legendre transformation from the Lagrangians (A1)-(A8) with “bare” masses $m_0^e$, $m_0$ and constants $g_0$, $e_0$ to the Hamiltonians H expressed in terms of the independent canonical variables and their conjugates. In this context, the model hadronic Hamiltonian can be separated into the free and interaction parts $H_h(\alpha )={H^h_F(\alpha )+H^h_I(\alpha )}$, where the interaction part

$$\begin{aligned} H^h_I(\alpha ) \equiv H_{\mathcal {M}N}=M_\text {ren}+V_\text {ren}+V_{\mathcal {M}N} \end{aligned}$$

(B31)

includes the mass $M_\text {ren}$ and vertex $V_\text {ren}$ counterterms. The interaction is a function of creation (destruction) operators in the BPR, i.e., referred to the bare particles with physical masses [1]. Formally, the latter can be introduced via the mass-changing Bogoliubov-type transformation, which is familiar in the theory of superfluidity and superconductivity. We will use the Hamiltonian $H^h_I \equiv H_{\mathcal {M}N}$ from [18], where fermions (nucleons and antinucleons) and bosons ($\delta$-, $\pi$-, $\rho$-mesons, etc.) interact via the Yukawa-type couplings for scalar (s), pseudoscalar (ps), and vector (v) mesons. For example, interaction between the nucleon and $\pi$ meson fields (${\mathcal {M}}N = ps$) is

$$\begin{aligned} V_{ps}=i g_{ps} \int d \textbf{x}:{\bar{\psi }}(\textbf{x}) \gamma _5 {\varvec{\mathbf {\tau }}} \psi (\textbf{x}): {\varvec{\mathbf {\varphi }}}_{ps}(\textbf{x}), \end{aligned}$$

(B32)

with fields $\psi ({\varvec{\textbf{x}}})$, ${\varvec{\mathbf {\phi }}}({\varvec{\textbf{x}}})$ in the Schrödinger (S) picture, and symbol  :  denotes the normal ordering, where all the creation operators are to the left of the destruction ones.

The Nöther current density $J_h^\alpha ({\varvec{\textbf{x}}})= J_{N}^\alpha ({\varvec{\textbf{x}}}) + J_{\mathcal {M}}^\alpha ({\varvec{\textbf{x}}})$ in the S-picture consists of the nucleon part

$$\begin{aligned} J_{N}^\alpha ({\varvec{\textbf{x}}})= e:{\bar{\psi }}({\varvec{\textbf{x}}}) \gamma ^\alpha \frac{1 + \tau ^z}{2} \psi ({\varvec{\textbf{x}}}):, ~\text {where}~ \psi = \begin{pmatrix} \psi _{1/2} \\ \psi _{-1/2} \end{pmatrix}, \end{aligned}$$

(B33)

and mesonic one. For the latter, we have the following definitions

$$\begin{aligned} J_{ps}^\alpha ({\varvec{\textbf{x}}})= e:[{\varvec{\mathbf {\varphi }}}_{ps}({\varvec{\textbf{x}}}) \times \partial ^\alpha {\varvec{\mathbf {\varphi }}}_{ps}({\varvec{\textbf{x}}})]^z:, \end{aligned}$$

(B34)

$$\begin{aligned} J_{s}^\alpha ({\varvec{\textbf{x}}})= e:[{\varvec{\mathbf {\varphi }}}_{s}({\varvec{\textbf{x}}}) \times \partial ^\alpha {\varvec{\mathbf {\varphi }}}_{s}({\varvec{\textbf{x}}})]^z:, \end{aligned}$$

(B35)

$$\begin{aligned} J_{v}^\alpha ({\varvec{\textbf{x}}})= e:[{\varvec{\mathbf {\varphi }}}_{v}^{\alpha \beta }({\varvec{\textbf{x}}}) \times {\varvec{\mathbf {\varphi }}}_{v\,\beta }({\varvec{\textbf{x}}})]^z:, \end{aligned}$$

(B36)

with ${\varvec{\mathbf {\varphi }}}_{v}^{\alpha \beta } (x) = \partial ^\alpha {\varvec{\mathbf {\varphi }}}_{v}^{\beta }( x) - \partial ^\beta {\varvec{\mathbf {\varphi }}}_{v}^{\alpha }( x)$. Also, any operator $O_S$ in the Schrödinger picture is connected with $O_H$ in the Heisenberg picture via the equation $O_H(t)=e^{i H_S t} O_S e^{-i H_S t}$.

We prefer to work with the Fock space made up of the subspaces with certain particle numbers by introducing the Fourier expansions

$$\begin{aligned} \begin{aligned}&\psi _\eta ({\varvec{\textbf{x}}}) = \sqrt{\frac{m}{(2\pi )^3}} \sum \limits _{\mu } \int \frac{d{\varvec{\textbf{p}}}}{E_{{\varvec{\textbf{p}}}}} \left[ {\bar{u}} ( p \mu ) b_c( p \mu \eta ) + v( p_- \mu ) d_c^{\dagger }( p_- \mu \eta ) \right] e^{i {\varvec{\textbf{p}}} {\varvec{\textbf{x}}}}, \\&{\varvec{\mathbf {\varphi }}}_{s,ps}({\varvec{\textbf{x}}}) = \frac{1}{\sqrt{2(2\pi )^3}} \int \frac{d {\varvec{\textbf{k}}}}{\omega _{{\varvec{\textbf{k}}}}} \left[ {\varvec{\textbf{a}}}_c(k) + {\varvec{\textbf{a}}}_c^{\dagger }( k_-) \right] e^{i {\varvec{\textbf{k}}} {\varvec{\textbf{x}}}}, \\&{\varvec{\mathbf {\varphi }}}_{v}^\alpha ({\varvec{\textbf{x}}}) = \frac{1}{\sqrt{2(2\pi )^3}} \int \frac{d {\varvec{\textbf{k}}}}{\omega _{{\varvec{\textbf{k}}}}} \left[ e^\alpha (k\sigma ){\varvec{\textbf{a}}}_c(k\sigma ) + e^\alpha (k_-\sigma ){\varvec{\textbf{a}}}_c^{\dagger }( k_-\sigma ) \right] e^{i {\varvec{\textbf{k}}} {\varvec{\textbf{x}}}}, \end{aligned} \end{aligned}$$

(B37)

i.e., giving preference to the corpuscular picture with ${E_{{\varvec{\textbf{p}}}}=\sqrt{m^2 + {\varvec{\textbf{p}}}^2}}$, ${\omega _{{\varvec{\textbf{k}}}}=\sqrt{m_{\mathcal {M}}^2 + {\varvec{\textbf{k}}}^2}}$, nucleon mass m and the corresponding meson mass $m_{\mathcal {M}}$. The independent polarization vectors $e(k\sigma )$ ($\sigma =+1,0,-1$) in the last expansion are transverse $k^\alpha e_\alpha (k\sigma )=0$ and normalized as

$$\begin{aligned} \sum _\sigma e_\alpha (k\sigma )e_\beta ^*(k\sigma ) =-g_{\alpha \beta }+\frac{k_\alpha k_\beta }{m_b^2} \equiv P_{\alpha \beta }(k). \end{aligned}$$

(B38)

As usually, we apply the following commutation rules $[a(k\sigma ),a^\dagger (k'\sigma ')]=\omega _{\textbf{k}}\delta _{\sigma '\sigma }\delta (\textbf{k}'-\textbf{k})$, $\{b(p\mu ),b^\dagger (p'\mu ')\}=\{d(p\mu ),d^\dagger (p'\mu ')\}=E_{\textbf{p}}\delta _{\mu '\mu }\delta (\textbf{p}'-\textbf{p})$.

Appendix C: Parametrization of the Deuteron Wave Functions

Fig. 9

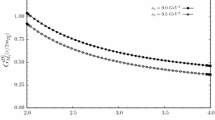
Deuteron WF $\psi _0(p)$, $\psi _2(p)$. Points are calculated using the Kharkiv potential with the parameters UCT GS fit [40]. Solid (Dashed) lines are our (Bonn B) parameterized WFs

Table 1 Coefficients for the parameterized deuteron WF

Deuteron WF $\psi _0(p)$, $\psi _2(p)$ have been calculated using the Kharkiv potential in Refs. [17, 40]. In Fig. 9 they are compared with WFs obtained by the Bonn group [19].

But for many applications it is convenient to have parameterized analytical functions instead of an array of points. We have chosen a fairly common form

$$\begin{aligned} \begin{aligned}&\psi _0^a(p)=\sqrt{\frac{2}{\pi }}\sum _{i=1}^{11} \frac{C_i}{p^2 + m_i^2} \\&\psi _2^a(p)=\sqrt{\frac{2}{\pi }}\sum _{i=1}^{11} \frac{D_i}{p^2 + m_i^2} \end{aligned}, \end{aligned}$$

(C39)

with $m_i = \gamma + (i-1)m_0$, $\gamma =0.2315380~fm^{-1}$ and $m_0=0.9~fm^{-1}$. Additional conditions on the fitting coefficients $C_i$, $D_i$ are imposed due to the asymptotic behavior of the functions $\psi _0(r)$, $\psi _2(r)$ (Fourier transforms of $\psi _0(p)$, $\psi _2(p)$) at large r. Namely, (details in Ref. [19])

$$\begin{aligned} C_{11}= & {} -\sum _{j=1}^{10} C_j, \end{aligned}$$

(C40)

$$\begin{aligned} D_{n-2}= & {} \frac{m_{n-2}^2}{\left( m_n^2-m_{n-2}^2\right) \left( m_{n-1}^2-m_{n-2}^2\right) }\bigg (-m_{n-1}^2 m_n^2 \sum _{j=1}^{n-3} \frac{D_j}{m_j^2} \nonumber \\{} & {} +\left( m_{n-1}^2+m_n^2\right) \sum _{j=1}^{n-3} D_j-\sum _{j=1}^{n-3} D_j m_j^2\bigg ). \end{aligned}$$

(C41)

In order to fit the coefficients we can start with functionals

$$\begin{aligned} I_S^2=\int _0^\infty d p\, p^2 (\psi _0(p)-\psi _0^a(p))^2,\quad I_D^2=\int _0^\infty d p\, p^2 (\psi _2(p)-\psi _2^a(p))^2 \end{aligned}$$

(C42)

that should be minimized. One can note that we already know from the numerical calculations

$$\begin{aligned} \begin{aligned}&P_S=\int _0^\infty d p\, p^2 (\psi _0(p))^2 = \sum _j \omega _j p_j^2 (\psi _0(p_j))^2 = 0.946762, \\&P_D=\int _0^\infty d p\, p^2 (\psi _2(p))^2 = \sum _j \omega _j p_j^2 (\psi _2(p_j))^2 = 0.053238. \end{aligned} \end{aligned}$$

(C43)

Let us impose the restriction that the analytical functions must also be appropriately normalized, then we have bilinear conditions

$$\begin{aligned} N_S\equiv \sum _{i,j} \frac{C_i C_j}{P_S\,(m_i+m_j)}=1 \,\quad N_S\equiv \sum _{i,j} \frac{D_i D_j}{P_D\,(m_i+m_j)}=1. \end{aligned}$$

(C44)

Now, it is easy to see that the functionals (C42)

take minimum when

$$\begin{aligned} O_S\equiv \int _0^\infty d p\, p^2 \psi _0(p) \psi _0^a(p) = \sum _i C_i I^S_i,\quad O_D\equiv \int _0^\infty d p\, p^2 \psi _2(p) \psi _2^a(p) = \sum _i D_i I^D_i \end{aligned}$$

(C45)

take maximum values. Here

$$\begin{aligned} I^S_i \equiv P_S^{-1}\sqrt{\frac{\pi }{2}}\sum _j \omega _j p_j^2 \frac{\psi _0(p_j)}{p^2 + m_i^2},\quad I^D_i \equiv P_D^{-1}\sqrt{\frac{\pi }{2}}\sum _j \omega _j p_j^2 \frac{\psi _2(p_j)}{p^2 + m_i^2}. \end{aligned}$$

(C46)

The coefficients in Table 1 were calculated based on the maximization of $O_S$, $O_D$ with the restrictions (C44). So the accuracy of the parametrization is characterized by

$$\begin{aligned} \begin{aligned}&|O_S-1|= 1.47161\times 10^{-9},\quad |N_S-1|= 1.27898\times 10^{-13}, \\&|O_D-1|= 9.48202\times 10^{-7},\quad |N_D-1|= 5.68434\times 10^{-13}. \end{aligned} \end{aligned}$$

(C47)

Appendix D: Some Details on Calculations of the Relevant Integrals

Expressing in Eq. (90) the components of the momentum ${\varvec{\textbf{p}}}$ via the spherical harmonics

$$\begin{aligned} \begin{aligned}&p_x=\sqrt{\frac{2\pi }{3}} \,p\, (Y_{1-1}(\hat{{\varvec{\textbf{p}}}})-Y_{11}(\hat{{\varvec{\textbf{p}}}})), \quad p_y=i\sqrt{\frac{2\pi }{3}} \,p\, (Y_{1-1}(\hat{{\varvec{\textbf{p}}}})+Y_{11}(\hat{{\varvec{\textbf{p}}}})), \\&p_z=2\sqrt{\frac{\pi }{3}} \,p\, Y_{10}(\hat{{\varvec{\textbf{p}}}}) \quad \text {with} \quad p \equiv |{\varvec{\textbf{p}}}| \end{aligned} \end{aligned}$$

(D48)

and applying the formula

$$\begin{aligned} Y_{l_1m_1}(\hat{{\varvec{\textbf{p}}}}) Y_{l_2m_2}(\hat{{\varvec{\textbf{p}}}})= & {} \sum _{LM_L} \sqrt{\frac{(2l_1+1)(2l_2+1)}{4 \pi (2L+1)}} (l_1m_1l_2m_2|LM_L) \nonumber \\ {}{} & {} \times (l_10l_20|L0)\, Y_{LM_L}(\hat{{\varvec{\textbf{p}}}}) \end{aligned}$$

(D49)

the x-component of the one-body matrix elements are reduced to

$$\begin{aligned} {J}^{[1]x}_{fi}({\varvec{\textbf{q}}}\,M'M)= & {} e\,\sum (l'm'_l1M'_S|1M')(lm_l1M_S|1M) \left( \tfrac{1}{2} \mu '_1 \tfrac{1}{2} \mu |1 M'_S \right) \nonumber \\ {}{} & {} \times \left( \tfrac{1}{2} \mu _1 \tfrac{1}{2} \mu |1 M_S \right) (-1)^{m'_l} \int _0^\infty dp\, p^2\, \psi _{l'}(p) \, \bigg \{ i {\sigma }^y_{\mu '_1\mu _1} F_{3}^{S}(-q^2) \frac{q}{2m} \,I_{l'-m'_l,lm_l}(p,q) \nonumber \\{} & {} + \delta _{\mu '_1\mu _1} F_{1}^{S}(-q^2) \frac{p}{m} \sum _{LM_L} \sqrt{\frac{(2l'+1)}{2 (2L+1)}} (l'010|L0) \nonumber \\ {}{} & {} \times [(l'-m'_l1-1|LM_L)-(l'-m'_l11|LM_L)]\,I_{LM_L,lm_l}(p,q) \bigg \}, \end{aligned}$$

(D50)

with

$$\begin{aligned} I_{LM_L,lm_l}(p,q) \equiv \int d\hat{{\varvec{\textbf{p}}}} Y_{Lm_L}(\hat{{\varvec{\textbf{p}}}})\, Y_{lm_l}(\widehat{{\varvec{\textbf{p}}}-\tfrac{1}{2}{\varvec{\textbf{q}}}}) \psi _{l}(|{\varvec{\textbf{p}}}-\tfrac{1}{2}{\varvec{\textbf{q}}}|). \end{aligned}$$

(D51)

Using the parameterized wave function (C39) and Eqs. (A.1)–(A.7) from [2] the integrals (D51) are equal to

$$\begin{aligned} I_{LM_L,lm_l}(p,q)= & {} \sum _{\lambda } \sqrt{\frac{(2L+1)(2l+1)}{4 \pi (2\lambda +1)}} (Lm_Llm_l|\lambda \, M_L+m_l) \nonumber \\ {}{} & {} \times (L0\,l0|\lambda 0)\, Y_{\lambda M_L+m_l}(\hat{{\varvec{\textbf{q}}}}) a_l^{L\lambda }(p,q), \end{aligned}$$

(D52)

with

$$\begin{aligned} \begin{aligned} a_0^{L\lambda }(p,q)&= \delta _{L\lambda } \frac{4\sqrt{2\pi }}{pq}\,\sum _n C_n Q_L(z_n), \\ a_2^{L\lambda }(p,q)&= -\frac{4\sqrt{2\pi }}{pq}\,\sum _n \frac{D_n}{m_n^2} \bigg \{ p^2 Q_\lambda (z_n) + \frac{q^2}{4} Q_L(z_n) \\&\quad + pq\sqrt{\frac{15}{2}(2\lambda + 1)} \sum _{\lambda '} \frac{(L010|\lambda ' 0)(\lambda 010|\lambda ' 0)}{(L020|\lambda 0)} \begin{Bmatrix} 1&1&2\\ \lambda&L&\lambda ' \end{Bmatrix} Q_{\lambda '}(z_n) \bigg \}, \\ z_n&= \frac{p^2+q^2/4 + m_n^2}{pq}. \end{aligned} \end{aligned}$$

(D53)

Note that $Y_{\lambda M_L+m_l}(\hat{{\varvec{\textbf{q}}}})=\sqrt{\frac{2\lambda +1}{4 \pi }} \delta _{-M_L\,m_l}$ since the vector ${\varvec{\textbf{q}}}$ is directed along the z axis. The last integral in Eq. (D50) could be computed numerically.

Analogously, the x-component of the two-body matrix elements (95) are reduced to the integrals

$$\begin{aligned} K^\mathcal {M}_{L'm'_L,Lm_L}(q,p',p)= & {} \left[ \Lambda _\mathcal {M}^2-m_\mathcal {M}^2\right] ^{2 n_\mathcal {M}} \int d \hat{{\varvec{\textbf{p}}}}' d \hat{{\varvec{\textbf{p}}}}\, Y_{L'm'_L}(\hat{{\varvec{\textbf{p}}}}') Y_{Lm_L}(\hat{{\varvec{\textbf{p}}}}) \nonumber \\ {}{} & {} \times \left[ \Lambda _\mathcal {M}^2+\left( \tfrac{1}{2}{\varvec{\textbf{q}}} - {\varvec{\textbf{p}}}'+{\varvec{\textbf{p}}}\right) ^2\right] ^{-2 n_\mathcal {M}} \left[ m_\mathcal {M}^2+\left( \tfrac{1}{2}{\varvec{\textbf{q}}} - {\varvec{\textbf{p}}}'+{\varvec{\textbf{p}}}\right) ^2\right] ^{-1} \nonumber \\= & {} I^{(1)}_{L'm'_L,Lm_L}(m_\mathcal {M},q,p',p) - \sum _{n=1}^{2n_\mathcal {M}} \left[ \Lambda _\mathcal {M}^2-m_\mathcal {M}^2\right] ^{n-1} I^{(n)}_{L'm'_L,Lm_L}(\Lambda _\mathcal {M},q,p',p)\nonumber \\ \end{aligned}$$

(D54)

with

$$\begin{aligned}{} & {} I^{(n)}_{L'm'_L,Lm_L}(a,q,p',p)\nonumber \\ {}{} & {} \quad =\int d \hat{{\varvec{\textbf{p}}}}' d \hat{{\varvec{\textbf{p}}}}\, Y_{L'm'_L}(\hat{{\varvec{\textbf{p}}}}') Y_{Lm_L}(\hat{{\varvec{\textbf{p}}}}) \left[ a^2+\left( \tfrac{1}{2}{\varvec{\textbf{q}}} - {\varvec{\textbf{p}}}'+{\varvec{\textbf{p}}}\right) ^2\right] ^{-n} \nonumber \\ {}{} & {} \quad = 2 \pi \delta _{-m'_L,m_L} \sqrt{(2L+1)(2L'+1)} \sqrt{\frac{(L-m_L)!(L'+m_L)!}{(L+m_L)!(L'-m_L)!}} \nonumber \\ {}{} & {} \qquad \times \int _{-1}^{1} dx\, P_{L'}^{-m_L}(x) P_{L}^{m_L}\left( \frac{p' x - \tfrac{1}{2} q}{\sqrt{p'^2 + \tfrac{1}{4} q^2 - p' q x}}\right) [2 p \sqrt{p'^2 + \tfrac{1}{4} q^2 - p' q x}]^{-1} \frac{(-i)^{n-1}}{(n-1)!} \nonumber \\ {}{} & {} \qquad \times Q_L^{n-1}(z_a) [\sqrt{2a^2( \tfrac{1}{2} a^2 + p'^2 + \tfrac{1}{4} q^2 + p^2 - p' q x)+(p'^2 + \tfrac{1}{4} q^2 -p^2 - p' q x)^2}]^{-n+1},\nonumber \\ \end{aligned}$$

(D55)

$$\begin{aligned}{} & {} z_a = \frac{a^2 + p'^2 + \tfrac{1}{4} q^2 + p^2 - p' q x}{2 p \sqrt{p'^2 + \tfrac{1}{4} q^2 - p' q x}}. \end{aligned}$$

(D56)

For example, for the $\delta$ mesons the matrix elements are reduced to

$$\begin{aligned} {\varvec{\textbf{J}}}_{fi}^{[2]} ({q}\,M'M)= & {} - \frac{3 e g_\delta ^2 }{(2 \pi )^6 (4m^2-m_\delta ^2)} (l'm'_l1M'_S|1M')(lm_l1M_S|1M) \left( \tfrac{1}{2} \mu '_1 \tfrac{1}{2} \mu '_2 |1 M'_S \right) \nonumber \\ {}{} & {} \times \left( \tfrac{1}{2} \mu _1 \tfrac{1}{2} \mu _2 |1 M_S \right) (-1)^{m'_l} \int _{0}^{\infty } p^2 dp\,\int _{0}^{\infty } p'^2 dp'\, \psi _{l'}({p}') \psi _{l}({p}) \delta _{\mu '_2\mu _2} \nonumber \\ {}{} & {} \times \bigg ( i{\sigma }^y_{\mu '_1\mu _1} q \delta _{m'_lm_l} K^\delta _{l'-m_l,lm_l}(q,p',p) + \delta _{\mu '_1\mu _1} \frac{\sqrt{2}}{2} \sum _L \bigg ( p' \sqrt{\frac{2l'+1}{2L+1}} \nonumber \\ {}{} & {} \times [(l'-m'_l 1-1|L-m_l) -(l'-m'_l 11|L-m_l)](l'010|L0)K^\delta _{L-m_l,lm_l}(q,p',p) \nonumber \\{} & {} + p \sqrt{\frac{2l+1}{2L+1}} [(lm_l 1-1|Lm'_l)-(lm_l 11|Lm'_l)](l010|L0)K^\delta _{l'-m'_l,Lm'_l}(q,p',p) \bigg ) \bigg ),\nonumber \\ \end{aligned}$$

(D57)

In such a way, among the six overlapping integrals in Eq. (95), three are calculated analytically, while the other three remain for the numerical computation.