Skip to main content
SpringerLink
Log in

Influence of Hall and Slip on MHD Reiner-Rivlin blood flow through a porous medium in a cylindrical tube

  • Foundation, algebraic, and analytical methods in soft computing
  • Published:
Soft Computing Aims and scope Submit manuscript

Abstract

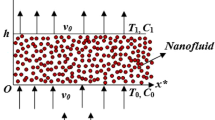
The current study aims to scrutinize the peristalsis of magnetohydrodynamics Reiner-Rivlin fluid model in a cylindrical tube through porous medium. The basic equations, including momentum, heat, and concentration are tackled in the valuable presence of Hall current, Joule heating, viscous-dissipation, and Soret effects. Moreover, slip effect is also entertained. The considered system is simplified by approximating with long wavelengths and very low Reynolds numbers. Perturbation technique is chosen to obtain the closed form analytical solutions. The impact of influential parameters is presented through plots and physically discussed in detail. The main conclusions of this work are that the velocity and temperature fields exhibit opposite behavior for the Hartman number and Hall parameter. The slip parameter has minimizing impact on velocity distribution. The fluid velocity tends to increase when Reiner-Rivlin fluid parameter is incremented. The Darcy number has upgrading impact on fluid temperature. The solute concentration minifies when slip parameter is incremented. It can be visualized that size of trapped bolus becomes larger on increasing the value of Hall and slip parameter. This research investigation basically examine the blood flow through an artery under strong electric and magnetic field effects. Therefore, this study laid the ground work for scientists, engineers, and medical practitioners working in physiological field.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data availability

The data used to support the findings of this study are included within the article.

Abbreviations

\(\overline{\user2{V}} = \left( {u\left( {r,z,t} \right),0,w\left( {r,z,t} \right)} \right)\) :

Velocity vector

\({\varvec{J}} = \frac{A}{{m^{2} }}\) :

Current density

\(\sigma = \frac{S}{m}\) :

Electric conductivity

\(m = \left( {\frac{{\sigma B_{0} }}{{en_{e} }}} \right)\) :

Hall effect

\(\tau_{ij}\) :

Cauchy stress tensor

\(\theta\) :

Temperature in dimensionless form

(\(T_{0} ,T_{1} )\) :

Temperature at lower and upper wall, respectively

\(Q\) :

Volume flow rate

\(\delta_{ij}\) :

Kronecker delta

\(e_{ij}\) :

Strain tensor rate

\(\mu_{c}\) :

Cross viscosity parameter

\(k\) :

Permeability parameter

\(\rho = \frac{{{\text{kg}}}}{{{\text{m}}^{3} }}\) :

Fluid density

\({\text{Sr}} = \left( {\frac{{\rho \left( {T_{1} - T_{0} } \right)DK_{T} }}{{\mu T_{m} \left( {C_{1} - C_{0} } \right)}}} \right)\) :

Soret number

\(\mu\) :

Fluid viscosity

\(\rho C_{{\text{p}}}\) :

Specific heat constant

\(D_{{\text{m}}}\) :

Diffusion constant

\(T_{{\text{m}}}\) :

Mean temperature

\(\kappa = \frac{{\text{W}}}{{{\text{m}}.{\text{k}}}}\) :

Thermal conductivity

\(\left( {\beta ,\beta_{1} ,\beta_{2} } \right)\) :

Slip parameters

\(\phi\) :

Concentration in dimensionless form

\(\left( {C_{0} ,C_{1} } \right)\) :

Concentration at lower and upper wall, respectively

\(B = \left( {0,0,B_{0} } \right)\) :

Magnetic field

\(\delta = \left( {\frac{d}{\lambda }} \right)\) :

Wave number

\({\text{Re}} = \frac{\rho cd}{\mu }\) :

Reynolds number

\(\lambda = \frac{{\overline{\mu }_{{\text{c}}} c}}{\mu d}\) :

Fluid parameter

\(M^{2} = \frac{{\sigma B_{0}^{2} d^{2} }}{\mu }\) :

Hartmann number

\(D_{{\text{a}}} = \frac{{k_{0} }}{{d^{2} }}\) :

Darcy resistance parameter

\({\text{Pr}} = \left( {\frac{{\mu C_{{\text{p}}} }}{\kappa }} \right)\) :

Prandtl number

\({\text{Sc}} = \left( {\frac{\mu }{\rho D}} \right)\) :

Schmidt number

References

  • Abd-Alla AM, Abo-Dahab SM (2015) Magnetic field and rotation effects on peristaltic transport of a Jeffrey fluid in an asymmetric channel. J Magn Mag Mater 374:680–689

    Article  ADS  CAS  Google Scholar 

  • Akbar NS, Nadeem S (2010) Simulation of heat and chemical reactions on Reiner Rivlin fluid model for blood flow through tapered artery with stenosis. Heat Mass Trans 46:531–539

    Article  ADS  Google Scholar 

  • Akbar NS, Nadeem S, Mekheimer KhS (2016) Rheological properties of Reiner Rivlin fluid model for blood flow through tapered artery with stenosis. J Egyp Mathe Soc 24:138–142

    Article  MathSciNet  Google Scholar 

  • Alsaedi A, Nisar Z, Hayat T, Ahmad B (2021) Analysis of mixed convection and Hall current for MHD peristaltic transport of nanofluid with complaint wall. Inter Comm Heat Mass Trans 121:105121

    Article  CAS  Google Scholar 

  • Asghar Z (2023) Enhancing motility of micro-swimmers via electric and dynamical interactions effects. Euro Phys J plus. https://doi.org/10.1140/epjp/s13360-023-03963-w

    Article  Google Scholar 

  • Asghar Z, Ali N, Javid K, Waqas M, Khan WA (2021) Dynamical interaction effects on soft-bodied organisms in a multi-sinusoidal passage. Eur Phys J plus 693:10. https://doi.org/10.1140/epjp/s13360-021-01669-5

    Article  Google Scholar 

  • Asghar Z, Waqas M, Gondal MA, Khan WA (2022) Electro-osmotically driven generalized Newtonian blood flow in a divergent micro-channel. Alex Eng J 61:4519–4528

    Article  Google Scholar 

  • Asghar Z, Khan MWS, Gondal MA, Ghaffari A (2023) Magnet hydrodynamic flow of Carreau Yasuda fluid inside a complex wavy passage formed by beating cilia. Proc Inst Mech Eng Part E. https://doi.org/10.1177/09544089231171037

    Article  Google Scholar 

  • Attia HA (2007) The effect of ion slip on the flow of Reiner Rivlin fluid due to rotating disk with heat transfer. J Mech Sci Tech 43:174–183

    Article  Google Scholar 

  • Burns JC, Parkes T (1967) Peristaltic motion. J Fluid Mech 29:731–743

    Article  ADS  Google Scholar 

  • El Shehawey EF, El Sebaei W (2000) Peristaltic transport in a cylindrical tube through a porous medium. Inter J Math Mathe Sci 24:265874

    MathSciNet  Google Scholar 

  • Eldabe NT, Elogail MA, Elshaboury SM, Hasan AA (2016) Hall effects on the peristaltic transport of Williamson fluid through a porous medium with heat and mass trans. App Math Mod 40:315–328

    Article  Google Scholar 

  • Forbes LK (2018) Steady flow of Reiner Rivlin fluid between rotating plates. Phys Fluids 30:103104

    Article  ADS  Google Scholar 

  • Fung YC, Yin F (1968) Peristaltic transport. J App Mech 35:669–675

    Article  Google Scholar 

  • Gad NS (2014) Effects of Hall currents on peristaltic transport with complaint walls. Appl Math Comp 235:546–554

    Article  Google Scholar 

  • Hasan MM, Samad MA, Hossain MM (2020) Effects of Hall current and Ohmic heating on non-Newtonian fluid flow in a channel due to peristaltic wave. App Mathe 11:292–306

    Article  Google Scholar 

  • Hayat T, Shafique M, Tanveer A, Alsaedi A (2017) Slip and joule heating effects on radiative peristaltic flow of hyperbolic tangent nano-fluid. J Heat Mass Transf 112:559–567

    Article  CAS  Google Scholar 

  • Hayat T, Nisar Z, Alsaedi A (2020) Impacts of slip in radiative MHD peristaltic flow of fourth-grade nanomaterial with chemical reaction. Int Comm Heat Mass Transf 119:104976

    Article  CAS  Google Scholar 

  • Hina S, Yasin M (2018) Slip effects on peristaltic flow of magnetohydrodynamics second grade fluid through a flexible channel with heat/mass transfer. J Thermal Sci Eng App 10:051002

    Article  Google Scholar 

  • Hina S, Hayat T, Alsaedi A (2013) Slip effects on MHD peristaltic motion with heat and mass transfer. Arab J Sci Eng 39:593–603

    Article  MathSciNet  Google Scholar 

  • Javid K, Ali N, Khan S (2019) Numerical study of Hall effects on the peristaltically induced motion of a viscous fluid through a non-uniform regime: an application to the medical science. Eur Phys J plus 134:395

    Article  Google Scholar 

  • Khabazi NP, Taghavi SM, Sadegy K (2016) The peristaltic flow of Bingham fluid at large Reynolds number: a numerical study. J Non Newton Fluid Mech 227:20–34

    Article  MathSciNet  Google Scholar 

  • Kothandapani M, Srinivas S (2008) On the influence of wall properties in the MHD peristaltic transport with heat transfer and porous medium. Phys Lett A 372:4586–4591

    Article  ADS  CAS  Google Scholar 

  • Kothandapani M, Prakash J, Srinivas S (2015) Peristaltic transport of a MHD Carreau fluid in a tapered asymmetric channel with permeable walls. Int J Biomathe 8:1550054

    Article  MathSciNet  Google Scholar 

  • Latham TW (1966) Fluid motion in peristaltic pumps. MS. Thesis, MIT, Cambridge, MA

  • Noreen S, Kausar K (2019) Hall, slip and Ohmic heating effects in the thermally active sinusoidal channel. Prop Power Res 8:263–273

    Google Scholar 

  • Rafiq T, Mustafa M, Ahmad J (2022) Rotationally symmetric flow of Reiner Rivlin fluid over heated porous wall using numerical approach. Pro Inst Mech Eng J Mech Eng Sci 236:2803–2814

    Article  Google Scholar 

  • Rani J, Hina S, Mustafa M (2020) A novel formulation for MHD slip flow of Elastico-viscous fluid induced by peristaltic waves with heat/Mass transfer effects. Arab J Sci Eng 45:9213–9225

    Article  CAS  Google Scholar 

  • Rathod VP, Channakote MM (2011) A study of ureteral peristalsis in cylindrical tube through porous medium. Adv Appl Sci Res 2:134–140

    CAS  Google Scholar 

  • Reiner M (1945) A mathematical theory of dilatancy. Am J Math Soc 67:350–362

    Article  ADS  MathSciNet  Google Scholar 

  • Rivlin RS (1948) The hydrodynamics of non-Newtonian fluids. I Proc Roy Soc Lond A 193:260–281

    Article  ADS  MathSciNet  Google Scholar 

  • Srinivas S, Muthuraj R (2011) Effects of chemical reactions and space porosity on MHD mixed convective flow in a vertical asymmetric channel with peristalsis. Math Comp Mod 54:1213–1227

    Article  MathSciNet  Google Scholar 

Download references

Funding

The authors received no financial support for this research and for publication of this article.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics and Statistics, Institute of Space Technology 2750, Islamabad, 44000, Pakistan

    M. Yasin & R. Naz

  2. Department of Mathematical Sciences, Fatima Jinnah Women University, Rawalpindi, 46000, Pakistan

    S. Hina

Authors
  1. M. Yasin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Hina
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. R. Naz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Hina.

Ethics declarations

Conflict of interest

The authors have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$ H = \left( {\frac{{M^{2} }}{{1 + m^{2} + \frac{1}{{{\text{Da}}}}}}} \right); L = H^{2} \eta \left( {{\text{BesselY}}\left[ {1,H\eta } \right] + H\beta {\text{BesselY}}\left[ {0,H\eta } \right]} \right), {\text{L}}_{1} = - {\text{BesselJ}}\left[ {1,H\eta } \right] $$
$$ L_{2} = H\beta BesselJ\left[ {0,H\eta } \right], L_{3} = \left( {BesselY\left[ {1,H\eta } \right] + H\beta BesselY\left[ {0,H\eta } \right]} \right), $$
$$ L_{4} = \eta BesselY\left[ {1,H\eta } \right] - H\beta \eta BesselY\left[ {0,H\eta } \right], L_{5} = L_{3} H\eta^{3} BesselJ\left[ {2,H\eta } \right], $$
$$ \frac{{\partial p_{0} }}{\partial z} = - \frac{{QL - L_{5} }}{{\eta L_{4} }}, L{\prime} = H^{3} \beta \eta \left( { - HBesselY\left[ {1,H\eta } \right] + BesselY\left[ {0,H\eta } \right]\left( {\beta + \eta } \right)} \right)\eta^{\prime}, $$
$$ L_{1}{\prime} = \frac{1}{2}H\eta^{\prime}\left( { - BesselJ\left[ {0,H\eta } \right] + BesselJ\left[ {2,H\eta } \right]} \right), L_{2}{\prime} = - H^{2} \beta \eta^{\prime}BesselJ\left[ {1,H\eta } \right], $$
$$ L_{3}{\prime} = - \frac{1}{2}H\left( { - BesselY\left[ {0,H\eta } \right] + BesselY\left[ {2,H\eta } \right] + 2H\beta \eta^{\prime}BesselY\left[ {1,H\eta } \right]} \right), $$
$$ L_{4}{\prime} = H\left( {HBesselY\left[ {1,H\eta } \right]\beta \eta + BesselY\left[ {0,H\eta } \right]\left( { - \beta + \eta } \right)} \right)\eta^{\prime}, $$
$$ L_{5}{\prime} = H\eta^{2} \left( {BesselJ\left[ {2,H\eta } \right]\eta L_{3}{\prime} + L_{3} \left( {BesselJ\left[ {2,H\eta } \right] + H\eta BesselJ\left[ {1,H\eta } \right]} \right)\eta^{\prime}} \right), $$
$$ L_{7} = H^{2} \left( { - BesselJ\left[ {0,H\eta } \right] + H\beta BesselJ\left[ {1,H\eta } \right]} \right), L_{8} = BesselJ\left[ {0,H\eta } \right] - H\beta BesselJ\left[ {1,H\eta } \right], $$
$$ L_{9} = \left( { - 1 + H^{2} \eta \beta } \right), L_{10} = - BesselJ\left[ {0,H\eta } \right] + H\beta BesselJ\left[ {1,H\eta } \right], $$
$$ \frac{{\partial^{2} p}}{{\partial z^{2} }} = \frac{{\left( {QL - L_{5} } \right)\eta L_{4}{\prime} + L_{4} *\left( {\eta *\left( { - QL^{\prime} + L_{5}{\prime} } \right) + \left( {QL - L_{5} } \right)\eta^{\prime}} \right)}}{{ L_{4}^{2} *\eta^{2} }},\frac{{\partial p_{1} }}{\partial z} = \frac{{2QH L_{7} - L_{11} }}{{ L_{12} }}, $$
$$ L_{11} = 2A_{1} \eta \left( {H L_{8} \eta + 2 L_{9} BesselJ\left[ {1,H\eta } \right]} \right), L_{12} = \eta \left( {H L_{10} \eta + 2BesselJ\left[ {1,H\eta } \right]} \right), $$
$$ A_{1} = \frac{1}{{L^{3} }}Hr\left( {H^{2} L_{3} \eta BesselJ\left[ {0,Hr} \right] + BesselY\left[ {0,Hr} \right]\left( {H^{2} \left( { L_{1} - L_{2} } \right)\eta + \frac{{\partial p_{0} }}{\partial z}} \right)} \right) \times $$
$$ \left( {H^{2} rBesselJ\left[ {1,Hr} \right]\left( {L L_{3} \eta^{\prime} - L_{3} \eta L^{\prime} + L\eta L_{3}{\prime} } \right) + L_{4} L^{\prime}\frac{{\partial p_{0} }}{\partial z} - L L_{4}{\prime} \frac{{\partial p_{0} }}{\partial z} - L L_{4} \frac{{\partial^{2} p}}{{\partial z^{2} }} + rBesselY\left[ {1,Hr} \right]\left( { - L^{\prime}\left( {H^{2} \left( { L_{1} - L_{2} } \right)\eta + \frac{{\partial p_{0} }}{\partial z}} \right) + L\left( {H^{2} \left( { L_{1} \eta^{\prime} - L_{2} \eta^{\prime} + \eta L_{1}{\prime} - \eta L_{2}{\prime} } \right) + \frac{{\partial^{2} p}}{{\partial z^{2} }}} \right)} \right)} \right), $$
$$ B_{1} = \frac{Br}{{L^{2} \left( {1 + m^{2} } \right)}}\left( {H^{2} Mr\eta \left( { L_{3} BesselJ\left[ {1,Hr} \right] + \left( { L_{1} - L_{2} } \right)BesselY\left[ {1,Hr} \right]} \right) + M\left( { - L_{4} + rBesselY\left[ {1,Hr} \right]} \right)\frac{{\partial p_{0} }}{\partial z}} \right)^{2} , $$
$$ + \frac{Br}{{L^{2} }}\left( {H^{3} L_{3} r\eta BesselJ\left[ {0,Hr} \right] + HrBesselY\left[ {0,Hr} \right]\left( {H^{2} \left( { L_{1} - L_{2} } \right)\eta + \frac{{\partial p_{0} }}{\partial z}} \right)} \right)^{2} $$
$$ B_{11} = - \frac{2}{{L* L_{7}^{3} \left( {1 + m^{2} } \right)}}Br^{2} Hr(H^{2} L_{3} \eta BesselJ\left[ {0,Hr\left] { + BesselY} \right[0,Hr} \right](H^{2} \left( { L_{1} - L_{2} } \right)\eta $$
$$ + \frac{{\partial p_{0} }}{\partial z}))\left( {2M\left( { L_{8} + L_{9} BesselJ\left[ {0,Hr} \right]} \right)A_{1} + M\left( { L_{10} + BesselJ\left[ {0,Hr} \right]} \right)\frac{{\partial p_{1} }}{\partial z}} \right)^{2} \left( { - L_{7} A_{1} + HBesselJ\left[ {1,Hr} \right]\left( {2 L_{9} A_{1} + \frac{{\partial p_{1} }}{\partial z}} \right)} \right). $$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yasin, M., Hina, S. & Naz, R. Influence of Hall and Slip on MHD Reiner-Rivlin blood flow through a porous medium in a cylindrical tube. Soft Comput 28, 2799–2810 (2024). https://doi.org/10.1007/s00500-023-09538-2

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-023-09538-2

Keywords

Search

Navigation