Left residuated lattices induced by lattices with a unary operation

Abstract

In a previous paper, the authors defined two binary term operations in orthomodular lattices such that an orthomodular lattice can be organized by means of them into a left residuated lattice. It is a natural question if these operations serve in this way also for more general lattices than the orthomodular ones. In our present paper, we involve two conditions formulated as simple identities in two variables under which this is really the case. Hence, we obtain a variety of lattices with a unary operation which contains exactly those lattices with a unary operation which can be converted into a left residuated lattice by use of the above mentioned operations. It turns out that every lattice in this variety is in fact a bounded one and the unary operation is a complementation. Finally, we use a similar technique by using simpler terms and identities motivated by Boolean algebras.

It was recently shown by the authors in Chajda and Länger (2017a, b) that every orthomodular lattice $\mathbf L$ can be converted into a left residuated lattice, i.e., there exist binary operations $\otimes$ and $\rightarrow$ such that $x\otimes 1\approx 1\otimes x\approx x$ and the so-called left adjointness is satisfied by $\otimes$ and $\rightarrow$. It is known that orthomodular lattices were used in the 1930s by Husimi (1937) and Birkhoff and Von Neumann (1936) as an algebraic axiomatization of the semantics of the logic of quantum mechanics. However, it was recognized later that this axiomatization is not precise since e.g., joins of elements in these ordered sets need not exist. This was the reason why so-called orthomodular posets were introduced. Because the precise algebraic axiomatization is not known until now, it motivates us to study more general lattices than orthomodular ones which, however, formally satisfy the law of orthomodularity which is the keystone in this theory. However, we do not demand additional conditions on the unary operation, i.e., neither complementarity nor antitone involution is supposed. The aim of this paper is to get reasonable sufficient conditions under which this lattice can be converted into a left residuated lattice.

We start with the following definition.

FormalPara Definition 1

An algebra $\mathbf L=(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ of type (2, 2, 2, 2, 0, 0) is called a left residuated lattice [see Chajda and Länger (2017a)] if

• (i) $(L,\vee ,\wedge ,0,1)$ is a bounded lattice,

• (ii) $x\otimes 1\approx 1\otimes x\approx x$,

• (iii) for arbitrary $x,y,z\in L$, $x\otimes y\le z$ if and only if $x\le y\rightarrow z$.

Condition (iii) is called left adjointness. If, moreover, $\otimes$ is commutative and associative then $\mathbf L$ is called a residuated lattice. Given a left residuated lattice, we define $x^{\prime }:=x\rightarrow 0$ for all $x\in L$.

Throughout this paper, we assume that all lattices are non-empty.

The following concepts were introduced by the authors in Chajda and Länger (2018):

A lattice $\mathbf L=(L,\vee ,\wedge ,{}^{\prime })$ with a unary operation is called weakly orthomodular if

$$\begin{aligned} x\le y\text { implies }y=x\vee (y\wedge x^{\prime }), \end{aligned}$$

and dually weakly orthomodular if

$$\begin{aligned} x\le y\text { implies }x=y\wedge (x\vee y^{\prime }). \end{aligned}$$

It should be remarked that every of the aforementioned conditions can be easily rewritten as identities. Thus, weakly orthomodular lattices as well as dually weakly orthomodular lattices form a variety. The properties of these varieties were studied in Chajda and Länger (2018).

Let us note that every orthomodular lattice [see e.g., Beran (1985)] is both weakly and dually weakly orthomodular and that its unary operation is both an antitone involution and a complementation. However, when defining weak orthomodularity or dually weak orthomodularity, we neither ask the unary operation to be an antitone involution nor a complementation. Moreover, we do not assume the existence of a least or greatest element. The following was also shown in Chajda and Länger (2018).

FormalPara Remark 2

If $\mathbf L$ is weakly orthomodular then

$$\begin{aligned} x\le x\vee y=y\vee ((x\vee y)\wedge y^{\prime })\le y\vee y^{\prime } \end{aligned}$$

for all $x,y\in L$, and hence, $\mathbf L$ has a greatest element 1 and satisfies the identity $x\vee x^{\prime }\approx 1$. If $\mathbf L$ is dually weakly orthomodular then

$$\begin{aligned} y\wedge y^{\prime }\le y\wedge ((x\wedge y)\vee y^{\prime })=x\wedge y\le x \end{aligned}$$

for all $x,y\in L$, and hence, $\mathbf L$ has a smallest element 0 and satisfies the identity $x\wedge x^{\prime }\approx 0$. This means that if $\mathbf L$ is both weakly and dually weakly orthomodular then $\mathbf L$ is bounded and the unary operation $^{\prime }$ is a complementation, i.e., the identities $x\vee x^{\prime }\approx 1$ and $x\wedge x^{\prime }\approx 0$ are satisfied. Contrary to the case of orthomodular lattices, this complementation need not be an antitone involution.

The following examples are taken from Bonzio and Chajda (2017) and Chajda and Länger (2018).

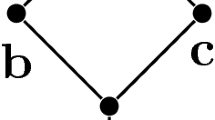
FormalPara Example 3

The lattice with the Hasse diagram depicted in Fig. 1 and the unary operation defined by

Fig. 1

A weakly orthomodular lattice

$$\begin{aligned} \begin{array}{c|ccccccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad d &{}\quad e &{}\quad f &{}\quad g &{}\quad 1 \\ \hline x^{\prime } &{}\quad 1 &{}\quad g &{}\quad g &{}\quad f &{}\quad e &{}\quad d &{}\quad c &{}\quad b &{}\quad 0 \end{array} \end{aligned}$$

is weakly orthomodular since

$$\begin{aligned} a\vee (a^{\prime }\wedge e)=a\vee (g\wedge e)=a\vee c=e \end{aligned}$$

(the remaining conditions obviously hold), but not dually weakly orthomodular since

$$\begin{aligned} e\wedge (a\vee e^{\prime })=e\wedge (a\vee d)=e\wedge 1=e\ne a \end{aligned}$$

and hence not orthomodular. Observe that $^{\prime }$ is an antitone complementation, but not an involution (for instance, $a''=g^{\prime }=b\ne a$).

FormalPara Example 4

The lattice with the Hasse diagram depicted in Fig. 2 and the unary operation defined by

Fig. 2

A dually weakly orthomodular lattice

$$\begin{aligned} \begin{array}{c|ccccccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad d &{}\quad e &{}\quad f &{}\quad g &{}\quad 1 \\ \hline x^{\prime } &{}\quad 1 &{}\quad f &{}\quad e &{}\quad d &{}\quad c &{}\quad b &{}\quad a &{}\quad a &{}\quad 0 \end{array} \end{aligned}$$

is dually weakly orthomodular since

$$\begin{aligned} g\wedge (c\vee g^{\prime })=g\wedge (c\vee a)=g\wedge e=c \end{aligned}$$

(the remaining conditions obviously hold), but not weakly orthomodular since

$$\begin{aligned} c\vee (c^{\prime }\wedge g)=c\vee (d\wedge g)=c\vee 0=c\ne g \end{aligned}$$

and hence not orthomodular. Observe that $^{\prime }$ is an antitone complementation, but not an involution.

Now, we define two binary term operations for lattices $\mathbf L=(L,\vee ,\wedge ,{}^{\prime })$ with a unary operation as follows:

$$\begin{aligned}&x\otimes y :=(x\vee y^{\prime })\wedge y\quad \text { for all }\;x,y\in L, \end{aligned}$$

(1)

$$\begin{aligned}&x\rightarrow y :=(x\wedge y)\vee x^{\prime }\quad \text { for all }\;x,y\in L. \end{aligned}$$

(2)

(It should be remarked that the unary operation $^{\prime }$ is not assumed to satisfy further properties.) These operations coincide with those defined in Chajda and Länger (2017b) within orthomodular lattices. If $(L,\vee ,\wedge )$ has a smallest element 0 then the identity $x\rightarrow 0\approx x^{\prime }$ holds. Moreover, we consider the following two identities for lattices with a unary operation:

$$\begin{aligned}&x\wedge (((x\vee y^{\prime })\wedge y)\vee y^{\prime }) \approx x, \end{aligned}$$

(3)

$$\begin{aligned}&x\vee (((x\wedge y)\vee y^{\prime })\wedge y) \approx x. \end{aligned}$$

(4)

Of course, these identities can be expressed in form of inequalities as follows:

• (3) $x\le ((x\vee y^{\prime })\wedge y)\vee y^{\prime }$,

• (4) $x\ge ((x\wedge y)\vee y^{\prime })\wedge y$.

FormalPara Lemma 5

Let $\mathbf L=(L,\vee ,\wedge ,{}^{\prime })$ be a lattice with a unary operation.

• (i) Assume $\mathbf L$ to satisfy identity (3). Then, $\mathbf L$ has a greatest element 1 and satisfies the identity $x\vee x^{\prime }\approx 1$. If, moreover, $\mathbf L$ has a smallest element 0 then it satisfies the identity $0'\approx 1$.

• (ii) Assume $\mathbf L$ to satisfy identity (4). Then, $\mathbf L$ has a smallest element 0 and satisfies the identity $x\wedge x^{\prime }\approx 0$. If, moreover, $\mathbf L$ has a greatest element 1 then it satisfies the identity $1'\approx 0$.

• (iii) Assume $\mathbf L$ to satisfy both identities (3) and (4). Then, $\mathbf L$ is bounded, $'$ a complementation of $\mathbf L$ and, moreover, $'$ is switching, i.e., the identities $0'\approx 1$ and $1'\approx 0$ are satisfied.

FormalPara Proof

• (i) Since $(x\vee y^{\prime })\wedge y\le y$, we have $x\le y\vee y^{\prime }$ for all $x,y\in L$, and hence, $\mathbf L$ has a greatest element 1 and satisfies the identity $x\vee x^{\prime }\approx 1$. If, moreover, $\mathbf L$ has a smallest element 0 then

  $$\begin{aligned} x=x\wedge (((x\vee 0^{\prime })\wedge 0)\vee 0^{\prime })=x\wedge 0^{\prime }\le 0^{\prime } \end{aligned}$$

  for all $x\in L$, i.e., $\mathbf L$ satisfies the identity $0'\approx 1$.

• (ii) Since $y'\le (x\wedge y)\vee y'$, we have $y'\wedge y\le x$ for all $x,y\in L$, and hence, $\mathbf L$ has a smallest element 0 and satisfies the identity $x\wedge x'\approx 0$. If, moreover, $\mathbf L$ has a greatest element 1 then

  $$\begin{aligned} 1'\le x\vee 1'=x\vee (((x\wedge 1)\vee 1')\wedge 1)=x \end{aligned}$$

  for all $x\in L$, i.e., $\mathbf L$ satisfies the identity $1'\approx 0$.

• (iii) follows from (i) and (ii).$\square$

In the following, let $\mathcal V$ denote the variety of all algebras $(L,\vee ,\wedge ,{}',0,1)$ of type (2, 2, 1, 0, 0) such that $(L,\vee ,\wedge ,0,1)$ is a bounded lattice and $(L,\vee ,\wedge ,{}')$ satisfies both identities (3) and (4).

If $(L,\vee ,\wedge )$ is a lattice having a smallest element 0, but no greatest element and $x':=0$ for all $x\in L$ then $(L,\vee ,\wedge ,{}')$ satisfies (4), but not (3). If, conversely, $(L,\vee ,\wedge )$ is a lattice having a greatest element 1, but no smallest element and $x':=1$ for all $x\in L$ then $(L,\vee ,\wedge ,{}')$ satisfies (3), but not (4). This shows that (3) and (4) are independent.

Using the operations $\otimes$ and $\rightarrow$ defined by (1) and (2), identities (3) and (4) can be rewritten as follows:

• (3) $x\wedge ((x\otimes y)\vee y')\approx x$ or $x\wedge (y\rightarrow (x\vee y'))\approx x$,

• (4) $x\vee ((x\wedge y)\otimes y)\approx x$ or $x\vee ((y\rightarrow x)\wedge y)\approx x$,

respectively.

Lattices with a unary operation satisfying (3) or (4) are not curious.

FormalPara Remark 6

Assume $\mathbf L=(L,\vee ,\wedge ,{}')$ to be a modular lattice with a unary operation. If $\mathbf L$ has a greatest element 1 and satisfies the identity $x\vee x'\approx 1$ then it satisfies identity (3) since

$$\begin{aligned} x\le x\vee y'= & {} 1\wedge (x\vee y')=(y'\vee y)\wedge (x\vee y')\\= & {} y'\vee (y\wedge (x\vee y'))=((x\vee y')\wedge y)\vee y' \end{aligned}$$

for all $x,y\in L$. If $\mathbf L$ has a smallest element 0 and satisfies the identity $x\wedge x'\approx 0$ then it satisfies identity (4) since

$$\begin{aligned} ((x\wedge y)\vee y')\wedge y=(x\wedge y)\vee (y'\wedge y)=(x\wedge y)\vee 0=x\wedge y\le x \end{aligned}$$

for all $x,y\in L$. Since every finite distributive lattice is dually pseudocomplemented, it satisfies identity (3) with the dual pseudocomplementation as unary operation. Since every finite distributive lattice is pseudocomplemented, it satisfies identity (4) with the pseudocomplementation as unary operation.

The following examples show two typical members of $\mathcal V$. The first one is even modular.

FormalPara Example 7

The modular lattice with the Hasse diagram depicted in Fig. 3 and the unary operation defined by

Fig. 3

A modular member of $\mathcal V$

$$\begin{aligned} \begin{array}{c|ccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad 1 \\ \hline x' &{}\quad 1 &{}\quad c &{}\quad c &{}\quad a &{}\quad 0 \end{array} \end{aligned}$$

belongs to $\mathcal V$.

FormalPara Example 8

The non-modular lattice with the Hasse diagram depicted in Fig. 4 and the unary operation defined by

Fig. 4

A non-modular member of $\mathcal V$

$$\begin{aligned} \begin{array}{c|ccccccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad d &{}\quad e &{}\quad f &{}\quad g &{}\quad 1 \\ \hline x' &{}\quad 1 &{}\quad g &{}\quad f &{}\quad e &{}\quad e &{}\quad c &{}\quad b &{}\quad a &{}\quad 0 \end{array} \end{aligned}$$

belongs to $\mathcal V$.

In the following, we investigate connections between the identities (3) and (4) and left adjointness.

FormalPara Lemma 9

Let $\mathbf L=(L,\vee ,\wedge ,{}')$ be a lattice with a unary operation, $a,b,c\in L$ and $\otimes$ and $\rightarrow$ defined by (1) and (2), respectively. Then the following hold:

• (i) If $\mathbf L$ satisfies identity (3) then $a\otimes b\le c$ implies $a\le b\rightarrow c$,

• (ii) if $\mathbf L$ satisfies identity (4) then $a\le b\rightarrow c$ implies $a\otimes b\le c$.

FormalPara Proof

We have

• (i) $a\le ((a\vee b')\wedge b)\vee b'=(b\wedge (a\vee b')\wedge b)\vee b'\le (b\wedge c)\vee b'=b\rightarrow c$ and

• (ii) $a\otimes b=(a\vee b')\wedge b\le ((b\wedge c)\vee b'\vee b')\wedge b=((c\wedge b)\vee b')\wedge b\le c$.$\square$

Using these results, we can characterize the class of all lattices with a unary operation which can be organized into left residuated lattices via the operations $\otimes$ and $\rightarrow$ defined by (1) and (2), respectively.

FormalPara Theorem 10

Let $(L,\vee ,\wedge ,{}')$ be a lattice with a unary operation and $\otimes$ and $\rightarrow$ defined by (1) and (2), respectively. Then, $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice satisfying $x\rightarrow 0\approx x'$ if and only if $(L,\vee ,\wedge ,{}',0,1)\in \mathcal V$.

FormalPara Proof

Let $a,b\in L$. If $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice then $(L,\vee ,\wedge ,0,1)$ is a bounded lattice, since $a\otimes b\le a\vee b'$ we have $a\le b\rightarrow (a\vee b')$, i.e., identity (3) is satisfied, and because of $a\wedge b\le b\rightarrow a$ we have $(a\wedge b)\otimes b\le a$, i.e., identity (4) is satisfied which shows $(L,\vee ,\wedge ,{}',0,1)\in \mathcal V$. Conversely, assume $(L,\vee ,\wedge ,{}')$ to satisfy both identities (3) and (4). According to Lemma 5, $(L,\vee ,\wedge )$ is bounded and $'$ a complementation on it. Hence, $(L,\vee ,\wedge ,{}',0,1)\in \mathcal V$ and we have

$$\begin{aligned} x\otimes 1&\approx (x\vee 1')\wedge 1\approx x\text { and} \\ 1\otimes x&\approx (1\vee x')\wedge x\approx x. \end{aligned}$$

The rest follows from Lemma 9.$\square$

The importance of this approach is that residuated lattices serve as an algebraic semantics of certain substructural logics. Hence, this is the link between orthomodular, weakly orthomodular and dually weakly orthomodular lattices on one side and kinds of fuzzy logics on the other side.

In the following, we investigate connections between the identities (3) and (4) and (dually) weak orthomodularity.

FormalPara Lemma 11

Let $\mathbf L=(L,\vee ,\wedge ,{}')$ be a lattice with a unary operation. Then the following hold:

• (i) If $\mathbf L$ satisfies the identity $x''\approx x$ then identity (3) is equivalent to weak orthomodularity of $\mathbf L$,

• (ii) identity (4) is equivalent to dually weak orthomodularity of $\mathbf L$.

FormalPara Proof

Let $a,b\in L$.

• (i) Assume $\mathbf L$ to satisfy the identity $x''\approx x$. If $\mathbf L$ satisfies identity (3) and $a\le b$ then

  $$\begin{aligned} b\le ((b\vee a'')\wedge a')\vee a''=a\vee (b\wedge a')\le b. \end{aligned}$$

  If, conversely, $\mathbf L$ is weakly orthomodular then

  $$\begin{aligned} a\le a\vee b'=b'\vee ((a\vee b')\wedge b'')=((a\vee b')\wedge b)\vee b'. \end{aligned}$$

• (ii) If $\mathbf L$ satisfies identity (4) and $a\le b$ then

  $$\begin{aligned} a\le b\wedge (a\vee b')=((a\wedge b)\vee b')\wedge b\le a. \end{aligned}$$

  If, conversely, $\mathbf L$ is dually weakly orthomodular then

  $$\begin{aligned} ((a\wedge b)\vee b')\wedge b=b\wedge ((a\wedge b)\vee b')=a\wedge b\le a.\square \end{aligned}$$

If the identity $x''\approx x$ is not satisfied then identity (3) need not be equivalent to weak orthomodularity as the following example shows:

FormalPara Example 12

The lattice with the Hasse diagram depicted in Fig. 5 and the unary operation defined by

Fig. 5

A lattice satisfying (3)

$$\begin{aligned} \begin{array}{c|ccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad 1 \\ \hline x' &{}\quad 1 &{}\quad b &{}\quad c &{}\quad b &{}\quad 0 \end{array} \end{aligned}$$

satisfies identity (3), but is not weakly orthomodular since

$$\begin{aligned} a\vee (c\wedge a')=a\vee (c\wedge b)=a\vee 0=a\ne c. \end{aligned}$$

Summarizing, we obtain the following result:

FormalPara Theorem 13

If $\mathbf L=(L,\vee ,\wedge ,{}')$ is a lattice with a unary operation satisfying the identity $x''\approx x$ and being both weakly and dually weakly orthomodular then $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice.

FormalPara Proof

This follows from Lemma 5, Theorem 10 and Lemma 11.$\square$

FormalPara Example 14

The lattice with a unary operation from Example 7 is both weakly and dually weakly orthomodular. However, if we define the unary operation as follows

$$\begin{aligned} \begin{array}{c|ccccc} x &{}\quad 0 &{}\quad a &{}\quad b &{}\quad c &{}\quad 1 \\ \hline x' &{}\quad 1 &{}\quad c &{}\quad c &{}\quad c &{}\quad 0 \end{array} \end{aligned}$$

then this lattice with a unary operation is neither weakly orthomodular since

$$\begin{aligned} 1\ne c=c\vee c=c\vee (1\wedge c)=c\vee (1\wedge c') \end{aligned}$$

nor dually weakly orthomodular since

$$\begin{aligned} 0\ne c=c\wedge c=c\wedge (0\vee c)=c\wedge (0\vee c') \end{aligned}$$

and it satisfies neither identity (3) since

$$\begin{aligned} 1\not \le c=c\vee c=(1\wedge c)\vee c=((1\vee c)\wedge c)\vee c=((1\vee c')\wedge c)\vee c' \end{aligned}$$

nor identity (4) because of Lemma 11.

The variety $\mathcal V$ has several important subvarieties, e.g., the variety of bounded complemented modular lattices (due to Remark 6), the variety $\mathcal O$ of orthomodular lattices and, of course, the variety of Boolean algebras. Since $\mathcal V\supseteq \mathcal O$, the variety $\mathcal V$ is residually large since for every infinite cardinal k there exists a subdirectly irreducible orthomodular lattice of cardinality k [(cf. Chajda and Länger (2020)].

In the lattice from Example 14, we have $c\vee c'\ne 1$. We show that this is just the condition needed to prove left adjointness provided the operation $\rightarrow$ is defined by (2).

FormalPara Theorem 15

Let $\mathbf L=(L,\vee ,\wedge ,{}',0,1)$ be a bounded lattice with a unary operation and $a\in L$ with $a\vee a'\ne 1$. Then there does not exist a binary operation $\otimes$ on L such that $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice where $\rightarrow$ is defined by (2).

FormalPara Proof

Assume there exists some binary operation $\otimes$ on L such that $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice. Because of $1\otimes a\le a$ we conclude $1\le a\rightarrow a=(a\wedge a)\vee a'=a\vee a'$, a contradiction.$\square$

However, also in the case that L contains an element a satisfying $a\vee a'\ne 1$, $\mathbf L$ may be converted into a left residuated lattice if the operations $\otimes$ and $\rightarrow$ are defined in an appropriate way, see the following example.

FormalPara Example 16

If $L=\{0,a,1\}$ is a three-element chain and $a'=a$ then the assumptions of Theorem 15 are satisfied. On the contrary, if one defines $\otimes$ and $\rightarrow$ by

$$\begin{aligned} \begin{array}{c|ccc} \otimes &{}\quad 0 &{}\quad a &{}\quad 1 \\ \hline 0 &{}\quad 0 &{}\quad 0 &{}\quad 0 \\ a &{}\quad 0 &{}\quad 0 &{}\quad a \\ 1 &{}\quad 0 &{}\quad a &{}\quad 1 \end{array} \quad \quad \quad \begin{array}{c|ccc} \rightarrow &{}\quad 0 &{}\quad a &{}\quad 1 \\ \hline 0 &{}\quad 1 &{}\quad 1 &{}\quad 1 \\ a &{}\quad a &{}\quad 1 &{}\quad 1 \\ 1 &{}\quad 0 &{}\quad a &{}\quad 1 \end{array} \end{aligned}$$

then $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a left residuated lattice.

It is well-known that a Boolean algebra $(B,\vee ,\wedge ,{}',0,1)$ can be converted into a residuated lattice when the operations $\otimes$ and $\rightarrow$ are the following term operations:

$$\begin{aligned}&x\otimes y :=x\wedge y\quad \text { for all }\;x,y\in L, \end{aligned}$$

(5)

$$\begin{aligned}&x\rightarrow y :=x'\vee y\quad \text { for all }\;x,y\in L. \end{aligned}$$

(6)

It is a natural question if the operations $\otimes$ and $\rightarrow$ defined in this way may convert a lattice $(L,\vee ,\wedge ,{}')$ with a unary operation into a residuated lattice. Similarly as in the previous part of the paper, we will consider the following two identities:

$$\begin{aligned} x\wedge ((x\wedge y)\vee y')&\approx x, \end{aligned}$$

(7)

$$\begin{aligned} x\vee ((x\vee y')\wedge y)&\approx x. \end{aligned}$$

(8)

Of course, these identities can be expressed in form of inequalities as follows:

• (7) $x\le (x\wedge y)\vee y'$,

• (8) $x\ge (x\vee y')\wedge y$.

One can easily check that if $\mathbf L$ is bounded and satisfies both identities (7) and (8) then $'$ is a complementation since

$$\begin{aligned} x\vee x'&\approx 1\wedge ((1\wedge x)\vee x')\approx 1, \\ x\wedge x'&\approx 0\vee ((0\vee x')\wedge x)\approx 0. \end{aligned}$$

If $\mathbf L$ is bounded then

$$\begin{aligned} x\otimes 1&\approx 1\otimes x\approx x, \\ x\rightarrow 0&\approx x'. \end{aligned}$$

Now, we can prove

FormalPara Lemma 17

Let $\mathbf L=(L,\vee ,\wedge ,{}')$ be a lattice with a unary operation, $a,b,c\in L$ and $\otimes$ and $\rightarrow$ defined by (5) and (6), respectively. Then the following hold:

• (i) If $\mathbf L$ satisfies identity (7) then $a\otimes b\le c$ implies $a\le b\rightarrow c$,

• (ii) if $\mathbf L$ satisfies identity (8) then $a\le b\rightarrow c$ implies $a\otimes b\le c$.

FormalPara Proof

We have

• (i) $a\le (a\wedge b)\vee b'=b'\vee (a\wedge b)\le b'\vee c=b\rightarrow c$ and

• (ii) $a\otimes b=a\wedge b\le (b'\vee c)\wedge b=(c\vee b')\wedge b\le c$.$\square$

From Lemma 17, we conclude

FormalPara Theorem 18

If $\mathbf L=(L,\vee ,\wedge ,{}',0,1)$ is a bounded lattice with a unary operation satisfying both identities (7) and (8) and $\otimes$ and $\rightarrow$ are defined by (5) and (6), respectively, then $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a residuated lattice.

Using these results, we can characterize the class of all bounded lattices with a unary operation which can be organized into residuated lattices via the operations $\otimes$ and $\rightarrow$ defined by (5) and (6), respectively.

FormalPara Theorem 19

Let $(L,\vee ,\wedge ,{}',0,1)$ be a bounded lattice with a unary operation and $\otimes$ and $\rightarrow$ defined by (5) and (6), respectively. Then, $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a residuated lattice satisfying $x\rightarrow 0\approx x'$ if and only if $(L,\vee ,\wedge ,{}')$ satisfies both identities (7) and (8).

FormalPara Proof

Let $a,b\in L$. If $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a residuated lattice satisfying $x\rightarrow 0\approx x'$ then $(L,\vee ,\wedge ,0,1)$ is a bounded lattice, since $a\otimes b\le a\wedge b$ we have $a\le b\rightarrow (a\wedge b)=(a\wedge b)\vee b'$, i.e., identity (7) is satisfied, and because of $b'\vee a\le b\rightarrow a$ we have $(a\vee b')\wedge b=(b'\vee a)\otimes b\le a$, i.e., identity (8) is satisfied. Conversely, if $(L,\vee ,\wedge ,{}')$ satisfies both identities (7) and (8) then $(L,\vee ,\wedge ,\otimes ,\rightarrow ,0,1)$ is a residuated lattice satisfying $x\rightarrow 0\approx x'$ according to Lemma 17.$\square$

FormalPara Corollary 20

The class $\mathcal C$ of bounded lattices with a unary operation $'$ satisfying the identity $x''\approx x$ which become residuated lattices with respect to the operations $\otimes$ and $\rightarrow$ defined by (5) and (6) coincides with the variety of Boolean algebras.

FormalPara Proof

According to Theorem 19, $\mathcal C$ satisfies both identities (7) and (8). From (7), we infer $x\le (x\wedge y)\vee y'$. Evidently, $y'\le (x\wedge y)\vee y'$. Together we obtain $x\vee y'\le (x\wedge y)\vee y'$. Since the converse inequality holds trivially, $\mathcal C$ satisfies the identity

$$\begin{aligned} x\vee y'\approx (x\wedge y)\vee y'. \end{aligned}$$

(9)

Further, from (8), we infer $y\ge (y\vee x'')\wedge x'$. Evidently, $x'\ge (y\vee x'')\wedge x'$. Together we obtain $x'\wedge y\ge x'\wedge (x''\vee y)$. Since the converse inequality holds trivially, $\mathcal C$ satisfies the identity $x'\wedge y\approx x'\wedge (x''\vee y)$ which, because of the identity $x''\approx x$, can be written in the form

$$\begin{aligned} x'\wedge y\approx x'\wedge (x\vee y). \end{aligned}$$

(10)

According to Chajda and Padmanabhan (2017), the identities (9) and (10) characterize the variety of Boolean algebras.$\square$

Recall that an algebra$\mathbf A$ is called congruence distributive if its congruence lattice is distributive, and $\mathbf A$ is called congruence permutable if $\Theta \circ \Phi =\Phi \circ \Theta$ for all congruences $\Theta ,\Phi$ on $\mathbf A$. A variety is called congruence distributive (congruence permutable) if any of its members has the corresponding property. For further information concerning these notions cf. Chajda et al. (2012).

Since $\mathcal V$ is a variety of lattices, it is congruence distributive. Let $\mathcal W$ denote the subvariety of $\mathcal V$ satisfying the additional identity

$$\begin{aligned} x\le (x\rightarrow y)\rightarrow y \end{aligned}$$

where $\rightarrow$ is defined by (2).

Then, we can prove even more.

FormalPara Theorem 21

The variety $\mathcal W$ is congruence permutable.

FormalPara Proof

Let p denote the term defined by

$$\begin{aligned} p(x,y,z):=((x\rightarrow y)\rightarrow z)\wedge ((z\rightarrow y)\rightarrow x) \end{aligned}$$

where the binary operation $\rightarrow$ is defined by (2). According to Lemma 5 we have

$$\begin{aligned} x\rightarrow x&\approx (x\wedge x)\vee x'\approx 1, \\ 1\rightarrow x&\approx (1\wedge x)\vee 1'\approx x. \end{aligned}$$

This shows

$$\begin{aligned} p(x,x,y)&\approx ((x\rightarrow x)\rightarrow y)\wedge ((y\rightarrow x)\rightarrow x)\\&\approx y\wedge ((y\rightarrow x)\rightarrow x)\approx y, \\ p(y,x,x)&\approx ((y\rightarrow x)\rightarrow x)\wedge ((x\rightarrow x)\rightarrow y)\\&\approx ((y\rightarrow x)\rightarrow x)\wedge y\approx y \end{aligned}$$

showing that p is a Maltsev term. Hence, $\mathcal W$ is congruence permutable.$\square$