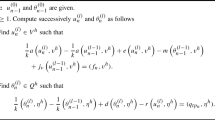Abstract
This paper presents an extension of Nitsche’s method to finite deformation thermomechanical contact problems including friction. The mechanical contact constraints, i.e. non-penetration and Coulomb’s law of friction, are introduced into the weak form using a stabilizing consistent penalty term. The required penalty parameter is estimated with local generalized eigenvalue problems, based on which an additional harmonic weighting of the boundary traction is introduced. A special focus is put on the enforcement of the thermal constraints at the contact interface, namely heat conduction and frictional heating. Two numerical methods to introduce these effects are presented, a substitution method as well as a Nitsche-type approach. Numerical experiments range from two-dimensional frictionless thermo-elastic problems demonstrating optimal convergence rates to three-dimensional thermo-elasto-plastic problems including friction.











Similar content being viewed by others
References
Annavarapu C, Hautefeuille M, Dolbow JE (2012) A robust Nitsches formulation for interface problems. Comput Method Appl M 225:44–54
Ball JM (1976) Convexity conditions and existence theorems in nonlinear elasticity. Arch Ration Mech Anal 63(4):337–403
Baumberger T, Berthoud P, Caroli C (1999) Physical analysis of the state-and rate-dependent friction law. ii. dynamic friction. Phys Rev B 60(6):3928
Burman E, Zunino P (2011) Numerical approximation of large contrast problems with the unfitted Nitsche method. In: Blowey J, Jensen M (eds) Frontiers in numerical analysis-Durham 2010 Lecture notes in computational science and engineering, vol 85. Springer, Berlin, pp 227–282
Chouly F (2014) An adaptation of Nitsches method to the Tresca friction problem. J Math Anal Appl 411(1):329–339
Chouly F, Hild P (2013) A Nitsche-based method for unilateral contact problems: numerical analysis. SIAM J Numer Anal 51(2):1295–1307
Chouly F, Hild P, Renard Y (2015) Symmetric and non-symmetric variants of Nitsches method for contact problems in elasticity: theory and numerical experiments. Math Comput 84(293):1089–1112
Cottrell JA, Hughes TJR, Bazilevs Y (2009) Isogeometric analysis: toward integration of CAD and FEA. Wiley, Hoboken
Curnier A, He QC, Klarbring A (1995) Continuum mechanics modelling of large deformation contact with friction. In: Raous M, Jean M, Moreau JJ (eds) Contact mechanics. Springer, Berlin, pp 145–158
Danowski C, Gravemeier V, Yoshihara L, Wall WA (2013) A monolithic computational approach to thermo-structure interaction. Int J Numer Methods Eng 95(13):1053–1078
De Saracibar CA (1998) Numerical analysis of coupled thermomechanical frictional contact problems. Computational model and applications. Arch Comput Method E 5(3):243–301
Dittmann M, Franke M, Temizer I, Hesch C (2014) Isogeometric analysis and thermomechanical mortar contact problems. Comput Method Appl M 274:192–212
Dolbow J, Harari I (2009) An efficient finite element method for embedded interface problems. Int J Numer Methods Eng 78(2):229–252
Farah P, Popp A, Wall WA (2015) Segment-based versus element-based integration for mortar methods in computational contact mechanics. Comput Mech 55(1):209–228
Gitterle M, Popp A, Gee MW, Wall WA (2010) Finite deformation frictional mortar contact using a semi-smooth Newton method with consistent linearization. Int J Numer Methods Eng 84(5):543–571
Griebel M, Schweitzer MA (2003) A particle-partition of unity method part V: boundary conditions. In: Hildebrandt S, Karcher H (eds) Geometric analysis and nonlinear partial differential equations. Springer, Berlin, pp 519–542
Hager C, Wohlmuth B (2009) Nonlinear complementarity functions for plasticity problems with frictional contact. Comput Method Appl M 198(41–44):3411–3427
Hansbo P (2005) Nitsche’s method for interface problems in computational mechanics. GAMM-Mitt 28(2):183–206
Hansen G (2011) A Jacobian-free Newton Krylov method for mortar-discretized thermomechanical contact problems. J Comput Phys 230(17):6546–6562
Holzapfel GA (2000) Nonlinear solid mechanics: a continuum approach for engineering. Wiley, Hoboken
Hüeber S, Stadler G, Wohlmuth BI (2008) A primal-dual active set algorithm for three-dimensional contact problems with Coulomb friction. J Sci Comput 30(2):572–596
Hüeber S, Wohlmuth BI (2005) A primal-dual active set strategy for non-linear multibody contact problems. Comput Method Appl M 194(27–29):3147–3166
Hüeber S, Wohlmuth BI (2009) Thermo-mechanical contact problems on non-matching meshes. Comput Method Appl M 198(15–16):1338–1350
Johansson L, Klarbring A (1993) Thermoelastic frictional contact problems: modelling, finite element approximation and numerical realization. Comput Method Appl M 105(2):181–210
Juntunen M, Stenberg R (2009) Nitsches method for general boundary conditions. Math Comput 78(267):1353–1374
Khoei A, Saffar H, Eghbalian M (2015) An efficient thermo-mechanical contact algorithm for modeling contact-impact problems. Asian J Civ Eng (Build Hous) 16(5):681–708
Laursen TA (2002) Computational contact and impact mechanics. Springer, Berlin
Mlika R, Renard Y, Chouly F (2017) An unbiased Nitsche’s formulation of large deformation frictional contact and self-contact. Comput Method Appl M 325:265–288
Nitsche J (1971) Über ein Variationsprinzip zur Lösung von Dirichlet-Problemen bei Verwendung von Teilräumen, die keinen Randbedingungen unterworfen sind. Abh Math Semin Univ Hambg 36(1):9–15
Oancea VG, Laursen TA (1997) A finite element formulation of thermomechanical rate-dependent frictional sliding. Int J Numer Methods Eng 40(23):4275–4311
Pantuso D, Bathe KJ, Bouzinov PA (2000) A finite element procedure for the analysis of thermo-mechanical solids in contact. Comput Struct 75(6):551–573
Popp A, Gee MW, Wall WA (2009) A finite deformation mortar contact formulation using a primal-dual active set strategy. Int J Numer Methods Eng 79(11):1354–1391
Popp A, Gitterle M, Gee MW, Wall WA (2010) A dual mortar approach for 3D finite deformation contact with consistent linearization. Int J Numer Methods Eng 83(11):1428–1465
Poulios K, Renard Y (2015) An unconstrained integral approximation of large sliding frictional contact between deformable solids. Comput Struct 153:75–90
Qi L, Sun J (1993) A nonsmooth version of Newton’s method. Math Program 58(1):353–367
Renard Y (2013) Generalized Newtons methods for the approximation and resolution of frictional contact problems in elasticity. Comput Method Appl M 256:38–55
Sauer RA, De Lorenzis L (2015) An unbiased computational contact formulation for 3D friction. Int J Numer Methods Eng 101(4):251–280
Seitz A, Farah P, Kremheller J, Wohlmuth BI, Wall WA, Popp A (2016) Isogeometric dual mortar methods for computational contact mechanics. Comput Method Appl M 301:259–280
Seitz A, Wall WA, Popp A (2018) A computational approach for thermo-elasto-plastic frictional contact based on a monolithic formulation using non-smooth nonlinear complementarity functions. Adv Model Simul Eng Sci 5(1):5
Simo JC, Miehe C (1992) Associative coupled thermoplasticity at finite strains: formulation, numerical analysis and implementation. Comput Method Appl M 98(1):41–104
de Souza Neto EA, Perić D, Dutko M, Owen DRJ (1996) Design of simple low order finite elements for large strain analysis of nearly incompressible solids. Int J Solids Struct 33(20–22):3277–3296
Temizer I (2014) Multiscale thermomechanical contact: computational homogenization with isogeometric analysis. Int J Numer Methods Eng 97(8):582–607
Temizer I, Wriggers P, Hughes T (2011) Contact treatment in isogeometric analysis with NURBS. Comput Method Appl M 200(9–12):1100–1112
Verdugo F, Wall WA (2016) Unified computational framework for the efficient solution of n-field coupled problems with monolithic schemes. Comput Method Appl M 310:335–366
Wall WA, Ager C, Grill M, Kronbichler M, Popp A, Schott B, Seitz A (2018) BACI: A multiphysics simulation environment. Institute for Computational Mechanics, Technical University of Munich, Tech. rep
Wiesner T, Popp A, Gee M, Wall W (2018) Algebraic multigrid methods for dual mortar finite element formulations in contact mechanics. Int J Numer Methods Eng 114(4):399–430
Winter M, Schott B, Massing A, Wall W (2018) A Nitsche cut finite element method for the Oseen problem with general Navier boundary conditions. Comput Method Appl M 330:220–252
Wohlmuth BI (2011) Variationally consistent discretization schemes and numerical algorithms for contact problems. Acta Numer 20:569–734
Wohlmuth BI, Popp A, Gee MW, Wall WA (2012) An abstract framework for a priori estimates for contact problems in 3D with quadratic finite elements. Comput Mech 49:735–747
Wriggers P, Laursen TA (2006) Computational contact mechanics, vol 30167. Springer, New York
Wriggers P, Miehe C (1994) Contact constraints within coupled thermomechanical analysis—a finite element model. Comput Method Appl M 113(3):301–319
Wriggers P, Zavarise G (2008) A formulation for frictionless contact problems using a weak form introduced by Nitsche. Comput Mech 41(3):407–420
Xing H, Makinouchi A (2002) Three dimensional finite element modeling of thermomechanical frictional contact between finite deformation bodies using R-minimum strategy. Comput Method Appl M 191(37):4193–4214
Zavarise G, Wriggers P, Stein E, Schrefler B (1992) Real contact mechanisms and finite element formulation—a coupled thermomechanical approach. Int J Numer Methods Eng 35(4):767–785
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: A Consistency of the thermal weak form using Nitsche’s method
Appendix: A Consistency of the thermal weak form using Nitsche’s method
To show the consistency of (39), i.e. equivalence of (39) with (30) in combination with (24) and (25), we first observe, that \(\bar{\beta }_c\) and \({\mathcal {P}}_\tau \) are, due to (31) and (33), consistent substitutes for \(\beta _c\vert p_n \vert \) and \(\varvec{{t}}_\tau \cdot \varvec{{v}}_\tau \) in (24) and (25). Next, (24) and (25) provide the following equivalences for weighted heat fluxes:
with which (39) can be re-written as
Next, let us take a closer look at the fifth and sixth line containing all terms tested with \( \left\{ q_c(\delta T)\right\} _{\omega _\vartheta }\):
What remains from (54) is
which is indeed equivalent to (30).
Rights and permissions
About this article
Cite this article
Seitz, A., Wall, W.A. & Popp, A. Nitsche’s method for finite deformation thermomechanical contact problems. Comput Mech 63, 1091–1110 (2019). https://doi.org/10.1007/s00466-018-1638-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00466-018-1638-x