Time-domain BEM for 3-D transient elastodynamic problems with interacting rigid movable disc-shaped inclusions

Abstract

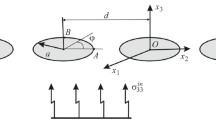
Formulation of time-domain boundary element method for elastodynamic analysis of interaction between rigid massive disc-shaped inclusions subjected to impinging elastic waves is presented. Boundary integral equations (BIEs) with time-retarded kernels are obtained by using the integral representations of displacements in a matrix in terms of interfacial stress jumps across the inhomogeneities and satisfaction of linearity conditions at the inclusion domains. The equations of motion for each inclusion complete the problem formulation. The time-stepping/collocation scheme is implemented for the discretization of the BIEs by taking into account the traveling nature of the generated wave field and local structure of the solution at the inclusion edges. Numerical results concern normal incidence of longitudinal wave onto two coplanar circular inclusions. The inertial effects are revealed by the time dependencies of inclusions’ kinematic parameters and dynamic stress intensity factors in the inclusion vicinities for different mass ratios and distances between the interacting obstacles.

Appendices

Appendix 1: Analytical values of retarded-type operator acting on linear shape function

Evaluation of operator $\mathbf{B}_1^R$ involved in Eq. (26) with the shape function (27) gives

$$\begin{aligned} \left. {\mathbf{B}_1^R \left[ {{\upvartheta }_l (t)} \right] } \right| _{t=t_r =r\Delta t} =B_1^{r-l+1} +B_2^{r-l} , \end{aligned}$$

(31)

where after the temporal integration different expressions take place for the coefficients $B_1^H$ and $B_2^H (H=r-l+1, r-l)$ depending on the distance $R$. With the denotation $E=H-$1 they are:

• Case I: $R<Ec_2 \Delta t$ or $R>Hc_1 \Delta t$, then

  $$\begin{aligned} B_1^H =B_2^H =0; \end{aligned}$$

  (32)

• Case II: $Ec_2 \Delta t<R<Hc_2 \Delta t$ and $R<Ec_1 \Delta t$, then

  $$\begin{aligned} B_1^H&= \frac{1}{2}H-\frac{2}{3}\frac{R}{\Delta tc_2 }+\frac{1}{2}\frac{(\Delta t)^{2}c_2^2}{R^{2}}E^{2}\left( {1+\frac{1}{3}E} \right) , \nonumber \\ B_2^H&= -\frac{1}{2}E+\frac{2}{3}\frac{R}{\Delta tc_2 }-\frac{1}{6}\frac{(\Delta t)^{2}c_2^2}{R^{2}}E^{3}; \end{aligned}$$

  (33)

• Case III: $Ec_1 \Delta t<R<Hc_2 \Delta t$, then

  $$\begin{aligned} B_1^H&= \frac{1}{2}H-\frac{2}{3}\frac{R}{\Delta tc_2 }+\frac{1}{2}{\upgamma }^{2}\left( {H-\frac{2}{3}\frac{R}{\Delta tc_1}} \right) , \nonumber \\ B_2^H&= -\frac{1}{2}E+\frac{2}{3}\frac{R}{\Delta tc_2 }-\frac{1}{2}{\upgamma }^{2}\left( {E-\frac{2}{3}\frac{R}{\Delta tc_1}} \right) ; \end{aligned}$$

  (34)

• Case IV: $Ec_1 \Delta t<R<Hc_1 \Delta t$ and $R>Hc_2 \Delta t$, then

  $$\begin{aligned} B_1^H&= -\frac{1}{2}{\upgamma }^{2}\left( {H-\frac{2}{3}\frac{R}{\Delta tc_1}} \right) -\frac{1}{6}H^{3}\frac{(\Delta t)^{2}c_2^2}{R^{2}}, \nonumber \\ B_2^H&= -\frac{1}{2}{\upgamma }^{2}\left( {E-\frac{2}{3}\frac{R}{\Delta tc_1}} \right) \nonumber \\&-\frac{1}{2}\frac{(\Delta t)^{2}c_2^2}{R^{2}}H^{2}\left( {1-\frac{1}{3}H} \right) ; \end{aligned}$$

  (35)

• Case V: $Hc_2 \Delta t<R<Ec_1 \Delta t$, then

  $$\begin{aligned} B_1^H&= -\frac{1}{6}\frac{(\Delta t)^{2}c_2^2}{R^{2}}\left( {3E+1} \right) , \nonumber \\ B_2^H&= -\frac{1}{6}\frac{(\Delta t)^{2}c_2^2}{R^{2}}\left( {E+2H} \right) . \end{aligned}$$

  (36)

Appendix 2: Coefficients of resulting system of linear algebraic equations for piecewise-constant spatial approximation

From Eqs. (26) and (27) it follows

$$\begin{aligned}&c_i^{(n)} =\int \int \limits _{\tilde{S}_{ni}} {\sin {\upeta }_1^{(n)} dS_{\upeta }},\nonumber \\&c_{ji}^{(n)} =\int \int \limits _{\tilde{S}_{ni}} {\sin ^{2}{\upeta }_1^{(n)} ({\updelta }_{1j} \hbox {sin}{\upeta }_2^{(n)} -{\updelta }_{2j} \hbox {cos}{\upeta }_2^{(n)} )dS_{\upeta }} , \nonumber \\&\tilde{S}_{ni} :\left\{ { {\uppi }(m-1)L_n}/{\left( {2Q_n} \right) }\le {\upeta }_1^{(n)} \le { {\uppi }mL_n}/{\left( {2Q_n} \right) }; \right. \nonumber \\&\quad \left. { 2{\uppi }(l-1)}/{L_n}\le {\upeta }_2^{(n)} \le {2{\uppi }l}/{L_n} \right\} , \nonumber \\&\quad m=1,2,\ldots , {Q_n}/{L_n}; \qquad n=1,2,\ldots ,L_n ; \nonumber \\&\quad i=(m-1)L_n +l. \end{aligned}$$

(37)

After integration we obtain

$$\begin{aligned} c_i^{(n)} \!&= \!\frac{2{\uppi }}{L_n}\left\{ {\cos \left[ {{ {\uppi }(m-1)L_n }/{\left( {2Q_n} \right) }} \right] \!-\!\cos \left[ {{ {\uppi }mL_n }/{\left( {2Q_n} \right) }} \right] } \right\} , \nonumber \\ c_{ji}^{(n)}&= \frac{1}{4}{\updelta }_{1j} \left\{ \cos \left[ {{ 2{\uppi }(l-1)}/{L_n}} \right] \right. \nonumber \\&\left. -\cos \left[ {{ 2{\uppi }l}/{L_n}} \right] \right\} \left\{ \sin \left[ {{ {\uppi }(m-1)L_n}/{Q_n}} \right] \right. \nonumber \\&\left. -\sin \left[ {{ {\uppi }mL_n}/{Q_n}} \right] +{ {\uppi }L_n}/{Q_n} \right\} \nonumber \\&+\frac{1}{4}{\updelta }_{2j} \left\{ \sin \left[ {{ 2{\uppi }(l-1)}/{L_n }} \right] \right. \nonumber \\&\left. -\sin \left[ {{ 2{\uppi }l}/{L_n}} \right] \right\} \left\{ \sin \left[ {{ {\uppi }(m-1)L_n}/{Q_n}} \right] \right. \nonumber \\&\left. -\sin \left[ {{ {\uppi }mL_n}/{Q_n}} \right] +{ {\uppi }L_n}/{Q_n} \right\} . \end{aligned}$$

(38)