Skip to main content
SpringerLink
Log in

Concyclic Intervals in the Plane

  • Published:
Discrete & Computational Geometry Aims and scope Submit manuscript

Abstract

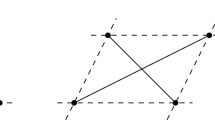
We study arrangements of intervals in \({\mathbb {R}}^2\) for which many pairs are concyclic. We show that any set of intervals with many concyclic pairs must have underlying algebraic and geometric structure. In the most general case, we prove that the endpoints of many intervals belong to a single bicircular quartic curve.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

Notes

  1. A circle is ordinary if it contains exactly three points of P.

References

  1. Casey, J.: On bicircular quartics. Trans. R. Irish Acad. 24(Science), 457–569 (1871)

    Google Scholar 

  2. Chazelle, B., Edelsbrunner, H.: An optimal algorithm for intersecting line segments in the plane. J. Assoc. Comput. Mach. 39(1), 1–54 (1992)

  3. Di Benedetto, D., Solymosi, J., White, E.P.: Combinatorics of intervals in the plane I: trapezoids. Discrete Comput. Geom. 69(1), 232–249 (2023)

    Article  MathSciNet  MATH  Google Scholar 

  4. Fletcher, R.R., III.: Geometric ramifications of invariant expressions in the ternary hypercommutative variety. In: New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. STEAM-H: Science, Technology, Engineering, Agriculture, Mathematics & Health, pp. 107–122. Springer, Cham (2017)

  5. Foraker, F.A.: Determinants in elementary analytic geometry. Am. Math. Mon. 27(2), 57–61 (1920)

    Article  MathSciNet  Google Scholar 

  6. Glaister, P.: Intersecting chords theorem: 30 years on. Math. Sch. 36(1), 22 (2007)

    Google Scholar 

  7. Green, B., Tao, T.: On sets defining few ordinary lines. Discrete Comput. Geom. 50(2), 409–468 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  8. Guth, L., Katz, N.H.: Algebraic methods in discrete analogs of the Kakeya problem. Adv. Math. 225(5), 2828–2839 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  9. Guth, L., Katz, N.H.: On the Erdős distinct distances problem in the plane. Ann. Math. 181(1), 155–190 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  10. Hilton, H.: Plane Algebraic Curves. Clarendon Press, Oxford (1920)

    MATH  Google Scholar 

  11. Kaplan, H., Sharir, M., Shustin, E.: On lines and joints. Discrete Comput. Geom. 44(4), 838–843 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  12. Kollár, J.: Szemerédi–Trotter-type theorems in dimension 3. Adv. Math. 271, 30–61 (2015)

  13. Lin, A., Makhul, M., Mojarrad, H.N., Schicho, J., Swanepoel, K., de Zeeuw, F.: On sets defining few ordinary circles. Discrete Comput. Geom. 59(1), 59–87 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  14. Pach, J., Sharir, M.: On vertical visibility in arrangements of segments and the queue size in the Bentley–Ottmann line sweeping algorithm. SIAM J. Comput. 20(3), 460–470 (1991)

  15. Pach, J., Sharir, M.: Combinatorial Geometry and its Algorithmic Applications: The Alcalá Lectures. Mathematical Surveys and Monographs, vol. 152. American Mathematical Society, Providence (2009)

    MATH  Google Scholar 

  16. Salmon, G.: A Treatise on Conic Sections, 6th edn. Chelsea, New York (1954)

  17. Salmon, G.: A Treatise on the Higher Plane Curves: Intended as a Sequel to “A Treatise on Conic Sections’’, 3rd edn. Chelsea, New York (1960)

    MATH  Google Scholar 

  18. Solymosi, J., de Zeeuw, F.: On a question of Erdős and Ulam. Discrete Comput. Geom. 43(2), 393–401 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  19. Tóth, Cs.D.: Binary plane partitions for disjoint line segments. Discrete Comput. Geom. 45(4), 617–646 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  20. Wiernik, A., Sharir, M.: Planar realizations of nonlinear Davenport–Schinzel sequences by segments. Discrete Comput. Geom. 3(1), 15–47 (1988)

Download references

Acknowledgements

The authors thank Daniel Di Benedetto for valuable suggestions and conversations throughout the preparation of this paper. The research of the first author was supported in part by an NSERC Discovery grant and OTKA K 119528 grant. The research of the second author was supported in part by Killam and NSERC doctoral scholarships.

Author information

Authors and Affiliations

  1. The University of British Columbia, Vancouver, Canada

    József Solymosi & Ethan Patrick White

  2. Obuda University, Budapest, Hungary

    József Solymosi

Authors
  1. József Solymosi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ethan Patrick White
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ethan Patrick White.

Additional information

Editor in Charge: Csaba D. Tóth

Dedicated to the memory of Eli Goodman.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

Below we give the bicircular quartic curve \(P(a,b)=0\) discussed in Sect. 4.3. The constant term is 0. The degree four homogeneous component:

$$\begin{aligned}{} & {} (a^2+b^2)^2(a_1d_1b_2-a_1d_1d_2-b_1c_1b_2+b_1c_1d_2\\{} & {} \qquad \qquad \qquad \qquad \qquad -\,b_1a_2d_2+b_1b_2c_2+d_1a_2d_2-d_1b_2c_2). \end{aligned}$$

Degree three homogeneous component:

$$\begin{aligned}&(a^2+b^2)(a_1^2c_1b_2b-a_1^2c_1d_2b-a_1^2d_1b_2a +a_1^2d_1d_2a+a_1^2a_2d_2b-a_1^2b_2c_2b\\&\quad -a_1c_1^2b_2b + a_1c_1^2d_2b -a_1d_1^2b_2b+a_1d_1^2d_2b-a_1d_1a_2^2b +a_1d_1a_2b-a_1d_1b_2^2b\\&\quad -a_1d_1b_2a + a_1d_1c_2^2b - a_1d_1c_2b +a_1d_1d_2^2b+a_1d_1d_2a-a_1a_2d_2b+a_1b_2c_2b\\&\quad +b_1^2c_1b_2b-b_1^2c_1d_2b-b_1^2d_1b_2a + b_1^2d_1d_2a +b_1^2a_2d_2b-b_1^2b_2c_2b+b_1c_1^2b_2a\\&\quad -b_1c_1^2d_2a+b_1c_1a_2^2b - b_1c_1a_2b + b_1c_1b_2^2b + b_1c_1b_2a -b_1c_1c_2^2b+b_1c_1c_2b \\&\quad -b_1c_1d_2^2b-b_1c_1d_2a +b_1d_1^2b_2a-b_1d_1^2d_2a - b_1a_2^2c_2b + b_1a_2^2d_2a +b_1a_2c_2^2b\\&\quad +b_1a_2d_2^2b+b_1a_2d_2a -b_1b_2^2c_2b+b_1b_2^2d_2a-b_1b_2c_2^2a-b_1b_2c_2a - b_1b_2d_2^2a \\&\quad -c_1^2a_2d_2b+c_1^2b_2c_2b+c_1a_2d_2b-c_1b_2c_2b -d_1^2a_2d_2b+d_1^2b_2c_2b+d_1a_2^2c_2b \\&\quad - d_1a_2^2d_2a -d_1a_2c_2^2b-d_1a_2d_2^2b-d_1a_2d_2a+d_1b_2^2c_2b -d_1b_2^2d_2a + d_1b_2c_2^2a \\&\quad + d_1b_2c_2a + d_1b_2d_2^2a). \end{aligned}$$

Degree two homogeneous component:

$$\begin{aligned}&-a_1^2c_1a_2^2b^2 + a_1^2c_1a_2b^2 - a_1^2c_1b_2^2b^2 - a_1^2c_1b_2ab + a_1^2c_1c_2^2b^2 \\&\quad - a_1^2c_1c_2b^2 + a_1^2c_1d_2^2b^2 + a_1^2c_1d_2ab + a_1^2d_1a_2^2ab - a_1^2d_1a_2d_2a^2 \\&\quad - a_1^2d_1a_2d_2b^2 - a_1^2d_1a_2ab + a_1^2d_1b_2^2ab + a_1^2d_1b_2c_2a^2 + a_1^2d_1b_2c_2b^2\\&\quad + a_1^2d_1b_2a^2 - a_1^2d_1c_2^2ab + a_1^2d_1c_2ab - a_1^2d_1d_2^2ab - a_1^2d_1d_2a^2 \\&\quad + a_1^2a_2^2c_2b^2 - a_1^2a_2^2d_2ab - a_1^2a_2c_2^2b^2 - a_1^2a_2d_2^2b^2 + a_1^2b_2^2c_2b^2 \\&\quad - a_1^2b_2^2d_2ab + a_1^2b_2c_2^2ab + a_1^2b_2d_2^2ab + a_1c_1^2a_2^2b^2 - a_1c_1^2a_2b^2\\&\quad +a_1c_1^2b_2^2b^2 + a_1c_1^2b_2ab - a_1c_1^2c_2^2b^2 + a_1c_1^2c_2b^2 - a_1c_1^2d_2^2b^2 \\&\quad - a_1c_1^2d_2ab + a_1d_1^2a_2^2b^2 - a_1d_1^2a_2b^2 +a_1d_1^2b_2^2b^2+a_1d_1^2b_2ab\\&\quad - a_1d_1^2c_2^2b^2 + a_1d_1^2c_2b^2 - a_1d_1^2d_2^2b^2 - a_1d_1^2d_2ab + a_1d_1a_2^2d_2a^2 \\&\quad + a_1d_1a_2^2d_2b^2 + a_1d_1b_2^2d_2a^2 + a_1d_1b_2^2d_2b^2 - a_1d_1b_2c_2^2a^2 - a_1d_1b_2c_2^2b^2 \\&\quad - a_1d_1b_2d_2^2a^2 - a_1d_1b_2d_2^2b^2 - a_1a_2^2c_2b^2 + a_1a_2^2d_2ab + a_1a_2c_2^2b^2 \\&\quad + a_1a_2d_2^2b^2 - a_1b_2^2c_2b^2 + a_1b_2^2d_2ab - a_1b_2c_2^2ab - a_1b_2d_2^2ab \\&\quad - b_1^2c_1a_2^2b^2 + b_1^2c_1a_2b^2 - b_1^2c_1b_2^2b^2 - b_1^2c_1b_2ab + b_1^2c_1c_2^2b^2 \\&\quad - b_1^2c_1c_2b^2 + b_1^2c_1d_2^2b^2 + b_1^2c_1d_2ab + b_1^2d_1a_2^2ab - b_1^2d_1a_2d_2a^2 \\&\quad - b_1^2d_1a_2d_2b^2 - b_1^2d_1a_2ab + b_1^2d_1b_2^2ab + b_1^2d_1b_2c_2a^2 + b_1^2d_1b_2c_2b^2 \\&\quad + b_1^2d_1b_2a^2 -b_1^2d_1c_2^2ab+b_1^2d_1c_2ab - b_1^2d_1d_2^2ab - b_1^2d_1d_2a^2 \\&\quad + b_1^2a_2^2c_2b^2 - b_1^2a_2^2d_2ab - b_1^2a_2c_2^2b^2 - b_1^2a_2d_2^2b^2 + b_1^2b_2^2c_2b^2 \\&\quad - b_1^2b_2^2d_2ab + b_1^2b_2c_2^2ab + b_1^2b_2d_2^2ab - b_1c_1^2a_2^2ab + b_1c_1^2a_2d_2a^2 \\&\quad + b_1c_1^2a_2d_2b^2 + b_1c_1^2a_2ab - b_1c_1^2b_2^2ab - b_1c_1^2b_2c_2a^2 - b_1c_1^2b_2c_2b^2 \\&\quad - b_1c_1^2b_2a^2 + b_1c_1^2c_2^2ab - b_1c_1^2c_2ab + b_1c_1^2d_2^2ab + b_1c_1^2d_2a^2 \\&\quad - b_1c_1a_2^2d_2a^2 - b_1c_1a_2^2d_2b^2 - b_1c_1b_2^2d_2a^2 - b_1c_1b_2^2d_2b^2 + b_1c_1b_2c_2^2a^2 \\&\quad + b_1c_1b_2c_2^2b^2 + b_1c_1b_2d_2^2a^2 + b_1c_1b_2d_2^2b^2 - b_1d_1^2a_2^2ab + b_1d_1^2a_2d_2a^2\\&\quad + b_1d_1^2a_2d_2b^2 + b_1d_1^2a_2ab - b_1d_1^2b_2^2ab - b_1d_1^2b_2c_2a^2 - b_1d_1^2b_2c_2b^2 \\&\quad - b_1d_1^2b_2a^2 + b_1d_1^2c_2^2ab - b_1d_1^2c_2ab + b_1d_1^2d_2^2ab + b_1d_1^2d_2a^2 \\&\quad + b_1a_2^2c_2ab - b_1a_2^2d_2a^2 - b_1a_2c_2^2ab - b_1a_2d_2^2ab + b_1b_2^2c_2ab\\&\quad - b_1b_2^2d_2a^2 + b_1b_2c_2^2a^2 + b_1b_2d_2^2a^2 - c_1^2a_2^2c_2b^2 + c_1^2a_2^2d_2ab\\&\quad + c_1^2a_2c_2^2b^2 + c_1^2a_2d_2^2b^2 - c_1^2b_2^2c_2b^2 + c_1^2b_2^2d_2ab - c_1^2b_2c_2^2ab \\&\quad - c_1^2b_2d_2^2ab + c_1a_2^2c_2b^2 - c_1a_2^2d_2ab - c_1a_2c_2^2b^2 - c_1a_2d_2^2b^2\\&\quad + c_1b_2^2c_2b^2 - c_1b_2^2d_2ab + c_1b_2c_2^2ab + c_1b_2d_2^2ab - d_1^2a_2^2c_2b^2\\&\quad + d_1^2a_2^2d_2ab + d_1^2a_2c_2^2b^2 + d_1^2a_2d_2^2b^2 - d_1^2b_2^2c_2b^2 + d_1^2b_2^2d_2ab \\&\quad - d_1^2b_2c_2^2ab - d_1^2b_2d_2^2ab - d_1a_2^2c_2ab + d_1a_2^2d_2a^2 + d_1a_2c_2^2ab \\&\quad + d_1a_2d_2^2ab - d_1b_2^2c_2ab + d_1b_2^2d_2a^2 - d_1b_2c_2^2a^2 - d_1b_2d_2^2a^2. \end{aligned}$$

Degree one homogeneous component:

$$\begin{aligned}&a_1^2c_1a_2^2d_2b - a_1^2c_1a_2d_2b + a_1^2c_1b_2^2d_2b - a_1^2c_1b_2c_2^2b + a_1^2c_1b_2c_2b\\&\quad - a_1^2c_1b_2d_2^2b - a_1^2d_1a_2^2c_2b + a_1^2d_1a_2c_2^2b + a_1^2d_1a_2d_2^2b + a_1^2d_1a_2d_2a \\&\quad - a_1^2d_1b_2^2c_2b - a_1^2d_1b_2c_2a - a_1c_1^2a_2^2d_2b + a_1c_1^2a_2d_2b - a_1c_1^2b_2^2d_2b \\&\quad + a_1c_1^2b_2c_2^2b - a_1c_1^2b_2c_2b + a_1c_1^2b_2d_2^2b - a_1d_1^2a_2^2d_2b + a_1d_1^2a_2d_2b\\&\quad - a_1d_1^2b_2^2d_2b + a_1d_1^2b_2c_2^2b - a_1d_1^2b_2c_2b + a_1d_1^2b_2d_2^2b + a_1d_1a_2^2c_2b \\&\quad - a_1d_1a_2^2d_2a - a_1d_1a_2c_2^2b - a_1d_1a_2d_2^2b + a_1d_1b_2^2c_2b-a_1d_1b_2^2d_2a\\&\quad +a_1d_1b_2c_2^2a+a_1d_1b_2d_2^2a+b_1^2c_1a_2^2d_2b - b_1^2c_1a_2d_2b + b_1^2c_1b_2^2d_2b \\&\quad - b_1^2c_1b_2c_2^2b + b_1^2c_1b_2c_2b - b_1^2c_1b_2d_2^2b - b_1^2d_1a_2^2c_2b + b_1^2d_1a_2c_2^2b\\&\quad + b_1^2d_1a_2d_2^2b + b_1^2d_1a_2d_2a\\&\quad - b_1^2d_1b_2^2c_2b - b_1^2d_1b_2c_2a + b_1c_1^2a_2^2c_2b - b_1c_1^2a_2c_2^2b - b_1c_1^2a_2d_2^2b\\&\quad - b_1c_1^2a_2d_2a + b_1c_1^2b_2^2c_2b +b_1c_1^2b_2c_2a-b_1c_1a_2^2c_2b + b_1c_1a_2^2d_2a\\&\quad + b_1c_1a_2c_2^2b + b_1c_1a_2d_2^2b - b_1c_1b_2^2c_2b + b_1c_1b_2^2d_2a - b_1c_1b_2c_2^2a\\&\quad -b_1c_1b_2d_2^2a+b_1d_1^2a_2^2c_2b - b_1d_1^2a_2c_2^2b - b_1d_1^2a_2d_2^2b - b_1d_1^2a_2d_2a \\&\quad + b_1d_1^2b_2^2c_2b + b_1d_1^2b_2c_2a. \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Solymosi, J., White, E.P. Concyclic Intervals in the Plane. Discrete Comput Geom (2023). https://doi.org/10.1007/s00454-023-00515-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00454-023-00515-y

Keywords

Mathematics Subject Classification

Search

Navigation