A new bending model for composite laminated shells based on the refined zigzag theory

Abstract

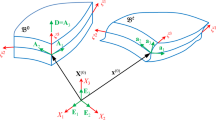
In this paper, a new bending model for composite laminated shells is proposed based on the refined zigzag theory. This new model is superior to the previous shell models based on the first-order shear deformation theory (FSDT) by introducing in-plane linear zigzag functions along the thickness direction, such that the shear correction coefficients can be omitted. In the present formulation, the bending equilibrium equations and boundary conditions of composite laminated shells are established by the virtual work principle. Without losing generality, Navier series solutions are used to describe the static properties of the composite laminated shells. In order to evaluate the effectiveness and performance of this new model, numerical examples are carried out to discuss the effect of the lamination schemes and geometric parameters on the static properties. In addition, the results are compared with the three-dimensional (3D) elastic theory and FSDT as well as the highly accurate theories in the literature to examine the accuracy. It is observed that the present model not only has the high accuracy of the high-order or other highly accurate models, but also has the intrinsic linear terms of the first-order models, such that it is very suitable for the future deducing finite element method to analyze the large-scale laminates with high computational efficiency. Therefore, the present new model can be a good candidate for engineering applications.

Appendices

Appendix A

The refined zigzag functions are defined by piecewise linear functions [42]

$$\begin{gathered} \phi_{{1}}^{k)} = \frac{1}{2}(1 - \xi^{(k)} )u_{(k - 1)} + \frac{1}{2}(1 + \xi^{(k)} )u_{(k)} \hfill \\ \phi_{{2}}^{k)} = \frac{1}{2}(1 - \zeta^{(k)} v_{(k - 1)} + \frac{1}{2}(1 + \xi^{(k)} )v_{(k)} \hfill \\ \xi^{(k)} = \left[ {\frac{{z - z_{(k - 1)} }}{{h^{(k)} }} - 1} \right] \in \left[ { - 1,1} \right]{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} (k = 1,2,....N). \hfill \\ \end{gathered}$$

(A1)

The first layer starts at $z_{(0)} = - h$ and the last layer ends at $z_{(N)} = h.$$z_{(k)} = z_{(k - 1)} + 2h^{(k)}$, where $2h^{(k)}$ represents the thickness of the kth layer.

In addition, the integrals of the slope functions $\beta_{\alpha }^{(k)} {(}\alpha { = 1,2)}$ through the thickness disappear according to RZT [42], which yields

$$\int_{{{ - }h}}^{h} {\left\{ \begin{gathered} \beta_{{1}}^{(k)} \hfill \\ \beta_{2}^{(k)} \hfill \\ \end{gathered} \right\}} {\text{d}}z = \left\{ \begin{gathered} \sum\limits_{k = 1}^{N} {2h^{(k)} \beta_{1}^{(k)} } \hfill \\ \sum\limits_{k = 1}^{N} {2h^{(k)} \beta_{2}^{(k)} } \hfill \\ \end{gathered} \right\} = \left\{ \begin{gathered} 0 \hfill \\ 0 \hfill \\ \end{gathered} \right\}.$$

(A2)

The average transverse shear strains can be expressed as [42]

$$\left\{ \begin{gathered} \gamma_{{1}} \hfill \\ \gamma_{{2}} \hfill \\ \end{gathered} \right\} = \frac{{1}}{{{2}h}}\int_{ - h}^{h} {\left\{ \begin{gathered} \gamma_{1z}^{(k)} \hfill \\ \gamma_{2z}^{(k)} \hfill \\ \end{gathered} \right\}{\text{d}}z} .$$

(A3)

Moreover, the transverse shear stresses can be expressed as

$$\begin{gathered} \left\{ \begin{gathered} \tau_{{{1}z}} \hfill \\ \tau_{2z} \hfill \\ \end{gathered} \right\}^{(k} = \left[ {\begin{array}{*{20}c} {Q_{11} } & {Q_{12} } \\ {Q_{21} } & {Q_{22} } \\ \end{array} } \right]^{(k)} \left\{ \begin{gathered} \eta_{1} \hfill \\ \eta_{2} \hfill \\ \end{gathered} \right\} + Q_{11}^{(k)} (1 + \beta_{1}^{(k)} )\left\{ \begin{gathered} 1 \hfill \\ Q_{12}^{(k)} /Q_{11}^{(k)} \hfill \\ \end{gathered} \right\}\psi_{1} + \hfill \\ Q_{22}^{(k)} (1 + \beta_{2}^{(k)} )\left\{ \begin{gathered} Q_{12}^{(k)} /Q_{22}^{(k)} \hfill \\ 1 \hfill \\ \end{gathered} \right\}\psi_{2} . \hfill \\ \end{gathered}$$

(A4)

It is noted that $\eta_{\alpha } = \gamma_{\alpha } - \psi_{\alpha } {\kern 1pt} (\alpha = 1,{\kern 1pt} {\kern 1pt} 2).$ The above constraints give rise to the following expressions [42]:

$$\left\{ \begin{gathered} \beta_{{1}}^{k)} \hfill \\ \beta_{2}^{(k)} \hfill \\ \end{gathered} \right\} = \left\{ \begin{gathered} \frac{{G_{1} }}{{Q_{11}^{(k)} }} - 1 \hfill \\ \frac{{G_{2} }}{{Q_{22}^{(k)} }} - 1 \hfill \\ \end{gathered} \right\}$$

(A5)

where

$$\left\{ \begin{gathered} G_{1} \hfill \\ G_{2} \hfill \\ \end{gathered} \right\} = \left[ {\begin{array}{*{20}c} {(\frac{1}{2h}\int_{ - h}^{h} {\frac{{{\text{d}}z}}{{Q_{11}^{(k)} }})^{ - 1} } } \\ {(\frac{1}{2h}\int_{ - h}^{h} {\frac{{{\text{d}}z}}{{Q_{22}^{(k)} }})^{ - 1} } } \\ \end{array} } \right]$$

(A6)

in which $G_{1}$ and $G_{2}$ can be regarded as the weighted average transverse shear stiffness coefficient of the each layer.

Substituting (A6) into (A4) gives the transverse shear stresses [42]

$$\left\{ {\begin{array}{*{20}c} {\tau_{{{\text{1z}}}} } \\ {\tau_{2z} } \\ \end{array} } \right\}^{(k)} = \left[ {\begin{array}{*{20}c} {Q_{11} } & {Q_{12} } \\ {Q_{21} } & {Q_{22} } \\ \end{array} } \right]^{(k)} \left\{ {\begin{array}{*{20}c} {\gamma_{1} + \psi_{1} (\frac{{G_{1} }}{{Q_{11}^{(k)} }} - 1)} \\ {\gamma_{2} + \psi_{2} (\frac{{G_{2} }}{{Q_{22}^{(k)} }} - 1)} \\ \end{array} } \right\}.$$

(A7)

Bring (A5) into (A1) and get the zigzag functions [42]

$$\begin{gathered} \phi_{a}^{(1)} = (z + h)(\frac{{G_{a} }}{{Q_{aa}^{(1)} }} - 1){ (}k{ = 1),} \hfill \\ \phi_{a}^{(k)} = (z + h)(\frac{{G_{a} }}{{Q_{aa}^{(k)} }} - 1) + \sum\limits_{i = 2}^{k} {2h^{(i - 1)} } (\frac{{G_{a} }}{{Q_{aa}^{(i - 1)} }} - \frac{{G_{a} }}{{Q_{aa}^{(k)} }}){ (}k{ = 2,}...{,}{\kern 1pt} {\kern 1pt} N{),} \hfill \\ z \in [z_{(k - 1),} z_{(k)} ],{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} z_{(0)} = - h,z_{(k)} = z_{(k - 1)} + 2h^{(k)} { (}k{ = 1,}...{,}{\kern 1pt} {\kern 1pt} N{);}{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {(}a{ = 1,}{\kern 1pt} {\kern 1pt} {\kern 1pt} {2)}{\text{.}} \hfill \\ \end{gathered}$$

(A8)

Appendix B

The constitutive equation in the kth layer of an orthotropic shell can be shown as

$${\varvec{\sigma}}^{(k)} = T^{(k)T} M^{(k)} T^{(k)} {\varvec{\varepsilon}}^{(k)} ,T^{(k)} = \left( {\begin{array}{*{20}l} {m^{2} } \hfill & {n^{2} } \hfill & {mn} \hfill & {} \hfill & {} \hfill \\ {n^{2} } \hfill & {m^{2} } \hfill & { - mn} \hfill & {} \hfill & {} \hfill \\ { - mn} \hfill & {mn} \hfill & {m^{2} - n^{2} } \hfill & {} \hfill & {} \hfill \\ {} \hfill & {} \hfill & {} \hfill & m \hfill & n \hfill \\ {} \hfill & {} \hfill & {} \hfill & { - n} \hfill & m \hfill \\ \end{array} } \right),$$

(B1)

$$M^{(k)} = \left( {\begin{array}{*{20}l} {c_{11}^{(k)} - \frac{{c_{13}^{(k)} c_{13}^{(k)} }}{{c_{33}^{k} }}} \hfill & {c_{12}^{(k)} - \frac{{c_{13}^{(k)} c_{23}^{(k)} }}{{c_{33}^{k} }}} \hfill & {} \hfill & {} \hfill & {} \hfill \\ {c_{12}^{k} - \frac{{c_{13}^{(k)} c_{23}^{(k)} }}{{c_{33}^{(k)} }}} \hfill & {c_{22}^{(k)} - \frac{{c_{23}^{(k)} c_{23}^{(k)} }}{{c_{33}^{(k)} }}} \hfill & {} \hfill & {} \hfill & {} \hfill \\ {} \hfill & {} \hfill & {c_{66}^{(k)} } \hfill & {} \hfill & {} \hfill \\ {} \hfill & {} \hfill & {} \hfill & {c_{44}^{(k)} } \hfill & {} \hfill \\ {} \hfill & {} \hfill & {} \hfill & {} \hfill & {c_{55}^{(k)} } \hfill \\ \end{array} } \right),$$

(B2)

where ${\varvec{T}}^{k}$ is the transform matrix and ${\varvec{M}}^{k}$ is the stiffness matrix in the local coordinate. In addition, $m = \cos (\vartheta^{k} )$,$n = \sin (\vartheta^{k} )$ and $\vartheta^{k}$ is the angle of ply. Moreover, $c_{11}^{(k)} = (1 - \nu_{23}^{(k)} \nu_{32}^{(k)} )/E_{2}^{(k)} E_{3}^{(k)} \Delta ,$ $C_{22}^{(k)} = (1 - \nu_{13}^{(k)} \nu_{31}^{(k)} )/E_{1}^{(k)} E_{3}^{(k)} \Delta ,$$C_{12}^{(k)} = (\nu_{12}^{(k)} + \nu_{13}^{(k)} \nu_{32}^{(k)} )/E_{1}^{(k)} E_{3}^{(k)} \Delta ,$ $C_{13}^{(k)} = (\nu_{31}^{(k)} + \nu_{21}^{(k)} \nu_{32}^{(k)} )/E_{2}^{(k)} E_{3}^{(k)} \Delta ,$$C_{23}^{(k)} = (\nu_{23}^{(k)} + \nu_{13}^{(k)} \nu_{21}^{(k)} )/E_{1}^{(k)} E_{3}^{(k)} \Delta ,$$C_{33}^{(k)} = (1 - \nu_{21}^{(k)} \nu_{12}^{(k)} )/E_{1}^{(k)} E_{2}^{(k)} \Delta ,$$C_{66}^{(k)} = G_{12}^{(k)} ,$$C_{44}^{(k)} = G_{13}^{(k)} ,$$C_{55}^{(k)} = G_{23}^{(k)} ,$$\nu_{21}^{(k)} { = }E_{2}^{(k)} \nu_{12}^{(k)} /E_{1}^{(k)} ,$$\nu_{31}^{(k)} { = }E_{3}^{(k)} \nu_{13}^{(k)} /E_{1}^{(k)} ,$$\nu_{32}^{(k)} { = }E_{3}^{(k)} \nu_{23}^{(k)} /E_{2}^{(k)}$ and $\Delta = (1 - \nu_{12}^{(k)} \nu_{21}^{(k)} - \nu_{13}^{(k)} \nu_{31}^{(k)} - \nu_{23}^{(k)} \nu_{32}^{(k)} - \nu_{12}^{(k)} \nu_{23}^{(k)} \nu_{31}^{(k)} - \nu_{13}^{(k)} \nu_{21}^{(k)} \nu_{32}^{(k)} )/E_{1}^{(k)} E_{2}^{(k)} E_{3}^{(k)} .$

$E_{1}^{(k)}$,$E_{2}^{(k)}$ and $E_{3}^{(k)}$ are elastic modulus. $G_{12}^{(k)}$,$G_{13}^{(k)}$ and $G_{23}^{(k)}$ are shear modulus. $\nu_{12}^{(k)}$,$\nu_{13}^{(k)}$ and $\nu_{23}^{(k)}$ are Poisson ratios.

Appendix C

The components in (19) are listed as follows:

$$C = \left[ {\begin{array}{*{20}c} {C_{11} } & {C_{12} } & {C_{16} } \\ {C_{12} } & {C_{22} } & {C_{26} } \\ {C_{16} } & {C_{26} } & {C_{66} } \\ \end{array} } \right]^{(k)} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} B_{\varphi } = \left[ {\begin{array}{*{20}c} z & {\varphi_{1}^{(k)} } & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & z & {\varphi_{2}^{(k)} } & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & z & {\varphi_{1}^{(k)} } & {\varphi_{2}^{(k)} } \\ \end{array} } \right],$$

(C1)

$$Q = \left[ {\begin{array}{*{20}c} {Q_{{22}} } & {Q_{{12}} } \\ {Q_{{12}} } & {Q_{{11}} } \\ \end{array} } \right]^{{(k)}} ,\;B_{\beta } = \left[ {\begin{array}{*{20}c} 1 & {\beta _{2}^{{(k)}} } & 0 & 0 \\ 0 & 0 & 1 & {\beta _{1}^{{(k)}} } \\ \end{array} } \right],$$

(C2)

$$A = A_{3\times3} = \int_{ - h}^{h} {C{\text{d}}z} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} B = B_{3\times7} = \int_{ - h}^{h} {CB_{\phi } {\text{d}}z} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} D = D_{7\times7} = \int_{ - h}^{h} {B_{\phi }^{T} CB_{\phi } {\text{d}}z} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} G = \int_{ - h}^{h} {B_{\beta }^{T} } QB_{\beta } {\text{d}}z,$$

(C3)

$$e_{m}^{T} = \{ u_{,1} + \frac{w}{{R_{1} }},v_{,2} + \frac{w}{{R_{2} }},u_{,2} + v_{,1} \} ,$$

(C4)

$$e_{b}^{T} = \{ \theta_{1,1} ,\psi_{1,1} ,\theta_{2,2} ,\psi_{2,2} ,\theta_{1,2} + \theta_{2,1} ,\psi_{1,2} ,\psi_{2,1} \} ,$$

(C5)

$$e_{s}^{T} = \{ w_{,2} + \theta_{2} - \frac{v}{{R_{2} }},\psi_{2} ,w_{,1} + \theta_{1} - \frac{u}{{R_{1} }},\psi_{1} \} .$$

(C6)