Simulation of martensitic microstructures in a low-alloy steel

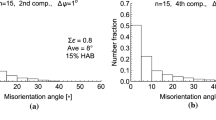
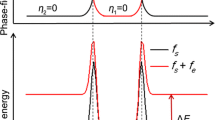
Abstract

The martensite structure of steel is of great importance in mechanical engineering and is usually adjusted by heat treatment. Of particular interest is the morphology of martensite, as it has a significant influence on mechanical properties. In this work, a phase field model is presented, where the order parameter is used to describe the evolution of martensite in order to predict the resulting morphology. In a first step, simulations with two martensite variants with different transformation strains by means of the finite element method in the small strain context show the basic applicability of the model in a two-dimensional environment. With a concept based on the phenomenological theory of martensite crystallography, good agreement with the transformation mechanics of the experiment is achieved. Furthermore, an illustrative three-dimensional simulation takes the crystallographic variants of the Nishiyama–Wasserman orientation relationship into account. The size of the simulation domain corresponds to the size of a prior austenite grain. The calculated block sizes agree with the experimental observations.

Appendices

Weighting factors of the phase field potential

Consider the standard equilibrium case ($\varDelta g = 0$) of an indefinitely extended one-dimensional continuum, which consists of two phases as depicted in Fig. 14. In equilibrium state, the order parameter function

$$\begin{aligned} \varphi = \frac{1}{2} \left[ {{\mathrm {tanh}}}\left( \frac{2 x}{l} \right) + 1 \right] \end{aligned}$$

(42)

solves the Euler–Lagrange equation

$$\begin{aligned} \frac{\partial \psi ^{{{\mathrm {int}}}}}{\partial \varphi } - \frac{d}{d x} \left[ \frac{\partial \psi ^{{{\mathrm {int}}}}}{\varphi ^\prime } \right] = 0 \quad \text {with} \quad \varphi ^\prime = \frac{d \varphi }{d x} \end{aligned}$$

(43)

of the variational problem

$$\begin{aligned} F^{{\mathrm {int}}} = \int _{- \infty }^{\infty } \psi ^{{{\mathrm {int}}}} \, dx. \end{aligned}$$

(44)

Multiplying Eq. (43) with $\varphi ^\prime$ and utilizing the identity

$$\begin{aligned} \frac{d}{d x} \left[ \frac{\partial \psi ^{{{\mathrm {int}}}}}{\varphi ^\prime } \right] \, \frac{d \varphi }{d x} = \frac{d}{d x} \left[ \frac{\partial \psi ^{{{\mathrm {int}}}}}{\varphi ^\prime } \, \frac{d \varphi }{d x} \right] - \frac{\partial \psi ^{{{\mathrm {int}}}}}{\varphi ^\prime } \, \frac{d^2 \varphi }{d x^2} \end{aligned}$$

(45)

lead to

$$\begin{aligned} \frac{d}{dx} \left[ \psi ^{{{\mathrm {int}}}} - \frac{\partial \psi ^{{{\mathrm {int}}}}}{\partial \varphi ^{\prime }} \frac{d \varphi }{d x} \right] = 0. \end{aligned}$$

(46)

Fig. 14

Indefinitely extended two phase continuum, one-dimensional with two phases a field plot, b profile plot of the order parameter

From Eq. (46) follows

$$\begin{aligned} \psi ^{{{\mathrm {int}}}} - \frac{\partial \psi ^{{{\mathrm {int}}}}}{\partial \varphi ^{\prime }} \frac{d \varphi }{d x} = \text {const.} = c. \end{aligned}$$

(47)

The constant c is found to be $c=0$ by evaluation of Eq. (47) regarding the specific order parameter $\varphi =0$ with $\psi (\varphi =0) = 0$ and $\left. \frac{d \varphi }{d x} \right| _{\varphi =0} = 0$. Analogous to Eq. (8), it is

$$\begin{aligned} \begin{aligned} \psi ^{{{\mathrm {int}}}}&= 16 {\hat{g}} \alpha ^* f^{{{\mathrm {int}}}} + \frac{1}{2} \beta ^* |\varphi ^{\prime }|^2, \\ \frac{\partial \psi ^{{{\mathrm {int}}}}}{\partial \varphi ^\prime }&= \beta ^* \varphi ^{\prime }. \end{aligned} \end{aligned}$$

(48)

Inserting Eq. (48) in (47) leads to

$$\begin{aligned} d x = \sqrt{\frac{\beta ^*}{32 \alpha ^* {\hat{g}} f^{{\mathrm {int}}}}} \, d \varphi . \end{aligned}$$

(49)

With Eq. (49), the integral with respect to x in Eq. (44) can be rewritten as an integral with respect to $\varphi$. Eventually,

$$\begin{aligned} F^{{{\mathrm {int}}}} = \sqrt{32 \alpha ^* \beta ^* {\hat{g}} } \int _{0}^{1} \sqrt{f^{{\mathrm {int}}}} \, d \varphi = \gamma \end{aligned}$$

(50)

is obtained. Evaluation of the integral leads to

$$\begin{aligned} \frac{\sqrt{32 \alpha ^* \beta ^* {\hat{g}} }}{6} = \gamma \end{aligned}$$

(51)

Furthermore, Eq. (49) can be used to derive

$$\begin{aligned} \left. \frac{d \varphi }{d x} \right| _{x=0} = \left. \sqrt{\frac{32 \alpha ^* {\hat{g}} f^{{{\mathrm {int}}}}}{\beta ^*}} \right| _{\varphi =\frac{1}{2}} = \sqrt{\frac{2 \alpha ^* {\hat{g}}}{\beta ^*}} = \frac{1}{l}. \end{aligned}$$

(52)

By solving Eqs. (51) and (52), the parameters $\alpha ^*$ and $\beta ^*$ according to Eq. (9) are determined.

Numerical implementation with two spatial dimensions

For the sake of simplicity, the two-dimensional plane strain case is considered. The weak forms

$$\begin{aligned} \begin{aligned}&\int _{\varOmega } \delta \varphi _i \left( \frac{1}{M} {\dot{\varphi }}_i + \frac{\partial \psi ^{{{\mathrm {el}}}}}{\partial \varphi _i} + 12 \, \frac{\gamma }{l} \frac{\partial f^{{{\mathrm {int}}}}}{\partial \varphi } + \varDelta g \,\frac{\partial f^{{{\mathrm {bulk}}}}}{\partial \varphi _i} \right) \\&\quad + \frac{3}{2} \gamma l \left( {\varvec{\nabla }}\delta \varphi _i \right) \cdot \left( {\varvec{\nabla }} \varphi _i\right) \, dV = 0 \end{aligned} \end{aligned}$$

(53)

and

$$\begin{aligned} \int _{\varOmega } \delta {\varvec{\varepsilon }} : {\varvec{\sigma }} \, dV = 0 \end{aligned}$$

(54)

of Eqs. (12) and (13) are used. A domain $\varOmega$ with the continuous volume V is approximated by $N_{{\mathrm {el}}}$ elements. The assembly of the system is described by

$$\begin{aligned} V \approx {\tilde{V}} = \bigcup \limits _{e = 1}^{N_{{\mathrm {el}}}} \varOmega _e, \end{aligned}$$

(55)

where $\varOmega _e$ occupies the element domain. Using the following equations, the displacement ${\varvec{u}}$ as well as the order parameters $\varphi _i$ and their rates $\dot{\varphi _i}$ are discretized,

$$\begin{aligned} {\varvec{u}} \approx \sum _{I=1}^{n_{{\mathrm {el}}}} N_I \hat{{\varvec{u}}}_I, \qquad \varphi _i \approx \sum _{I=1}^{n_{{\mathrm {el}}}} N_I {\hat{\varphi }}_{i I}, \qquad \dot{\varphi _i} \approx \sum _{I=1}^{n_{{\mathrm {el}}}} N_I \hat{{\dot{\varphi }}}_{i I}. \end{aligned}$$

(56)

Here, $N_I$ is the bilinear shape function of node I and $n_{{\mathrm {el}}}$ is the total number of nodes per element. The superimposed hat $\hat{\left( \bullet \right) }$ indicates nodal quantities. With the matrices

$$\begin{aligned} {\varvec{B^u}}_I = \begin{bmatrix} \frac{\partial N_{I}}{\partial x} &{} 0 \\ 0 &{} \frac{\partial N_{I}}{\partial y} \\ \frac{\partial N_{I}}{\partial y} &{} \frac{\partial N_{I}}{\partial x} \\ \end{bmatrix} \quad \text {and} \quad {\varvec{B}}_I^{\varphi _i} = \begin{bmatrix} \frac{\partial N_{I}}{\partial x} \\ \frac{\partial N_{I}}{\partial y} \\ \end{bmatrix} \end{aligned}$$

(57)

the gradients are discretized by

$$\begin{aligned} {\varvec{\varepsilon }} \approx \sum _{I=1}^{n_{{\mathrm {el}}}} {\varvec{B^u}}_I \hat{{\varvec{u}}}_I \quad \text {and} \quad {\varvec{\nabla }} \varphi _i \approx \sum _{I=1}^{n_{{\mathrm {el}}}} {\varvec{B}}_I^{\varphi _i} {\hat{\varphi }}_{i I}. \end{aligned}$$

(58)

In Eq. (58), Voigt notation is applied for the symmetrical strain tensor ${\varvec{\varepsilon }}= \frac{1}{2} \left( {\varvec{\nabla u}} + ({\varvec{\nabla u}})^T \right)$. Analogously, the virtual field variables

$$\begin{aligned} \begin{aligned} \delta \varphi _i&\approx \sum _{I=1}^{n_{{\mathrm {el}}}} N_I \hat{\varphi _i}_I, \qquad {\varvec{\nabla }} \delta \varphi _i \approx \sum _{I=1}^{n_{{\mathrm {el}}}} {\varvec{B}}_I^{\varphi _i} \delta {\hat{\varphi }}_{i I}, \\ \delta {\varvec{\varepsilon }}&\approx \sum _{I=1}^{n_{{\mathrm {el}}}} {\varvec{B}}_I^{{\varvec{u}}} \delta \hat{{\varvec{u}}}_{I} \end{aligned} \end{aligned}$$

(59)

are approximated. According to the isoparametric concept, the geometry of the element is approximated with identical shape functions.

Applying discretization to the weak forms by inserting Eqs. (56), (58) and (59) in Eqs. (53) and (54) yields the nodal element residuals

$$\begin{aligned} \begin{aligned} R_I^{\varphi _i}&= \int _{\varOmega _{{\mathrm {el}}}} \left( N_I \frac{1}{M} N_J \hat{{\dot{\varphi }}}_i + N_I \left( \frac{\partial \psi ^{{{\mathrm {el}}}}}{\partial \varphi _i} + 12 \frac{\gamma }{l} \frac{\partial f^{{{\mathrm {int}}}}}{\partial \varphi _i} + \frac{\partial f^{{{\mathrm {bulk}}}}}{\partial \varphi _i} \right) + \frac{3}{2} \gamma l \left( {\varvec{B}}_I^{\varphi _i}\right) ^T {\varvec{B}}_J^{\varphi _i} {\hat{\varphi }}_i \right) \,dV_{{\mathrm {el}}} \end{aligned} \end{aligned}$$

(60)

for the $i^{{{\mathrm {th}}}}$ phase field and

$$\begin{aligned} {\varvec{R}}^{{\varvec{u}}}_I = \int _{\varOmega _{{\mathrm {el}}}} \left( {\varvec{B^u}}_I \right) ^T {\varvec{{\mathcal {C}}}} \underbrace{\left( {\varvec{B^u}}_{J} \hat{{\varvec{u}}} - {\varvec{\varepsilon }}^0 \right) }_{{\varvec{\varepsilon }}^{{\mathrm {el}}}} \,dV_{{\mathrm {el}}} \end{aligned}$$

(61)

for the mechanical field. With the nodal residual vector ${\varvec{R}}_I = \begin{pmatrix} {\varvec{R^u}}_I,&R_I^{\varphi _i},&\cdots ,&R_I^{\varphi _N} \end{pmatrix}^T$ and the residual vector of an element ${\varvec{R}}_e = \begin{pmatrix} {\varvec{R}}_I,&\cdots ,&{\varvec{R}}_{n_{{\mathrm {el}}}} \end{pmatrix}^T$, the residual of the model

$$\begin{aligned} {\varvec{R}} \left( \hat{{\varvec{d}}},\hat{\dot{{\varvec{d}}}} \right) = \bigcup _{e=1}^{N_{{\mathrm {el}}}} {\varvec{R}}_e \end{aligned}$$

(62)

is assembled. The nodal residual vector ${\varvec{R}}_{I}$ depends on the nodal degrees of freedom $\hat{{\varvec{d}}} = \begin{pmatrix} {\varvec{u}},&\varphi _i,&\cdots ,&\varphi _N \end{pmatrix}^T$ and their rates. In order to solve the nonlinear equation system ${\varvec{R}}={\varvec{0}}$ at each time step, the backward Euler method is utilized for discretization in time. The degrees of freedom $\hat{{\varvec{d}}}$ in the current time step are computed with the Newton–Raphson method. This requires a system matrix consisting of a stiffness component and a damping component.

The nodal stiffness matrix ${\varvec{K}}_{IJ}$ reads

$$\begin{aligned} {\varvec{K}}_{IJ} = \begin{pmatrix} {\varvec{K^{uu}}} &{} {\varvec{K}}^{{\varvec{u}}\varphi _1} &{} \cdots &{} {\varvec{K}}^{{\varvec{u}}\varphi _i} &{} \cdots {\varvec{K}}^{{\varvec{u}}\varphi _N} \\ {\varvec{K}}^{\varphi _1 {\varvec{u}}} &{} K^{\varphi _1\varphi _1} &{} \cdots &{} K^{\varphi _1 \varphi _i} &{} \cdots K^{\varphi _1\varphi _N} \\ \vdots &{} \vdots &{} \ddots &{} \vdots &{} \vdots \\ {\varvec{K}}^{\varphi _i {\varvec{u}}} &{} K^{\varphi _i \varphi _1} &{} \cdots &{} K^{\varphi _i \varphi _i} &{} K^{\varphi _i \varphi _N} \\ \vdots &{} \vdots &{} \ddots &{} \vdots &{} \vdots \\ {\varvec{K}}^{\varphi _N {\varvec{u}}} &{} K^{\varphi _N \varphi _1} &{} \cdots &{} K^{\varphi _N \varphi _i} &{} K^{\varphi _N \varphi _N} \\ \end{pmatrix}_{IJ}, \end{aligned}$$

(63)

where the matrix entries are

$$\begin{aligned} {\varvec{K^{uu}}}_{IJ}&= \frac{\partial {\varvec{R^u}}_I}{\partial \hat{{\varvec{u}}}} = \int _{\varOmega _{{\mathrm {el}}}} \left( {\varvec{B^u}}_I \right) ^T {\varvec{{\mathcal {C}}}} {\varvec{B^u}}_{J} \, dV_{{\mathrm {el}}}, \end{aligned}$$

(64)

$$\begin{aligned} {\varvec{K}}^{{\varvec{u}} \varphi _i }_{IJ}&= \frac{\partial {\varvec{R^u}}_I}{\partial {\hat{\varphi }}_i} = \int _{\varOmega _{{\mathrm {el}}}} \left( {\varvec{B^u}}_I \right) ^T \frac{\partial h_i}{\partial \varphi _i} \left( \left( {\varvec{{\mathcal {C}}}}^{\alpha }_i - {\varvec{{\mathcal {C}}}}^{\gamma } \right) {\varvec{\varepsilon }}^{{\mathrm {el}}} - {\varvec{{\mathcal {C}}}} \tilde{{\varvec{\varepsilon }}}_i \right) N_J \, dV_{{\mathrm {el}}}, \end{aligned}$$

(65)

$$\begin{aligned} {\varvec{K}}^{ \varphi _i{\varvec{u}} }_{IJ}&= \left( {\varvec{K}}^{{\varvec{u}} \varphi _i }_{IJ}\right) ^T, \nonumber \\ K^{\varphi _i \varphi _i }_{IJ}&= \frac{\partial R^{\varphi _i}_I}{\partial {\hat{\varphi }}_i} \nonumber \\&=\int _{\varOmega _{{\mathrm {el}}}} N_I \left( \frac{\partial ^2 \psi ^{{{\mathrm {el}}}}}{\partial \varphi _i^2} + 12 \frac{\gamma }{l} \frac{\partial ^2 f^{{{\mathrm {int}}}}}{\partial \varphi _i^2} + \frac{\partial ^2 f^{{{\mathrm {bulk}}}}}{\partial \varphi _i^2} \right) N_J + \frac{3}{2} \gamma l \left( {\varvec{B}}_I^{\varphi _i}\right) ^T {\varvec{B}}_J^{\varphi _i} \, dV_{{\mathrm {el}}}, \end{aligned}$$

(66)

$$\begin{aligned} K^{\varphi _i \varphi _j }_{IJ}&= \frac{\partial R^{\varphi _i}_I}{\partial {\hat{\varphi }}_j} \nonumber \\&= \int _{\varOmega _{{\mathrm {el}}}} N_I \left( \frac{\partial ^2 \psi ^{{{\mathrm {el}}}}}{\partial \varphi _j \partial \varphi _i} + 12 \frac{\gamma }{l} \frac{\partial ^2 f^{{{\mathrm {int}}}}}{\partial \varphi _j \partial \varphi _i} + \frac{\partial ^2 f^{{{\mathrm {bulk}}}}}{\partial \varphi _j \partial \varphi _i} \right) N_J \, dV_{{\mathrm {el}}}, \nonumber \\ K^{ \varphi _j \varphi _i }_{IJ}&= K^{ \varphi _i \varphi _j }_{IJ}. \end{aligned}$$

(67)

Note the symmetry of ${\varvec{K}}_{IJ}$. The corresponding damping matrix reads

$$\begin{aligned} \begin{aligned} {\varvec{D}}_{IJ} =&- \frac{\partial {\varvec{R}}_{{\mathrm {e}}}}{\partial \hat{\dot{{\varvec{d}}}}} \\&=\frac{1}{M} \int _{\varOmega _{{\mathrm {el}}}} N_I N_J \, dV_{{\mathrm {el}}} \begin{pmatrix} {\varvec{0}}^{(2\times 2)} &{} {\varvec{0}}^{(2\times N )} \\ {\varvec{0}}^{(N \times 2)} &{} {\varvec{I}}^{(N\times N)} \end{pmatrix} \end{aligned} \end{aligned}$$

(68)

which concludes the discretization of the problem. As common in the finite element method, the Gaussian quadrature rule is used for numeric evaluation of the integrals.

Shape deformation according to the PTMC

Table 4 Deformation gradients ${\varvec{P}}$ obtained from the PTMC with material constants of pure iron

Table 5 Martensite shape strains $\tilde{{\varvec{\varepsilon }}}$ obtained from the PTMC with material constants of pure iron

Table 6 Deformation gradients ${\varvec{P}}$ obtained from the PTMC with material constants of pure iron with adapted lattice constant ratio

Table 7 Martensite shape strains $\tilde{{\varvec{\varepsilon }}}$ obtained from the PTMC with material constants of pure iron with adapted lattice constant ratio