Skip to main content
SpringerLink
Log in

Rayleigh quotient and orthogonality in the linear space of boundary functions, finding accurate upper bounds of natural frequencies of non-uniform beams

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

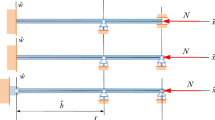
Instead of the traditional method of utilizing the extremal values of Rayleigh quotient, in this research we develop an upper bound theory to determine the natural frequencies in a linear space of boundary functions. The boundary function will have the following restrictions: boundary conditions are satisfied, fourth-order polynomial at least with unit leading coefficient. It proves that the maximum value of Rayleigh quotient in terms of kth-order boundary function builds a good upper bound when the \((k - 3)\)th-order natural frequency is approximated. There are four boundary conditions of the beam, so the fourth-order boundary function is unique without having any parameter. On the contrary, the kth-order boundary function has \(k-4\) free parameters, which leave us a chance to optimize them. We employ the orthogonality to derive the higher-order optimal boundary functions from the lower-order optimal boundary functions exactly, which are constructed sequentially by starting from the fourth-order boundary function. We examine the upper bound theory for uniform beams under three types of supports, and the single- and double- tapered beams under cantilevered support. Comparison is made between the first four natural frequencies with the exact/numerical ones, which proves the usefulness of the upper bound theory.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Richard, M.C.: Mechanics of Composite Materials. John Wiley and Sons, New York (1979)

    Google Scholar 

  2. Robert, J.M.: Mechanics of Composite Materials. Hemisphere, New York (1975)

    Google Scholar 

  3. Qin, H., Sun, Y., Liu, J.Z., Liu, Y.: Mechanical properties of wrinkled graphene generated by topological defects. Carbon 108, 204–214 (2016)

    Article  Google Scholar 

  4. Liu, Y., Wang, L.: Enhanced stiffness, strength and energy absorption for co-continuous composites with liquid filler. Compos. Struct. 128, 274–283 (2015)

    Article  Google Scholar 

  5. Goorman, D.J.: Free Vibrations of Beams and Shafts. Wiley, New York (1975)

    Google Scholar 

  6. Graff, K.F.: Wave Motion in Elastic Solids. Ohio State University Press, Columbus (1975)

    MATH  Google Scholar 

  7. Timoshenko, S.P., Goodier, J.N.: Theory of Elasticity, 3rd edn. McGraw-Hill, New York (1983)

    MATH  Google Scholar 

  8. Hibbeler, R.C.: Engineering Mechanics Dynamics. Prentice-Hall, New York (2001)

    MATH  Google Scholar 

  9. Cem Ece, M., Aydogdu, M., Taskin, V.: Vibration of a variable cross-section beam. Mech. Res. Commun. 34, 78–84 (2007)

    Article  Google Scholar 

  10. Lenci, S., Clementi, F., Mazzilli, C.E.N.: Simple formulas for the natural frequencies of non-uniform cables and beams. Int. J. Mech. Sci. 77, 155–163 (2013)

    Article  Google Scholar 

  11. Chakraverty, S., Behera, L.: Free vibration of non-uniform nanobeams using Rayleigh–Ritz method. Phys. E 67, 38–46 (2015)

    Article  Google Scholar 

  12. Li, B., Dong, L., Zhu, L., Chen, X.: On the natural frequency and vibration mode of composite beam with non-uniform cross-section. J. Vibroeng. 17, 2491–2502 (2015)

    Google Scholar 

  13. Jafari-Talookolaei, R.A., Maryam, A., Kargarnovin, M.H., Ahmadian, M.T.: An analytical approach for the free vibration analysis of generally laminated composite beams with shear effect and rotary inertia. Int. J. Mech. Sci. 65, 97–104 (2012)

    Article  Google Scholar 

  14. Oh, S.J., Lee, B.K., Lee, I.W.: Free vibrations of non-circular arches with non-uniform cross-section. Int. J. Solids Struct. 37(36), 4871–4891 (2000)

    Article  Google Scholar 

  15. Kennedy, G.J., Martins, J.R.R.A.: A homogenization-based theory for anisotropic beams with accurate through-section stress and strain prediction. Int. J. Solids Struct. 49, 54–72 (2012)

    Article  Google Scholar 

  16. Malaeke, H., Moeenfard, H.: Analytical modeling of large amplitude free vibration of non-uniform beams carrying a both transversely and axially eccentric tip mass. J. Sound Vib. 366, 211–229 (2016)

    Article  Google Scholar 

  17. Mazanoglu, K., Sabuncu, M.: Flexural vibration of non-uniform beams having double-edge breathing cracks. J. Sound Vib. 329, 4181–4191 (2010)

    Article  Google Scholar 

  18. Yang, X.D., Zhang, W., Melnik, R.V.N.: Energetics and invariants of axially deploying beam with uniform velocity. AIAA J. 54(7), 2181–2187 (2016)

    Article  Google Scholar 

  19. Hajianmaleki, M., Qatu, M.S.: Vibrations of straight and curved composite beams: a review. Compos. Struct. 100, 218–232 (2013)

    Article  Google Scholar 

  20. Bahrami, M.N., Arani, M.K., Saleh, N.R.: Modified wave approach for calculation of natural frequencies and mode shapes in arbitrary non-uniform beams. Sci. Iran. B 18, 1088–1094 (2011)

    Article  Google Scholar 

  21. Cranch, E.T., Adler, A.A.: Bending vibration of variable section beams. J. Appl. Mech. 23, 103–108 (1956)

    MATH  Google Scholar 

  22. Naguleswaran, S.: Vibration of an Euler–Bernoulli beam of constant depth and with linearly varying breadth. J. Sound Vib. 153, 509–522 (1992)

    Article  Google Scholar 

  23. Naguleswaran, S.: A direct solution for the transverse vibration of Euler–Bernoulli wedge and cone beams. J. Sound Vib. 172, 289–304 (1994)

    Article  Google Scholar 

  24. Abrate, S.: Vibration of non-uniform rods and beams. J. Sound Vib. 185, 703–716 (1995)

    Article  MathSciNet  Google Scholar 

  25. Laura, P.A.A., Gutierrez, R.H., Rossi, R.E.: Free vibration of beams of bilinearly varying thickness. Ocean Eng. 23, 1–6 (1996)

    Article  Google Scholar 

  26. Datta, A.K., Sil, S.N.: An analysis of free un-damped vibration of beams of varying cross-section. J. Comput. Struct. 59, 479–483 (1996)

    Article  Google Scholar 

  27. Hoffmann, J.A., Wertheimer, T.: Cantilever beam vibration. J. Sound Vib. 229, 1269–1276 (2000)

    Article  Google Scholar 

  28. Sarkar, K., Ganguli, R.: Closed-form solutions for non-uniform Euler–Bernoulli free-free beams. J. Sound Vib. 332, 6078–6092 (2013)

    Article  Google Scholar 

  29. Aucielloa, N.M., Ercolanob, A.: A general solution for dynamic response of axially loaded non-uniform Timoshenko beams. Int. J. Solids Struct. 41(18–19), 4861–4874 (2004)

    Article  Google Scholar 

  30. Vidal, P., Polit, O.: A family of sinus finite elements for the analysis of rectangular laminated beams. Compos. Struct. 84, 56–72 (2008)

    Article  Google Scholar 

  31. Ozutok, A., Madenci, E.: Free vibration analysis of cross-ply laminated composite beams by mixed finite element formulation. Int. J. Struct. Stab. Dyn. 13, 1250056 (2013)

    Article  MathSciNet  Google Scholar 

  32. Ozbasaran, H.: Convergence of the Rayleigh-Ritz method for buckling analysis of arbitrarily configured I-section beam-columns. Arch. Appl. Mech. 89, 2397–2414 (2019)

    Article  Google Scholar 

  33. Monterrubio, L.E., Ilanko, S.: Proof of convergence for a set of admissible functions for the Rayleigh–Ritz analysis of beams and plates and shells of rectangular planform. Comput. Struct. 147, 236–243 (2015)

    Article  Google Scholar 

Download references

Acknowledgements

The work of Botong Li is supported by the Fundamental Research Funds for the Central Universities.

Author information

Authors and Affiliations

  1. College of Mechanics and Materials, Hohai University, Nanjing, 210098, Jiangsu, China

    Chein-Shan Liu

  2. Center of Excellence for Ocean Engineering, Center of Excellence for the Oceans, National Taiwan Ocean University, Keelung, 202-24, Taiwan

    Chein-Shan Liu

  3. School of Mathematics and Physics, University of Science and Technology Beijing, Beijing, 100083, China

    Botong Li

Authors
  1. Chein-Shan Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Botong Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Botong Li.

Ethics declarations

Conflict of interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, CS., Li, B. Rayleigh quotient and orthogonality in the linear space of boundary functions, finding accurate upper bounds of natural frequencies of non-uniform beams . Arch Appl Mech 90, 1737–1753 (2020). https://doi.org/10.1007/s00419-020-01693-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-020-01693-4

Keywords

Search

Navigation