Abstract
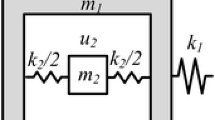
This paper presents a metamaterial plate (metaplate) consisting of a periodic array of holes on a homogeneous thin plate with slot-embedded resonators. The study numerically proves that the proposed model can generate a complete vibrational bandgap in the low-frequency range. A simplified analytical model was proposed for feasibly and accurately capturing the dispersion behavior and first bandgap characteristics in the low-frequency range, which can be used for initial design and bandgap study of the metaplate. A realistic and practical unit metaplate was subsequently designed to verify the analytical model through finite element simulations. The metaplate not only generated a complete vibrational bandgap but also exhibited excellent agreement in both analytical and finite element models for predicting the bandgap characteristics. This study facilitates the design of opening and tuning bandgaps for potential applications such as low-frequency vibration isolation and stress wave mitigation.







Similar content being viewed by others
References
Liu, Z., Zhang, X., Mao, Y., Zhu, Y.Y., Yang, Z., Chan, C.T., Sheng, P.: Locally resonant sonic materials. Science 289, 1734–1736 (2000). https://doi.org/10.1126/science.289.5485.1734
Liu, Z., Chan, C.T., Sheng, P.: Analytic model of phononic crystals with local resonances. Phys. Rev. B 71, 014103 (2005). https://doi.org/10.1103/PhysRevB.71.014103
Yao, S., Zhou, X., Hu, G.: Experimental study on negative effective mass in a 1D mass-spring system. New J. Phys. 10, 043020 (2008). https://doi.org/10.1088/1367-2630/10/4/043020
Huang, H.H., Sun, C.T., Huang, G.L.: On the negative effective mass density in acoustic metamaterials. Int. J. Eng. Sci. 47, 610–617 (2009). https://doi.org/10.1016/j.ijengsci.2008.12.007
Yu, D., Liu, Y., Zhao, H., Wang, G., Qiu, J.: Flexural vibration band gaps in Euler–Bernoulli beams with locally resonant structures with two degrees of freedom. Phys. Rev. B 73, 064301 (2006). https://doi.org/10.1103/PhysRevB.73.064301
Yu, D., Liu, Y., Wang, G., Zhao, H., Qiu, J.: Flexural vibration band gaps in Timoshenko beams with locally resonant structures. J. Appl. Phys. 100, 124901 (2006). https://doi.org/10.1063/1.2400803
Huang, H.H., Lin, C.K., Tan, K.T.: Attenuation of transverse waves by using a metamaterial beam with lateral local resonators. Smart Mater. Struct. 25, 085027 (2016). https://doi.org/10.1088/0964-1726/25/8/085027
Huang, G.L., Sun, C.T.: Band gaps in a multiresonator acoustic metamaterial. J. Vib. Acoust. 132, 031003 (2010). https://doi.org/10.1115/1.4000784
Tan, K.T., Huang, H.H., Sun, C.T.: Blast-wave impact mitigation using negative effective mass density concept of elastic metamaterials. Int. J. Impact Eng. 64, 20–29 (2014). https://doi.org/10.1016/j.ijimpeng.2013.09.003
Zhu, R., Liu, X.N., Hu, G.K., Sun, C.T., Huang, G.L.: A chiral elastic metamaterial beam for broadband vibration suppression. J. Sound Vib. 333, 2759–2773 (2014). https://doi.org/10.1016/j.jsv.2014.01.009
Huang, H.H., Sun, C.T.: Locally resonant acoustic metamaterials with 2D anisotropic effective mass density. Philos. Mag. 91, 981–996 (2011). https://doi.org/10.1080/14786435.2010.536174
Zhu, R., Huang, H.H., Huang, G.L., Sun, C.T.: Microstructure continuum modeling of an elastic metamaterial. Int. J. Eng. Sci. 49, 1477–1485 (2011). https://doi.org/10.1016/j.ijengsci.2011.04.005
Wu, T.T., Huang, Z.G., Tsai, T.C., Wu, T.C.: Evidence of complete band gap and resonances in a plate with periodic stubbed surface. Appl. Phys. Lett. 93, 111902 (2008). https://doi.org/10.1063/1.2970992
Oudich, M., Li, Y., Assouar, B.M., Hou, Z.: A sonic band gap based on the locally resonant phononic plates with stubs. New J. Phys. 12, 083049 (2010). https://doi.org/10.1088/1367-2630/12/8/083049
Oudich, M., Senesi, M., Assouar, M.B., Ruzenne, M., Sun, J.H., Vincent, B., Hou, Z., Wu, T.T.: Experimental evidence of locally resonant sonic band gap in two-dimensional phononic stubbed plates. Phys. Rev. B 84, 165136 (2011). https://doi.org/10.1103/PhysRevB.84.165136
Xiao, Y., Wen, J., Wen, X.: Flexural wave band gaps in locally resonant thin plates with periodically attached spring-mass resonators. J. Phys. D Appl. Phys. 45, 195401 (2012). https://doi.org/10.1088/0022-3727/45/19/195401
Zhu, R., Huang, G.L., Huang, H.H., Sun, C.T.: Experimental and numerical study of guided wave propagation in a thin metamaterial plate. Phys. Lett. A 375, 2863–2867 (2011). https://doi.org/10.1016/j.physleta.2011.06.006
Huang, T.Y., Shen, C., Jing, Y.: Membrane-and plate-type acoustic metamaterials. J. Acoust. Soc. Am. 139(6), 3240–3250 (2016). https://doi.org/10.1121/1.4950751
Ma, F., Huang, M., Wu, J.H.: Ultrathin lightweight plate-type acoustic metamaterials with positive lumped coupling resonant. J. Appl. Phys. 121(1), 015102 (2017). https://doi.org/10.1063/1.4972839
Bilal, O.R., Hussein, M.I.: Trampoline metamaterial: local resonance enhancement by springboards. Appl. Phys. Lett. 103(11), 111901 (2013). https://doi.org/10.1063/1.4820796
Ma, J., Hou, Z., Assouar, B.M.: Opening a large full phononic band gap in thin elastic plate with resonant units. J. Appl. Phys. 115(9), 093508 (2014). https://doi.org/10.1063/1.4867617
Li, Y., Chen, T., Wang, X., Xi, Y., Liang, Q.: Enlargement of locally resonant sonic band gap by using composite plate-type acoustic metamaterial. Phys. Lett. A 379(5), 412–416 (2015). https://doi.org/10.1016/j.physleta.2014.11.028
Li, Y., Zhu, L., Chen, T.: Plate-type elastic metamaterials for low-frequency broadband elastic wave attenuation. Ultrasonics 73, 34–42 (2017). https://doi.org/10.1016/j.ultras.2016.08.019
Wang, Y.F., Wang, Y.S., Su, X.X.: Large bandgaps of two-dimensional phononic crystals with cross-like holes. J. Appl. Phys. 110, 113520 (2011). https://doi.org/10.1063/1.3665205
Wang, Y.F., Wang, Y.S.: Complete bandgaps in two-dimensional phononic crystal slabs with resonators. J. Appl. Phys. 114, 043509 (2013). https://doi.org/10.1063/1.4816273
Wang, Y.F., Wang, Y.S.: Multiple wide complete bandgaps of two-dimensional phononic crystal slabs with cross-like holes. J. Sound Vib. 332(8), 2019–2037 (2013). https://doi.org/10.1016/j.jsv.2012.11.031
Wang, Y.F., Wang, Y.S., Zhang, C.: Two-dimensional locally resonant elastic metamaterials with chiral comb-like interlayers: bandgap and simultaneously double negative properties. J. Acoust. Soc. Am. 139(6), 3311–3319 (2016). https://doi.org/10.1121/1.4950766
Beli, D., Arruda, J.R.F., Ruzzene, M.: Wave propagation in elastic metamaterial beams and plates with interconnected resonators. Int. J. Solids Struct. (2018). https://doi.org/10.1016/j.ijsolstr.2018.01.027
Baravelli, E., Ruzzene, M.: Internally resonating lattices for bandgap generation and low-frequency vibration control. J. Sound Vib. 332, 6562–6579 (2013). https://doi.org/10.1016/j.jsv.2013.08.014
Peng, H., Pai, P.F.: Acoustic metamaterial plates for elastic wave absorption and structural vibration suppression. Int. J. Mech. Sci. 89, 350–361 (2014). https://doi.org/10.1016/j.ijmecsci.2014.09.018
Gao, N., Wu, J.H., Yu, L.: Research on bandgaps in two-dimensional phononic crystal with two resonators. Ultrasonics 56, 287–293 (2015). https://doi.org/10.1016/j.ultras.2014.08.006
Li, Y., Chen, T., Wang, X., Yu, K., Song, R.: Band structures in two-dimensional phononic crystals with periodic Jerusalem cross slot. Phys. B 456, 261–266 (2015). https://doi.org/10.1016/j.physb.2014.08.035
Frandsen, N.M.M., Bilal, O.R., Jensen, J.S., Hussein, M.I.: Inertial amplification of continuous structures: large band gaps from small masses. J. Appl. Phys. 119, 124902 (2016). https://doi.org/10.1063/1.4944429
Li, B., Tan, K.T.: Asymmetric wave transmission in a diatomic acoustic/elastic metamaterial. J. Appl. Phys. 120, 075103 (2016). https://doi.org/10.1063/1.4961209
Qureshi, A., Li, B., Tan, K.T.: Numerical investigation of band gaps in 3D printed cantilever-in-mass metamaterials. Sci. Rep. 6, 28314 (2016). https://doi.org/10.1038/srep28314
Colquitt, D.J., Colombi, A., Craster, R.V., Roux, P., Guenneau, S.R.L.: Seismic metasurfaces: sub-wavelength resonators and Rayleigh wave interaction. J. Mech. Phys. Solids 99, 379–393 (2017). https://doi.org/10.1016/j.jmps.2016.12.004
Love, A.E.H.: The small free vibrations and deformations of elastic shells. Philos. Trans. A Math. Phys. Eng. Sci. 179, 491–549 (1888). https://doi.org/10.1098/rsta.1888.0016
Osterberg, H., Cookson, J.W.: A theory of two-dimensional longitudinal and flexural vibrations in rectangular isotropic plates. J. Appl. Phys. 6, 234–246 (1935). https://doi.org/10.1063/1.1745325
Acknowledgements
HH Huang acknowledges the support (Grant No. 106-2221-E-002-018-MY3) provided by the Ministry of Science and Technology (MOST), Taiwan.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
He, JH., Huang, HH. Complete vibrational bandgap in thin elastic metamaterial plates with periodically slot-embedded local resonators. Arch Appl Mech 88, 1263–1274 (2018). https://doi.org/10.1007/s00419-018-1371-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00419-018-1371-0