Skip to main content
SpringerLink
Log in

The Couette–Poiseuille flow of a suspension modeled as a modified third-grade fluid

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

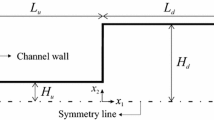
In this paper, we modify the thermodynamically compatible third-grade fluid model by introducing a shear-rate- and volume-fraction-dependent viscosity into the equation. With this new model, it is possible to predict not only the normal stress differences, but also the variable viscosity observed in many suspensions. We study the Couette–Poiseuille flow of such a fluid between two horizontal flat plates. The steady fully developed flow equations are made dimensionless and are solved numerically; the effects of different dimensionless numbers are discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Notes

  1. Morgan [29] suggested that in general, an implicit constitutive equation is a relation between a kinetic tensor (for example the stress tensor T) and one kinematic tensor (for example B, or D) of the types [see Eqs. (3.5) and (3.6) of that paper]: \(\mathbf{G(T,B)}=\mathbf{0};\;\mathbf{H(T,D)}=\mathbf{0}\).

  2. Criminale et al. [3] obtained an expression for T, valid for any laminar shear flow:

    $$\begin{aligned} \mathbf{T}=-p\mathbf{I}+\beta _1 \mathbf{A}_\mathbf{1} +\beta _2 \mathbf{A}_\mathbf{2} +\beta _3 \left( {\mathbf{A}_\mathbf{1}^\mathbf{2} +\frac{1}{2}\mathbf{A}_\mathbf{2} } \right) ;\qquad \qquad \qquad \qquad (*) \end{aligned}$$

    where \(\beta _{1}\), \(\beta _{2}\) and \(\beta _{3}\) are functions of \(\Pi \), where \(\Pi =\frac{1}{2}\mathrm{tr} \mathbf{A}_1^2 \) , and they are given by

    $$\begin{aligned} \beta _1= & {} \gamma _1 +2\gamma _5 \Pi +4\gamma _7 \Pi ^{2},\\ \beta _2= & {} \gamma _2 +0.5\gamma _3 +2\left( {\gamma _4 +\gamma _6 } \right) +4\gamma _8 \Pi ^{2},\\ \beta _3= & {} \gamma _3 ,\\ \gamma _m= & {} \alpha _m \left( {2\Pi , 0, 4\Pi ^{2}, 8\Pi ^{3},\;0,\;2\Pi ^{2}, 0, 4\Pi ^{4}} \right) , \end{aligned}$$

    The model given by Eq. (*) is known as CEF model. It can be seen that when \(\beta _{2}\) = 2\(\beta _{3}\), this equation reduces to the Reiner–Rivlin fluid model [38, 39]. Now, since \(\beta _{1}\), \(\beta _{2}\) and \(\beta _{3}\) can be assigned arbitrarily as function of \(\Pi \), in theory, the CEF model can predict shear-thinning (or thickening) as well as normal stress effects.

  3. Note that the simplest expression for the nonlinear behavior of fluids is that of the generalized power-law model where \(\mathbf{T}=-p\mathbf{I}+\mu _0 \left( {\mathrm{tr}\mathbf{A}_\mathbf{1}^\mathbf{2} } \right) ^{m}\mathbf{A}_\mathbf{1} \) when \(m < 0\), the fluid is shear thinning, and if \(m > 0\), the fluid is shear thickening. This equation is a subclass of the model presented here.

Abbreviations

b :

Body force vector

D :

Symmetric part of the velocity gradient

g :

Acceleration due to gravity

H :

Characteristic length

l :

Identity tensor

L :

Gradient of the velocity vector

t :

Time

T :

Cauchy’s stress tensor

U :

Reference velocity

x :

Spatial position occupied at time t

y :

Direction normal to the inclined plane

(Y) or \(\overline{y} \) :

Dimensionless y

\(\phi \) :

Volume fraction

div:

Divergence operator

\(\nabla \) :

Gradient symbol

References

  1. Barnes, H.A., Hutton, J.F., Walters, K.: An Introduction to Rheology. Elsevier, Amsterdam (1989)

    MATH  Google Scholar 

  2. Bird, R.B., Armstrong, R.C., Hassager, J.: Dynamics of Polymeric Liquids, vol. 1. Wiley, New York (1977)

    Google Scholar 

  3. Criminale, W.O., Ericksen, J.L., Filbey, G.L.: Steady shear flow of non-Newtonian fluids. Arch. Ration. Mech. Anal. 1, 410–417 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  4. Dunn, J.E., Fosdick, R.L.: Thermodynamics, stability, and boundedness of fluids of complexity 2 and fluids of second grade. Arch. Ration. Mech. Anal. 56, 191–252 (1974)

    Article  MathSciNet  MATH  Google Scholar 

  5. Dunn, J.E., Rajagopal, K.R.: Fluids of differential type: critical review and thermodynamic analysis. Int. J. Eng. Sci. 33, 689–729 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  6. Ekmann, J.M., Wildman, D.J., Chen, J.L.S.: Laminar flow studies of highly loaded suspensions in horizontal pipes. In: Second International Symposium on Slurry Flows ASME FED, vol. 38, pp. 85 (1986)

  7. Fosdick, R.L., Rajagopal, K.R.: Thermodynamics and stability of fluids of third grade. Proc. R. Soc. Lond. A 339, 351–377 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  8. Govier, G.W., Aziz, K.: The Flow of Complex Mixtures in Pipes. Krieger Publishing Company, Malabar (1972)

    Google Scholar 

  9. Gupta, G., Massoudi, M.: Flow of a generalized second grade fluid between heated plates. Acta Mech. 99, 21–33 (1993)

    Article  MATH  Google Scholar 

  10. Happel, V., Brenner, H.: Low Reynolds Number Hydrodynamics. Prentice Hall, Upper Saddle River (1965)

    MATH  Google Scholar 

  11. Kabir, M.A., Jasti, V.K., Higgs III, C.F., Lovell, M.R.: An evaluation of the explicit finite-element method approach for modelling dense flows of discrete grains in a Couette shear cell. Proc. IMechE Part J J. Eng. Tribol. 222, 715–720 (2008)

    Article  Google Scholar 

  12. Larson, R.G.: The Structure and Rheology of Complex Fluids. Oxford University Press, New York (1999)

    Google Scholar 

  13. Liu, I.S.: Continuum Mechanics. Springer, Berlin (2002)

    Book  MATH  Google Scholar 

  14. Macosko, C.W.: Rheology: Principles, Measurements and Applications. Wiley, New York (1994)

    Google Scholar 

  15. Man, C.-S., Massoudi, M.: On the thermodynamics of some generalized second-grade fluids. Contin. Mech. Thermodyn. 22, 27–46 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  16. Man, C.S.: Nonsteady channel flow of ice as a modified second-order fluid with power-law viscosity. Arch. Ration. Mech. Anal. 119, 35–57 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  17. Man, C.S., Sun, Q.K.: On the significant of normal stress effects in the flow of glaciers. J. Glaciol. 33, 268 (1987)

    Google Scholar 

  18. Mansutti, D., Rajagopal, K.R.: Flow of a shear thinning fluid between intersecting planes. Int. J. Non-Linear Mech. 26, 769–775 (1991)

    Article  MATH  Google Scholar 

  19. Mansutti, D., Pontrelli, G., Rajagopal, K.R.: Non-similar flow of a non-Newtonian fluid past a wedge. Int. J. Eng. Sci. 31, 637–647 (1993)

    Article  MATH  Google Scholar 

  20. Marcus, R.D., Leung, L.S., Klinzing, G.E., Rizk, F.: Pneumatic Conveying of Solids. Chapman and Hall, London (1990)

    Book  Google Scholar 

  21. Massoudi, M.: On the flow of granular materials with variable material properties. Int. J. Nonlinear Mech. 36, 25–37 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  22. Massoudi, M.: A note on the meaning of mixture viscosity using the classical continuum theories of mixtures. Int. J. Eng. Sci. 46, 677–689 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  23. Massoudi, M.: A mixture theory formulation for hydraulic or pneumatic transport of solid particles. Int. J. Eng. Sci. 48, 1440–1461 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  24. Massoudi, M., Vaidya, A.: On some generalizations of the second grade fluid model. Nonlinear Anal. Part II Real World Appl. 9, 1169–1183 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  25. Massoudi, M., Phuoc, T.X.: Fully developed flow of a modified second grade fluid with temperature dependent viscosity. Acta Mech. 150, 23–37 (2001)

    Article  MATH  Google Scholar 

  26. Massoudi, M., Phuoc, T.X.: Flow of a generalized second grade non-Newtonian fluid with variable viscosity. Contin. Mech. Thermodyn. 16, 529–538 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  27. Massoudi, M., Christie, I.: Effects of variable viscosity and viscous dissipation on the flow of a third grade fluid. Int. J. Non-Linear Mech. 30, 687–699 (1995)

    Article  MATH  Google Scholar 

  28. Massoudi, M., Sanchez, G., Vaidya, A.: An engineering approach to pipeline transport of biomass. Bull. N. J. Acad. Sci. 57, 9–11 (2012)

    Google Scholar 

  29. Morgan, A.J.A.: Some properties of media by constitutive equations in implicit form. Int. J. Eng. Sci. 4, 155–178 (1966)

    Article  MATH  Google Scholar 

  30. Oldroyd, J.G.: On the formulation of rheological equations of state. Proc. R. Soc. Lond. A200, 523–541 (1950)

    Article  MathSciNet  MATH  Google Scholar 

  31. Oldroyd, J.G.: An approach to non-Newtonian fluid-mechanics. J. Non-Newton. Fluid Mech. 14, 9–46 (1984)

    Article  MATH  Google Scholar 

  32. Papachristodoulou, G., Trass, O.: Coal slurry fuel technology. Can. J. Chem. Eng. 65, 177 (1987)

    Article  Google Scholar 

  33. Phillips, R.J., Armstrong, R.C., Brown, R.A., Graham, A.L., Abbott, J.R.: A constitutive equation for concentrated suspensions that accounts for shear-induced particle migration. Phys. Fluids A 4, 30–40 (1992)

    Article  MATH  Google Scholar 

  34. Rajagopal, K.R.: On implicit constitutive theories for fluids. J. Fluid Mech. 550, 243–249 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  35. Rajagopal, K.R.: A note on unsteady unidirectional flows of a non-Newtonian fluid. Int. J. Non-Linear Mech. 17, 369–373 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  36. Rajagopal, K.R.: On boundary conditions for fluids of differential type. In: Sequeira, A. (ed.) Navier–Stokes Equations and Related Nonlinear Problems, pp. 273–278. Plenum Press, New York (1995)

    Chapter  Google Scholar 

  37. Rajagopal, K.R., Kaloni, P.N.: Some remarks on boundary conditions for flows of fluids of the differential type. In: Graham, G.A.C., Malik, S.K. (eds.) Continuum Mechanics and its Applications. Hemisphere Press, pp. 935–942 (1989)

  38. Reiner, M.: A mathematical theory of dilatancy. Am. J. Math. 67, 350–362 (1945)

    Article  MathSciNet  MATH  Google Scholar 

  39. Rivlin, R.S.: The hydrodynamics of non-Newtonian fluids. I. Proc. R. Soc. Lond. 193, 260–281 (1948)

    Article  MathSciNet  MATH  Google Scholar 

  40. Rivlin, R.S., Ericksen, J.L.: Stress deformation relations for isotropic materials. J. Ration. Mech. Anal. 4, 323–425 (1955)

    MathSciNet  MATH  Google Scholar 

  41. Roh, N.S., Shin, D.Y., Kim, D.C., Kim, J.D.: Rheological behavior of coal–water mixtures: 1. Effects of coal type, loading and particle size. Fuel 74, 1220 (1995)

    Article  Google Scholar 

  42. Schlichting, H.: Boundary Layer Theory, 7th edn. McGraw-Hill, New York (1979)

    MATH  Google Scholar 

  43. Schowalter, E.R.: Mechanics of Non-Newtonian Fluids. Pergamon Press, New York (1978)

    Google Scholar 

  44. Shook, C.A., Roco, M.C.: Slurry Flow: Principles and Practices. Butterworth-Heinemann, Boston (1991)

    Google Scholar 

  45. Slattery, J.C.: Advanced Transport Phenomena. Cambridge University Press, Cambridge (1999)

    Book  MATH  Google Scholar 

  46. Soo, S.L.: Particulates and Continuum: Multiphase Fluid Dynamics. Hemisphere Publishing House, New York (1989)

    MATH  Google Scholar 

  47. Truesdell, C.: The mechanical foundations of elasticity and fluid dynamics. J. Ration. Mech. Anal. 2, 593–616 (1953)

    MathSciNet  MATH  Google Scholar 

  48. Truesdell, C.: Hypo-elasticity. J. Ration. Mech. Anal. 4, 83–131 (1955)

    MathSciNet  MATH  Google Scholar 

  49. Truesdell, C., Noll, W.: The Non-Linear Field Theories of Mechanics. Springer, New York (1992)

    Book  MATH  Google Scholar 

  50. Tsai, S.C., Knell, E.W.: Viscosity and rheology of coal water slurry. Fuel 65, 566 (1986)

    Article  Google Scholar 

  51. Walton, O.R., Braun, R.L.: Stress calculations for assemblies of inelastic spheres in uniform shear. Acta Mech. 63(1–4), 73–86 (1986)

    Article  Google Scholar 

  52. Walton, O.R., Braun, R.L.: Viscosity, granular-temperature, and stress calculations for shearing assemblies of inelastic frictional disks. J. Rheol. 30, 949–980 (1986)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. National Energy Technology Laboratory (NETL), U.S. Department of Energy, 626 Cochrans Mill Road, P.O. Box 10940, Pittsburgh, PA, 15236, USA

    Mehrdad Massoudi & Phuoc X. Tran

Authors
  1. Mehrdad Massoudi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Phuoc X. Tran
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mehrdad Massoudi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Massoudi, M., Tran, P.X. The Couette–Poiseuille flow of a suspension modeled as a modified third-grade fluid. Arch Appl Mech 86, 921–932 (2016). https://doi.org/10.1007/s00419-015-1070-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-015-1070-z

Keywords

Search

Navigation