The energy cycle in atmospheric models

Abstract

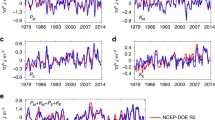
The energy cycle characterizes basic aspects of the physical behaviour of the climate system. Terms in the energy cycle involve first and second order climate statistics (means, variances, covariances) and the intercomparison of energetic quantities offers physically motivated “second order” insight into model and system behaviour. The energy cycle components of 12 models participating in AMIP2 are calculated, intercompared and assessed against results based on NCEP and ERA reanalyses. In general, models simulate a modestly too vigorous energy cycle and the contributions to and reasons for this are investigated. The results suggest that excessive generation of zonal available potential energy is an important driver of the overactive energy cycle through “generation push” while excessive dissipation of eddy kinetic energy in models is implicated through “dissipation pull‘’. The study shows that “ensemble model” results are best or among the best in the comparison of energy cycle quantities with reanalysis-based values. Thus ensemble approaches are apparently “best” not only for the simulation of 1st order climate statistics as in Lambert and Boer (Clim Dyn 17:83–106, 2001) but also for the higher order climate quantities entering the energy cycle.

Appendix

1.1 Energy cycle terms

The energy cycle equations used in this investigation are given by (4) and we list here the expressions used for calculating the terms in (4). Boundary conditions in pressure coordinates are incorporated by means of the β function $\beta(\lambda, \varphi, p, t)=\left\{\begin{array}{l}1,\quad p < p_{s}\\0, \quad p > p_{s}\end{array}\right.$ following Boer (1982). Reanalysis and model data are given on pressure surfaces and values which are “underground” (i.e. for which p _s <  p) are filled by interpolation. The approach used here masks out these unphysical values in a consistent way so that they do not enter the calculation.

The general energetic equations consider the decomposition of into zonal, standing eddy and transient eddy components as in Table 1 but taking theβ term into account as

$$\begin{aligned} X&=[\overline{X}]_{R}+\overline{X}^{*}+X^{\prime}=[\overline{X}]_{R}+X_{E}\\ [\overline{X}]_{R}&=[\overline{\beta}\, \overline{X}]/\overline{\beta},X_{E}=X-[\overline{X}]_{R}\\ [\overline{\beta} \overline{XY}]&=[\overline{\beta}][\overline{X}]_{R}[\overline{Y}]_{R}+[\overline{\beta}\overline{X_{E}Y_{E}}] \end{aligned}.$$

Available potential and kinetic energy are

$$\begin{aligned} A&=A_{Z}+A_{S}+A_{T}=\frac{1}{2}C_{p}\int \gamma [\overline{\beta}][\overline{T}]^{+2}dm+\frac{1}{2}C_{p}\int \gamma [\overline{\beta}\overline{T}^{*2}] dm +\frac{1}{2}C_{p}\int \gamma [\overline{\beta}\overline{T^{\prime 2}}]dm\\ K&=K_{Z}+K_{S}+K_{T}=\frac{1}{2}\int [\overline{\beta}] [\overline{{\user2{V}}}]_{R}\cdot[\overline{{\user2{V}}}]_{R}dm+\frac{1}{2}\int [\overline{\beta}\overline{{\user2{V}}}^{*}\cdot \overline{{\user2{V}}}^{*}] dm + \frac{1}{2}\int [\overline{\beta}\overline{{\bf V}^{\prime}\cdot {\user2{V}}^{\prime}}] dm\\ \end{aligned}$$

(A1)

but, as mentioned in the text, a number of terms involving the stationary eddy components are not available in the AMIP2 data set. For this reason among others, the stationary and transient eddy components are considered together in a combined eddy term.

The components of the available potential and kinetic energy become

$$\begin{aligned}A&=A_{Z}+A_{e}=\frac{1}{2}C_{p}\int \gamma[\overline{\beta}] [\overline{T}]^{+2}dm+\frac{1}{2}C_{p}\int \gamma [\overline{\beta}\overline{T^{2}_{E}}] dm\\ K&=K_{Z}+K_{E}=\frac{1}{2}\int [\overline{\beta}] [\overline{{\user2{V}}}]_{R}\cdot[\overline{{\user2{V}}}]_{R}dm +\frac{1}{2}[\overline{\beta}\overline{{\user2{V}}_{E}\cdot {\user2{V}}_{E}}] dm \\ \end{aligned}$$

(A2)

and the generation of available potential energy and the dissipation of kinetic energy is

$$\begin{aligned}G&=G_{Z}+G_{E}= \int \gamma[\overline{\beta}][\overline{T}]^{+}[\overline{Q}]^{+}dm+\int \gamma [\overline{\beta}\overline{T_{E}Q_{E}}]dm\\ D&=D_{Z}+D_{E}=-\int [\overline{\beta}] [\overline{{\user2{V}}}]_{R}\cdot [\overline{\bf F}]_{R}dm -\int[\overline{\beta}\overline{{\user2{V}}_{E}\cdot {\bf F}_{E}}] dm\end{aligned}$$

(A3)

The conversion between available potential and kinetic energy is

$$C(A,K)=C(A_{Z},K_{Z})+C(A_{E},K_{E})=-\int [\overline{\beta}] [\overline{\omega}]^{+}[\overline{\alpha}]^{+}dm-\int [\overline{\beta}\overline{\omega_{E}\alpha_{E}}] dm,$$

(A4)

that between zonal and eddy available potential energy is

$$C(A_{Z},A_{E})=-\int C_{p}\left(\frac{\theta}{T}\right) \left\{[\overline{\beta} \overline{T_{E}\nu_{E}}] \frac{1}{a}\frac{\partial}{\partial \varphi}+[\overline{\beta}\overline{T_{E}\omega_{E}}] \frac{\partial}{\partial p}\right\} \left(\frac{T}{\theta}\right) \gamma [\overline{T}]^{+}dm,$$

(A5)

and that between zonal and eddy kinetic energy is

$$\begin{aligned} C(K_{Z},K_{E})&=-\int \hbox{acos}\,\varphi \left\{\left([\overline{\beta}\overline{u_{E}\nu_{E}}] \frac{1}{a}\frac{\partial}{\partial \varphi}+[\overline{\beta}\overline{u_{E}\omega_{E}}] \frac{\partial}{\partial p}\right) \left(\frac{[\overline{u}]_{R}}{\hbox{acos}\,\varphi}\right)\right. \\ & \left. \quad +\left([\overline{\beta} \overline{\nu}_{E}^{2}] \frac{1}{a}\frac{\partial}{\partial \varphi} +[\overline{\beta}\overline{\nu_{E}\omega_{E}}] \frac{\partial}{\partial p}-[\overline{\beta} \overline{{\user2{V}}_{E} \cdot {\user2{V}}_{E}}] \frac{\hbox{tan}\varphi}{a}\right) \left(\frac{[\overline{{\nu}}_{R}]}{\hbox{acos}\,\varphi}\right)\right\} dm. \end{aligned}$$

(A6)

The symbols have their usual meteorological meanings and the integration is over the mass of the atmosphere. Terms are calculated on a monthly basis, that is, the means are monthly means and the variances and covariances are calculated from the synoptic variability about these monthly means.

1.2 Multi-model quantities

The ensemble model value of any of an energy budget terms, say C, is simply the ensemble mean {C} over all model values. These results are labelled MBUD in the diagrams. The mean model value, on the other hand uses the ensemble mean of the model statistics entering the calculation of C and the result is labelled MMOD in the diagrams. Symbolically if $C=f(\overline{x},\overline{y},\overline{x_{E}y_{E}},\ldots)$ represents the calculation of an energetic quantity C, the “ensemble model” value is $\{C\}=\{f(\overline{x},\overline{y},\overline{x_{E}y_{E}},\ldots)\},$ where {X} is model ensemble mean, while the “mean model” value is calculated from the ensemble average of the model values/statistics themselves, i.e. as $C_{m}=f(\{\overline{x}\}, \{\overline{y}\}, \{\overline{x_{E} y_{E}}\}, \ldots).$