On Upper Total Domination Versus Upper Domination in Graphs

Abstract

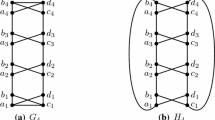
A total dominating set of a graph G is a dominating set S of G such that the subgraph induced by S contains no isolated vertex, where a dominating set of G is a set of vertices of G such that each vertex in $V(G){\setminus } S$ has a neighbor in S. A (total) dominating set S is said to be minimal if $S{\setminus } \{v\}$ is not a (total) dominating set for every $v\in S$. The upper total domination number $\varGamma _t(G)$ and the upper domination number $\varGamma (G)$ are the maximum cardinalities of a minimal total dominating set and a minimal dominating set of G, respectively. For every graph G without isolated vertices, it is known that $\varGamma _t(G)\le 2\varGamma (G)$. The case in which $\frac{\varGamma _t(G)}{\varGamma (G)}=2$ has been studied in Cyman et al. (Graphs Comb 34:261–276, 2018), which focused on the characterization of the connected cubic graphs and proposed one problem to be solved and two questions to be answered in terms of the value of $\frac{\varGamma _t(G)}{\varGamma (G)}$. In this paper, we solve this problem, i.e., the characterization of the subcubic graphs G that satisfy $\frac{\varGamma _t(G)}{\varGamma (G)}=2$, by constructing a class of subcubic graphs, which we call triangle-trees. Moreover, we show that the answers to the two questions are negative by constructing connected cubic graphs G that satisfy $\frac{\varGamma _t(G)}{\varGamma (G)}>\frac{3}{2}$ and a class of regular non-complete graphs G that satisfy $\frac{\varGamma _t(G)}{\varGamma (G)}=2$.