Skip to main content
SpringerLink
Log in

Bijections of Motzkin Paths Using Shifted Riordan Decompositions

  • Original Paper
  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

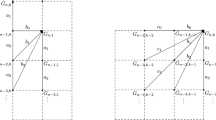
This paper concerns bijections between Motzkin and Łukasiewicz paths arising from Riordan array decompositions. Bijections have been shown between Motzkin paths and Łukasiewicz paths with constant weights (Hennessy in A study of riordan srrays with applications to continued fractions, orthogonal polynomials and lattice paths. Ph.D. thesis, Waterford Institute of Technology, 2011). We introduce a shifting matrix technique to induce a bijection between Motzkin paths and Łukasiewicz paths with non constant weighted steps. We will show a generating function proof and a construction of these bijections.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. Tridiagonal forms of production matrices have previously been referred to as Stieltjes matrices [14]. For consistency, we will refer to these matrices as production matrices throughout this article.

  2. A combinatorial proof of this identity was one of ten open questions proposed by Louis Shapiro at the Riordan array conference 2016

References

  1. Barcucci, E., Del Lungo, A., Pergola, E., Pinzani, R.: A Construction for Enumerating k-Coloured Motzkin Paths. Computing and Combinatorics, pp. 254–263. Springer, Berlin Heidelberg (1995)

  2. Barry, P.: Generalized narayana polynomials, riordan arrays, and lattice paths. J. Integer Seq. 15 (2012). https://cs.uwaterloo.ca/journals/JIS/VOL15/Barry2/barry190r. Accessed 1 Aug 2017

  3. Chen, W.Y., Yan, S.H., Yang, L.L.: Identities from weighted motzkin paths. Adv. Appl. Math. 41(3), 329–334 (2008). https://doi.org/10.1016/j.aam.2004.11.007. http://www.sciencedirect.com/science/article/pii/S0196885808000158. Accessed 1 Sept 2018

  4. Cheon, G.S., Kim, H., Shapiro, L.W.: Riordan group involutions. Linear Algebra Appl. 428(4), 941–952 (2008). https://doi.org/10.1016/j.laa.2007.09.003. http://www.sciencedirect.com/science/article/pii/S0024379507004156. Accessed 1 Aug 2017

  5. Deutsch, E., Ferrari, L., Rinaldi, S.: Production matrices. Adv. Appl. Math. 34(1), 101–122 (2005). https://doi.org/10.1016/j.aam.2004.05.002. http://www.sciencedirect.com/science/article/pii/S0196885804000673. Accessed 1 Aug 2017

  6. Deutsch, E., Ferrari, L., Rinaldi, S.: Production matrices and riordan arrays. Ann. Comb. 13(1), 65–85 (2009). https://doi.org/10.1007/s00026-009-0013-1. Accessed 1 Aug 2017

  7. Deutsch, E., Shapiro, L.W.: A bijection between ordered trees and 2-motzkin paths and its many consequences. Discrete Math. 256(3), 655–670 (2002). https://doi.org/10.1016/S0012-365X(02)00341-2. http://www.sciencedirect.com/science/article/pii/S0012365X02003412. (LaCIM 2000 Conference on Combinatorics, Computer Science and Appl ications). Accessed 1 Aug 2017

  8. Flajolet, P.: Combinatorial aspects of continued fractions. Discrete Math. 32(2), 125–161 (1980). https://doi.org/10.1016/0012-365X(80)90050-3. http://www.sciencedirect.com/science/article/pii/0012365X80900503. Accessed 1 Aug 2017

  9. He, T.X., Sprugnoli, R.: Sequence characterization of riordan arrays. Discrete Math. 309(12), 3962–3974 (2009). https://doi.org/10.1016/j.disc.2008.11.021. http://www.sciencedirect.com/science/article/pii/S0012365X0800650X. Accessed 1 Aug 2017

  10. Hennessy, A.: A study of riordan srrays with applications to continued fractions, orthogonal polynomials and lattice paths. Ph.D. thesis, Waterford Institute of Technology (2011)

  11. Lehner, F.: Cumulants, lattice paths, and orthogonal polynomials. Discrete Math. 270(1), 177–191 (2003). https://doi.org/10.1016/S0012-365X(02)00834-8. http://www.sciencedirect.com/science/article/pii/S0012365X02008348. Accessed 1 Aug 2017

  12. Merlini, D., Sprugnoli, R.: The relevant prefixes of coloured motzkin walks: An average case analysis. Theor. Comput. Sci. 411(1), 148–163 (2010). https://doi.org/10.1016/j.tcs.2009.09.021. http://www.sciencedirect.com/science/article/pii/S0304397509006690. Accessed 1 Sept 2018

  13. Merlini, D., Sprugnoli, R.: Arithmetic into geometric progressions through riordan arrays. Discrete Math. 340(2), 160–174 (2017). https://doi.org/10.1016/j.disc.2016.08.017. http://www.sciencedirect.com/science/article/pii/S0012365X16302758. Accessed 1 Aug 2017

  14. Peart, P., Woan, W.J.: Generating functions via hankel and stieltjes matrices. J. Integer Sequences 3 (2000). https://cs.uwaterloo.ca/journals/JIS/VOL15/Barry2/barry190r. Accessed 1 Aug 2017

  15. Shapiro, L.W., Getu, S., Woan, W.J., Woodson, L.C.: The riordan group. Discrete Appl. Math. 34(1), 229–239 (1991). https://doi.org/10.1016/0166-218X(91)90088-E. http://www.sciencedirect.com/science/article/pii/0166218X9190088E. Accessed 1 Aug 2017

  16. Sloane, N.J.A.: The Encyclopedia of Integer Sequences. http://oeis.org. Accessed 1 Aug 2017

  17. Sprugnoli, R.: Riordan arrays and combinatorial sums. Discrete Math. 132(1), 267–290 (1994). https://doi.org/10.1016/0012-365X(92)00570-H. http://www.sciencedirect.com/science/article/pii/0012365X9200570H. Accessed 1 Aug 2017

  18. Stanley, R.: Catalan Addendum. http://www-math.mit.edu. Accessed 1 Aug 2017

  19. Varvak, A.: Encoding Properties of Lattice Paths. Ph.D. thesis, Brandeis University (2004)

  20. Viennot, X.: Une théorie combinatoire des polynômes orthogonaux généraux lecture notes (1983)

  21. Wall, H.S.: Analytic Theory of Continued Fractions. D. Van Nostrand (1948). https://books.google.ie/books?id=OEl9QwAACAAJ. Accessed 1 Aug 2017

Download references

Author information

Authors and Affiliations

  1. Waterford Institute of Technology, Waterford, Republic of Ireland

    Aoife Hennessy

Authors
  1. Aoife Hennessy
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Aoife Hennessy.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hennessy, A. Bijections of Motzkin Paths Using Shifted Riordan Decompositions. Graphs and Combinatorics 35, 169–187 (2019). https://doi.org/10.1007/s00373-018-1982-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00373-018-1982-9

Keywords

Search

Navigation