$M_{24}$-Orbits of Octad Triples

Abstract

An octad triple is a set of three octads, octads being the blocks of the S(5, 8, 24) Steiner system. In this paper we determine the orbits of $M_{24}$, the largest Mathieu group, upon the set of octad triples.

1 Introduction

The Mathieu group $M_{24}$ of degree 24 trails in its wake myriad exotic and varied combinatorial structures. For example the Golay code [11] and the Leech lattice [7, 8], not to mention the many sporadic simple groups such as the other four Mathieu groups and Conway’s largest simple group which have close ties with $M_{24}$. Arguably though the most fundamental combinatorial object is the Steiner system S(5, 8, 24) of which $M_{24}$ is its automorphism group. This slant on $M_{24}$ was first revealed by Witt in [13, 14]. Let $\Omega$ be a 24-element set, equipped with this Steiner system. The blocks of this system will be referred to as octads, and we denote the set of octads of $\Omega$ by ${\mathcal {O}}$. We shall make extensive use of Curtis’s MOG and shall assume that $\Omega$ has the Steiner system as described in [9]. Our principal interest here is in octad triples, by which we mean a subset of ${\mathcal {O}}$ of size 3. Indeed, an octad triple $\{X_1, X_2, X_3\}$ in which $X_i \cap X_j = \emptyset$ for $i \ne j$ is called a trio and has already appeared in the literature in relation to the subgroup structure of $M_{24}$ [9, 10]. Trios also make appearances in various group geometries [12]. The main aim of this paper is to analyse the $M_{24}$-orbits of the set of octad triples. While this is of independent interest, this investigation was prompted by Boston [2] as these results will have application to various questions in the area of coding theory concerning pseudocodewords of AGWN pseudoweight less than 8 in the extended Golay code. For further details the reader may consult Boston [3] and Calderbank et al. [5]. And for other papers which also enumerate $M_{24}$-orbits on sets related to $\Omega$ see Choi [6] and Brouwer et al. [4].

We shall use ${{\mathcal {O}}}^c_{\{c_{12}, c_{13}, c_{23}\}}$ to denote the set of octad triples $\{X_1, X_2, X_3\}$ for which $|X_i \cap X_j|= c_{ij}, 1 \le i < j \le 3$ and $|X_1 \cap X_2 \cap X_3| = c$. Our main result is as follows.

Theorem 1.1

$M_{24}$ has 16 orbits on the set of octad triples,  the orbits being listed below.

$M_{24}$-orbits	Size	Representative triple
${\mathcal {O}}^0_{\{0,0,0\}}$	3795	$\{Y_1, Y_{2}, Y_{3}\}$
${\mathcal {O}}^0_{\{0,0,4\}}$	318,780	$\{Y_1, Y_3, Y_{4}\}$
${\mathcal {O}}^0_{\{0,2,2\}}$	2,550,240	$\{Y_1, Y_2, Y_{5}\}$
${\mathcal {O}}^0_{\{0,2,4\}}$	5,100,480	$\{Y_1, Y_3, Y_{5}\}$
${\mathcal {O}}^0_{\{0,4,4\}}$	318,780	$\{Y_1, Y_2, Y_{4}\}$
${\mathcal {O}}^0_{\{2,2,2\}}$	10,200,960	$\{Y_1, Y_5, Y_{6}\}$
${\mathcal {O}}^1_{\{2,2,2\}}$	4,080,384	$\{Y_1, Y_5, Y_{7}\}$
${\mathcal {O}}^0_{\{2,2,4\}}$	7,650,720	$\{Y_1, Y_5, Y_8\}$
${\mathcal {O}}^1_{\{2,2,4\}}$	20,401,920	$\{Y_1, Y_5, Y_{9}\}$
${\mathcal {O}}^2_{\{2,2,4\}}$	2,550,240	$\{Y_1, Y_5, Y_{10}\}$
${\mathcal {O}}^1_{\{2,4,4\}}$	6,800,640	$\{Y_1, Y_4, Y_{11}\}$
${\mathcal {O}}^2_{\{2,4,4\}}$	7,650,720	$\{Y_1, Y_4, Y_{12}\}$
${\mathcal {O}}^0_{\{4,4,4\}}$	35,420	$\{Y_1, Y_4, Y_8\}$
${\mathcal {O}}^2_{\{4,4,4\}}$	2,550,240	$\{Y_1, Y_4, Y_{13}\}$
${\mathcal {O}}^3_{\{4,4,4\}}$	2,266,880	$\{Y_1, Y_4, Y_{14}\}$
${\mathcal {O}}^4_{\{4,4,4\}}$	106,260	$\{Y_1, Y_4, Y_{10}\}$

The octads $Y_1,\ldots ,Y_{14}$ appearing in Theorem 1.1 are described in Sect. 3. It is interesting to observe that the intersection data suffices to describe the $M_{24}$-orbits. The $M_{24}$-orbits on sets of ${\mathcal {O}}$ of size two have long been known, see Lemma 2.1, and they are also determined by their intersection data. The remainder of this section introduces the notation and terminology we shall be using. As indicated earlier we shall be employing the MOG (Figure 4; [9]) in proving Theorem 1.1 and we recommend the reader has the MOG to hand. We note that the heavy bricks of [10] are named $Y_1, Y_2, Y_3$ here. We shall view the MOG array as a matrix and identify a particular member of it by (i, j) where it is in the ith row and jth column. Sometimes, it will be convenient to have names for the elements of $\Omega$ and we shall employ Curtis’s labelling as given in the (2, 1)th position of the MOG.

We shall have occasion to use sextets in our argument. Recall that a sextet is the disjoint union of 6 tetrads (tetrads being 4-element subsets of $\Omega$) with the property that the union of any two tetrads is an octad. We use the numbers $1,\ldots ,6$ in the MOG to indicate the tetrads. For example, means that $\{0, 14, 3, 15\}$, $\{ \infty , 8, 18, 20\}$ and so on are the tetrads of this sextet. Note that describes the same sextet.

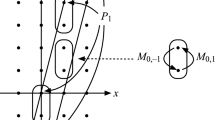
For $g \in M_{24}$, we use $fix_{\Omega }(g)$ to denote the elements of $\Omega$ fixed by g. We use pictures such as

to describe the involution of $M_{24}$ which is fixing $O_1=Y_1$ pointwise and interchanging those pairs of elements of $\Omega$ joined by a line. We recall that for $X \in {\mathcal {O}}$ and a 2-subset D of $\Omega \backslash X$ there is a unique involution $\tau$ in $M_{24}$ such that $fix_{\Omega } (\tau ) =X$ and $\tau$ interchanges the two elements in D, see [9].

2 Orbits on Octad Triples

We begin by recollecting some well known facts about S(5, 8, 24) and the action of $M_{24}$ on this Steiner system. For the remainder of this paper, we set $G=M_{24}$.

Lemma 2.1

Let $X \in {\mathcal {O}}$ and set $G_{X}=Stab_{G} X$. Then

• (i) G is transitive on ${\mathcal {O}}$ and $| {\mathcal {O}} | =759;$

• (ii) $G_X$ is transitive on $\{ Y \mid Y \in {\mathcal {O}}, X \cap Y = \emptyset \}$ which consists of 30 octads; 

• (iii) $G_X$ is transitive on $\{ Y \mid Y \in {\mathcal {O}}, |X \cap Y| = 2\}$ which consists of 448 octads;  and

• (iv) $G_X$ is transitive on $\{ Y \mid Y \in {\mathcal {O}}, |X \cap Y| = 4\}$ which consists of 280 octads.

Proof

See Lemma 19.2 (1)–(3) of [1]. $\square$

Lemma 2.2

Let $X,Y \in {\mathcal {O}}$ with $|X \cap Y| = 2$ and set $K=Stab_G X \cap Stab_G Y$. Then $K \cong Sym(6)$ with K acting in its usual degree 6 representation on $X \backslash (X \cap Y)$ and $Y \backslash (X \cap Y)$.

Proof

See Lemma 19.2(5) of [1]. $\square$

Proof of Theorem 1.1

Throughout we take T to be the octad triple $\{X_1, X_2, X_3\}$ and $H = \bigcap _{i=1}^3 Stab_G (X_i)$. Because the triples are not an ordered set we need to avoid double counting for cases such as ${\mathcal {O}}_{\{0,2,2\}}^0$ and ${\mathcal {O}}_{\{2,2,2\}}^0$, and so we divide by 2 or $3!=6$ as appropriate when counting.

The set ${\mathcal {O}}_{\{0,0,0\}}^0$ is just the set of trios of $\Omega$ and is well known to be a G-orbit of size 3795 (see, for example, [9] or Lemma 20.2 of [1]).

Let $T=\{ X_1, X_2, X_3\}$ be an octad triple in ${\mathcal {O}}_{\{0,0,4\}}^0$. Since G is transitive on ${\mathcal {O}}$, we may assume that $X_1 = Y_1$. By Lemma 2.1(iv) we may also assume $X_2 = Y_{13}$. As $|X_1 \cap X_3| =0=|X_2 \cap X_3|$ there are now three choices for $X_3$, namely

Therefore

$$\begin{aligned} |{\mathcal {O}}^0_{\{0,0,4\}} | = \frac{759 \cdot 280 \cdot 3}{2} = 318{,}780. \end{aligned}$$

$\rho \in Stab_GX_1 \cap Stab_G X_2$, so ${\mathcal {O}}_{\{0,0,4\}}^0$ is a G-orbit.

Suppose $\{X_1, X_2, X_3\} \in {\mathcal {O}}_{\{0,2,2\}}^0 \cup {\mathcal {O}}_{\{0,2,4\}}^0$. Then by Lemma 2.1 we may suppose $X_1= Y_1$ and $X_2 = Y_{5}$. By Lemma 2.2$Stab_G X_1\cap Stab_G X_2 \cong Sym(6)$ acts as Sym(6) on $X_2 \backslash (X_1 \cap X_2).$ In particular, $Stab_G X_1 \cap Stab_G X_2$ acts transitively on the 2-sets and 4-sets of $X_2 \backslash (X_1 \cap X_2).$ Now for a given 2-set, respectively, 4-set there is a unique $X_3 \in {\mathcal {O}}$ such that $|X_1 \cap X_3| = 0$ and $|X_2 \cap X_3| =2$, respectively, $|X_2 \cap X_3| =4$. Hence

$$\begin{aligned} |{\mathcal {O}}_{\{0,2,2\}}^0 |= & {} \frac{759 \cdot 448 \cdot 15}{2} = 2{,}550{,}240,\quad \text {and}\\ |{\mathcal {O}}_{\{0,2,4\}}^0 |= & {} 759 \cdot 448 \cdot 15 = 5{,}100{,}480 \end{aligned}$$

with each of ${\mathcal {O}}_{\{0,2,2\}}^0$ and ${\mathcal {O}}_{\{0,2,4\}}^0$ being G-orbits.

Next we consider ${\mathcal {O}}^0_{\{0,4,4\}}$. If $\{ X_1, X_2, X_3\}$ is an octad triple in this set, we may, without loss take $X_1=Y_1$ and $X_2=Y_4$, whence there are three possible choices for $X_3$

So

$$\begin{aligned} |{\mathcal {O}}^0_{\{0,4,4\}}| = \frac{759 \cdot 280 \cdot 3}{2} = 318{,}780. \end{aligned}$$

Considering the sextet , by the structure of the sextet stabilizer, [9], there exists a $g \in G$ which induces (4, 5, 6) on the tetrads of S. Since $g \in Stab_G X_1 \cap Stab_G X_2$, we infer that ${\mathcal {O}}^0_{\{0,4,4\}}$ is a G-orbit.

Consider $T=\{X_1, X_2, X_3 \} \in {\mathcal {O}}_{\{2,2,2\}}^1$. Without loss we can take $X_1= Y_1$ and $X_2=Y_5$, and so we know . In order to have $| X_1 \cap X_2 \cap X_3|=1$ we need one point of $X_3$ in $\{ \infty , 14\}$ and one in $\{ 0, 8, 3, 20, 15, 18\}$. These choices are independent and so we have $2 \cdot 6 =12$ choices for $X_1 \cap X_3$. Since $L=Stab_G X_1 \cap Stab_G X_2$ is transitive on 2-subsets D of $X_1$ with $|D \cap X_1 \cap X_2| = 1 = | D \cap (X_1 \backslash (X_1 \cap X_2 ) |$, we may further suppose that . By looking at the MOG we can find which octads have this as the first brick and $|X_2 \cap X_3|=2$. We obtain the following octads.

From (1, 5) we obtain , and and (1, 6) gives , (4, 5) gives , (4, 6) gives . With this choice of the first brick there are 6 octads and so $12 \cdot 6 =72$ choices in total when $X_1$ and $X_2$ are fixed. This means there are $\frac{759 \cdot 448 \cdot 72}{6}=4{,}080{,}384$ triples in ${\mathcal {O}}_{\{2,2,2\}}^1$.

Again using the transitively of L on 2-subsets D with $|D \cap X_1 \cap X_2 | =1= |D \cap ( X_1 \backslash (X_1 \cap X_2)|$, we may assume that . Hence, when choosing $X_3$ we must have that $X_3 \cap (X_2 \backslash X_1)$ consists of one element.

Let . Observe that $Stab_G T / H$ will be a subgroup of Sym(3). Hence, as $|{\mathcal {O}}^1_{\{2,2,2\}}| =4{,}080{,}384$, $|Stab_G T| \ge 2^2 \cdot 3 \cdot 5$. Note that H leaves invariant and consequently must leave invariant the sextet . Furthermore H must fix $\infty$ and 0, and so $H \le (Stab_G X_1 \cap Stab_G X_2)_{0, \infty } \cong Alt(5)$.

Suppose that $|H| > 2 \cdot 5$. Then we must have that $H \cong Alt(4)$ or Alt(5). In particular H must contain an element g of order 3 with cycle type $1^2 \cdot 3^1$ on $X_1 \backslash \{ \infty , 14,0\}$. Note that $\{ \infty , 14, 0, 17\} \subseteq fix_ {\Omega } (g)$. If, say, g fixes 3 and 8, then, as g leaves $X_2$ invariant, it must also fix 1 and 11. But then $| fix_{\Omega } (g)| \ge 8$, contrary to [9]. Thus $|H| \le 2 \cdot 5$ and this then forces $|H| = 2 \cdot 5$ and $Stab_G T / H \cong Sym(3)$. Therefore ${\mathcal {O}}^1_{\{2,2,2\}}$ is a G-orbit.

Consider $T=\{ X_1, X_2, X_3 \} \in {\mathcal {O}}^0_{\{2,2,2\}}$ with $X_1=Y_1$ and $X_2=Y_5$. So , and hence we know the first brick of $X_3$ must have 2 points in $\{0,8,3,20,15,18\}$. This means we have $\left( {\begin{array}{c}6\\ 2\end{array}}\right) =15$ choices as to where to put our two points. Without loss of generality we can choose the two points to be $\{0,8\}$. Searching among the square tetrads of the MOG we find that (3, 1),  (4, 1), (5, 1) and (6, 1) have this as their first brick in the square tetrad. Using the condition that $|X_2 \cap X_3| =2$ we find the following octads. From (3, 1) we obtain $\{0,8,4,16,10,11, 1,9\}$ and $\{0,8,17,13,7,2,1,9\}$ and from (4, 1) we find $\{0,8,17,16,10,13,22,19\}$ and $\{0,8,4,22,7,2,22,19\}$. While (5, 1) gives $\{0,8,10,2,22,1,21,5\}$, $\{0,8,10,2,12,19,9,6\}$, $\{0,8,16,2,11,13,21,6\}$, and $\{0,8,17,4,10,7,21,6\}$. And finally (6, 1) gives $\{0,8,16,7,21,19,9,5\}$, $\{0,8,16,7,22,1,12,6\}$, $\{0,8,10,11,13,7,12,5\}$, and $\{0,8,17,4,16,2,12,5\}$. Therefore for this particular choice for the first brick there are 12 choices for octads and so there are $12 \cdot 15 = 180$ choices for $X_3$ when $X_1$ and $X_2$ are fixed. In total there are $\frac{759 \cdot 448 \cdot 180}{6}=10{,}200{,}960$ triples in ${\mathcal {O}}^0_{\{2,2,2\}}.$

Having determined $|{\mathcal {O}}^0_{\{2,2,2\}}|$, we now show that ${\mathcal {O}}^0_{\{2,2,2\}}$ is a G-orbit. Choose . So $T=\{X_1, X_2, X_3\} \in {\mathcal {O}}^0_{\{2,2,2\}}$. We shall prove that $|H|=4$. Let $1 \ne g \in H$, and note that g leaves $\{ \infty , 14\}$, $\{0,8\}$ and $\{9,11\}$ invariant. So g leaves the tetrads $\{ \infty , 14, 0,8 \}$ and $\{5,6,17,22\}$ invariant and hence fixes the sextets and . Thus g must leave all the tetrads of $S_1$ invariant, excluding $T_1=\{17,11,4,13\}$ and $T_2=\{22,19,1,9\}$. The latter two tetrads are either left invariant or interchanged by g. Suppose g leaves $\{11,9\}$, $\{17,1\}$ and $\{4,1\}$ invariant, we conclude that g fixes $Y=\{17,11,4,13,22,19,1,9\}$ point-wise. Therefore g is an involution with $fix_\Omega (g)=Y$, and, since g must then interchange $\infty$ and 14, we infer

Next consider the case where g interchanges $T_1$ and $T_2$. On $T_1 \cup T_2$, g must act as

This is impossible as $\underrightarrow{g}$ which is not an octad, so $g \notin G$. Therefore g has fixed points and, as it fixes $S_2$, there are two possibilities for g

As a consequence $|H|=4$. From $|Stab_G T/ H| \le 6$ and $|{\mathcal {O}}^0_{\{2,2,2\}}|$ we now infer that $|Stab_G T| =24$ and that ${\mathcal {O}}^0_{\{2,2,2\}}$ is a G-orbit.

If we let $\{ X_1, X_2, X_3 \} \in {\mathcal {O}}^2_{\{2,2,4\}}$ with $X_1= Y_1$ and $X_2= Y_5$, then . As $|X_1 \cap X_3| =4$ we can assume that the first block is the heavy brick, and we also know that $X_1 \cap X_2$ is a subset of $X_3$. We can search among the MOG for the corresponding heavy bricks. We find (1, 1),  (1, 2),  (2, 2),  (2, 3),  (3, 2),  (3, 3),  (4, 4),  (4, 5),  (4, 6),  (5, 4),  (5, 5),  (5, 6),  (6, 4),  (6, 5),  (6, 6) all have the heavy brick we want. With the further intersection conditions we find that each give one octad that satisfies all the conditions on $X_3.$ For example (1, 1) gives the octad $\{ \infty , 14, 20, 18, 16, 2, 1, 19 \}$. So given $X_1$ and $X_2$ we have 15 choices for $X_3$ and so

$$\begin{aligned} |{\mathcal {O}}^2_{\{2,2,4\}}| = \frac{759 \cdot 448 \cdot 15}{2} = 2{,}550{,}240. \end{aligned}$$

Let $T=\{X_1, X_2, X_3 \} \in {\mathcal {O}}^2_{\{2,2,4\}}$ with $X_1 = Y_1$, $X_2= Y_5$. So $|X_1 \cap X_2 \cap X_3 | = \{\infty , 14\}$. Now $Stab_G X_1 \cap Stab_G X_2 \cong Sym(6)$ is transitive on 2-subsets of $X_1 \backslash \{ \infty , 14\}$. If we pick a 2-subset D of $X_1 \backslash \{ \infty , 14\}$, then there is exactly one octad X such that $X \cap X_1 = \{ \infty , 14\} \cup D$ and $X \cap X_2 \backslash \{ \infty , 14\} = \emptyset$. Hence ${\mathcal {O}}^2_{\{2,2,4\}}$ is a G-orbit.

Let $T= \{X_1, X_2, X_3\} \in {\mathcal {O}}^1_{\{2,2,4\}}$ with $X_1 = Y_1$ and $X_2 = Y_5$ then the first brick of $X_3$ can either have the point $\infty$ and not 14, or 14 and not $\infty$. Consider the first case and search among the MOG for the corresponding heavy bricks. We find (1, 3),  (1, 4),  (1, 5),  (1, 6),  (2, 4),  (2, 5),  (2, 6),  (3, 1),  (3, 4),  (3, 5),  (3, 6),  (4, 1),  (4, 2),  (4, 3),  (5, 1),  (5, 2),  (5, 3),  (6, 1),  (6, 2),  (6, 3). Each of these 20 choices give 3 octads which satisfy the conditions on $X_3$. So for this choice of the heavy brick we have $20 \cdot 3 = 60$ choices for $X_3$. We could have also chosen to have 14 but not $\infty$ in the heavy brick, so when $X_1$ and $X_2$ are fixed we have $60 \cdot 2 =120$ choices for $X_3$. And so

$$\begin{aligned} | {\mathcal {O}}^1_{\{2,2,4\}}| = \frac{759 \cdot 448 \cdot 120}{2} = 20{,}401{,}920. \end{aligned}$$

Since $L= Stab_G X_1 \cap Stab_G X_2 \cong Sym(6)$ is transitive on 4-sets $F \subseteq X_1$ with $|F \cap \{ \infty , 14\}|=1$, we may suppose $X_1 \cap X_3 = \{ \infty , 0, 3, 15\}$. We also have $H \le Stab_L (X_1 \cap X_3) \sim 3^2 \cdot 2$. Let $g \in Stab_L (X_1 \cap X_3)$ be of order 3. Suppose that g has cycle type $1^3 \cdot 3^1$ on $X_1 \backslash \{ \infty , 14 \}$. Then $fix_\Omega (g)= \{ \infty , 14, 17, 0,3,15\}$ or $\{ \infty , 14, 17, 8,20,18\}$. From [9] (see Corollary 2), we have that g cycles $\{11,22,19\}$. But then $g \notin Stab_G X_2$. Thus g cannot have cycle type $1^3 \cdot 3^1$ on $X_1 \backslash \{ \infty , 14 \}$ and hence $|H| \le 3 \cdot 2$. From $|{\mathcal {O}}^1_{\{2,2,4\}}|$ we now conclude that $|Stab_G T| = 2^2 \cdot 3$ and that ${\mathcal {O}}^1_{\{2,2,4\}}$ is a G-orbit.

Let $\{X_1, X_2, X_3\} \in {\mathcal {O}}^0_{\{2,2,4\}}$ with $X_1=Y_1$ and $X_2=Y_5$. We need $|X_1 \cap X_3| =4$ and $|X_1 \cap X_2 \cap X_3| =0$ and so the first brick of $X_3$ needs 4 points in $\{0,8,3,20,15,18\}$. Since $L=Stab_G X_1 \cap Stab_G X_2 \cong Sym(6)$ is transitive on the $\left( {\begin{array}{c}6\\ 4\end{array}}\right) =15$ 4-sets of $X_1 \backslash \{ \infty , 14\}$, without loss we may suppose $X_3 \cap X_1 = \{3,20,15,8\}$. This only corresponds to MOG picture (4,4). Using $|X_2 \cap X_3| =2$ we find 3 choices for $X_3$, namely

So with $X_1$ and $X_2$ fixed we have $15 \cdot 3 =45$ choices for $X_3$, which means that

$$\begin{aligned} |{\mathcal {O}}^0_{\{2,2,4\}}| = \frac{759 \cdot 448 \cdot 45}{2} = 7{,}650{,}720. \end{aligned}$$

Again using the fact that L is transitive on 4-sets of $X_1 \backslash \{ \infty , 14\}$, we may suppose $X_3 \cap X_1 = \{3,20,15,8\}$. Then $Stab_L (X_3 \cap X_1) \cong 2 \times Sym(4)$ fixes the sextet .

Let $g \in Stab_L(X_3 \cap X_1)$ with g of order 3. We see that g leaves the tetrads $\{\infty , 14, 0, 8\}$, $\{3,20, 15, 18\}$, and $\{16,7,10,2\}$ invariant (the latter because it is the only tetrad in $\Omega \backslash X_1$ missing $X_2$). So g cycles the remaining three tetrads. Now the possible choices for $X_3$ are

whence ${\mathcal {O}}^0_{\{2,2,4\}}$ is a G-orbit.

We next determine $|{\mathcal {O}}^1_{\{2,4,4\}}|$ and $|{\mathcal {O}}^2_{\{2,4,4\}}|$. Let $\{X_1, X_2, X_3\} \in {\mathcal {O}}^1_{\{2,4,4\}}$ with $X_1 = Y_1$ and $X_2 =Y_4$. Recall that $L= Stab_G X_1 \cap Stab_G X_2$ is transitive on 2 subsets D of $X_1$ such that $|D \cap ( X_1 \cap X_2)| = 1 = | D \cap X_1 \backslash X_2|$. So we may assume and searching among the MOG for a heavy brick with only 14 in its top block we find the pictures (2, 5),  (3, 6),  (5, 3),  (6, 2). The condition that $|X_2 \cap X_3| =2$ doesn’t restrict $X_3$ any further, and so for each picture we obtain all 4 possibilities for the MOG and so $4 \cdot 4 =16$ octads for this choice for top block of the heavy brick. We have 4 possibilities for the positioning of the single point in the top block of the heavy brick, and so $4 \cdot 16 = 64$ choices for $X_3$ when $X_1$ and $X_2$ are fixed as above. Therefore

$$\begin{aligned} |{\mathcal {O}}^1_{\{2,4,4\}}|= \frac{759 \cdot 280 \cdot 64}{2} = 6{,}800{,}640. \end{aligned}$$

Let $\{X_1, X_2, X_3\} \in {\mathcal {O}}^2_{\{2,4,4\}}$ with $X_{1} = Y_1$ and $X_{2} = Y_4$ Therefore . Because $L= Stab_G X_1 \cap Stab_G X_2$ is transitive on the $\left( {\begin{array}{c}4\\ 2\end{array}}\right) =6$ 2-element subsets of Z, without loss we can assume . To find $X_3$ we now search among the MOG for heavy bricks that only have $\infty$ and 4 in their top blocks. We find these are (1, 1),  (1, 2),  (4, 5),  (4, 6),  (5, 4),  and (6, 4). Using the further condition that $|X_3 \cap X_2|=2$ gives that each of these MOG pictures offers 2 choices for $X_3$. For example (1, 1) gives $\{ \infty , 14, 20,18, 16, 2, 1, 19\}$ and $\{ \infty , 14, 20,18, 10, 7, 22,9\}$. So with this choice of 2 element subset we find 12 octads, and therefore we have $12 \cdot 6 =72$ choices for $X_3$ when $X_1$ and $X_2$ are fixed. Hence

$$\begin{aligned} |{\mathcal {O}}^2_{\{2,4,4\}}| = \frac{759 \cdot 280 \cdot 72}{2} = 7{,}650{,}720. \end{aligned}$$

Let $T=\{X_1, X_2, X_3\}\in {\mathcal {O}}^1_{\{2,4,4\}} \cup {\mathcal {O}}^2_{\{2,4,4\}}$. Without loss we may suppose $X_1 = Y_1$ and $X_2 = Y_4$.

First we look at ${\mathcal {O}}_{\{2,4,4\}}^1$ and select $X_3 = Y_{11}$. Note that $X_1 \cap X_2 \cap X_3 = \{14\}$. Now $L=Stab_G X_2 \cap Stab_G X_3 \cong Sym(6)$ and $H \le Stab_L \{3,20,15 \} \cap Stab_L \{14\}$, and therefore $|H| \big |2 \cdot 3^2$. Taking into account $| {\mathcal {O}}^1_{\{2,4,4\}}|$, we infer that $|Stab_G T| = 2^2 \cdot 3^2$ and hence that ${\mathcal {O}}^1_{\{2,4,4\}}$ is a G-orbit.

For $T \in {\mathcal {O}}^2_{\{2,4,4\}}$, we choose . So $X_1 \cap X_2 \cap X_3 =\{ \infty , 14\}$, $X_1 \cap X_2 = \{ \infty , 14, 0, 8\}$ and $X_1 \cap X_3 = \{ \infty , 14, 3 ,20 \}$. Consequently $H \le Stab_L \{ \infty , 14\} \cap Stab_L \{0,8\} \cap Stab_L \{3,20\} \cap Stab_L\{15, 18\}$ where $L=Stab_G X_1$ and hence $|H| \big |2^7$. A similar argument gives $H \le Stab_L \{22,19\} \cap Stab_L\{12,5\}$ and consequently $H \cap O_2 (L) \le \langle (11,7)(4,13)(7,16)(2,10)(19,22)(1,9)(5,12)(6,21) \rangle$. So $|H| \big |2^4$ and, as $|Stab_G T / H| \le 2$, we must have $|Stab_G T|=2^5$ with ${\mathcal {O}}^2_{\{2,4,4\}}$ being a G-orbit.

Let $T=\{X_1, X_2, X_3\} \in {\mathcal {O}}^0_{\{4,4,4\}}$ with $X_1 = Y_1$ and $X_2 = Y_4$, therefore . In order to have $|X_1 \cap X_3|=4$, $|X_2 \cap X_3|=4$ and $|X_1 \cap X_2 \cap X_3|=0$ with this choice of $X_1$ and $X_2$ we only have the one possibility . Consequently

$$\begin{aligned} |{\mathcal {O}}^0_{\{4,4,4\}}| = \frac{759 \cdot 280 \cdot 1}{6}=35{,}420. \end{aligned}$$

In addition as there is only one choice for $X_3$ clearly ${\mathcal {O}}^0_{\{4,4,4\}}$ is a G-orbit.

For $T=\{X_1, X_2, X_3\} \in {\mathcal {O}}^2_{\{4,4,4\}}$ choose $X_1 = Y_1$ and $X_2 = Y_4$. As $|X_1 \cap X_2 \cap X_3|=2$ we need 2 points of $X_3$ in $\{ \infty , 14, 0,8\}$ and so there are $\left( {\begin{array}{c}4\\ 2\end{array}}\right) =6$ possibilities. Consider the case where the two points are $\{0, 14\}$. We need another 2 points in this brick so can assume its the heavy block. Searching among the MOG we find that (1, 5),  (1, 6),  (2, 4),  (3, 1),  (3, 4),  and (4, 1) have a heavy block of the right type. The condition that $|X_3 \cap X_2| =4$ each of the MOG pictures gives 2 options for $X_3$. So when $X_1$ and $X_2$ are fixed there are $6 \cdot 12 = 72$ choices for $X_3$, therefore

$$\begin{aligned} |{\mathcal {O}}^2_{\{4,4,4\}}|=\frac{759 \cdot 280 \cdot 72}{6} = 2{,}550{,}240. \end{aligned}$$

Let $X_3 = Y_{13}$. So $T \in {\mathcal {O}}^2_{\{4,4,4\}}$ and $X_1 \cap X_2 \cap X_3 = \{0,14\}$, $X_1 \cap X_3 = \{0, 14, 3, 15\}$, $X_2 \cap X_3 = \{0,14,11,17\}$. Consequently $H \le Stab_L \{0,14\} \cap Stab_L \{ \infty , 8\} \cap Stab_L \{3,15\} \cap Stab_L \{11,17\}$ where $L=Stab_GX_1$ and so, just as in the case of ${\mathcal {O}}^2_{\{2,4,4\}}$, we conclude that $|H| \big |2^4$. Since $|Stab_G T / H| \big |6$, we must have $|Stab_G T| = 2^5 \cdot 3$ and thus using $|{\mathcal {O}}^2_{\{4,4,4\}}|$ we have that ${\mathcal {O}}^2_{\{4,4,4\}}$ is a G-orbit.

Let $T=\{X_1, X_2, X_3\} \in {\mathcal {O}}^3_{\{4,4,4\}}$ with $X_1 = Y_1$ and $X_2 = Y_4$. As $|X_1 \cap X_2 \cap X_3|=3$ we need 3 points of $X_3$ in $\{ \infty , 14, 0,8\}$ and so there are 4 possibilities.

Consider the case where the 3 points are $\{ \infty , 14, 8\}$, we can then search among the MOG for heavy bricks of this form and find (2, 2),  (3, 3),  (5, 6),  (6, 5). Each of these options give 4 possibilities for $X_3$ and so there are $4 \cdot 4 =16$ possibilities for $X_3$ with this choice of top block of the first brick. This then gives $4 \cdot 16 = 64$ choices for $X_3$ when $X_1$ and $X_2$ are fixed as above. Therefore

$$\begin{aligned} |{\mathcal {O}}^3_{\{4,4,4\}}|=\frac{759 \cdot 280 \cdot 64}{6} = 2{,}266{,}880. \end{aligned}$$

Choosing , gives $T \in {\mathcal {O}}^3_{\{4,4,4\}}$. Taking account of the various intersections we deduce that H leaves $\{ \infty , 14, 8\}$, $\{3, 15, 18\}$, $\{4, 11, 13\}$ and $\{2,9,12\}$ invariant and fixes 0, 20 and 17. Put $L = Stab_G X_1$. From H fixing 17 we have $H \cap O_2(L) =1$, and so by the action of H on $X_1$ we see that $|H| \big |2 \cdot 3^2$. Using $|{\mathcal {O}}^3_{\{4,4,4\}}|$ we infer that $|H| = 2 \cdot 3^2$ and that ${\mathcal {O}}^3_{\{4,4,4\}}$ is a G-orbit.

Now let $T=\{X_1, X_2, X_3\} \in {\mathcal {O}}^4_{\{4,4,4\}}$ with $X_1 = Y_1$ and $X_2 = Y_4$. In order to have $|X_1 \cap X_2 \cap X_3|=4$ we need to be the first block of $X_3$. Consulting the MOG we only have (4, 4) as an option. The further condition that $|X_2 \cap X_3| =4$ gives 3 choices for $X_3$ with this choice of $X_1$ and $X_2$ which are , and so

$$\begin{aligned} |{\mathcal {O}}^4_{\{4,4,4\}} |= \frac{759 \cdot 280 \cdot 3}{6} = 106{,}260. \end{aligned}$$

It follows easily as in case of ${\mathcal {O}}_{\{2,2,4\}}^0$ that ${\mathcal {O}}^4_{\{4,4,4\}}$ is a G-orbit.

There are $\left( {\begin{array}{c}759\\ 3\end{array}}\right) = 72{,}586{,}459$ octad triples. By summing the sizes of the G-orbits we’ve found so far we can see that we have covered all of the triples. This completes the proof of Theorem 1.1. $\square$

3 A Few Octads