A Characterization of Q-Polynomial Distance-Regular Graphs Using the Intersection Numbers

Abstract

We consider a primitive distance-regular graph $\varGamma$ with diameter at least 3. We use the intersection numbers of $\varGamma$ to find a positive semidefinite matrix G with integer entries. We show that G has determinant zero if and only if $\varGamma$ is Q-polynomial.

Appendices

Appendix 1

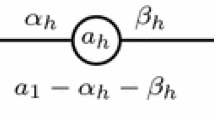
Recall the distance-regular graph $\varGamma$ with diameter D. Recall for $0\le h \le D$

$$\begin{aligned} \begin{array}{lll} p^h_{1,h-1}=c_h, &{} \quad p^h_{1h}=a_h, &{} \quad p^h_{1,h+1}=b_h, \\ p^1_{h,h-1}=\dfrac{k_hc_h}{k}, &{} \quad p^1_{hh}=\dfrac{k_ha_h}{k}, &{} \quad p^1_{h,h+1}=\dfrac{k_hb_h}{k}.\\ \end{array} \end{aligned}$$

We now give $p^h_{2j}$ for $h-2\le j \le h+2$.

$$\begin{aligned}&p^h_{2,h-2}=\dfrac{c_{h-1}c_h}{c_2}, \\&p^h_{2,h-1}=\dfrac{c_h(a_{h-1}+a_h-a_1)}{c_2}, \\&p^h_{2h}= \dfrac{c_h(b_{h-1}-1)+a_h(a_h-a_1-1)+b_h(c_{h+1}-1)}{c_2}, \\&p^h_{2,h+1}= \dfrac{b_h(a_{h+1}+a_h-a_1)}{c_2}, \\&p^h_{2,h+2}= \dfrac{b_hb_{h+1}}{c_2}. \end{aligned}$$

We now give $p^h_{3j}$ for $h-3\le j \le h+3$.

$$\begin{aligned} p^h_{3,h-3}= & {} \dfrac{c_{h-2}c_{h-1}c_h}{c_2c_3},\\ p^h_{3,h-2}= & {} \dfrac{(a_h-a_2)c_{h-1}c_h}{c_2c_3}+\dfrac{c_{h-1}c_h(a_{h-2}+a_{h-1}-a_1)}{c_2c_3},\\ p^h_{3,h-1}= & {} \dfrac{c_{h-1}c_h(b_{h-2}-1)+c_ha_{h-1}(a_{h-1}-a_1-1)+c_hb_{h-1}(c_h-1)}{c_2c_3}\\&+\,\dfrac{c_h(a_h-a_2)(a_{h-1}+a_h-a_1)}{c_2c_3}+\dfrac{b_hc_hc_{h+1}}{c_2c_3}-\dfrac{b_1c_h}{c_3}, \\ p^h_{3h}= & {} \dfrac{c_hb_{h-1}(a_{h}+a_{h-1}-a_1)}{c_2c_3}\\&+\,\dfrac{(a_h-a_2)(c_h(b_{h-1}-1)+a_h(a_h-a_1-1)+b_h(c_{h+1}-1))}{c_2c_3}\\&+\,\dfrac{b_hc_{h+1}(a_h+a_{h+1}-a_1)}{c_2c_3}-\dfrac{b_1a_h}{c_3}, \\ p^h_{3,h+1}= & {} \dfrac{c_hb_{h-1}b_h}{c_2c_3} +\dfrac{b_h(a_h-a_2)(a_{h+1}+a_h-a_1)}{c_2c_3}\\&+\,\dfrac{b_h(c_{h+1}(b_h-1)+a_{h+1}(a_{h+1}-a_1-1)+b_{h+1}(c_{h+2}-1))}{c_2c_3}-\dfrac{b_1b_h}{c_3}, \\ p^h_{3,h+2}= & {} \dfrac{(a_h-a_2)b_hb_{h+1}}{c_2c_3} +\dfrac{b_hb_{h+1}(a_{h+2}+a_{h+1}-a_1)}{c_2c_3}, \\ p^h_{3,h+3}= & {} \dfrac{b_hb_{h+1}b_{h+2}}{c_2c_3}. \end{aligned}$$

Appendix 2

Recall the matrix G from Theorem 1. In this appendix we give G for $D=3$.

Example 1

Assume $D=3$. The rows and columns of G are indexed by the following matrices, in the specified order:

So the matrix G is $12\times 12$. G has the form

$$\begin{aligned} G=\left[ \begin{array}{c@{\quad }c@{\quad }c@{\quad }c} \mathbb {X} &{} 0 &{} 0 &{} \mathbb {S}_1 \\ 0 &{} \mathbb {Y} &{} 0 &{} \mathbb {S}_2 \\ 0 &{} 0 &{} \mathbb {Z} &{} \mathbb {S}_3 \\ \mathbb {S}_1 &{} \mathbb {S}_2 &{} \mathbb {S}_3 &{} \mathbb {W} \\ \end{array}\right] , \end{aligned}$$

where each block is a $3\times 3$ symmetric matrix as shown below.

$$\begin{aligned} \mathbb {X}= & {} \left[ \begin{array}{c@{\quad }c@{\quad }c} 2k_3k &{} -2k_3c_3 &{} -2k_3a_3 \\ -2k_3c_3 &{} 2k_3(k_2-p^3_{22}) &{} -2k_3p^3_{23} \\ -2k_3a_3 &{} -2k_3p^3_{23} &{} 2k_3(k_3-p^3_{33}) \end{array}\right] ,\\ \mathbb {Y}= & {} \left[ \begin{array}{c@{\quad }c@{\quad }c} 2k_2(k-c_2) &{} -2k_2a_2 &{} -2k_2b_2 \\ -2k_2a_2 &{} 2k_2(k_2-p^2_{22}) &{} -2k_2p^2_{23} \\ -2k_2b_2 &{} -2k_2p^2_{23} &{} 2k_2(k_3-p^2_{33}) \end{array}\right] ,\\ \mathbb {Z}= & {} \left[ \begin{array}{c@{\quad }c@{\quad }c} 2k(k-a_1) &{} -2kb_1 &{} 0 \\ -2kb_1 &{} 2k(k_2-p^1_{22}) &{} -2kp^1_{23} \\ 0 &{} -2kp^1_{23} &{} 2k(k_3-p^1_{33}) \end{array}\right] ,\\ \mathbb {S}= & {} 2k_2 \left[ \begin{array}{ccc} a_1-c_2 &{} \quad b_1-a_2 &{}\quad -b_2 \\ b_1-a_2 &{}\quad p^1_{22}-p^2_{22} &{}\quad p^1_{23}-p^2_{23} \\ -b_2 &{}\quad p^1_{23}-p^2_{23} &{}\quad p^1_{33}-p^2_{33} \end{array}\right] , \end{aligned}$$

$\mathbb {S}_1=b_2\mathbb {S}$, $\mathbb {S}_2=a_2\mathbb {S}$ and $\mathbb {S}_3=c_2\mathbb {S}$.

The matrix $\mathbb {W}$ is symmetric with entries

$$\begin{aligned} {\mathbb W}_{11}= & {} 2(k^2k_2+(k_2a_1a_2-kb_1^2)a_1 +(k_2(c_2(b_1-1)+a_2(a_2-a_1-1)\\&+\,b_2(c_3-1))-k_2a_2^2)c_2),\\ {\mathbb W}_{12}= & {} 2((k_2a_1a_2-kb_1^2)b_1 +(k_2(c_2(b_1-1)+a_2(a_2-a_1-1)\\&+\,b_2(c_3-1)) -k_2a_2^2)a_2-k_3c_3^3),\\ {\mathbb W}_{13}= & {} 2((k_2(c_2(b_1-1)+a_2(a_2-a_1-1)+b_2(c_3-1))-k_2a_2^2)b_2-k_3c_3^2a_3),\\ {\mathbb W}_{22}= & {} 2(kk_2^2+(k_2a_1a_2-kb_1^2)p^1_{22} +(k_2(c_2(b_1-1)+a_2(a_2-a_1-1)\\&+\,b_2(c_3-1))-k_2a_2^2)p^2_{22}-k_3c_3^2p^3_{22}),\\ {\mathbb W}_{23}= & {} 2((k_2a_1a_2-kb_1^2)p^1_{23} +(k_2(c_2(b_1-1)+a_2(a_2-a_1-1)\\&+\,b_2(c_3-1))-k_2a_2^2)p^2_{23}-k_3c_3^2p^3_{23}),\\ {\mathbb W}_{33}= & {} 2(kk_2k_3+(k_2a_1a_2-kb_1^2)p^1_{33} +(k_2(c_2(b_1-1)+a_2(a_2-a_1-1)\\&+\,b_2(c_3-1))-k_2a_2^2)p^2_{33}-k_3c_3^2p^3_{33}). \end{aligned}$$

From Appendix 1, we find

$$\begin{aligned} p^1_{22}= & {} \dfrac{k_2a_2}{k},\quad p^2_{22}=\dfrac{c_2(b_1-1)+a_2(a_2-a_1-1)+b_2(c_3-1)}{c_2},\\ p^3_{22}= & {} \dfrac{c_3(a_2+a_3-a_1)}{c_2}, \\ p^1_{23}= & {} \dfrac{k_2b_2}{k},\quad p^2_{23}=\dfrac{b_2(a_3+a_2-a_1)}{c_2},\\ p^3_{23}= & {} \dfrac{c_3(b_2-1)+a_3(a_3-a_1-1)-b_3}{c_2}, \\ p^1_{33}= & {} \dfrac{k_3a_3}{k},\quad p^2_{33}=\dfrac{b_2(c_3(b_2-1)+a_3(a_3-a_1-1)-b_3)}{c_2c_3}, \\ p^3_{33}= & {} \dfrac{c_3b_{2}(a_3+a_2-a_1)}{c_2c_3} +\dfrac{(a_3-a_2)(c_3(b_2-1)+a_3(a_3-a_1-1)-b_3)}{c_2c_3}\\&-\,\dfrac{b_1a_3}{c_3}. \end{aligned}$$