Skip to main content
SpringerLink
Log in

An efficient spectral-Galerkin method for solving two-dimensional nonlinear system of advection–diffusion–reaction equations

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

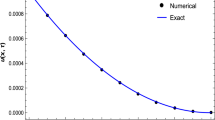
The spectral Legendre–Galerkin method for solving a two-dimensional nonlinear system of advection–diffusion–reaction equations on a rectangular domain is presented and compared with analytical solution. The proposed method is based on the Legendre–Galerkin formulation for the linear terms and computation of the nonlinear terms in the Chebyshev–Gauss–Lobatto points. The main difference of the spectral Legendre–Galerkin method presented in the current paper with the classic Legendre–Galerkin method is in treating the nonlinear terms and imposing boundary conditions. Indeed, in the spectral Legendre–Galerkin method the nonlinear terms are efficiently handled using the Chebyshev–Gauss–Lobatto points and also the boundary conditions are imposed strongly as collocation methods. Combination of the proposed method with a semi-implicit time integration method such as the Leapfrog–Crank–Nicolson scheme leads to reducing the complexity of computations and obtaining a linear algebraic system of equations. Efficiency and spectral accuracy of the proposed method are demonstrated numerically by some examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Alpert BK, Rokhlin V (1991) A fast algorithm for the evaluation of Legendre expansions. SIAM J Sci Stat Comput 12:158–179

    MathSciNet  MATH  Google Scholar 

  2. Boyd JP (2000) Chebyshev and Fourier spectral methods, 2nd edn. Dover, New York

    Google Scholar 

  3. Boyd JP (1994) Time-marching on the slow manifold: the relationship between the nonlinear Galerkin method and implicit timestepping algorithms. Appl Math Lett 7:95–99

    MathSciNet  MATH  Google Scholar 

  4. Caliari M, Vianello M, Bergamaschi L (2007) The LEM exponential integrator for advection–diffusion–reaction equations. J Comput Appl Math 210(1–2):56–63

    MathSciNet  MATH  Google Scholar 

  5. Canuto C, Hussaini MY, Quarteroni A, Zang TA (1988) Spectral methods in fluid dynamics. Springer, New York

    MATH  Google Scholar 

  6. Cencini M, Lopez C, Vergni D (2003) Reaction–diffusion systems: front propagation and spatial structures. Lect Notes Phys 636:187–210

    MathSciNet  MATH  Google Scholar 

  7. Dehghan M (2004) Numerical solution of the three-dimensional advection–diffusion equation. Appl Math Comput 150:5–19

    MathSciNet  MATH  Google Scholar 

  8. Dehghan M, Sabouri M (2013) A Legendre spectral element method on a large spatial domain to solve the predator–prey system modeling interacting populations. Appl Math Model 37:1028–1038

    MathSciNet  MATH  Google Scholar 

  9. Don WS, Gottlieb D (1994) The Chebyshev–Legendre method: implementing Legendre methods on Chebyshev points. SIAM J Numer Anal 31:1519–1534

    MathSciNet  MATH  Google Scholar 

  10. Elbarbary E (2008) Efficient Chebyshev–Petrov–Galerkin method for solving second-order equations. J Sci Comput 34:113–126

    MathSciNet  MATH  Google Scholar 

  11. El Alaoui L, Ern A (2006) Nonconforming finite element methods with subgrid viscosity applied to advection–diffusion–reaction equations. Numer Methods Partial Differ Equ 22(5):1106–1126

    MathSciNet  MATH  Google Scholar 

  12. Fakhar-Izadi F, Dehghan M (2013) An efficient pseudo-spectral Legendre Galerkin method for solving a nonlinear partial integro-differential equation arising in population dynamics. Math Methods Appl Sci 36(12):1485–1511

    MathSciNet  MATH  Google Scholar 

  13. Fazio R, Jannelli A (2009) Second order numerical operator splitting for 3D advection–diffusion–reaction models. In: Kreiss G et al (eds) Numerical mathematics and advanced applications. Springer, Berlin, pp 317–324

    MATH  Google Scholar 

  14. Fitzhugh R (1961) Impulses and physiological states in theoretical models of nerve membranes. J Biophys 1:445–466

    Google Scholar 

  15. Ganesh M, Mustapha K (2006) A fully discrete \(H^1\)-Galerkin method with quadrature for nonlinear advection–diffusion–reaction equations. Numer Algorithms 43:355–383

    MathSciNet  MATH  Google Scholar 

  16. Gottlieb D, Orszag SA (1997) Numerical analysis of spectral methods: theory and applications. SIAM-CMBS, Philadelphia

    MATH  Google Scholar 

  17. Gottlieb D, Xiu D (2008) Galerkin method for wave equations with uncertain coefficients. Commun Comput Phys 3(2):505–518

    MathSciNet  MATH  Google Scholar 

  18. Goubet O, Shen J (2007) On the dual Petrov–Galerkin formulation of the KdV equation. Adv Differ Equ 12:221–239

    MathSciNet  MATH  Google Scholar 

  19. Greengard L, Rokhlin V (1987) A fast algorithm for particle simulations. J Comput Phys 73:325–348

    MathSciNet  MATH  Google Scholar 

  20. Gu Y, Liao W, Zhu J (2003) An efficient high-order algorithm for solving systems of 3-D reaction–diffusion equations. J Comput Appl Math 155:1–17

    MathSciNet  MATH  Google Scholar 

  21. Guo B-Y, Shen J (2000) Laguerre–Galerkin method for nonlinear partial differential equations on a semi-infinite interval. Numer Math 86:635–654

    MathSciNet  MATH  Google Scholar 

  22. Guo B-Y (1998) Spectral methods and their applications. World Scientific, River Edge

    MATH  Google Scholar 

  23. Guo B-Y, Shen J, Wang L-L (2006) Optimal spectral-Galerkin methods using generalized Jacobi polynomials. J Sci Comput 27(1–3):305–322

    MathSciNet  MATH  Google Scholar 

  24. Hidalgo A, Dumbser M (2011) ADER schemes for nonlinear systems of stiff advection–diffusion–reaction equations. J Sci Comput 48:173–189

    MathSciNet  MATH  Google Scholar 

  25. Hoff D (1978) Stability and convergence of finite difference methods for systems of nonlinear reaction–diffusion. SIAM J Numer Anal 15:1161–1177

    MathSciNet  MATH  Google Scholar 

  26. Houston P, Schwab C, Süli E (2002) Discontinuous hp-finite element methods for advection–diffusion–reaction problems. SIAM J Numer Anal 39:2133–2163

    MathSciNet  MATH  Google Scholar 

  27. Houzeaux G, Eguzkitza B, Vázquez M (2009) A variational multiscale model for the advection–diffusion–reaction equation. Commun Numer Methods Eng 25:787–809

    MathSciNet  MATH  Google Scholar 

  28. Hrinca I (2002) An optimal control problem for the Lotka–Volterra system with diffusion. Panamer Math J 12(3):23–46

    MathSciNet  MATH  Google Scholar 

  29. Huang W (2001) Uniqueness of the bistable traveling wave for mutualist species. J Dyn Differ Equ 13(1):147–183

    MathSciNet  MATH  Google Scholar 

  30. Hundsdorfer W, Verwer JG (2003) Numerical solution of time-dependent advection–diffusion–reaction equations, vol 33. Springer series in computational mathematics. Springer, Berlin

    MATH  Google Scholar 

  31. Khan LA, Liu Philip L-F (1995) An operator splitting algorithm for coupled one-dimensional advection–diffusion–reaction equations. Comput Methods Appl Mech Eng 127:181–201

    MathSciNet  MATH  Google Scholar 

  32. Ladyzenskaja OA, Solonnikov VA, Uralceva NN (1968) Linear and quasi-linear equations of parabolic type, vol 23. Translations of mathematical monographs. American Mathematical Society, Providence

    Google Scholar 

  33. Lagzi I, Kármán D, Turányi T, Tomlin A, Haszpra L (2004) Simulation of the dispersion of nuclear contamination using an adaptive Eulerian grid model. J Environ Radioact 75:59–82

    Google Scholar 

  34. Lagzi I, Mészáros R, Horváth L, Tomlin A et al (2004) Modelling ozone fluxes over Hungary. Atmos Environ 38:6211–6222

    Google Scholar 

  35. Li H, Wu H, Ma H (2003) The Legendre Galerkin–Chebyshev collocation method for Burgers-like equations. IMA J Numer Anal 23:109–124

    MathSciNet  MATH  Google Scholar 

  36. Liao W, Zhu J, Khaliq Abdul QM (2002) An efficient high-order algorithm for solving systems of reaction–diffusion equations. Numer Methods Partial Differ Equ 18:340–354

    MathSciNet  MATH  Google Scholar 

  37. Liu B (2009) An error analysis of a finite element method for a system of nonlinear advection–diffusion–reaction equations. Appl Numer Math 59:1947–1959

    MathSciNet  MATH  Google Scholar 

  38. Liu B, Allen MB, Kojouharov H, Chen B (1996) Finite-element solution of reaction-diffusion equations with advection. In: Aldama AA et al (eds) Computational methods in water resources, vol 1. Computational methods in subsurface flow and transport problems. Computational Mechanics Publications, Southampton, pp 3–12

    Google Scholar 

  39. Lutscher F, McCauley E, Lewis MA (2007) Spatial patterns and coexistence mechanisms in systems with unidirectional flow. Theor Popul Biol 71:267–277

    MATH  Google Scholar 

  40. Ma HP (1998) Chebyshev–Legendre spectral viscosity method for nonlinear conservation laws. SIAM J Numer Anal 35:893–908

    MathSciNet  MATH  Google Scholar 

  41. Ma HP (1998) Chebyshev–Legendre super spectral viscosity method for nonlinear conservation laws. SIAM J Numer Anal 35:869–892

    MathSciNet  MATH  Google Scholar 

  42. Ma HP, Sun WW (2000) A Legendre–Petrov–Galerkin and Chebyshev collocation method for third-order differential equations. SIAM J Numer Anal 38:1425–1438

    MathSciNet  MATH  Google Scholar 

  43. Ma HP, Sun WW (2001) Optimal error estimates of the Legendre–Petrov–Galerkin method for the Korteweg–de Vries equation. SIAM J Numer Anal 39:1380–1394

    MathSciNet  MATH  Google Scholar 

  44. Matthies HG, Keese A (2005) Galerkin methods for linear and nonlinear elliptic stochastic partial differential equations. Comput Methods Appl Math Eng 194:1295–1331

    MathSciNet  MATH  Google Scholar 

  45. McKibbin R, Lim LL, Smith TA, Sweatman WL (2005) A model for dispersal of eruption ejecta. In: Proceedings world geothermal congress, April 24–29, Antalya, Turkey

  46. Mickens RE (2000) Nonstandard finite difference schemes for reaction–diffusion equations having linear advection. Numer Methods Partial Differ Equ 16:361–364

    MathSciNet  MATH  Google Scholar 

  47. Mohebbi A, Dehghan M (2010) High-order compact solution of the one-dimension heat and advection–diffusion equation. Appl Math Model 34:3071–3084

    MathSciNet  MATH  Google Scholar 

  48. Nakagaki T, Yamada H, Ito M (1999) Reaction–diffusion–advection model for pattern formation of rhythmic contraction in a giant amoeboid cell of the Physarum plasmodium. J Theor Biol 197:497–506

    Google Scholar 

  49. Nagumo J, Arimoto S, Yoshizawa S (1960) An active pulse transmission line simulating 1214-nerve axons. Proc IRL 50:2061

    Google Scholar 

  50. Naser G, Karney BW (2007) A 2-D transient multicomponent simulation model: application to pipe wall corrosion. J Hydro Environ Res 1:56–69

    Google Scholar 

  51. Nicolis G, Prigogine I (1977) Self-organization in nonequilibrium systems. Wiley, New York

    MATH  Google Scholar 

  52. Pao CV (1990) Numerical methods for coupled systems of nonlinear parabolic boundary value problems. J Math Anal Appl 15:581–608

    MathSciNet  MATH  Google Scholar 

  53. Pao CV (1985) Monotone iterative methods for finite difference system of reaction diffusion equations. Numer Math 46:571–586

    MathSciNet  MATH  Google Scholar 

  54. Pao CV (1995) Finite difference reaction–diffusion solutions with nonlinear boundary conditions. Numer Methods Partial Differ Equ 11:355–374

    MATH  Google Scholar 

  55. Pao CV (1999) Numerical analysis of coupled systems of nonlinear parabolic equations. SIAM J Numer Anal 36:393–416

    MathSciNet  MATH  Google Scholar 

  56. Pao CV (2002) Finite difference reaction diffusion equations with coupled boundary conditions and time delays. J Math Anal Appl 272:407–434

    MathSciNet  MATH  Google Scholar 

  57. Pan Z, Wang Y (1991) Numerical method for the system of reaction–diffusion equations with a small parameter. Appl Math Mech 12:813–819

    MathSciNet  MATH  Google Scholar 

  58. Perthame B (2007) Transport equations in biology. Frontiers in mathematics. Birkhäuser, Basel

    MATH  Google Scholar 

  59. Perthame B, Ǵenieys S (2007) Concentration in the nonlocal Fisher equation: the Hamilton–Jacobi limit. Math Model Nat Phenom 4:135–151

    MathSciNet  MATH  Google Scholar 

  60. Polyanin AD, Zaitsev VF (2004) Handbook of nonlinear partial differential equations. Chapman & Hall/CRC, Boca Raton

    MATH  Google Scholar 

  61. Pudykiewicz JA (2006) Numerical solution of the reaction–advection–diffusion equation on the sphere. J Comput Phys 213:358–390

    MathSciNet  MATH  Google Scholar 

  62. Qiu Y, Sloan DM (1998) Numerical solution of Fisher’s equation using a moving mesh method. J Comput Phys 146:726–746

    MathSciNet  MATH  Google Scholar 

  63. Ritchie H (1985) Application of a semi-Lagrangian integration scheme to the moisture equation in a regional forecast model. Mon Weather Rev 113:424–435

    Google Scholar 

  64. Roberts LS, Janovy J, Schmidt GD (2008) Foundations of parasitology. McGraw Hill, Boston

    Google Scholar 

  65. Zhao TG, Liang YT, Ma HP (2011) Chebyshev–Legendre pseudo-spectral method for the modified Kawahara equation. Adv Mater Res 143–144:191–195

    Google Scholar 

  66. Shen J (1996) Efficient Chebyshev–Legendre Galerkin methods for elliptic problems. In: Ilin AV, Scott R (eds) Proceedings of ICOSA-HOM’95, Houston J Math,pp 233–240

  67. Shen J (1994) Efficient spectral-Galerkin method I. Direct solvers for second- and fourth-order equations by using Legendre polynomials. SIAM J Sci Comput 15:1489–1505

    MathSciNet  MATH  Google Scholar 

  68. Shen J (1995) Efficient spectral-Galerkin method II. Direct solvers for second- and fourth-order equations by using Chebyshev polynomials. SIAM J Sci Comput 16:74–87

    MathSciNet  MATH  Google Scholar 

  69. Shen J, Wang L-L (2006) Laguerre and composite Legendre–Laguerre dual-Petrov–Galerkin methods for third-order equations. Discret Contin Dyn Syst Ser B 6(6):1381–1402

    MathSciNet  MATH  Google Scholar 

  70. Shen J (2003) A new dual-Petrov–Galerkin method for third and higher odd-order differential equations: application to the KDV equation. SIAM J Numer Anal 41:1595–1619

    MathSciNet  MATH  Google Scholar 

  71. Shen J (1997) Efficient spectral-Galerkin methods III. Polar and cylindrical geometries. SIAM J Sci Comput 18:1583–1604

    MathSciNet  MATH  Google Scholar 

  72. Shen J (1999) Efficient spectral-Galerkin methods IV. Spherical geometries. SIAM J Sci Comput 20:1438–1455

    MathSciNet  MATH  Google Scholar 

  73. Shen TT, Xing KZ, Ma HP (2011) A Legendre Petrov–Galerkin method for fourth-order differential equations. Comput Math Appl 61:8–16

    MathSciNet  MATH  Google Scholar 

  74. Spee EJ, Verwer JG, de Zeeuw PM, Blom JG, Hundsdorfer W (1998) A numerical study for global atmospheric transport-chemistry problems. Math Comput Simul 48:177–204

    Google Scholar 

  75. Sun P (1996) A pseudo-non-time-splitting method in air quality modeling. J Comput Phys 127:152–157

    MATH  Google Scholar 

  76. Toro M, van Rijn L, Meijer K (1989) Three-dimensional modelling of sand and mud transport in current and waves. Technical Report No. H461, Delft Hydraulics, Delft, The Netherlands

  77. Trefethen LN (2000) Spectral methods in MATLAB. SIAM, Philadelphia

    MATH  Google Scholar 

  78. Veldhuizen SV, Vuik C, Kleijn CR (2007) Inexact Newton methods for solving stiff systems of advection–diffusion–reaction equations. In: Giraud L et al (eds) Proceedings of the international conference on preconditioning techniques for large sparse matrix problems in scientific and industrial applications, France, Toulouse

  79. Verwer JG, Sommeijer BP, Hundsdorfer W (2004) RKC time-stepping for advection–diffusion–reaction problems. J Comput Phys 201:61–79

    MathSciNet  MATH  Google Scholar 

  80. Wang Y-M, Guo B-Y (2008) A monotone compact implicit scheme for nonlinear reaction–diffusion equations. J Comput Math 26:123–148

    MathSciNet  MATH  Google Scholar 

  81. Wang Y-M, Pao CV (2006) Time-delayed finite difference reaction–diffusion systems with nonquasimonotone functions. Numer Math 103:485–513

    MathSciNet  MATH  Google Scholar 

  82. Wang Y-M, Zhanga H-B (2009) Higher-order compact finite difference method for systems of reaction–diffusion equations. J Comput Appl Math 233:502–518

    MathSciNet  Google Scholar 

  83. Wang Y-M (2001) Asymptotic behavior of the numerical solutions for a system of nonlinear integrodifferential reaction–diffusion equations. Appl Numer Math 39:205–223

    MathSciNet  MATH  Google Scholar 

  84. Williamson DL, Rash PJ (1989) Two-dimensional semi-Lagrangian transport with shape-preserving interpolation. Mon Weather Rev 117:102–129

    Google Scholar 

  85. Williamson DL, Drake JB, Hack JJ, Jakob R, Shwartzrauber PN (1992) A standard test set for numerical approximations to the shallow water equations in spherical geometry. J Comput Phys 102:211–224

    MathSciNet  MATH  Google Scholar 

  86. Yang J, Vatsala AS (2005) Numerical investigation of generalized quasilinearization method for reaction diffusion systems. Comput Math Appl 50:587–598

    MathSciNet  MATH  Google Scholar 

  87. Yuan JM, Shen J, Wu J (2008) A dual-Petrov–Galerkin method for the Kawahara-type equations. J Sci Comput 34:48–63

    MathSciNet  MATH  Google Scholar 

  88. Zhao S, Ovadia J, Liu X, Zhang Y-T, Nie Q (2011) Operator splitting implicit integration factor methods for stiff reaction–diffusion–advection systems. J Comput Phys 230:5996–6009

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author is grateful to the reviewers for carefully reading this paper and for their comments and suggestions which have improved the paper.

Author information

Authors and Affiliations

  1. Department of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), Tehran, Iran

    Farhad Fakhar-Izadi

Authors
  1. Farhad Fakhar-Izadi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Farhad Fakhar-Izadi.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fakhar-Izadi, F. An efficient spectral-Galerkin method for solving two-dimensional nonlinear system of advection–diffusion–reaction equations. Engineering with Computers 37, 975–990 (2021). https://doi.org/10.1007/s00366-019-00867-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-019-00867-1

Keywords

Search

Navigation