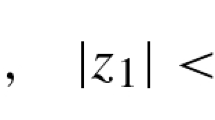Skip to main content
SpringerLink
Log in

Pluripotential Numerics

  • Published:
Constructive Approximation Aims and scope

Abstract

We study the numerical approximation of the fundamental quantities in pluripotential theory, namely the Siciak Zaharjuta extremal plurisubharmonic function\(V_E^*\) of a compact \(\mathcal {L}\)-regular set \(E\subset {\mathbb {C}}^n\), its transfinite diameter\(\delta (E),\) and the pluripotential equilibrium measure\(\mu _E:=\left( {{\mathrm{dd^c}}}V_E^*\right) ^n\). The developed methods rely on the computation of a polynomial mesh for E,  for which a suitable orthonormal polynomial basis can be defined. We prove the convergence of the approximation of \(\delta (E)\) and the local uniform convergence of our approximation to \(V_E^*\) on \({\mathbb {C}}^n\). Then the convergence of the proposed approximation of \(\mu _E\) follows. Our algorithms are based on the properties of polynomial meshes and Bernstein–Markov measures. Numerical tests on some simple cases with \(E\subset \mathbb {R}^2\) show the performance of the proposed methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

Notes

  1. The results of [5] and [6] hold indeed in the much more general setting of high powers of a line bundle on a complex manifold, see [29] as well.

  2. The original definition in [23] is actually a little weaker (sub-exponential growth instead of polynomial growth is allowed). Here we prefer to use the present one, which is now the most common in the literature.

References

  1. Baran, M.: Siciak’s extremal function of convex sets in \({\mathbb{C}}^N\). Ann. Pol. Math. 48(3), 275–280 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  2. Baran, M.: Siciak’s extremal function and complex equilibrium measures for compact sets of \(\mathbb{R}^n\). Ph.D. thesis, Jagellonian University (Krakow). Ph.D. Dissertation (1989)

  3. Bedford, E., Taylor, B.A.: The Dirichlet proiblem for a complex Monge Ampere equation. Invent. Math. 50, 129–134 (1976)

    Article  Google Scholar 

  4. Bedford, E., Taylor, B.A.: A new capacity for plurisubharmonic functions. Acta Math. 149(1), 1–40 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  5. Berman, R., Boucksom, S.: Growth of balls of holomorphic sections and energy at equilibrium. Invent. Math. 181(2), 337–394 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  6. Berman, R., Boucksom, S., Witt Nyström, D.: Fekete points and convergence towards equilibrium measures on complex manifolds. Acta Math. 207(1), 1–27 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  7. Berman, R., Ortega-Cerdá, J.: Sampling of real multivariate polynomials and pluripotential theory. Arxiv preprint, arXiv:1509.00956 (2015)

  8. Bloom, T.: Orthogonal polynomials in \(\mathbb{C}^n\). Indiana Univ. Math. J. 46(2), 427–452 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  9. Bloom, T., Bos, L., Levenberg, N.: The transfinite diameter of the real ball and simplex. Ann. Pol. Math. 106, 83–96 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bloom, T., Bos, L., Levenberg, N., Maú, S., Piazzon, F.: The extremal function for the complex ball for generalized notions of degree and multivariate polynomial approximation. arXiv:1801.02401, submitted to Annales Polonici Mathematici (2018)

  11. Bloom, T., Bos, L., Levenberg, N., Waldron, S.: On the convergence of optimal measures. Constr. Approx. 32(1), 159–179 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  12. Bloom, T., Bos, L.P., Calvi, J., Levenberg, N.: Approximation in \(\mathbb{C}^n\). Ann. Pol. Math. 106, 53–81 (2012)

    Article  MATH  Google Scholar 

  13. Bloom, T., Levenberg, N.: Weighted pluripotential theory in \({\bf C}^N\). Am. J. Math. 125(1), 57–103 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  14. Bloom, T., Levenberg, N.: Transfinite diameter notions in \(\mathbb{C}^N\) and integrals of Vandermonde determinants. Ark. Mat. 48(1), 17–40 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  15. Bloom, T., Levenberg, N.: Random polynomials and pluripotential-theoretic extremal functions. Potential Anal. 42(2), 311–334 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  16. Bloom, T., Levenberg, N., Piazzon, F., Wielonsky, F.: Bernstein-Markov: a survey. DRNA Dolomites Res Notes Approx 8, 75–91 (2015)

    MathSciNet  MATH  Google Scholar 

  17. Bloom, T., Shiffman, B.: Zeros of random polynomials on \(\mathbb{C}^m\). Math. Res. Lett. 14(3), 469–479 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  18. Bos, L., Calvi, J.-P., Levenberg, N., Sommariva, A., Vianello, M.: Geometric weakly admissible meshes, discrete least squares approximations and approximate Fekete points. Math. Comput. 80(275), 1623–1638 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  19. Bos, L., Levenberg, N.: Bernstein–Walsh theory associated to convex bodies and applications to multivariate approximation theory. Comput. Methods Funct. Theory 18(2), 361–388 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  20. Bos, L., Vianello, M.: Low cardinality admissible meshes on quadrangles, triangles and disks. Math. Inequal. Appl. 15(1), 229–235 (2012)

    MathSciNet  MATH  Google Scholar 

  21. Bos, L.P., Marchi, S.D., Sommariva, A., Vianello, M.: Weakly admissible meshes and discrete extremal sets. Numer. Math. Theory Methods Appl. 41(1), 1–12 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  22. Brezinski, C.: Accélération de la convergence en analyse numérique. Lecture Notes in Mathematics, vol. 584. Springer, Berlin (1977)

  23. Calvi, J.-P., Levenberg, N.: Uniform approximation by discrete least squares polynomials. J. Approx. Theory 152(1), 82–100 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  24. Cohen, A., Migliorati, G.: Multivariate approximation in downward closed polynomial spaces. arXiv:1612.06690 (2016)

  25. Ehlich, H., Zeller, K.: Schwankung von Polynomen zwischen Gitterpunkten. Math. Z. 86, 41–44 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  26. Embree, M., Trefethen, L.N.: Green’s functions for multiply connected domains via conformal mapping. SIAM Rev. 41(4), 745–761 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  27. Klimek, M.: Pluripotential Theory. Oxford University Press, Oxford (1991)

    MATH  Google Scholar 

  28. Kroó, A.: On optimal polynomial meshes. J. Approx. Theory 163(9), 1107–1124 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  29. Lev, N., Ortega-Cerdà, J.: Equidistribution estimates for Fekete points on complex manifolds. J. Eur. Math. Soc. (JEMS) 18(2), 425–464 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  30. Levenberg, N.: Ten lectures on weighted pluripotential theory. Dolomites Notes Approx. 5, 1–59 (2012)

    Google Scholar 

  31. Mantica, G.: Computing the equilibrium measure of a system of intervals converging to a cantor set. Dolomites Res. Notes Approx. DRNA 6, 51–61 (2013)

    Google Scholar 

  32. Ma’u, S.: Chebyshev constants and transfinite diameter on algebraic curves in \(\mathbb{C}^2\). Indiana Univ. Math. J. 60(5), 1767–1796 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  33. Narayan, A., Jakeman, J.D., Zhou, T.: A Christoffel function weighted least squares algorithm for collocation approximations. Math. Comput. 86, 1913–1947 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Olver, S.: Computation of equilibrium measures. J. Approx. Theory 163(9), 1185–1207 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  35. Piazzon, F.: Bernstein Markov properties. Ph.D. thesis, University of Padova Department of Mathemathics. Advisor: N. Levenberg (2016)

  36. Piazzon, F.: Optimal polynomial admissible meshes on some classes of compact subsets of \(\mathbb{R}^d\). J. Approx. Theory 207, 241–264 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  37. Piazzon, F.: Some results on the rational Bernstein–Markov property in the complex plane. Comput. Methods Funct. Theory 17(3), 405–443 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  38. Piazzon, F., Vianello, M.: Small perturbations of polynomial meshes. Appl. Anal. 92(5), 1063–1073 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  39. Piazzon, F., Vianello, M.: Constructing optimal polynomial meshes on planar starlike domains. Dolomites Res. Notes Approx. DRNA 7, 22–25 (2014)

    MATH  Google Scholar 

  40. Piazzon, F., Vianello, M.: Suboptimal polynomial meshes on planar Lipschitz domains. Numer. Funct. Anal. Optim. 35(11), 1467–1475 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  41. Ransford, T.: Potential Theory in the Complex Plane. London Mathematical Society Student Texts, vol. 28. Cambridge University Press, Cambridge (1995)

    Book  Google Scholar 

  42. Ransford, T., Rostand, J.: Computation of capacity. Math. Comput. 76(259), 1499–1520 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  43. Rostand, J.: Computing logarithmic capacity with linear programming. Exp. Math. 6(3), 221–238 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  44. Sadullaev, A.: An estimates for polynomials on analytic sets. Math. URSS Izv. 20(3), 493–502 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  45. Saff, E.B., Totik, V.: Logarithmic Potentials with External Fields. Springer, Berlin (1997)

    Book  MATH  Google Scholar 

  46. Shin, Y., Xiu, D.: On a near optimal sampling strategy for least squares polynomial regression. J. Comput. Phys. 326, 931–946 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  47. Siciak, J.: Extremal plurisubharmonic functions in \(\mathbb{C}^n\). Ann Pol. Math. 319, 175–211 (1981)

    Article  MATH  MathSciNet  Google Scholar 

  48. Townsend, A., Trefethen, L.N.: An extension of Chebfun to two dimensions. SIAM J. Sci. Comput. 35(6), C495–C518 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  49. Trefethen, L.N.: Multivariate polynomial approximation in the hypercube. Proc. Am. Math. Soc. 145(11), 4837–4844 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  50. Walsh, J.L.: Interpolation and Approximation by Rational Function on Complex Domains. AMS, Providence (1929)

    Google Scholar 

  51. Zaharjuta, V.P.: Extremal plurisubharmonic functions, Hilbert scales, and the isomorphism of spaces of analytic functions of several variables. I, (Russian). Teor. Funkciĭ Funkcional. Anal. i Priložen. 127(19), 133–157 (1974)

    MathSciNet  Google Scholar 

  52. Zaharjuta, V.P.: Extremal plurisubharmonic functions, Hilbert scales, and the isomorphism of spaces of analytic functions of several variables. II, (Russian). Teor. Funkciĭ Funkcional. Anal. i Priložen. 127(21), 65–83 (1974)

    MathSciNet  Google Scholar 

  53. Zeitouni, O., Zelditch, S.: Large deviations of empirical measures of zeros of random polynomials. Int. Math. Res. Not. 20, 3935–3992 (2010)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The findings of this work are essentially a part of the doctoral dissertation [35]. Consequently, much of what we present here has been deeply influenced by the discussions with our advisor, Prof. N. Levenberg (Indiana University). All the software used in the numerical tests we performed above has been developed in collaboration with the CAA group (see http://www.math.unipd.it/~marcov/CAA.html) at Padova University and Verona University, in particular with Prof. M. Vianello. The author deeply thanks him for the scientific collaboration. Also, we thank Prof. M. Putti and the University of Padova for its support.

Author information

Authors and Affiliations

  1. Department of Mathematics Tullio Levi-Civita, University of Padova, Padua, Italy

    Federico Piazzon

Authors
  1. Federico Piazzon
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Federico Piazzon.

Additional information

Communicated by Edward B. Saff.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Piazzon, F. Pluripotential Numerics. Constr Approx 49, 227–263 (2019). https://doi.org/10.1007/s00365-018-9441-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00365-018-9441-7

Keywords

Mathematics Subject Classification

Search

Navigation