Direct measurement of vorticity using tracer particles with internal markers

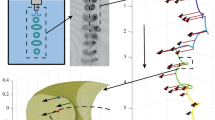
Abstract

Current experiment techniques for vorticity measurement suffer from limited spatial and temporal resolution to resolve the small-scale eddy dynamics in turbulence. In this study, we develop a new method for direct vorticity measurement in fluid flows based on digital inline holography (DIH). The DIH system utilizes a collimated laser beam to illuminate the tracers with internal markers and a digital sensor to record the generated holograms. The tracers made of the polydimethylsiloxane prepolymer mixed with internal markers are fabricated using a standard microfluidic droplet generator. A rotation measurement algorithm is developed based on the 3D location reconstruction and tracking of the internal markers and is assessed through synthetic holograms to identify the optimal parameter settings and measurement range (e.g., rotation rate from 0.3 to 0.7 rad/frame under numerical aperture of imaging of 0.25). Our proposed method based on DIH is evaluated by a calibration experiment of single tracer rotation, which yields the same optimal measurement range. Using von Kármán swirling flow setup, we further demonstrate the capability of the approach to simultaneously measure the Lagrangian rotation and translation of multiple tracers. Our method can measure vorticity in a small region on the order of 100 µm or less and can be potentially used to quantify the Kolmogorov-scale vorticity field in turbulent flows.

Graphical abstract

Appendices

Appendix

Appendix 1

The von Kármán swirling flow can be described by the equations below (Eqs. 2, 3, 4 and 5), assuming steady flow as the image data is taken long enough after turning on the motor

$$\frac{2u}{r} + \frac{dw}{{dz}} = 0,$$

(2)

$$\left( \frac{u}{r} \right)^{2} - \left( \frac{v}{r} \right)^{2} + w\frac{{d\left( {u{/}r} \right)}}{dz} = \frac{ - 1}{\rho }\frac{\partial p}{{\partial r}} + \nu \frac{{d^{2} \left( {u{/}r} \right)}}{{dz^{2} }},$$

(3)

$$\frac{2uv}{{r^{2} }} + w\frac{{d\left( {v{/}r} \right)}}{dz} = \nu \frac{{d^{2} \left( {v{/}r} \right)}}{{dz^{2} }},$$

(4)

$$w\frac{dw}{{dz}} = \frac{ - 1}{\rho }\frac{\partial p}{{\partial z}} + \nu \frac{{d^{2} w}}{{dz^{2} }},$$

(5)

with the boundary conditions for fluid with $z > 0$:

$$\begin{gathered} u = 0,v = \Omega r,w = 0,p = p_{0} \;for\;z = 0, \hfill \\ and\;u = 0,v = 0\;for\;z \to \infty . \hfill \\ \end{gathered}$$

In these equations, $\left( {u,v,w} \right)$ are the velocity components in cylindrical coordinates $\left( {r,\theta ,z} \right)$, with the origin at the center of the spinning disk and $\left( {r,\theta } \right)$ representing the plane parallel to the disk. Note that the coordinate system is defined in a way different from that of the image data. The cross section of the measurement sample volume is around 6.6 mm × 3.7 mm, much smaller compared to the size of the flow chamber, and it is only around one-fifth of the diameter of the disk. With the small sample volume, it is safe to assume that the boundaries of the flow chamber have little influence on the flow in the measurement volume and there is no rotation at infinity. Thus, the pressure becomes independent of $r$. By introducing the transformation: $\eta = \sqrt {\frac{\Omega }{\nu }} z$, $u = r\Omega F\left( \eta \right)$, $v = r\Omega G\left( \eta \right)$, $w = \sqrt {\nu \Omega } H\left( \eta \right)$, $p = p_{0} + \rho \nu \Omega P\left( \eta \right)$, where $\nu$ is the kinematic viscosity of the fluid, and $\Omega$ is the angular speed of the spinning disk, the self-similar equations (Eqs. 6, 7, 8 and 9) can be derived:

$$2F + H^{\prime} = 0,$$

(6)

$$F^{2} - G^{2} + F^{\prime}H = F^{\prime\prime},$$

(7)

$$2FG + G^{\prime}H = G^{\prime\prime},$$

(8)

$$P^{\prime} + HH^{\prime} - H^{\prime\prime} = 0,$$

(9)

and the boundary conditions for fluid with $\eta > 0$ are now:

$$\begin{gathered} F = 0,G = 1,H = 0,P = 0\;for\;\eta = 0, \hfill \\ and\;F = 0,G = 0\;for\;\eta \to \infty . \hfill \\ \end{gathered}$$

Moreover, the vorticity components in the cylindrical coordinates can also be derived as follows:

$$\omega_{r} = - r\sqrt {\Omega^{3} {/}v} G^{\prime},$$

(10)

$$\omega_{\theta } = r\sqrt {\Omega^{3} {/}v} F^{\prime},$$

(11)

$$\omega_{z} = 2\Omega G.$$

(12)

The self-similar equations (Eqs. 6, 7 and 8) are numerically solved using the bvp4c function in MATLAB to obtain the profiles of functions $F$, $G$, and $H$ as well as the derivatives $F^{\prime}$ and $G^{\prime}$. By applying $\nu = 9.5 \times 10^{ - 6} \ {\text{m}}^{2} /{\text{s}}$ and $\Omega = 198 \ {\text{rad}}/{\text{s}}$ to the equations for $\left( {u,v,w} \right)$ and $\left( {\omega_{r} ,\omega_{\theta } ,\omega_{z} } \right)$, the analytical velocity and vorticity fields can be derived. To compare with the measurement, the vorticity is converted to rotation rate by dividing two times of the imaging frame rate as the vorticity is twice the angular velocity.