Passive control of coherent structures in a modified backwards-facing step flow

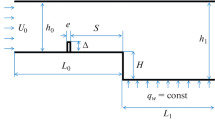
Abstract

We study a modified backwards-facing step flow, with the addition of two different plates; one is a baseline, impermeable plate and the second a perforated one. An experimental investigation is carried out for a turbulent reattaching shear layer downstream of the two plates. The proposed setup is a model configuration to study how the plate characteristics affect the separated shear layer and how turbulent kinetic energies and large-scale coherent structures are modified. Measurements show that the perforated plate changes the mean flow field, mostly by reducing the intensity of reverse flow close to the bottom wall. Disturbance amplitudes are significantly reduced up to five step heights downstream of the trailing edge of the plate, more specifically in the recirculation region. A loudspeaker is then used to introduce phase-locked, low-amplitude perturbations upstream of the plates, and phase-averaged measurements allow a quantitative study of large-scale structures in the shear-layer. The evolution of such coherent structures is evaluated in light of linear stability theory, comparing the eigenfunction of the Kelvin–Helmholtz mode to the experimental results. We observe a close match of linear-stability eigenfunctions with phase-averaged amplitudes for the two tested Strouhal numbers. The perforated plate is found to reduce the amplitude of the Kelvin–Helmholtz coherent structures in comparison to the baseline, impermeable plate, a behavior consistent with the predicted amplification trends from linear stability.

Appendix

1.1 Comparison between classical backwards-facing step (BFS) and impermeable case

In this section we present hot-wire measurements for the mean velocity and rms profiles at $X=1$ for two configurations: the impermeable plate, presented here as our baseline case, and the classical backwards-facing step. Figures 16 and 17, respectively, show the measured mean velocities and rms profiles using a single hot-wire anemometer, and we can observe that the general aspects of the flow for the impermeable plate closely resembles a classical BFS configuration. Since the measuring instrument does not differentiate forward to reverse flow, the positive velocities depicted in Fig. 16 close to the bottom wall ($Y<0$) should not be taken as an exact value; elsewhere, measurements are accurate. The two curves provide evidence that the main aspects of the flow for the impermeable case are similar to that of a classical BFS. Figure 17 presents the rms profiles for the two configurations. We observe that the fluctuations are similar in their measured amplitudes as well as in the overall shape of the curves along the vertical y-direction.

The underlying idea in the adoption of the impermeable plate as our baseline case is the advantage of isolating the effects of the perforations to small disparities that arise from the different boundary conditions between the classical BFS flow and the baseline case chosen for this work. The decision of taking the impermeable plate configuration as our baseline case is, therefore, justified considering that, although not identical, our baseline case is sufficiently similar to the well documented, classical BFS flow configuration.

Fig. 16

Absolute value of mean velocity profiles measured by hot-wire anemometry at $X=1$. Solid line (blue, open circles): impermeable plate. Dotted line (black, cross symbols): classical backwards-facing step. Even though the anemometer cannot differentiate reverse to forward flow, profiles show that both flows (impermeable case and classical BFS) are qualitatively similar

Fig. 17

Root mean square fluctuations measured by hot-wire anemometry at $X=1$. Solid line (blue, open circles): Impermeable plate. Dotted line (black, cross symbols): classical BFS configuration

1.2 Signal processing

The conditional sampling procedure is described in more details here, with a brief discussion about the quantities of interest in the eduction of coherent structures for this work.

A hot-wire signal $f(\mathbf {x},t)$ is acquired simultaneously with the forcing wave g(t). To calculate the phase-averaged signal and extract a meaningful information about the coherent fluctuations of the flow, we first evaluate the spectral content of the excitation g(t). Imperfections of the signal generation inevitably lead to slight changes of the excitation signal if compared to a purely sinusoidal wave, and there is often a slight frequency drift for longer time series. This brings a problem in the conditional sampling, since small oscillations in the phase of our reference signal can spoil the results, given that we are searching to extract a low-amplitude, periodic frequency component from a turbulent, high-amplitude signal $f(\mathbf {x},t)$.

This problem can be circumvented by applying the Hilbert transform H(g)(t) on the forcing signal (Luo et al. 2009). The Hilbert transform is defined as the convolution of g(t) with the function $1/\pi t$

$$\begin{aligned} H(g)(t) = \frac{1}{\pi t} * g(t) = \frac{1}{\pi } \ {\mathbf {p.v.}} \int \limits _{-\infty }^{+\infty }\frac{g\left( \tau \right) }{t-\tau } \mathrm {d}\tau \end{aligned}$$

(10)

where ${\mathbf {p.v.}}$ is the Cauchy principal value of the improper integral. The Hilbert transform is a linear operator, allowing the real-valued signal g(t) to be extended into the complex plane. If g(t) is a quasi-sinusoidal real-valued signal, its Hilbert transform h(t) is a similar real-valued signal, in quadrature with g(t); therefore, we can construct another function $z(t)=g(t)+\mathrm {i}h(t)$, and extract the instantaneous phase of the excitation signal as the argument of z(t). Now, the phase-averaged signal $\langle f(\mathbf {x},t) \rangle$ of the flow field for a finite signal $f(\mathbf {x},t)$ is calculated as

$$\begin{aligned} \langle f(\mathbf {x}, t) \rangle = \frac{1}{K} \sum _{k=0}^{K} \sum _{n=0}^{N} f(\mathbf {x}, \phi _n + 2 \pi k) \text {,} \end{aligned}$$

(11)

where $K = \frac{t_{record}}{\tau }$ is the number of total cycles contained in the recorded signal ($t_{record}$) and the phase is divided into N parts, e.g., $\phi _n = \frac{n 2\pi }{N} = \left[ \frac{1}{N} 2 \pi , \frac{2}{N} 2 \pi , \ldots , 2 \pi \right]$ and is equivalent to Eq. (3) as $K \rightarrow \infty$ and $N \rightarrow \infty$.

An example of the wave component $\tilde{f}(\mathbf {x},t) = \langle f(\mathbf {x}, t) \rangle - \bar{f}(\mathbf {x}, t)$ extracted from the anemometer’s time series $f(\mathbf {x},t)$ is shown in Fig. 18 in comparison to the turbulent hot-wire signal. The phase-averaged signal is Fourier transformed and the resulting Fourier coefficients $F_n = A_n + i B_n$ represent each mode, containing information about its amplitude and phase. $F_0$ is real, and represents the mean value (equal to zero for this case), while $F_{1}$ is related to the fundamental frequency, i.e., the excitation frequency we are interested in. The fundamental is thus the quantity used in this work to compare the coherent turbulence to predictions of linear stability theory.

Fig. 18

Excitation wave, hot-wire and phase-averaged signals from a measurement with external forcing at 112 Hz. Figure not drawn to scale. Solid bottom line (blue): excitation wave g(t). Solid upper line (black): hot-wire noisy signal; dashed line (red): phase averaged signal after processing $\tilde{f}(t)$