On the photon antibunching and amplitude squared squeezing of coherent light coupled to a periodically driven anharmonic oscillator

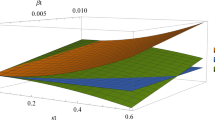
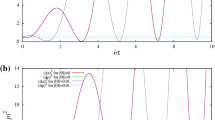
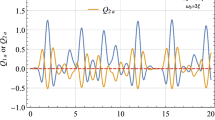
Abstract

A single mode of the quantized electromagnetic field coupled to a nonlinear medium leads to the model of an anharmonic oscillator. For third-order nonlinear medium, the corresponding anharmonic oscillator will be a quartic one. Under the rotating wave approximation (RWA), it is possible to get rid of the nonconserving energy terms and hence a two-photon anharmonic oscillator out of the quartic anharmonic oscillator. We derive the solution of a two-photon anharmonic oscillator with periodically driven term under rotating wave approximation (RWA). The driven term is assumed to be classical. The influence of the periodic force on the amplitude squared squeezing and on the antibunching of photons of the input coherent light are clearly indicated. It is established that the photon bunching and antibunching of the input coherent light coupled to the driven two-photon anharmonic oscillator are possible only if the driven term exists. In absence of nonlinear term (i.e two-photon term), the nonconservation of photon is possible and the generated photons exhibits the bunching effects. With the increase of the dimensionless interaction time, the antibunching of photons exhibit oscillatory behaviour vigorously provided the initial photon number is sufficiently high.

Appendices

Appendix-I

We now give few analytical expressions for the purpose of the calculation of the amplitude squared squeezing and the antibunching of photons of the coherent light coupled to a driven two photon anharmonic oscillator. These analytical equations are used in the body of the text. The temporal evolution of the average photon number ${\bar{N}}(t)=\langle \alpha |{\hat{n}}(t)|\alpha \rangle =\langle \alpha |a^{\dagger }(t)a(t)|\alpha \rangle$ in terms of initial coherent state follows as

$$\begin{aligned} {\bar{N}}(t) \nonumber \\= & {} |\alpha |^{2}+f(0)|\alpha |e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\left[ t\left( 1\right. \right. \nonumber \\&\left. \left. +\frac{3}{4}\lambda |\alpha |^{2}\cos \frac{3\lambda t}{4}\right) \sin \left( |\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \right. \nonumber \\&+ \frac{3}{4}\lambda |\alpha |^{2}t\sin \frac{3\lambda t}{4}\cos \left( |\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \nonumber \\&\left. +\sin t\sin \left( t+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \right] \nonumber \\&+ \frac{f^{2}(0)}{4}\left\{ \left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) ^{2}t^{2}\right. \nonumber \\&\left. +\left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) t\sin 2t+\sin ^{2}t\right\} \end{aligned}$$

(A.1)

In the calculation of ${\bar{N}}(t)=\langle \alpha |a^{\dagger }(t)a(t)|\alpha \rangle ,$ we have made use of the eigenvalue equation of the initial coherent state $a(0)|\alpha \rangle =\alpha |\alpha \rangle .$ The parameter $\alpha =|\alpha |\exp (i\theta )$ is in general complex, where $|\alpha |^{2}={\bar{n}}$ is regarded as the number of photons present in the initial coherent field. The angle $\theta$ corresponds the initial phase of the coherent light field. Now, we calculate $\bar{N^{2}}(t)=\langle \alpha |{\hat{n}}^{2}(t)|\alpha \rangle$

$$\begin{aligned} \bar{N^{2}}(t)=K_{1}+K_{2}+K_{3}+K_{4}+K_{6} \end{aligned}$$

(A.2)

where

$$\begin{aligned} K_{1}= & {} |\alpha |^{4}+|\alpha |^{2} \end{aligned}$$

(A.3)

$$\begin{aligned} K_{2}= & {} 2f(0)|\alpha |^{3}e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\left[ \left( 1+\frac{3}{4}\lambda \right) t\ \right. \nonumber \\&\times\sin \left( \sin \frac{3\lambda t}{4}+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) +\frac{3}{4}\lambda |\alpha |^{2}t\ \nonumber \\&\times\sin \left( \frac{3\lambda t}{2}+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \nonumber \\&+ \left. \sin t\sin \left( t+\frac{3\lambda t}{4}+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \right] \nonumber \\&+f(0)|\alpha |e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\left[ t\sin \left( |\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \right. \nonumber \\&+ \frac{3}{4}\lambda |\alpha |^{2}t\sin \left( \frac{3\lambda t}{4}+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \nonumber \\&\left. +\sin t\sin \left( t+|\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \right] \end{aligned}$$

(A.4)

$$\begin{aligned} K_{3}= & {} -\frac{f^{2}(0)|\alpha |^{2}}{4}e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\left[ \left( 1+\frac{3}{4}\lambda \right) t^{2}\ \right. \nonumber \\&\times\cos \left( \frac{3\lambda t}{4}+|\alpha |^{2}\sin \frac{3\lambda t}{2}-2\theta \right) \nonumber \\&+ \frac{3}{2}\lambda |\alpha |^{2}t^{2}\cos \left( \frac{9}{4}\lambda t+|\alpha |^{2}\sin \frac{3\lambda t}{2}-2\theta \right) \nonumber \\&+\left( 2+\frac{3}{4}\lambda \right) t\sin t\cos \left( t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3\lambda t}{2}-2\theta \right) \nonumber \\&+ \frac{3}{2}\lambda |\alpha |^{2}t\sin t\cos \left( t+\frac{9}{4}\lambda t+|\alpha |^{2}\sin \frac{3\lambda t}{2}-2\theta \right) \nonumber \\&\left. +\sin ^{2}t\cos \left( 2t+\frac{3}{4}\lambda t-2\theta +|\alpha |^{2}\sin \frac{3\lambda t}{2}\right) \right] \end{aligned}$$

(A.5)

$$\begin{aligned} K_{4}= & {} \frac{f^{3}(0)|\alpha |}{4}e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\nonumber \\&\times \left[ \left( 2t^{3}+\frac{3}{2}\lambda t^{3}+2t^{2}\sin 2t+\frac{3}{4}\lambda t{}^{2}\sin 2t+2t\sin ^{2}t\right) \ \right. \nonumber \\&\times \sin\left( |\alpha |^{2}\sin \frac{3\lambda t}{4}-\theta \right) \nonumber \\&+ \left( \frac{9}{2}\lambda t^{3}+3\lambda t{}^{2}\sin 2t+\frac{3}{2}\lambda t\sin ^{2}t\right) |\alpha |^{2}\ \nonumber \\&\times\sin \left( \frac{3}{4}\lambda t-\theta +|\alpha |^{2}\sin \frac{3\lambda t}{4}\right) \nonumber \\&+ \left( 2t^{2}\sin t+\frac{3}{2}\lambda t^{2}\sin t+2t\sin t\sin 2t\right. \nonumber \\&\left. +\frac{3}{4}\lambda t\sin t\sin 2t+2\sin ^{3}t\right) \ \nonumber \\&\times\sin \left( t-\theta +|\alpha |^{2}\sin \frac{3\lambda t}{4}\right) \nonumber \\&+ \left( 3\lambda t{}^{2}\sin t+\frac{3}{2}\lambda t\sin t\sin 2t\right) |\alpha |^{2}\ \nonumber \\&\left. \times\sin\left( t+\frac{3}{4}\lambda t-\theta +|\alpha |^{2}\sin \frac{3\lambda t}{4}\right) \right] \end{aligned}$$

(A.6)

$$\begin{aligned} K_{5}= & {} \frac{f^{2}(0)}{2}\left[ \left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) ^{2}t^{2}\right. \nonumber \\&\left. +\left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) t\sin 2t+\sin ^{2}t\right] \nonumber \\&+ f^{2}(0)|\alpha |^{2}\left[ \left( 1+\frac{3}{4}\lambda +\frac{3}{4}\lambda |\alpha |^{2}\right) ^{2}t^{2}\right. \nonumber \\&\left. +\left( 1+\frac{3}{4}\lambda +\frac{3}{4}\lambda |\alpha |^{2}\right) t\sin 2t+\sin ^{2}t\right] \end{aligned}$$

(A.7)

and

$$\begin{aligned} K_{6}= & {} \left( \frac{f^{2}(0)}{4}\left[ \left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) ^{2}t^{2}\right. \right. \nonumber \\&\left. \left. +\left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) t\sin 2t+\sin ^{2}t\right] \right) ^{2} \end{aligned}$$

(A.8)

Finally, the expressions for

$$\begin{aligned} K_{0}= & {} 2|\alpha |^{4}e^{-|\alpha |^{2}\left( 1-\cos 3\lambda t\right) }\nonumber \\&\times \cos \left( 4t+\frac{9}{2}\lambda t+|\alpha |^{2}\sin \lambda t-4\theta \right) \nonumber \\&-|\alpha |^{3}f(0)e^{-|\alpha |^{2}\left( 1-\cos \frac{9\lambda t}{4}\right) }\nonumber \\&\times \left[ \sin \left( 4t+\frac{9\lambda t}{4}+|\alpha |^{2}\sin \frac{9\lambda t}{4}-3\theta \right) \right. \nonumber \\&\times \left( 4+3\lambda \right) t+3\lambda |\alpha |^{2}t\nonumber \\&\times \sin \left( 4t+\frac{9\lambda t}{2}+|\alpha |^{2}\sin \frac{9\lambda t}{4}-3\theta \right) \nonumber \\&\left. +4\sin t\sin \left( 3t+\frac{9\lambda t}{4}+|\alpha |^{2}\sin \frac{9\lambda t}{4}-3\theta \right) \right] \nonumber \\&-\frac{f^{2}(0)|\alpha |^{4}}{2} e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{2}\right) }\nonumber \\&\times \left[ \left( 6+\frac{33\lambda }{4}\right) t^{2}\right. \nonumber \\&\times \cos \left( 4t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{2}\lambda t-2\theta \right) \nonumber \\&+9\lambda |\alpha |^{2}t^{2}\cos \left( 4t+\frac{9}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{2}\lambda t-2\theta \right) \nonumber \\&+ 2t\left( 6+\frac{9}{2}\lambda \right) \sin t\nonumber \\&\times \cos \left( 3t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{2}\lambda t-2\theta \right) +9\lambda |\alpha |^{2}t\sin t\nonumber \\&\times \cos \left( 3t+\frac{9}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{2}\lambda t-2\theta \right) \nonumber \\&+ \left. 6\sin ^{2}t\cos \left( 2t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{2}\lambda t-2\theta \right) \right] \nonumber \\&-\frac{f^{3}(0)|\alpha |}{4}e^{-|\alpha |^{2}\left( 1-\cos \frac{3\lambda t}{4}\right) }\nonumber \\&\times \left[ \left( 4+\frac{9\lambda }{2}\right) t^{3}\sin \left( 4t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \right. \nonumber \\&+ 9\lambda |\alpha |^{2}t^{3}\sin \left( 4t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \nonumber \\&+3t^{2}\left( 4+\frac{13}{4}\lambda \right) \sin t\cos \left( 3t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \nonumber \\&+ 18\lambda |\alpha |^{2}t^{2}\sin t\cos \left( 3t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \nonumber \\&+3t\left( 4+\frac{13}{4}\lambda \right) \sin ^{2}t\sin \left( 2t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \nonumber \\&+ 9\lambda |\alpha |^{2}t\sin ^{2}t\sin \left( 2t+\frac{3}{4}\lambda t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \nonumber \\&\left. +4\sin ^{3}t\sin \left( t+|\alpha |^{2}\sin \frac{3}{4}\lambda t-\theta \right) \right] +\frac{f^{2}(0)}{8}\nonumber \\&\times \left( 1+3\lambda |\alpha |^{2}\right) t^{4}\cos 4t+6t^{2}\left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) \nonumber \\&\times \left. \cos 2t\sin ^{2}t+4t^{3}\left( 1+\frac{9}{4}\lambda |\alpha |^{2}\right) \sin t\cos 3t\right] \nonumber \\&+ \left. 4t\left( 1+\frac{3}{4}\lambda |\alpha |^{2}\right) \sin ^{3}t\cos t+\sin ^{4}t\right] \end{aligned}$$

(A.9)

Therefore, the analytical expressions for the $\bar{N^{2}}$ is now available and could be used for calculating the ASS and bunching and antibunching of photons of the input coherent light coupled to the driven two-photon anharmonic oscillator.

Appendix-II

In order to take care the effects of surrounding, the Eq. (7) is casted in the quantum Heisenberg–Langevin equations of motion

$$\begin{aligned} \dot{{\hat{a}}}=-i{\hat{O}}{\hat{a}}-\frac{\gamma }{2}{\hat{a}}+{\hat{b}}-if(t) \end{aligned}$$

(B.1)

The parameter $\gamma$ is the dissipation/decay rate. The fluctuating Langevin force operator ${\hat{b}}$ is introduced in Eq. (B.1). Now, we follow the prescription [69] for the operator ${\hat{b}}$ and its Hermitian conjugate ${\hat{b}}^{\dagger }$

$$\begin{aligned} \langle {\hat{b}}^{\dagger }(t){\hat{b}}(t^{\prime })\rangle =0,\quad \langle {\hat{b}}(t){\hat{b}}^{\dagger }(t^{\prime })\rangle =2\gamma \,\delta (t-t^{\prime }) \end{aligned}$$

(B.2)

where $\delta$ stands for the Dirac delta function. After a small rearrangement we have,

$$\begin{aligned} \dot{{\hat{a}}}=-{\hat{O}}_{\gamma }{\hat{a}}+{\hat{b}}-if(t) \end{aligned}$$

(B.3)

where we define new parameter ${\hat{O}}_{\gamma }=i{\hat{O}}+\frac{\gamma }{2}$. Therefore, the effects of surrounding will modify the frequency as a complex one. Again, the forcing parameter is also modified by the presence of the term involving Langevin force parameter. The possibility of getting analytical solution of the Eq. (B.3) is to be explored after getting the functional form of ${\hat{b}}$ and f(t). In the present investigation we do not have any plan to do that.