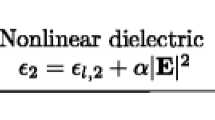Abstract
We show that negative resonant modes with an inverse relationship between the wavelength and the cavity length can be excited in a hyperbolic metamaterial slot cavity (HMMSC) in addition to positive resonant modes. The observed negative resonant modes in an HMMSC are in sharp contrast to that observed in a metal slot cavity. We analyze the dynamics of the excited negative resonant modes in the visible spectrum regime as HMMSC parameters vary.




Similar content being viewed by others
References
H.T. Miyazaki, Y. Kurokawa, Phys. Rev. Lett. 96, 097401 (2006)
V.R. Almeida, Q. Xu, C.A. Barrios, M. Lipson, Opt. Lett. 29, 1209 (2004)
Y. Kurokawa, H.T. Miyazaki, Phys. Rev. B 75, 035411 (2007)
A. Poddubny, I. Iorsh, P. Belov, Y. Kivshar, Nat. Photonics 7, 948 (2013)
P.A. Belov, Microw. Opt. Technol. Lett. 37, 259 (2003)
Z. Huang, E. Narimanov, Opt. Express 21, 15020 (2013)
E. Travkin, T. Kiel, S. Sadofev, S. Kalusniak, K. Busch, O. Benson, Opt. Lett. 45, 3665 (2020)
Y. He, S. He, X. Yang, Opt. Lett. 37, 2907 (2012)
E.D. Palik, Handbook of optical constants of solids (Academic Press, 1998)
3D/2D Maxwell's solver for nanophotonic devices. https://www.lumerical.com/products/fdtd/
A. Davoyan, S.I. Bozhevolnyi, Yu.S. Kivshar, I.V. Shadrivov, J. Nanophotonics 4, 043509 (2010)
E. Feigenbaum, N. Kaminski, M. Orenstein, Opt. Express 17, 18934 (2009)
T. Søndergaard, S.I. Bozhevolnyi, Phys. Status Solidi B 245, 9 (2008)
D.C. Marinica, A.K. Kazansky, P. Nordlander, J. Aizpurua, A.G. Borisov, Nano Letters 12, 1333 (2012)
Acknowledgements
We are grateful to Nicholas Kuhta and Alan Wang for the fruitful discussion on hyperbolic metamaterial and to Thomas Søndergaard for the discussion on transfer matrix.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
We derive the dispersion relation by calculating the transfer matrix for each of the interfaces of the layered HMMs. Transfer matrix for a dielectric–HMM interface is given by:
and for HMM–dielectric interface is given by
In Eqs. (3) and (4), \({k}_{y}\) is the y-component of wavevector inside HMM and \({\kappa} _{d}\) is the wavevector inside dielectric host, which are related by equations
where \({\varepsilon }_{dh}\) is the dielectric constant of host matrix. Transfer matrices for propagation through an HMM and dielectric of lengths t and g, respectively, become
Thus the overall transfer matrix for the HMM slot cavity shown in Fig. 1a is
The quantities M, N, and D are found from Eqs. (3), (4), and (6), respectively. Now, applying the boundary condition [13]
and solving for \({k}_{x}\) and \({k}_{y}\), the mode index is determined for particular set of parameter values, i.e., g, \({\varepsilon} _{x}\), \({\varepsilon} _{y}\), and \({\varepsilon} _{dh}\) at a desired wavelength.
Rights and permissions
About this article
Cite this article
Hasan, M., Hasan, D., Islam, M.S. et al. Negative resonant modes in a hyperbolic metamaterial slot cavity. Appl. Phys. B 127, 89 (2021). https://doi.org/10.1007/s00340-021-07631-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00340-021-07631-8