1 Introduction and Objectives

The shape that adopts a hanging chain under its own weight when suspended from its endpoints attracted the interest of scientists from times of Galileo and da Vinci. Galileo believed that the parabola was the shape of the chain but his argument was wrong. The solution curve is not so simple as a parabola (a quadratic polynomial function) but the catenary

$$\begin{aligned} y(x)=\frac{1}{a}\cosh (ax+b)-\lambda ,\quad a,b,\lambda \in {\mathbb {R}}, a>0, \end{aligned}$$
(1)

which is a curve involving transcendental functions as the exponential. The derivation of the solution was an independent work of R. Hooke, J. Bernoulli, G. Leibniz and C. Huygens among others. See Behroozi (2014); Conti et al. (2017) for an account of the history of the catenary. The catenary is a classical curve and one of the first examples, together with the brachistochrone, that illustrates the power of the calculus of variations (Giaquinta and Hildebrandt 2004). Related with the catenary, and also using calculus of variations, Euler proved that the catenary is the generating curve of the surface of revolution with minimum area and spanning to coaxial circles (Euler 1969). To be precise, if the catenary (1) rotates around the x-axis, the resulting surface of revolution is minimal if and only if \(\lambda =0\). For other mathematical properties of the catenary, see Coll and Harrison (2014); Coll and Dodd (2016); Kim et al. (2015); McIlvaine (2020); Parker (2010).

The catenary appears related with different topics in science, specially in engineering and architecture. For example, it is the model of an arch where the only force acting on the arch is its weight (Heyman 1977; Pottmann et al. 2007). This suggests its utilization in the construction of arches and roofs of corridors, such as the Spanish architect A. Gaudí used in many of its constructions, as for example, the Colegio Teresiano and La Pedrera (Barcelona). In this sense, it is very nice to read the two articles of R. Osserman about the shape of the Gateway Arch in St. Louis, Missouri, connecting the shape of the Gateway Arch and the catenary (Osserman 2010a, b).

Many extensions of the hanging chain problem have been investigated and the literature is huge. Without to give a complete list of references, we point out some of the modifications in the classical problem. For example, one can assume that: the density of the chain changes along its length (Fallis 1997; Kuczmarski and Kuczmarski 2015; O’Keefe 1996); the force vector field is radial (Denzler and Hinz 1999); the chain is made of an elastic material (Bowden 2004; Irvine 1975, 1981; Irvine and Sinclair 1976; Russell and Lardner 1997); the chain is subjected under the effect of the surface tension of a soap film adhered to the chain (Behroozi et al. 1994, 1995); the chain is suspended from a vertical line and rotates around this axis (Appell 1941; Mohazzabi and Schmidt 1999; Nordmark 2007); the two ends of the hanging chain move with stretching the chain along a path (Kajiyama 2020); and there are loads on the chain which pulling down on its lowest point (Zapolsky 1990).

In this paper we will investigate the generalization of the concept of catenary in the unit sphere \({\mathbb {S}}^2\) and in the hyperbolic plane \({\mathbb {H}}^2\). From the mathematical viewpoint, it is natural to ask for the extension of the hanging chain problem to other spaces. It is clear that the sphere and the hyperbolic plane are the first spaces to study because they are the models of the elliptic geometry and the hyperbolic geometry, respectively. However, it is surprising that this theme has not been considered in the literature, being the catenary well-known for centuries, as well as the sphere and the hyperbolic plane are classical models in geometry.

The purpose of this article is to give an approach to the generalization of the hanging chain problem in these two spaces. More specifically, to formulate a suitable problem that can be adopted as an extension of the Euclidean catenary. Once the concept of catenary is defined in these spaces as a critical point of a potential energy functional, different characterizations of the solution curve in terms of its curvature will be obtained. Finally, and if possible, we ask if the curves obtained as solutions of the hanging problem are the generating curves of minimal surfaces in the three-dimensional sphere \({\mathbb {S}}^3\) and hyperbolic space \({\mathbb {H}}^3\). This would extend the Euler’s result to these spaces.

Our motivation to generalize the notion of the catenary in the sphere and in the hyperbolic plane has its origin in the Euclidean catenary (1). We will recall the hanging chain problem in the Euclidean plane \({\mathbb {R}}^2\), pointing out which are the ingredients in its formulation. These can give us the clues to proceed when the ambient space is the sphere and the hyperbolic plane.

Let \({\mathbb {R}}^2\) denote the Euclidean plane where (x, y) stands for the Cartesian coordinates and let \(\langle ,\rangle \) be the Euclidean metric. The hanging chain problem consists in finding the shape of an inextensible chain with uniform linear mass density and suspended from two fixed endpoints. Suppose that the chain of mass m is idealized as a curve \(y:[a,b]\rightarrow {\mathbb {R}}^2\), \(y=y(x)\). The gravitational acceleration g is constant over the chain. The x-axis is taken as the level of zero potential energy. The gravitational potential energy of an infinitesimal element ds of the chain at (x, y) is \(gy\, dm=\sigma g y\, ds\), where \(\sigma \) is the density per unit length. Since \(ds=\sqrt{1+y'(x)^2}\, dx\), the total potential energy of the chain is

$$\begin{aligned} \int _a^b\sigma g y(x)\sqrt{1+y'(x)^2}\ dx. \end{aligned}$$
(2)

We are assuming in (2) that \(y(x)>0\) for all \(x\in [a,b]\). The hanging chain problem reduces to find a curve \(y=y(x)\) that minimizes this energy among all curves with the same ends and the same length. The latter hypothesis is due to the inextensibility of the chain and the absence of elastic forces. Simplifying the constant \(\sigma g\) by 1, the energy functional to minimize is

$$\begin{aligned} \mathcal {E}[y]= \int _a^b y\sqrt{1+y'^2}\, dx +\lambda \int _a^b \sqrt{1+y'^2}\, dx. \end{aligned}$$
(3)

The second term of \(\mathcal {E}[y]\) is a Lagrange multiplier because all curves have the same length. Consequently, the solution y(x) is a critical point of the energy \(\mathcal {E}\). Using standard arguments of calculus of variations, the Euler–Lagrange equation of (3) is

$$\begin{aligned} \frac{y''}{(1+y'^2)^{3/2}}=\frac{1}{(y+\lambda )\sqrt{1+y'^2}}. \end{aligned}$$
(4)

The solution of (4) is the catenary (1). Note that the left-hand side of (4) is the curvature \(\kappa _e\) of the plane curve \(y=y(x)\). The right-hand side of (4) has the following geometric interpretation. Consider the vector field \(\partial _y\) which is the (constant) gravitational field in \({\mathbb {R}}^2\). Since the unit normal vector \(\textbf{n}\) of the curve y(x) is \(\textbf{n}=(-y',1)/\sqrt{1+y'^2}\), then \(\langle \textbf{n},\partial _y\rangle =1/\sqrt{1+y'^2}\) and equation (4) can be expressed as

$$\begin{aligned} \kappa _e=\frac{\langle \textbf{n},\partial _y\rangle }{y+\lambda }. \end{aligned}$$
(5)

Equation (5) shows that the hanging chain problem is equivalent to a coordinate-free prescribed curvature problem.

Motivated by the above description of the problem, the main ingredients are the following. The first aspect concerns to the existence of a reference line which is prescribed in the problem. In the above arguments, this line is the x-axis of \({\mathbb {R}}^2\) and at this level, the potential is 0. A second aspect is the existence of a potential energy which depends on the position with respect to the reference line. In the case of the catenary, this potential is due to the gravity. It is to this potential that we want to calculate a minimum energy, or more exactly, a critical point. Finally, in the variational problem, all curves of the variation have prescribed endpoints and the same length. In particular, it is necessary to add a Lagrange multiplier to the potential energy that we want to minimize. Based on the above discussion, we will formulate the hanging chain problem in the sphere \({\mathbb {S}}^2\) and in the hyperbolic plane \({\mathbb {H}}^2\).

The objectives of this paper can be divided into three specific items:

  1. (T1)

    State the analogous hanging chain problem in \({\mathbb {S}}^2\) and in \({\mathbb {H}}^2\) and find the corresponding Euler–Lagrange equation.

  2. (T2)

    Obtain an analogous formulation of the prescribed curvature Eq. (5) in terms of a vector field that represents the ‘gravitational vector field’.

  3. (T3)

    Rotate the catenary in \({\mathbb {S}}^3\) and \({\mathbb {H}}^3\) and determine any unique properties of the mean curvature of the resulting surface.

The critical points of the potential energy will be also called catenaries. Catenaries in \({\mathbb {S}}^2\) will be discussed in Sect. 2 where two potential energies are used, first with the distance to a geodesic of \({\mathbb {S}}^2\) and second with the distance to a plane of \({\mathbb {R}}^3\). In the hyperbolic plane \({\mathbb {H}}^2\), the reference lines will be geodesics as well as horocycles. This work is carried out in Sect. 3.

After the submission of this paper, the author, together collaborators, has obtained generalizations of the notion of the catenary in other contexts and ambient spaces. See da Silva and López 2023, 2024a, b; López 2022, 2023.

2 The Hanging Chain Problem in the Sphere

In this section we will consider the hanging chain problem in the unit sphere \({\mathbb {S}}^2\). We first state the problem and then give different characterizations of its solution.

2.1 Spherical Catenaries: Definition

Consider the unit sphere \({\mathbb {S}}^2=\{(x,y,z)\in {\mathbb {R}}^3:x^2+y^2+z^2=1\}\). Let \(\Psi \) be the standard parametrization of \({\mathbb {S}}^2\) given by

$$\begin{aligned} \Psi (u,v)=(\cos {u}\cos {v},\cos {u}\sin {v},\sin {u}),\quad u\in [-\frac{\pi }{2},\frac{\pi }{2}],v\in {\mathbb {R}}. \end{aligned}$$
(6)

In the hanging chain problem in \({\mathbb {S}}^2\), consider a geodesic of \({\mathbb {S}}^2\) as the reference line to calculate the potential energy of a chain contained in \({\mathbb {S}}^2\). Let us fix the great circle \(P=\{(x,y,z)\in {\mathbb {S}}^2:z=0\}\) as the reference geodesic. Notice that on \({\mathbb {S}}^2\) we have not a concept of gravity g due to the curvature of \({\mathbb {S}}^2\).

At this point, we assign to each point of \({\mathbb {S}}^2\) a potential which measures its distance to the reference line P, being P the level of zero potential. This distance is realized along all geodesics (meridians) orthogonal to P. The distance d of a point \((x,y,z)=\Psi (u,v)\) to the geodesic P is \(d=|\arcsin (z)|=|u|\). We also consider the unit vector field \(X\in \mathfrak {X}({\mathbb {S}}^2)\) (except at the north and south poles) which is tangent to all these geodesics. This vector field is the gradient \(\nabla d\) of the distance function, which it is \(\frac{\partial \Psi }{\partial u}=\Psi _u\). The vector field X can be expressed in terms of the canonical vector fields \(\{\partial _x,\partial _y,\partial _z\}\) of \({\mathbb {R}}^3\) as

$$\begin{aligned} X(\Psi (u,v))=-\sin {u}\cos {v}\, \partial _x -\sin {u}\sin {v}\, \partial _y+\cos {u}\, \partial _z. \end{aligned}$$
(7)

As in the Euclidean case, we will consider curves of \({\mathbb {S}}^2\) that do not intersect P, hence \(u\not =0\). The geodesic P separates \({\mathbb {S}}^2\) in two domains, namely the half-spheres \({\mathbb {S}}^2_+=\{(x,y,z)\in {\mathbb {S}}^2:z>0\}\) and \({\mathbb {S}}^2_{-}=\{(x,y,z)\in {\mathbb {S}}^2:z<0\}\). Without loss of generality, we will assume that all curves will be contained in the upper half-sphere \({\mathbb {S}}^2_+\). Let \(\gamma :[a,b]\rightarrow {\mathbb {S}}^2_{+}\) be a regular curve. Let us write \(\gamma (t)=\Psi (u(t),v(t))\), \(t\in [a,b]\), with the condition \(u(t)\in (0,\pi /2]\) because \(\gamma (t)\in {\mathbb {S}}^2_{+}\). The arc-length element of \(\gamma \) is \(\sqrt{u'^2+v'^2(\cos {u})^2}\, dt\). Consequently, the potential energy of \(\gamma \) is

$$\begin{aligned} \mathcal {E}_S[\gamma ]=\int _a^b (u+\lambda )\, |\gamma '(t)|\, dt=\int _a^b (u+\lambda )\sqrt{u'^2+v'^2(\cos {u})^2}\, dt, \end{aligned}$$
(8)

where \(\lambda \in {\mathbb {R}}\). The second integral of \(\mathcal {E}_S[\gamma ]\) is a Lagrange multiplier because in the variational problem all curves have the same length.

Definition 2.1

A critical point of \(\mathcal {E}_S\) is called a spherical catenary.

Before to find the critical points of \(\mathcal {E}_S\), we will obtain a suitable expression for the curvature of a curve in \({\mathbb {S}}^2\). Here the curvature is understood to be the geodesic curvature \(\kappa _s\) of \(\gamma \) in \({\mathbb {S}}^2\). The sign of \(\kappa _s\) depends on the orientation of \({\mathbb {S}}^2\) which will be \(N(p)=-p\), \(p\in {\mathbb {S}}^2\). In such a case,

$$\begin{aligned} \begin{aligned} \kappa _s&=\frac{\langle \gamma '',\gamma '\times N(\gamma )\rangle }{|\gamma '|^3}=\frac{\langle \gamma ,\gamma '\times \gamma ''\rangle }{|\gamma '|^3}\\&=\frac{1}{|\gamma '|^3}\left( v'(2u'^2\sin {u}+v'^2(\cos {u})^2\sin {u})-\cos {u}(u'v''-u''v')\right) . \end{aligned} \end{aligned}$$
(9)

Related to the objective T1, we have the following result.

Theorem 2.2

Let \(\gamma (t)=\Psi (u(t),v(t))\) be a regular curve in \({\mathbb {S}}^2_+\). Then \(\gamma \) is a spherical catenary if and only if its curvature \(\kappa _s\) satisfies

$$\begin{aligned} \kappa _s= \frac{v'\cos {u}}{(u+\lambda )|\gamma '|}. \end{aligned}$$
(10)

Proof

We calculate the Euler–Lagrange equation of the energy (8). The Lagrangian of \(\mathcal {E}_S\) is \(J[u,v,u',v']=(u+\lambda )\sqrt{u'^2+v'^2(\cos {u})^2}\). Since the same computations will be done later in a similar context (see Proposition (2.6)), we assume a more general case of Lagrangian of type

$$\begin{aligned} J[u,v,u',v']=f(u)\sqrt{u'^2+v'^2(\cos u)^2}. \end{aligned}$$
(11)

A curve \(\gamma \) is a critical point if and only if \(\gamma \) satisfies

$$\begin{aligned} \frac{\partial J}{\partial u}-\frac{d}{dt} \left( \frac{\partial J}{\partial u'}\right) =0\quad \text{ and }\quad \frac{\partial J}{\partial v}-\frac{d}{dt} \left( \frac{\partial J}{\partial v'}\right) =0. \end{aligned}$$
(12)

After some computations, Eqs. (12) are, respectively,

$$\begin{aligned} \begin{aligned} \frac{v'\cos {u}}{|\gamma '|}\left( f'v'\cos {u}-fu'\sin {u}\right) -f\frac{d}{dt}\left( \frac{u'}{|\gamma '|}\right)&=0,\\ \frac{f'u'v'(\cos {u})^2}{|\gamma '|}+f\frac{d}{dt}\left( \frac{v'(\cos {u})^2}{|\gamma '|}\right)&=0. \end{aligned} \end{aligned}$$
(13)

Equation (13) can be written in terms of \(\kappa _s\) as follows. Using (9), we obtain

$$\begin{aligned} \begin{aligned} \frac{d}{dt}\left( \frac{u'}{|\gamma '|}\right)&=\frac{v'\cos {u}}{|\gamma '|^3}\left( v'u'^2\sin {u}-\cos {u}(u'v''-u''v')\right) \\&=v'\cos {u}\left( \kappa _s-\frac{v'\sin {u}}{|\gamma '|}\right) , \end{aligned} \end{aligned}$$
(14)

and

$$\begin{aligned} \begin{aligned}&\frac{d}{dt}\left( \frac{v'(\cos {u})^2}{|\gamma '|}\right) \\&\quad =-\frac{u'\cos {u}}{|\gamma '|^3}\left( 2u'^2v'\sin {u}+v'^3\sin {u}(\cos {u})^2-\cos {u}(u'v''-u''v')\right) \\&\quad =- u' \kappa _s \cos {u}. \end{aligned} \end{aligned}$$
(15)

From (14) and (15), the Euler–Lagrange Eq. (13) can be expressed as

$$\begin{aligned} \begin{aligned} v'\cos {u}\left( \frac{f'v'\cos {u}}{f|\gamma '|}-\kappa _s\right)&=0,\\ u'\cos {u}\left( \frac{f'v'\cos {u}}{f|\gamma '|}-\kappa _s\right)&=0. \end{aligned} \end{aligned}$$
(16)

Since \(\gamma \) is a regular curve, \(u'\) and \(v'\) cannot be simultaneously zero. Together with \(\cos {u}\not =0\) and (16), we deduce

$$\begin{aligned} \kappa _s=\frac{f'v'\cos {u}}{f|\gamma '|}. \end{aligned}$$
(17)

In the particular case that \(f(u)=u+\lambda \), then (17) is just (10). \(\square \)

Equation (10) is second order, but a first integration is possible because the Lagrangian \(J[u,v,u',v']\) does not depend on the function v. Indeed, there is a constant c such that

$$\begin{aligned} \frac{\partial J}{\partial v'}= (u+\lambda ) \frac{v'(\cos {u})^2}{\sqrt{u'^2+v'^2(\cos {u})^2}}=c. \end{aligned}$$
(18)

If \(c=0\), then \(v'=0\) and \(\gamma \) is a meridian of \({\mathbb {S}}^2\). Thus, if \(c\not =0\), then \(\gamma \) is never tangent to a meridian. In particular, \(\gamma \) does not across the north pole. Without loss of generality, we can assume that u is a function of v, \(u=u(v)\). Then \(\gamma (v)=\Psi (u(v),v)\) and (18) can be rewritten as

$$\begin{aligned} (u+\lambda ) \frac{ (\cos {u})^2}{\sqrt{u'^2+ (\cos {u})^2}}=c. \end{aligned}$$

Hence, an expression for \(u=u(v)\) is deduced, obtaining

$$\begin{aligned} \int ^u\frac{du}{\cos {u}\sqrt{(u+\lambda )^{2}(\cos {u})^2-c^2}}=\frac{v}{c}. \end{aligned}$$
(19)

This integral yields the following corollary.

Corollary 2.3

Let \(\gamma (v)=\Psi (u(v),v)\) be a regular curve in \({\mathbb {S}}^2_+\). Then \(\gamma \) is a spherical catenary if and only if \(u=u(v)\) satisfies (19) for some constant \(c\in {\mathbb {R}}\).

Remark 2.4

Identity (18) is a type of Clairaut relation for spherical catenaries. Since the Clairaut relation on \({\mathbb {S}}^2\) holds for geodesics, we need to eliminate the Lagrange constraint due to the length in the initial formulation of the variational problem. Thus, take \(\lambda =0\) in (18). Under this assumption, the angle \(\Theta \) that \(\gamma \) makes with the parallel \(v\mapsto \Psi (u,v)\) is

$$\begin{aligned} \cos \Theta =\frac{\langle \gamma ',\Psi _v\rangle }{|\gamma '||\Psi _v|}=\frac{v'\cos {u}}{\sqrt{u'^2+v'^2(\cos {u})^2}}. \end{aligned}$$

Then identity (18) can be expressed as

$$\begin{aligned} u \cos {u}\cos \Theta =c. \end{aligned}$$
(20)

The classical Clairaut relation establishes \(\cos {u}\cos \Theta =c\) (Do Carmo 1976, p. 257). In the case of spherical catenaries, identity (20) asserts that the radius of the parallel multiplied by u and by the cosine of the intersection angle with each parallel is constant.

Going back, Eq. (10) can be viewed as a prescribed equation of a curve \(\gamma \) in the sphere. Since \(\gamma \) is the image of the plane curve \(\beta (t)=(u(t),v(t))\) under the parametrization (6), Eq. (10) can be reformulated in terms of the curvature \(\kappa _\beta \) of \(\beta \). The curvature \(\kappa _\beta \) of \(\beta \) is

$$\begin{aligned} \kappa _\beta (t)=\frac{u'v''-u'' v'}{(u'^2+v'^2)^{3/2}}. \end{aligned}$$
(21)

Inserting in (9), we have

$$\begin{aligned} \kappa _s=\frac{1}{|\gamma '|^3}\left( 2u'^2v'\sin {u}+v'^3(\cos {u})^2\sin {u}-\kappa _\beta \cos {u}(u'^2+v'^2)^{3/2}\right) . \end{aligned}$$

Thus, Eq. (10) can be written as

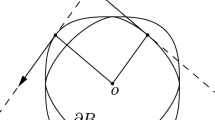
$$\begin{aligned} \kappa _\beta =\frac{2u'^2v'\sin {u}+v'^3(\cos {u})^2\sin {u}-\frac{v'\cos {u}}{u+\lambda }\left( u'^2+v'^2(\cos {u})^2\right) }{\cos {u}(u'^2+v'^2)^{3/2}}. \end{aligned}$$
(22)

This expression for \(\kappa _\beta \) allows to illustrate some spherical catenaries in Fig. 1. These plots have been made with the Mathematica software (Wolfram Research 2021). We briefly explain the method to obtain these figures. Suppose \(\gamma (t)=\Psi (u(t),v(t))\) and that the curve \(\beta (t)=(u(t),v(t))\) is parametrized by arc-length. Then \(u'(t)^2+v'(t)^2=1\). So, we can write \(u'(t)=\cos \theta (t)\) and \(v'(t)=\sin \theta (t)\) for some function \(\theta =\theta (t)\). According to (22), the functions u(t) and v(t) satisfy the ODE system,

$$\begin{aligned} \left\{ \begin{aligned} u'(t)&=\cos \theta (t)\\ v'(t)&=\sin \theta (t)\\ \theta '(t)&=\sin \theta \left( 2(\cos \theta )^2 \tan {u}+(\sin \theta )^2\cos {u}\sin {u}-\frac{1-(\sin \theta )^2(\sin {u})^2}{u+\lambda } \right) . \end{aligned} \right. \end{aligned}$$
(23)

Recall that the variation of the angle function \(\theta (t)\) coincides with the curvature \(\kappa _\beta \) of \(\beta \). Given initial conditions \(u(0)=u_0\), \(v(0)=v_0\) and \(\theta (0)=\theta _0\), Mathematica solves numerically the ODE (23) and then the software graphically represents the solution \(\gamma (t)= \Psi (u(t),v(t))\).

Fig. 1
figure 1

Spherical catenaries in \({\mathbb {S}}^2_{+}\) (top view from the north pole) for different initial conditions in the ODE system (23). The initial conditions are \(v(0)=0\), \(\theta (0)=\pi /2\) and different values for u(0): \(u(0)=0.3\) (left), \(u(0)=0.6\) (middle) and \(u(0)=1\) (right)

Full size image

2.2 Spherical Catenaries: Characterizations

In this subsection, we give a geometric interpretation of (10) completing the objective T2.

Theorem 2.5

Let \(\gamma \) be a regular curve in \({\mathbb {S}}^2_{+}\). Then \(\gamma \) is a spherical catenary if and only if its geodesic curvature \(\kappa _s\) satisfies

$$\begin{aligned} \kappa _s = \frac{\langle \textbf{n},X\rangle }{d+\lambda }, \end{aligned}$$
(24)

where \(\textbf{n}\) is the unit normal vector of the curve \(\gamma \) (as a tangent vector on \({\mathbb {S}}^2\) orthogonal to \(\gamma '\)), and d is the distance to P.

Proof

Since the unit normal vector to \({\mathbb {S}}^2\) along \(\gamma \) is \(N=-\gamma \), the vector \(\textbf{n}\) is

$$\begin{aligned} \textbf{n}=\frac{ \gamma '\times N(\gamma )}{|\gamma '|}=\frac{\gamma \times \gamma '}{|\gamma '|}. \end{aligned}$$
(25)

From the definition of X, \(\langle \textbf{n},X(\gamma )\rangle = v'\cos {u}/|\gamma '|\). Equation (24) follows from (10) because the function u in (10) is just the distance d to P. \(\square \)

Notice that Eq. (24) is the analogue of (5) for spherical catenaries of \({\mathbb {S}}^2\).

The last part of this section addresses the third objective T3. Minimal rotational surfaces of \({\mathbb {S}}^3\) have been studied in the literature. However, there is no known geometric property of the generating curves of these surfaces. More exactly, we ask if the generating curves can be viewed as solutions of a hanging chain problem in \({\mathbb {S}}^3\).

A surface of revolution in the three-dimensional sphere \({\mathbb {S}}^3\) will be constructed by rotating a spherical catenary around the geodesic P. Here, as in the Euclidean catenary, we will assume \(\lambda =0\) for the Lagrange multiplier. Let \({\mathbb {S}}^3=\{(x_1,x_2,x_3,x_4)\in {\mathbb {R}}^4:x_1^2+x_2^2+x_3^2+x_4^2=1\}\) and \({\mathbb {S}}^2\hookrightarrow {\mathbb {S}}^3\) be the natural inclusion defined by \((x_1,x_2,x_3)\mapsto (x_1,x_2,x_3,0)\). This embedding identifies \({\mathbb {S}}^2\) with \({\mathbb {S}}^2\times \{0\}\subset {\mathbb {S}}^3\). Let \(\gamma :I\rightarrow {\mathbb {S}}^2\subset {\mathbb {S}}^3\) be a curve contained in \({\mathbb {S}}_+^2\). Denote by \(S_\gamma \) the surface of revolution in \({\mathbb {S}}^3\) obtained by rotating \(\gamma \) with respect to \(P\subset {\mathbb {S}}^2\times \{0\}\). The one-parameter group of rotations whose axis is P is \(\mathcal {G}=\{\mathcal {R}_s:s\in {\mathbb {R}}\}\), where

$$\begin{aligned} \mathcal {R}_s=\left( \begin{array}{cccc}1&{}0&{}0&{}0\\ 0&{}1&{}0&{}0\\ 0&{}0&{}\cos {s}&{}-\sin {s}\\ 0&{}0&{}\sin {s}&{}\cos {s}\end{array}\right) . \end{aligned}$$

In order to simplify the computations, we can assume without loss of generality that \(\gamma \) is parametrized by \(\gamma (t)=\Psi (u(t),t)\). Then the parametrization of \(S_\gamma \) is

$$\begin{aligned} \begin{aligned} \Phi (t,s)&=\mathcal {R}_s\cdot \gamma (t)\\&=\left( \cos {(u(t))}\cos t,\cos {(u(t))}\sin {t},\sin {(u(t))}\cos {s},\sin {(u(t))}\sin {s}\right) , \end{aligned} \end{aligned}$$
(26)

where \(t\in I\subset {\mathbb {R}}\) and \(s\in {\mathbb {R}}\). We now calculate the mean curvature of \(S_\gamma \). The expression of the mean curvature of \(S_\gamma \) computed with the aid of (26) is

$$\begin{aligned} H= \frac{g_{11}h_{22}-2g_{12}h_{12}+g_{22} h_{11}}{2(g_{11}g_{22}-g_{12}^2)}, \end{aligned}$$
(27)

where, as usual, \(\{g_{11},g_{12},g_{22}\}\) and \(\{h_{11},h_{12},h_{22}\}\) are the coefficients of the first and second fundamental forms of \(S_\gamma \) for the parametrization (26):

$$\begin{aligned} g_{11}=\langle \Phi _{t},\Phi _{t}\rangle ,\quad g_{12}=\langle \Phi _{t},\Phi _{s}\rangle ,\quad g_{22}=\langle \Phi _{s},\Phi _{s}\rangle , \\ h_{11}=\langle G,\Phi _{tt}\rangle ,\quad h_{12}=\langle G,\Phi _{ts}\rangle ,\quad h_{22}=\langle G,\Phi _{ss}\rangle . \end{aligned}$$

Here G is the unit normal vector field on \(S_\gamma \). Note that \(G(\Phi (t,s))\) is not only orthogonal to \(\Phi _t(t,s)\) and \(\Phi _s(t,s)\), but also to \(\Phi (t,s)\) since G is a tangent vector of \({\mathbb {S}}^3\). A straightforward computation gives \(g_{12}=h_{12}=0\), \(g_{11}=|\gamma '|^2\), \(g_{22}=(\sin {u})^2\) and

$$\begin{aligned} h_{11}=\frac{4u'^2\sin {u}+\cos {u}(2u''+\sin (2u))}{2|\gamma '|},\quad h_{22}=-\frac{\sin {u}(\cos {u})^2}{|\gamma '|}. \end{aligned}$$

Thus, the mean curvature H in (27) is

$$\begin{aligned} H=\frac{\cos {u}(\cos {u}+\cos {3u})+(3\cos {2u}-1)u'^2-2u''\sin {u}\cos {u} }{2 \sin {u}|\gamma '|^3}. \end{aligned}$$
(28)

The expression (9) when \(v(t)=t\) is

$$\begin{aligned} \kappa _s=\frac{1}{|\gamma '|^3}\left( 2u'^2 \sin {u}+(\cos {u})^2\sin {u} +u''\cos {u}\right) . \end{aligned}$$
(29)

Equation (29) allows to write \(u''\) in terms of \(\kappa _s\). By replacing \(u''\) in (28), the mean curvature H becomes

$$\begin{aligned} H=\frac{ (\cos {u})^2 -\sin {u}|\gamma '|\kappa _s }{ \sin {u}|\gamma '|}. \end{aligned}$$
(30)

Thus, \(H=0\) if and only if \((\sin {u})|\gamma '|\kappa _s=(\cos {u})^2\). Therefore, if \(\gamma \) is a spherical catenary, the surface \(S_\gamma \) is not minimal.

Looking in the formula (30), we observe that the term \(\sin {u}\) in the numerator is just the Euclidean distance of the point \(\Psi (u,v)\) to the plane \(\Pi \) of equation \(z=0\). This suggests to consider the potential energy of \(\gamma \) calculated with respect to the plane \(\Pi \) instead of the geodesic P. Definitively, we will formulate a different hanging chain problem in \({\mathbb {S}}^2\) in such a way that the critical points of the corresponding energy functional can successfully answer to the question of the minimality of \(S_\gamma \).

Consider a plane \(\Pi \) of \({\mathbb {R}}^3\), which we can assume that it is the plane of equation \(z=0\). As usual, the z-axis is the direction of the gravity when the gravitational vector field is \(\partial _z\). Now, we replace the sphere \({\mathbb {S}}^2\) by an arbitrary surface S of the Euclidean space \({\mathbb {R}}^3\). The extrinsic hanging chain problem in S consists in determining the shape of a hanging chain supported on S where the potential energy of \(\gamma \) is calculated with the Euclidean distance to \(\Pi \). A critical point of this potential will be called an extrinsic catenary on S. Notice that the vector field \(\partial _z\) is not a vector of S but of the ambient space \({\mathbb {R}}^3\). In particular, coming back to the Euclidean context, now the chain in S is subjected to the Euclidean gravity, which is constant. In particular, we can assert that the potential at ds of the chain is \(\sigma g z\, ds\) as usual.

The extrinsic hanging chain problem was studied in the XIX century by Bobillier (1829/30), although it has not yet received much interest in the literature. See also (Appell 1941, Ch. VII) and Gudermann (1846), and more recently, Ferréol (2018). In the particular case that S is the unit sphere \({\mathbb {S}}^2\), a solution of this problem will be called an extrinsic spherical catenary (Bobillier coined the expression “spherical chaînette”). In this paper, we recall this problem and its solution and, in addition, we credit to the work of Bobillier, an almost forgotten French mathematician (Haubrichs dos Santos 2015). The potential energy of the hanging chain is

$$\begin{aligned} \mathcal {E}_S^{ex}[\gamma ]=\int _a^b(\sin {u}+\lambda ) |\gamma '(t)|\, dt= \int _a^b (\sin {u}+\lambda )\sqrt{u'^2+v'^2(\cos {u})^2}\, dt, \end{aligned}$$
(31)

where again \(\lambda \) is a Lagrange multiplier.

Proposition 2.6

Let \(\gamma \) be a regular curve in \({\mathbb {S}}^2_{+}\). Then \(\gamma \) is an extrinsic spherical catenary if and only if its geodesic curvature \(\kappa _s\) satisfies

$$\begin{aligned} \kappa _s= \frac{v'(\cos {u})^2}{(\sin {u}+\lambda )|\gamma '|}, \end{aligned}$$
(32)

or equivalently, if

$$\begin{aligned} \kappa _s= \frac{\langle \textbf{n},\partial _z\rangle }{\sin {u}+\lambda }. \end{aligned}$$
(33)

Proof

The Euler–Lagrange Eq. for (31) follows directly from (17), where now \(f(u)=\sin {u}+\lambda \). This gives (32). Formula (33) is a consequence of (32) and the expression (25) for \(\textbf{n}\). \(\square \)

Equation (33) is analogous to (5) because the term \(\sin {u}\) in the denominator is the height with respect to \(\Pi \) and the vector field \(\partial _z\) is the gravitational vector field.

Finally, we answer the question of when the mean curvature of the rotational surface \(S_\gamma \) is identically zero (objective T3).

Corollary 2.7

Let \(\gamma \) be a regular curve in \({\mathbb {S}}^2_{+}\times \{0\}\). Then \(S_\gamma \) is minimal if and only if \(\gamma \) is an extrinsic spherical catenary.

Proof

Without loss of generality, we can write \(\gamma \) as \(\gamma (t)=\Psi (u(t),t)\). From (30), the mean curvature H vanishes if and only if \((\cos {u})^2=\sin {u}|\gamma '|\kappa _s\) and this identity is just (32). \(\square \)

This result in \({\mathbb {S}}^3\) is analogous to the relation between the catenoid of \({\mathbb {R}}^3\) and the catenary curve obtained by Euler. Rotational surfaces in \({\mathbb {S}}^3\) with zero mean curvature (minimal surfaces) are known: see Arroyo et al. (2019); Ripoll (1989). Among these surfaces, the Clifford torus is the most famous example because it is the only minimal embedded torus in \({\mathbb {S}}^3\) (Brendle 2013). In the context of extrinsic spherical catenaries, the Clifford torus corresponds to the case \(\kappa _s(t)= 1\) and \(u(t)=\pi /4\) in (32). Indeed, the parametrization (26) is \(\Phi (t,s)=\frac{\sqrt{2}}{2}(\cos {t},\sin {t},\cos {s},\sin {s})\). Thus, \(S_\gamma ={\mathbb {S}}^1(\frac{1}{\sqrt{2}})\times {\mathbb {S}}^1(\frac{1}{\sqrt{2}})\) which it is the Clifford torus.

3 The Hanging Chain Problem in the Hyperbolic Plane

In this section, the hanging chain problem in the hyperbolic plane \({\mathbb {H}}^2\) is investigated. The model for \({\mathbb {H}}^2\) will be the upper half-plane \(({\mathbb {R}}^2_{+},g)\), where \({\mathbb {R}}^2_+=\{(x,y)\in {\mathbb {R}}^2:y>0\}\) and the metric is \(g=\frac{dx^2+dy^2}{y^2}\).

The hanging chain problem in the hyperbolic plane is richer than in the Euclidean plane because there are several possibilities of reference lines and potential energies. We will consider the situation that a horocycle is a reference line. Horocycles have some analogies with the straight-lines of \({\mathbb {R}}^2\) and provide the so-called horospherical geometry (Izumiya 2009). This section is divided into three parts according to this variety of choices:

  1. (1)

    Hyperbolic catenary: the reference line is a geodesic and the potential energy is calculated along geodesics of \({\mathbb {H}}^2\).

  2. (2)

    Hyperbolic horo-catenary: the reference line is a geodesic and the potential energy is calculated along horocycles of \({\mathbb {H}}^2\).

  3. (3)

    Horo-catenary: the reference line is a horocycle and the potential energy is calculated along geodesics of \({\mathbb {H}}^2\).

Let \(\gamma :[a,b]\rightarrow {\mathbb {H}}^2\) be a regular curve parametrized by \(\gamma (t)=(u(t),v(t))\). The energy to minimize in all these situations in this section is of type

$$\begin{aligned} \gamma \longmapsto \int _a^b\omega (u,v)|\gamma '(t)|\, dt= \int _a^b\omega (u,v)\frac{\sqrt{u'^2+v'^2}}{v}\, dt \end{aligned}$$
(34)

where \(\omega =\omega (u,v)\) is a smooth function on the variables u and v. Here \( \sqrt{u'^2+v'^2}/v\, dt\) is the arc-length element of \({\mathbb {H}}^2\). This energy can be interpreted as the length of \(\gamma \) in the conformal metric \(\widetilde{g}= \omega ^2g\), and consequently, its critical points coincide with the geodesics in the conformal space \(({\mathbb {R}}^2_{+},\widetilde{g})\). In order to simplify the presentation of this section, the Euler–Lagrange equations of the energy (34) are calculated in the following result.

Proposition 3.1

A regular curve \(\gamma (t)=(u(t),v(t))\) in \({\mathbb {H}}^2\) is a critical point of the energy (34) if and only if its curvature \(\kappa _h\) is

$$\begin{aligned} \kappa _h=\frac{v}{\omega \sqrt{m}}\left( u'\omega _v-v'\omega _u\right) , \end{aligned}$$
(35)

where \(m(t)=u'(t)^2+v'(t)^2\), \(\omega _u=\frac{\partial \omega }{\partial u}\) and \(\omega _v=\frac{\partial \omega }{\partial v}\).

Proof

A straightforward computation of (12) gives, respectively

$$\begin{aligned} \frac{u'}{v^2\sqrt{m}}\left( u'v\omega _v-v'\omega _u-\frac{u'\omega }{v}-\omega \frac{u'v''-v'u''}{m}\right) =0, \\ \frac{v'}{v^2\sqrt{m}}\left( u'v\omega _v-v'\omega _u-\frac{u'\omega }{v}-\omega \frac{u'v''-v'u''}{m}\right) =0. \end{aligned}$$

Since \(\gamma \) is regular, and using the Euclidean curvature \(\kappa _e\) given in (21), we deduce that \(\gamma \) is a critical point of the energy (34) if and only if

$$\begin{aligned} \kappa _e=\frac{1}{\omega \sqrt{m}}\left( u'\omega _v-v'\omega _u-\frac{u'\omega }{v}\right) . \end{aligned}$$
(36)

On the other hand, the curvature \(\kappa _h\) of \(\gamma \) is related to \(\kappa _e\) because the hyperbolic metric is conformal to the Euclidean one: see (Besse 1987, Chapter 1). This relation is

$$\begin{aligned} \kappa _h= v\kappa _e+\frac{u'}{\sqrt{m}}. \end{aligned}$$
(37)

Then (35) is consequence of (36) and (37). \(\square \)

The identity (35) can be also expressed as follows. Consider \(\{\partial _x,\partial _y\}\) the canonical vector fields of \({\mathbb {R}}^2\). Then the gradient \(\nabla \omega \) of \(\omega \) (in \({\mathbb {H}}^2\)) is

$$\begin{aligned} \nabla \omega = y^2(\omega _x\partial _x+\omega _y\partial _y). \end{aligned}$$

On the other hand, the unit normal vector of \(\gamma \) is \({\textbf{n}}(t)=v(t)\frac{(-v'(t),u'(t))}{\sqrt{m}}\). Thus, we obtain:

Corollary 3.2

A regular curve \(\gamma (t)=(u(t),v(t))\) in \({\mathbb {H}}^2\) is a critical point of the energy (34) if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h=\frac{g({\textbf{n}},\nabla \omega )}{\omega }, \end{aligned}$$
(38)

where \({\textbf{n}}\) is the unit normal vector of \(\gamma \).

3.1 Hyperbolic Catenaries

The first case to investigate follows the same motivation as in the Euclidean plane. For the choice of the reference line, we take a geodesic L of \({\mathbb {H}}^2\) which we can assume to be \(L=\{(0,y):y>0\}\). At this level, the potential will be 0. The potential energy at each point is determined by the hyperbolic distance to L which is calculated along the geodesics orthogonal to L. If \((x,y)\in {\mathbb {H}}^2\), its distance d to L is

$$\begin{aligned} d=\log \frac{x+\sqrt{x^2+y^2}}{y}. \end{aligned}$$

The geodesics orthogonal to L are half-circles of \({\mathbb {R}}^2_{+}\) centered at the origin of \({\mathbb {R}}^2\). Thus, the unit vector field \(Y\in \mathfrak {X}({\mathbb {H}}^2)\) which is orthogonal to all these geodesics at each point of \({\mathbb {H}}^2\) is

$$\begin{aligned} Y(x,y)=y\left( \frac{ y}{\sqrt{x^2+y^2}}\, \partial _x-\frac{x}{\sqrt{x^2+y^2}}\, \partial _y\right) . \end{aligned}$$
(39)

Given a curve \(\gamma (t)=(u(t),v(t))\), define the potential energy

$$\begin{aligned} \mathcal {E}_H[\gamma ]=\int _a^b (d+\lambda )|\gamma '(t)|\, dt=\int _a^b (d+\lambda )\frac{\sqrt{u'^2+v'^2}}{v}\, dt, \quad d=\log {\frac{u+r}{v}}, \end{aligned}$$
(40)

where \(r(t)=\sqrt{u(t)^2+v(t)^2}\). As in the case of the sphere \({\mathbb {S}}^2\), in \({\mathbb {H}}^2\) we have no notion of (constant) gravity. Let us observe that \(\mathcal {E}_H\) is a particular case of (34) by choosing \(\omega (u,v)=d+\lambda \). It will be assumed that \(d\not =0\), that is, \(u\not =0\). Equivalently, the curve \(\gamma \) is contained in one of the domains \({\mathbb {H}}^2_{+}=\{(x,y)\in {\mathbb {H}}^2:x>0\}\) or \({\mathbb {H}}^2_{-}=\{(x,y)\in {\mathbb {H}}^2:x<0\}\). Since each domain is mapped into other by means of the isometry \((x,y)\mapsto (-x,y)\), it will be assumed that \(\mathcal {E}_H\) acts on the class of all curves \(\gamma \) contained in \({\mathbb {H}}^2_{+}\).

Definition 3.3

A critical point of \(\mathcal {E}_H\) is called a hyperbolic catenary.

As in \({\mathbb {R}}^2\) and \({\mathbb {S}}^2\), a hyperbolic catenary will be characterized in terms of its curvature \(\kappa _h\) as curve of \({\mathbb {H}}^2\).

Theorem 3.4

A regular curve \(\gamma (t)=(u(t),v(t))\) in \({\mathbb {H}}^2_+\) is a hyperbolic catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h=-\frac{1}{d+\lambda }\frac{uu'+vv'}{ \sqrt{u^2+v^2}\sqrt{u'^2+v'^2}}=-\frac{ r'}{(d+\lambda )\sqrt{u'^2+v'^2}}. \end{aligned}$$
(41)

Proof

The energy \(\mathcal {E}_H\) is a particular case of (34). Using (35) with \(f=d+\lambda \), then Eq. (41) is obtained immediately. \(\square \)

With respect to T2, the next step consists of writing Eq. (41) in a similar manner as the formula (5) involving the curvature \(\kappa _h\) and the vector field Y. The following result is immediate by a direct computation or using (38) because the vector field Y is just \(\nabla d\).

Corollary 3.5

A regular curve \(\gamma \) in \({\mathbb {H}}^2_{+}\) is a hyperbolic catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h= \frac{g( {\textbf{n}},Y)}{d+\lambda }. \end{aligned}$$
(42)

As a consequence, Corollary 3.5 is the analogue in \({\mathbb {H}}^2\) of the statement (5) for hyperbolic catenaries.

3.2 Hyperbolic Horo-Catenaries

Consider a modified version of the above hanging chain problem replacing the potential calculated with the hyperbolic distance by the horocycle distance. The horocycle distance to the geodesic L is defined as the distance of a point \((x,y)\in {\mathbb {H}}^2\) to L calculated by the horocycle passing through (x, y) and orthogonal to L. In the present case that L is the geodesic of equation \(x=0\), this distance is |x|/y.

Let \(\gamma (t)=(u(t),v(t))\), \(t\in [a,b]\), be a regular curve contained in \({\mathbb {H}}^2_+\). The potential energy of \(\gamma \) calculated with the horocycle distance is

$$\begin{aligned} \mathcal {E}_H^{hor}[\gamma ]=\int _a^b (d_{hor}+\lambda ) |\gamma '(t)|\, dt=\int _a^b (d_{hor}+\lambda ) \frac{\sqrt{u'^2+v'^2}}{v}\, dt,\quad d_{hor}= \frac{u}{v}. \nonumber \\ \end{aligned}$$
(43)

Definition 3.6

A critical point of \(\mathcal {E}_H^{hor}\) is called an hyperbolic horo-catenary.

With respect to the objective T1, we prove:

Theorem 3.7

A regular curve \(\gamma (t)=(u(t),v(t))\) in \({\mathbb {H}}^2_+\) is a hyperbolic horo-catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h=- \frac{uu'+vv'}{(d_{hor}+\lambda )v\sqrt{m}}. \end{aligned}$$
(44)

Proof

The energy \(\mathcal {E}_H^{hor}\) in (43) is of type (34) and formula (44) is (35) for \(f= d_{hor}\). \(\square \)

To answer to T2, we replace the above vector field Y by the vector field \(W\in \mathfrak {X}({\mathbb {H}}^2)\) defined as

$$\begin{aligned} W(x,y)= y\, \partial _x- x\, \partial _y. \end{aligned}$$

The next result is a consequence of (38) because \(W=\nabla d_{hor}\).

Corollary 3.8

A regular curve \(\gamma \) in \({\mathbb {H}}^2_{+}\) is a hyperbolic horo-catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h= \frac{g( {\textbf{n}},W)}{d_{hor}+\lambda }. \end{aligned}$$
(45)

To conclude this subsection, we investigate problem T3 for this type of catenaries. The hyperbolic plane \({\mathbb {H}}^2\) is embedded into the three-dimensional hyperbolic space \({\mathbb {H}}^3=({\mathbb {R}}^3_{+},\frac{1}{x_3^2}(dx_1^2+dx_2^2+dx_3^2))\) via the natural inclusion \((x,y)\in {\mathbb {H}}^2\mapsto (x,0,y)\in {\mathbb {H}}^3\). With this identification, the geodesic \(L\subset {\mathbb {H}}^2\) is the \(x_3\)-axis in \({\mathbb {H}}^3\). Let \(S_\gamma \) denote the surface of revolution obtained by rotating \(\gamma (t)=(u(t),0,v(t))\) with respect to the \(x_3\)-axis. In the upper half-space model of \({\mathbb {H}}^3\), the rotations that leave pointwise fixed the \(x_3\)-axis coincide with the Euclidean rotations of \({\mathbb {R}}^3\) with the same axis. These surfaces of revolution in \({\mathbb {H}}^3\) are called of spherical type (Do Carmo and Dajcze 1983). Thus, a parametrization \(\Phi \) of \(S_\gamma \) is

$$\begin{aligned} \Phi (t,s)= (u(t)\cos {s},u(t)\sin {s},v(t)), \quad t\in [a,b],s\in {\mathbb {R}}. \end{aligned}$$
(46)

Theorem 3.9

A regular curve \(\gamma \) in \({\mathbb {H}}^2_+\) is a hyperbolic horo-catenary for \(\lambda =0\) if and only the rotational surface \(S_\gamma \) of spherical type is minimal.

Proof

In the upper half-space model of \({\mathbb {H}}^3\), the mean curvature H of a surface S can be computed with the aid of the Euclidean mean curvature \(H_e\) of S when S is viewed as a submanifold of the Euclidean space \({\mathbb {R}}^3_{+}\). This relation is similar to (37), namely,

$$\begin{aligned} H(p)=x_3H_e(p)+N_3(p), \end{aligned}$$
(47)

where \(p=(x_1,x_2,x_3)\in S\) and \(N=(N_1,N_2,N_3)\) is the Euclidean unit normal vector of S (Besse 1987, Chapter 1). If now S is the rotational surface \(S_\gamma \) parametrized by \(\Phi \) in (46), the value of \(H_e\) is

$$\begin{aligned} H_e=\frac{\kappa _e}{2}+\frac{v'}{2u\sqrt{m}}, \end{aligned}$$
(48)

and the expression of N is

$$\begin{aligned} N=\frac{1}{\sqrt{m}}(-v'\cos s,-v'\sin s,u'). \end{aligned}$$

Thus, \(N_3=u'/\sqrt{m}\), and using (48), the mean curvature H given in (47) becomes

$$\begin{aligned} H=\frac{v\kappa _e}{2}+\frac{vv'}{2u\sqrt{m}}+\frac{u'}{\sqrt{m}}. \end{aligned}$$

Using (37),

$$\begin{aligned} H=\frac{\kappa _h}{2}+\frac{uu'+vv'}{2\sqrt{m}}. \end{aligned}$$

Then \(H=0\) if and only if

$$\begin{aligned} \kappa _h=-\frac{uu'+vv'}{\sqrt{m}}. \end{aligned}$$
(49)

But this identity (49) is just Eq. (44) for \(\lambda =0\) because \(d_{hor}=u/v\). This proves the result. \(\square \)

We point out that do Carmo and Dajczer obtained all minimal rotational surfaces of \({\mathbb {H}}^3\). The statement of Theorem 3.9 gives a geometric interpretation of the generating curves of minimal rotational surfaces of spherical type of \({\mathbb {H}}^3\) proving that these curves are the solutions of a hanging chain problem in \({\mathbb {H}}^2\). As a consequence, this extends the Euler’s result to spherical minimal rotational surfaces.

3.3 Horo-Catenaries

We investigate the hanging chain problem considering a horocycle \(\mathcal {H}\) as reference line. Without loss of generality, we can assume \(\mathcal {H}=\{(t,1):t\in {\mathbb {R}}\}\). The potential energy at each point of \({\mathbb {H}}^2\) is given by its hyperbolic distance to \(\mathcal {H}\). In the upper half-plane model of \({\mathbb {H}}^2\), the geodesics orthogonal to \(\mathcal {H}\) are vertical lines of \({\mathbb {R}}_+^2\). If \((x,y)\in {\mathbb {H}}^2\), the hyperbolic distance \(d_b\) from (x, y) to \(\mathcal {H}\) is the length throughout the geodesic orthogonal to \(\mathcal {H}\) passing through (x, y). This distance is \(d_b=\log (y)\). Note that this distance coincides with the Busemann function in the horospherical geometry when the ideal point is \(\infty \) (Busemann 1955). The unit vector field \(V\in \mathfrak {X}({\mathbb {H}}^2)\) which is tangent to all these geodesics is given by

$$\begin{aligned} V(x,y)=y\partial _y. \end{aligned}$$

We will assume again that \(d_b\not =0\), that is, \(y\not =1\). The horocycle \(\mathcal {H}\) separates \({\mathbb {H}}^2\) in two domains, namely, \({\mathbb {H}}^2(+)=\{(x,y)\in {\mathbb {H}}^2:y>1\}\) and \({\mathbb {H}}^2(-)=\{(x,y)\in {\mathbb {H}}^2:y<1\}\), but both domains are not isometric. From now on, we will assume that all curves are contained in \({\mathbb {H}}^2(+)\) and a similar work can be done in the case that all curves are contained in \({\mathbb {H}}^2(-)\).

Let \(\gamma :[a,b]\rightarrow {\mathbb {H}}^2_+\) be a regular curve, \(\gamma (t)=(u(t),v(t))\). The potential energy of \(\gamma \) is

$$\begin{aligned} \mathcal {E}_{hor}[\gamma ]=\int _a^b ( d_b+\lambda )|\gamma '(t)|\, dt=\int _a^b ( d_b+\lambda ) \frac{\sqrt{u'^2+v'^2}}{v}\, dt.\quad d_b=\log {v}, \end{aligned}$$
(50)

where \(\lambda \in {\mathbb {R}}\) is a Lagrange parameter.

Definition 3.10

A critical point of \(\mathcal {E}_{hor}\) is called a horo-catenary.

We characterize the horo-catenaries in terms of their curvatures \(\kappa _h\).

Theorem 3.11

A regular curve \(\gamma (t)=(u(t),v(t))\) in \({\mathbb {H}}^2(+)\) is a horo-catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h= \frac{u'}{(d_b+\lambda )\sqrt{m}}. \end{aligned}$$
(51)

Proof

Expression (51) is just (35) for \(f=d_b+\lambda \). \(\square \)

The Lagrangian J of \(\mathcal {E}_{hor}\) is

$$\begin{aligned} J[u,v,u',v']=( d_b+\lambda ) \frac{\sqrt{u'^2+v'^2}}{v}, \end{aligned}$$
(52)

which does not depend on u. Thus, a first integration of the Euler–Lagrange equation can be deduced.

Corollary 3.12

A regular curve \(\gamma \) in \({\mathbb {H}}^2(+)\) is a horo-catenary if and only if \(\gamma \) can be locally expressed as

$$\begin{aligned} \gamma (v)=\left( \int ^v\frac{c\tau }{\sqrt{(\log {\tau }+\lambda )^{2}-c^2 \tau ^2}}\, d\tau ,v\right) , \end{aligned}$$
(53)

where \(c\in {\mathbb {R}}\) is a constant of integration.

Proof

From (52), there exists a constant c such that \(\frac{\partial J}{\partial u'}=c\). This identity is

$$\begin{aligned} \frac{u'(\log {v}+\lambda )}{v\sqrt{u'^2+v'^2}}=c. \end{aligned}$$
(54)

Without loss of generality, we can assume that \(\gamma \) writes locally as \(\gamma (v)=(u(v),v)\). Then (54) is

$$\begin{aligned} \frac{u'(\log {v}+\lambda )}{v\sqrt{1+u'^2}}=c. \end{aligned}$$

Hence, it follows (53).\(\square \)

With respect to the objective T2, we have the following characterization of horo-catenaries which is a consequence of (38) and the fact that \(V=\nabla d_b\).

Corollary 3.13

A regular curve \(\gamma \) in \({\mathbb {H}}^2(+)\) is a horo-catenary if and only if its curvature \(\kappa _h\) satisfies

$$\begin{aligned} \kappa _h = \frac{g( \textbf{n},V)}{d_b+\lambda }. \end{aligned}$$
(55)

Equation (55) is the analogue of the formula (5) in the context of horo-catenaries.

We finish this section with some pictures of the three types of catenaries for \(\lambda =0\). See Fig. 2. The process to plot these curves with Mathematica is the following. Suppose that \(\gamma (t)=(u(t),v(t))\) is parametrized by the Euclidean arc-length. Then \(\gamma '(t)= (\cos \theta (t),\sin \theta (t))\) for some function \(\theta =\theta (t)\), where \(\theta '(t)=\kappa _e(t)\) is the Euclidean curvature of \(\gamma \). For each type of catenary, the value of \(\kappa _e\) is obtained by combining (37) and each one of the expressions for \(\kappa _h\) in (41), (44) and (51):

$$\begin{aligned} \kappa _e&=-\dfrac{1}{v\sqrt{m}}\left( \dfrac{uu'+vv'}{rd}+u'\right) ,&\quad (\text{ hyperbolic } \text{ catenary}), \end{aligned}$$
(56)
$$\begin{aligned} \kappa _e&=-\dfrac{1}{v\sqrt{m}}\left( \dfrac{uu'+vv'}{vd_{hor}}+u'\right) ,&\quad (\text{ hyperbolic } \text{ horo-catenary}), \end{aligned}$$
(57)
$$\begin{aligned} \kappa _e&=\dfrac{1}{v\sqrt{m}}\dfrac{1-d_b}{d_b}u',&\quad (\text{ horo-catenary}). \end{aligned}$$
(58)

It follows that the functions u(t), v(t) and \(\theta (t)\) satisfy the ODE system

$$\begin{aligned} \left\{ \begin{aligned} u'(t)&=\cos \theta (t)\\ v'(t)&=\sin \theta (t)\\ \theta '(t)&=\kappa _e(t). \end{aligned} \right. \end{aligned}$$
(59)

Finally, and distinguishing the three types of catenaries of \({\mathbb {H}}^2\), the system (59) has been numerically solved with Mathematica once initial conditions

$$\begin{aligned} u(0)=u_0,\quad v(0)=v_0,\quad \theta (0)=\theta _0 \end{aligned}$$
(60)

have been prescribed.

Fig. 2
figure 2

Catenaries in \({\mathbb {H}}^2\) considering the upper half-plane model: hyperbolic catenary (left), hyperbolic horo-catenary (middle), horo-catenary (right). These curves are solutions of (59) and the initial conditions (60) are: \(u_0=1\), \(v_0=3\) and \(\theta _0=\pi /2\) (left and middle); \(u_0=0\), \(v_0=1.8\) and \(\theta _0=0\) (right)

Full size image

As a consequence of these plots, we observe that the horo-catenary (Fig. 2, right) is a graph on the x-axis. This is not a coincidence, but it holds in general.

Proposition 3.14

If \(\gamma \) is a horo-catenary, then \(\gamma \) is a vertical line or \(\gamma \) is a bounded entire graph on the x-axis.

Proof

Suppose that \(\gamma \) is parametrized by \(\gamma (t)=(u(t),v(t))\), \(t\in I\subset {\mathbb {R}}\), where t is the Euclidean arc-parameter and I is the maximal domain. Since \(\gamma \) is a horo-catenary, the curvature \(\kappa _e\) of \(\gamma \) satisfies (58). Thus, the ODE system (59) is

$$\begin{aligned} \left\{ \begin{aligned} u'(t)&=\cos \theta (t)\\ v'(t)&=\sin \theta (t)\\ \theta '(t)&=\frac{1-\log {v}}{v\log {v}}u'. \end{aligned} \right. \end{aligned}$$
(61)

We distinguish two cases.

  1. (1)

    Suppose there exists \(t_0\) such that \(u'(t_0)=0\). The first Eq. of (61) implies \(\cos \theta (t_0)=0\). Without loss of generality, we suppose that \(\theta (t_0)=\pi /2\). Thus, at \(t=t_0\), the initial conditions (60) are \((u(t_0),v(t_0),\pi /2)\). By uniqueness of (61)-(60), \(\gamma \) is a vertical straight-line.

  2. (2)

    Suppose \(u'(t)\not =0\) for all t. This implies that \(\gamma \) is a graph on some interval \(I=(a,b)\) of the x-axis. Reparametrizing \(\gamma \), the curve \(\gamma \) can be expressed by \(\gamma (x)=(x,v(x))\), \(x\in I\) and it will be proved that \(I={\mathbb {R}}\). Equation (54) for \(\kappa _e\) becomes

    $$\begin{aligned} \frac{\log {v}}{v\sqrt{1+v'^2}}=c. \end{aligned}$$
    (62)

    Since \(c\not =0\) and \(\gamma \) is contained in \({\mathbb {H}}^2(+)\), we have \(\log (v)>0\). From (62) we deduce

    $$\begin{aligned} 0<c=\frac{\log {v}}{v\sqrt{1+v'^2}}\le \frac{\log {v}}{v}. \end{aligned}$$
    (63)

    The function \(t\mapsto \log {t}/t \) is bounded in \((1,\infty )\) with the property

    $$\begin{aligned} \lim _{t\rightarrow \infty } \frac{\log {t}}{t}=\lim _{t\rightarrow 1} \frac{\log {t}}{t}=0. \end{aligned}$$
    (64)

    From (63) and (64), we conclude that \(v'\) is a bounded function. Moreover, there exist two constants \(m_1, m_2\in {\mathbb {R}}\) such that \(1<m_1<m_2\) and \(m_1\le v(x)\le m_2\) for all \(x\in (a,b)\). The fact that the function \(v'(t)\) is bounded proves finally that all solutions of (61) are defined in the entire real line \({\mathbb {R}}\).

\(\square \)

4 Conclusions and Outlook

The catenary is the solution of the hanging chain problem in \({\mathbb {R}}^2\) and this makes it so attractive in other fields of science, engineering and architecture. However, the hanging chain problem has not been formulated in spaces other than Euclidean one. Among these spaces, the sphere \({\mathbb {S}}^2\) and the hyperbolic plane \({\mathbb {H}}^2\) are the natural choices to extend this problem. It has been formulated this problem in \({\mathbb {S}}^2\) and in \({\mathbb {H}}^2\), defining in each case a potential energy that depends on the distance of a point with respect to a reference line. The resulting critical points of these energies (for different reference lines) have generalized the concept of catenary in both spaces.

A remarkable result is the characterization of the generating curves of minimal rotational surfaces of \({\mathbb {S}}^3\) proving that these curves are chains on \({\mathbb {S}}^2\) suspended by its weight where the force vector field is really the gravity of \({\mathbb {R}}^3\). In this particular situation, the initial hanging chain problem formulated in \({\mathbb {S}}^2\) must be replaced by other, which was called ‘extrinsic’, because the force field is a vector of \({\mathbb {R}}^3\), not of \({\mathbb {S}}^2\). Then it was proved that the generating curves are solutions of an old problem formulated by Bobillier in the nineteenth century and that it has been revisited in the present paper.

There are a number of problems in which this article could be expanded. For example, a question concerns to investigate the existence of closed spherical catenaries. In view of the pictures of Fig. 1, it seems plausible that such catenaries do exist. This problem was investigated in Arroyo et al. (2019) for extrinsic spherical catenaries in the context of rotational surfaces of \({\mathbb {S}}^3\) with constant mean curvature. Besides the closed catenaries, there are other catenaries which never close up and they are turning around the north pole of \({\mathbb {S}}^2\). The problem that arises here is that, although the curvature function is periodic, this is not enough to ensure that the corresponding catenary will be closed: see a discussion of this problem in Arroyo et al. (2008).

As in \({\mathbb {S}}^2\), it would be interesting to classify the catenaries in the hyperbolic plane. Proposition 3.14 is just an example, but the work to be done goes beyond that. According to Fig. 2, several questions are reasonable to ask. For example, (i) when does a catenary intersect the ideal boundary of \({\mathbb {H}}^2\)? and in such a case, determine whether the intersection is orthogonal; (ii) is every horo-catenary periodic? (iii) Which are the properties of the horo-catenaries contained in \({\mathbb {H}}^2(-)\)?

Another extension of the paper would be to consider the shape of a hanging surface in \({\mathbb {S}}^3\) and \({\mathbb {H}}^3\). In the Euclidean space, the analogue of the catenary in the two-dimensional case is called a singular minimal surface (Böhme et al. 1980; Dierkes and Huisken 1990). The extension is straightforward using the characterization (5). So, it suffices to replace the curvature of the catenary \(\kappa _e\) by the mean curvature H of the surface and the unit normal \(\textbf{n}\) of the curve by the unit normal vector field G to the surface. For example, in the three-dimensional unit sphere \({\mathbb {S}}^3\), the shape of a hanging surface with respect to \({\mathbb {S}}^2\times \{0\}\) is characterized by the equation \(H= \langle G,X\rangle /(d+\lambda )\), \(\lambda \in {\mathbb {R}}\). Here d is the distance to \({\mathbb {S}}^2\times \{0\}\) and \(X\in \mathfrak {X}({\mathbb {S}}^3\)) is the unit vector field tangent to the meridians of \({\mathbb {S}}^3\) which are orthogonal to \({\mathbb {S}}^2\times \{0\}\).

Finally, it could be interesting to obtain some geometric properties of the rotational surfaces in \({\mathbb {S}}^3\) and in \({\mathbb {H}}^3\) constructed by catenaries in its different possibilities. Although initially the hanging chain problem has no relation to the problem for rotational surfaces with minimum area, in some cases we have proved a connection between both problems (Corollary 2.7 and Theorem 3.9). It seems interesting to investigate geometric properties of the rotational surfaces of \({\mathbb {S}}^3\) and \({\mathbb {H}}^3\) whose generating curves are catenaries of \({\mathbb {S}}^2\) and \({\mathbb {H}}^2\), respectively.