Abstract
As vectors, mosquitoes transmit numerous mosquito-borne diseases. Among the many factors affecting the distribution and density of mosquitoes, climate change and warming have been increasingly recognized as major ones. In this paper, we make use of three diffusive logistic models with free boundary in one space dimension to explore the impact of climate warming on the movement of mosquito range. First, a general model incorporating temperature change with location and time is introduced. In order to gain insights of the model, a simplified version of the model with the change of temperature depending only on location is analyzed theoretically, for which the dynamical behavior is completely determined and presented. The general model can be modified into a more realistic one of seasonal succession type, to take into account of the seasonal changes of mosquito movements during each year, where the general model applies only for the time period of the warm seasons of the year, and during the cold season, the mosquito range is fixed and the population is assumed to be in a hibernating status. For both the general model and the seasonal succession model, our numerical simulations indicate that the long-time dynamical behavior is qualitatively similar to the simplified model, and the effect of climate warming on the movement of mosquitoes can be easily captured. Moreover, our analysis reveals that hibernating enhances the chances of survival and successful spreading of the mosquitoes, but it slows down the spreading speed.

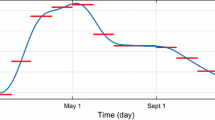
Source: Lamps Ontario climate change projections data portal (Zhu and Deng 2016)






Similar content being viewed by others
Notes
This interpretation arises from discussions with Professor Chris Cosner.
References
Abdelrazec A, Lenhart S, Zhu H (2014) Transmission dynamics of West Nile virus in mosquitoes and corvids and non-corvids. J Math Bio 68(6):553–1582
Amarasekare P, Coutinho RM (2013) The intrinsic growth rate as a predictor of population viability under climate warming. J Anim Ecol 82(6):1240–1253
Angenent S (1988) The zero set of a solution of a parabolic equation. J Reine Angew Math 390:79–96
Aregawi M, Cibulskis RE, Otten M, Williams R (2009) World malaria report 2009. World Health Organization, Geneva
Battisti A, Stastny M, Netherer S, Robinet C, Schopf A, Roques A, Larsson S (2005) Expansion of geographic range in the pine processionary moth caused by increased winter temperatures. Ecol Appl 15(6):2084–2096
Benedict MQ, Levine RS, Hawley WA, Lounibos LP (2007) Spread of the tiger: global risk of invasion by the mosquito Aedes albopictus. Vector Borne Zoonot 7(1):76–85
Cai J, Lou B, Zhou M (2014) Asymptotic behavior of solutions of a reaction diffusion equation with free boundary conditions. J Dyn Differ Equ 26(4):1007–1028
Cailly P, Tran A, Balenghien T, L’Ambert G, Toty C, Ezanno P (2012) A climate-driven abundance model to assess mosquito control strategies. Ecol Model 227:7–17
Campbell GL, Marfin AA, Lanciotti RS, Gubler DJ (2002) West Nile virus. Lancet Infect Dis 2(9):519–529
Cantrell S, Cosner C (2003) Spatial ecology via reaction-diffusion equations. John Wiley & Sons Ltd, Chichester
CDC (2016) Estimated range of Aedes albopictus and Aedes aegypti in the United States. http://www.cdc.gov/zika/vector/range.html
Chabot-Couture G, Nigmatulina K, Eckhoff P (2014) An environmental data set for vector-borne disease modeling and epidemiology. PLOS ONE 9(4):e94741
Clements AN (2011) The biology of mosquitoes: viral, arboviral and bacterial pathogens, vol 3. Cambridge University Press, Cambridge
Du Y (2006) Order structure and topological methods in nonlinear partial differential equations, vol 1. Maximum principles and applications. World Scientific Publishing Co., Pte. Ltd., Hackensack
Du Y, Guo Z (2006) The degenerate logistic model and a singularly mixed boundary blow-up problem. Discret Contin Dyn Syst Ser A 14:1–29
Du Y, Lin Z (2010) Spreading-vanishing dichotomy in the diffusive logistic model with a free boundary. SIAM J Math Anal 42(1):377–405
Du Y, Lin Z (2014) The diffusive competition model with a free boundary: invasion of a superior or inferior competitor. Discret Cont Dyn Sys B 19(10):3105–3132
Du Y, Lou B, Zhou M (2015) Nonlinear diffusion problems with free boundaries: convergence, transition speed and zero number arguments. SIAM J Math Anal 47:3555–3584
Elbers A, Koenraadt C, Meiswinkel R (2015) Mosquitoes and culicoides biting midges: vector range and the influence of climate change. Rev Sci Tech Off Int Epiz 34(1):123–137
Ezanno P, Aubry-Kientz M, Arnoux S, Cailly P, LAmbert G, Toty C, Balenghien T, Tran A (2015) A generic weather-driven model to predict mosquito population dynamics applied to species of Anopheles, Culex and Aedes genera of southern France. Prev Vet Med. doi:10.1016/j.prevetmed.2014.12.018
Ge J, Kim KI, Lin Z, Zhu H (2015) A SIS reaction-diffusion-advection model in a low-risk and high-risk domain. J Differ Equ 259(10):5486–5509
Gilles HM, Warrell DA et al (1996) Bruce–Chwatt’s essential malariology, 3rd edn. Edward Arnold (Publisher) Ltd, London
Githeko AK, Lindsay SW, Confalonieri UE, Patz JA (1969) Climate change and vector-borne diseases: a regional analysis. B World Health Organ 78(9):1136–1147
Gratz N (2004) Critical review of the vector status of Aedes albopictus. Med Vet Entomol 18(3):215–227
Gubler DJ (2002) The global emergence/resurgence of arboviral diseases as public health problems. Arch Med Res 33(4):330–342
Harley CD (2011) Climate change, keystone predation, and biodiversity loss. Science 334(6059):1124–1127
Hickling R, Roy DB, Hill JK, Fox R, Thomas CD (2006) The distributions of a wide range of taxonomic groups are expanding polewards. Glob Change Biol 12(3):450–455
Juliano SA (2007) Population dynamics. J Am Mosq Control Assoc 23(2 Suppl):265–275
Ladyženskaja OA, Solonnikov VA, Ural’ceva NN (1968) Linear and quasilinear equations of parabolic type. American Mathematical Society, Providence
Lambrechts L, Scott TW, Gubler DJ (2010) Consequences of the expanding global distribution of Aedes albopictus for dengue virus transmission. PLOS Negl Trop D 4(5):e646
Lassiter MT, Apperson CS, Roe RM (1995) Juvenile hormone metabolism during the fourth stadium and pupal stage of the southern house mosquito. Culex quinquefasciatus say. J Insect Physiol 41(10):869–876
Lin Z, Zhu H (2017) Spatial spreading model and dynamics of West Nile virus in birds and mosquitoes with free boundary. J Math Biol. doi:10.1007/s00285-017-1124-7
Madad SS, Masci J, Sr CN, Allen M (2016) Preparedness for zika virus disease—New York city, 2016. MMWR Morb Mortal Wkly Rep 65(42):1161–1165
Mitchell C (1995) The role of Aedes albopictus as an arbovirus vector. Parassitologia 37(2–3):109–113
Parmesan C (2006) Ecological and evolutionary responses to recent climate change. Annu Rev Ecol Evol Syst 37:637–669
Parmesan C, Yohe G (2003) A globally coherent fingerprint of climate change impacts across natural systems. Nature 421(6918):37–42
Peng R, Zhao XQ (2013) The diffusive logistic model with a free boundary and seasonal succession. Discret Contin Dyn Syst Ser A 33(5):2007–2031
Pialoux G, Gaüzère BA, Jauréguiberry S, Strobel M (2007) Chikungunya, an epidemic arbovirosis. Lancet Infect Dis 7(5):319–327
Saker L, Lee K, Cannito B, Gilmore A, Campbell-Lemdrum D (2004) Globalization and infectious: a review of the linages. World Health Organization, Geneva
Tran A, L’Ambert G, Lacour G, Benoît R, Demarchi M, Cros M, Cailly P, Aubry-Kientz M, Balenghien T, Ezanno P (2013) A rainfall-and temperature-driven abundance model for Aedes albopictus populations. Int J Env Res Pub He 10(5):1698–1719
Walther GR, Post E, Convey P, Menzel A, Parmesan C, Beebee TJ, Fromentin JM, Hoegh-Guldberg O, Bairlein F (2002) Ecological responses to recent climate change. Nature 416(6879):389–395
Wan H, Zhu H (2014) A new model with delay for mosquito population dynamics. Math Biosci Eng 11(6):1395–1410
Wang J, Ogden NH, Zhu H (2011) The impact of weather conditions on Culex pipiens and Culex restuans (Diptera: Culicidae) abundance: a case study in peel region. J Med Entomol 48(48):468–475
WHO (2016) World Health Organization: vector-borne diseases. http://www.who.int/mediacentre/factsheets/fs387/en/
Zhu H, Deng Z (2016) Lamps Ontario climate change portal. http://www.yorku.ca/OCCP
Acknowledgements
We thank the referees for their careful reading of the manuscript and useful suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
This project was partially supported by NSERC and CIHR of Canada. Wendi Bao was supported by a postdoctoral fellowship from Chinese Scholarship Council and the Shandong Provincial Natural Science Foundation, China (Grant No. ZR2014AQ004). Part of this work was done while Du and Lin were visiting Lamps at York University. The research of Du was partially supported by the Australian Research Council. Lin was partially supported by the NSFC of China (Grant No. 11371311).
Appendix
Appendix
1.1 Existence and uniqueness
First we present the following local existence and uniqueness result to problem (2.7) by the contraction mapping theorem.
Theorem 5.1
For any given \(\gamma \) satisfying \(0<\gamma <1\), a(x) satisfying (2.6) and \(u_0\) satisfying (2.5), there is a \(T>0\) such that problem (2.7) admits a unique solution
moreover,
where \(D_{T}=\{(t,x)\in \mathbb {R}^2: t\in [0,T]\, x\in [0, h(t)]\}\), C and T only depend on \(\gamma , h_0\), \(\Vert a\Vert _{C^{\alpha }([0, 2h_0])}\) and \(\Vert u_0\Vert _{C^{2}([0, h_0])}\).
Proof
The proof is similar as that of Theorem 2.1 in Du and Lin (2010). We give a sketch here. First we straighten the free boundary by the following transformation
Then the free boundary problem (2.7) becomes
For fixed \(t>0\), as long as \(h(t)>0\), the above transformation \(y=h_0x/h(t)\) is a diffeomorphism from \([0, +\infty )\) onto \([0, +\infty )\). It changes the free boundary \(x=h(t)\) to the line \(y=h_0\) but at the expense of making the equation more complicated, since now the coefficients in the first equation of (5.2) include the unknown function h(t).
Denote \(h^*=-\mu u_0'(h_0)+\alpha a_{-}(h_0)\) and set
If \(T\le T_1:=\frac{h_0}{2(|h^*|+1)}\) and \(h\in H_T\), then \(\frac{1}{2}h_0\le h(t)\le \frac{3}{2}h_0\). It is not difficult to see that \(\Gamma _T:=W_T\times H_T\) is a complete metric space with the metric
and that for \(h_1, h_2\in H_T\), by virtue of \(h_1(0)=h_2(0)=h_0\),
Now, we can apply the standard \(L^p\) theory and the Sobolev imbedding theorem (Ladyženskaja et al. 1968) to obtain that for any \((w, h)\in \Gamma _T\), the following initial boundary value problem
admits a unique solution \(\tilde{w}\in C^{(1+\gamma )/2,1+\gamma }([0,T]\times [0, h_0])\) and
where \(p=\frac{3}{1-\gamma }\), and \(C_1\) is a constant depending on \(\gamma , h_0\) and \(\Vert u_0\Vert _{C^{2}[0, h_0]}\).
From the third equation in (5.2), we can define a function \(\tilde{h}(t)\) as follows
which implies \(\tilde{h}'(t)=-\mu \frac{h_0}{h(t)}\frac{\partial {\tilde{w}}}{\partial y}(t,h_0)+\alpha a_{-}(h(t))\), \({\tilde{h}}(0)=h_0\) and \(\tilde{h}'(0)=h^*\). Hence, \(\tilde{h}'(t)\in C^{\gamma /2}([0,T])\) with
where we have used the fact that
for \(t_1>t_2\).
Next, we define a map \(\mathcal {F}\): \(\Gamma _{T}\rightarrow C([0,T]\times [0,h_0])\times C^1[0,T]\) by
It is easy to see that \((w(t,y), h(t))\in \Gamma _T\) is a fixed point of \(\mathcal {F}\) if and only if it solves (5.2).
It follows from (5.5), (5.7) and (5.8) that
Therefore, if we take \(T\le \min \{T_1,\ (C_3)^{-2/\gamma },\ C_1^{-2/(1+\gamma )}\}\), then \(\mathcal {F}\) maps \(\Gamma _T\) into itself.
The rest of the proof is similar as that in Du and Lin (2010). By using the \(L^p\) estimates for parabolic equations and Sobolev’s imbedding theorem, we can prove that \(\mathcal {F}\) is a contraction mapping over \(\Gamma _T\) for \(T>0\) sufficiently small. Then the contraction mapping theorem gives that \(\mathcal {F}\) has a unique fixed point (w, h) in \(\Gamma _T\). By the Schauder estimates again, we have additional regularity and then (w(y, t), h(t)) is a unique local classical solution of problem (5.2), and therefore (u(t, x), h(t)) is a unique local classical solution of problem (2.7). \(\square \)
Theorem 5.2
The solution of problem (2.7) exists and is unique for all \(t\in (0,\infty )\). Moreover, there exist constants \(C_4\) and \(C_5\) such that
where \(h_a\) is the smallest zero of a(x).
Proof
Let (u, h) be a solution to (2.7) defined for \(t\in (0,T_0]\) for some \(T_0\in (0, +\infty )\). The positivity of u is directly from the strong maximum principle. Also the maximum principle shows that
It is easy to check that \(u_x(t,h(t))< 0\), and we can also prove that \(u_x(t,h(t))\ge -C_6\) for \(t\in (0,T_0]\) and some \(C_6\) which is independent of \(T_0\) by using the similar method as that of Lemma 2.2 in Du and Lin (2010). Therefore,
We claim that \(h(t)\ge \min \{h_0, h_a\}\). Otherwise, there exists a \(t_0>0\) such that \(h(t_0)<\min \{h_0, h_a\}\) and \(h'(t_0)\le 0\). But the free boundary condition implies that \(h'(t)> \alpha a_-(h(t))\) for all \(t>0\). We then have \(h'(t_0)> \alpha a_-(h(t_0))=0\), which leads to a contradiction.
Since \(h(t)\ge \min \{h_0, h_a\}>0\), u and \(h'(t)\) are bounded in \((0, T_0]\times [0,h(t))\) by constants independent of \(T_0\), the global existence of the solution is guaranteed. Moreover, the above estimates hold for \(t\in (0, \infty )\). \(\square \)
1.2 Comparison principle
Theorem 5.3
(The Comparison Principle) Assume that \(\overline{h}\in C^1([0, +\infty ))\), \(g\in C([0, \infty ))\), \(0\le g(t)<\overline{h}(t)\) for \(t\ge 0\), \(D=\{(t,x): t>0, g(t)<x<h(t)\}\), \(\overline{u}(t,x) \in C^{1,0}(\overline{D} )\cap C^{1,2}(D)\), and
If the solution (u, h) to the free boundary problem (2.7) satisfies \(u(t, g(t))\le \overline{u}(t, g(t))\) for \(t\ge 0\), then
Proof
Similarly as in the proof of Lemma 3.5 in Du and Lin (2010) or Lemma 2.6 in Du and Lin (2014), we only prove it for the case that \(h(0)<\overline{h}(0)\), the result for the general case \(h(0)\le \overline{h}(0)\) can be established by approximation.
First under the assumption that \(h(0)<\overline{h}(0)\), we claim that \(h(t)<\overline{h}(t)\) for all \(t\in (0, \infty )\). If our claim does not hold, then we can find a first \(T^*>0\) such that \(h(t)<\overline{h}(t)\) for \(t\in (0, T^*)\), and \(h(T^*)=\overline{h}(T^*)\). It follows that
We now compare u and \(\overline{u}\) over the bounded region
Since \(U(t,x):=\overline{u}(t,x)-u(t,x)\) satisfies
using the strong maximum principle and the Hopf boundary lemma yields \(U(x, t)>0\) in \(\Omega _{T^*}\), and \(U_x(T^*, h(T^*))<0\). We then deduce that
But this contradicts (5.9). This proves our claim that \(h(t)<\overline{h}(t)\) for all \(t\in (0, \infty )\). Using again the maximum principle over \(\{(t,x): 0<t<T,\, 0\le x\le h(t)\}\) for any \(T>0\) we obtain that \(u\le \overline{u}\) for \(x\in [0, h(t)]\) and \(t\in (0, +\infty )\). \(\square \)
The pair \((\overline{u}(t,x), \overline{h}(t))\) in Theorem 5.3 is usually called an upper solution of (2.7). Similarly, we can define a lower solution by reversing all of the inequalities in the obvious places. Moreover, it is easy to prove that an analogue of Theorem 5.3 for lower solutions holds.
1.3 Proof of Lemma 3.3
We first observe that, if \(a(L)\ge 0\) and hence \(a_-(L)=0\), then the conclusion of Lemma 3.3 is evident. Indeed, if there exists \(t_0>0\) such that \(h(t_0)=L\), then
It follows immediately that \(h(t)-L\) can change sign at most once for \(t>0\).
Therefore we assume from now on that \(a(L)<0\) and so
For our proof below, we will make use of the following auxiliary problem:
Standard ODE theory for initial value problems guarantees that (5.10) has a unique solution V(x) and one of the following happens:
-
(i)
\(V(x)>0\) for all \(x<L\).
-
(ii)
There exists \(l\in [0,L)\) such that \( V(l)=0,\; V(x)>0\; \forall x\in (l, L). \)
-
(iii)
There exists \(l\in (-\infty , 0)\) such that \( V(l)=0,\; V(x)>0\; \forall x\in (l, L).\)
In the following, we will prove Lemma 3.3 for cases (i), (ii) and (iii) separately. In every case, our proof will be based on the zero number argument. To this end, we first extend a(x) to \(x<0\) as an even function and still donote it by a(x). We similarly extend u(t, x) to \(x\in [-h(t), 0)\) as an even function of x, and still denote it by u(t, x). We will consider the number of zeros of the function
over a certain interval of x where both \(u(t,\cdot )\) and \(V(\cdot )\) are defined, and examine how this zero number varies as t increases. Note that, for \(t>0\) and \(x\in (-h(t), h(t))\cap (l, L)\), w satisfies an equation of the form
for some \(L^\infty \) function c(t, x), where we take \(l=-\infty \) when case (i) happens.
We first recall a result which follows directly from Theorem D of Angenent (1988) [see Lemma 2.8 in Cai et al. (2014)].
Lemma 5.4
Let \(\xi _1(t)<\xi _2(t)\) be two continuous functions for \(t\in (t_1, t_2)\). If u(t, x) satisfies
for some \(L^\infty \) function C(t, x), then for each \(t\in (t_1, t_2)\), the number of zeros of \(u(t,\cdot )\) in \(I(t):=[\xi _1(t),\xi _2(t)]\) is finite. If we denote this number by \(Z[\xi _1(t), \xi _2(t)]\), or simply by Z(t), then \(t\rightarrow Z(t)\) is nonincreasing in t, and if for some \(s\in (t_1, t_2)\) the function \(u(s,\cdot )\) has a degenerate zero \(x_0\in I(s)\), then
1.3.1 Proof of Lemma 3.3 when V(x) falls into case (i)
Denote
Arguing indirectly we assume that \(h(t)-L\) changes sign infinitely many times as \(t\rightarrow \infty \). Then we can find \(t_1>t_0>0\) such that
It follows that
Clearly
Therefore we can apply Lemma 5.4 to conclude that, for \(t\in (t_0, t_1)\), the zero number \(\mathcal {Z}(t):=Z[l(t), L(t)]\) of \(w(t,\cdot )\) over the interval [l(t), L(t)] is finite, and has the properties stated in Lemma 5.4. It follows that there can exist at most finitely many time moments in \((t_0, t_1)\) such that \(w(t,\cdot )\) has a degenerate zero in (l(t), L(t)). Therefore we can find \({\tilde{t}}_1\in (t_0, t_1)\) such that \(w(t,\cdot )\) has a finite number of nondegenerate zeros for \(t\in [{\tilde{t}}_1, t_1)\). This implies that \(\mathcal {Z}(t)\) is a constant for \(t\in [{\tilde{t}}_1, t_1)\). We first show that this constant is not 0. Otherwise we have \(w(t,x)<0\) for \(x\in [l(t), L(t)]\) and \(t\in [{\tilde{t}}_1, t_1)\). We may then compare (u(t, x), h(t)) with \((\overline{u}(t,x), \overline{h}(t)):=(V(x), L)\) over \(t\ge {\tilde{t}}_1\), \(x\in [l(t), h(t)]\) to conclude that \(h(t)\le L\) and \(u(t,x)\le V(x)\) for such t and x. By the strong maximum principle, one further deduces \(h(t)<L\) for all \(t>{\tilde{t}}_1\), a contradiction to the existence of \(t_1\).
We may now assume \(m=\mathcal {Z}(t)\ge 1\) for \(t\in [{\tilde{t}}_1, t_1)\). By the implicit function theorem, these m nondegenerate zeros of \(w(t,\cdot )\) can be expressed as \(C^1\) functions \(\gamma _i(t)\) for \(t\in [{\tilde{t}}_1, t_1)\), \(i=1,\ldots , m\), with
As in the proof of Lemma 2.3 in Du et al. (2015), we can show that \(x_i:=\lim _{t\nearrow t_1}\gamma _i(t)\) exists, and each \(x_i\) is a zero of \(w(t_1,\cdot )\), and the set \(\{x_1,\ldots , x_m, L\}\) contains all the zeros of \(w(t_1, \cdot )\) in \([l(t_1), L(t_1)]=[l(t_1), L]\).
We claim that \(x_m=L\). Otherwise \(x_m<L\) and \(w(t,x)<0\) for \(x\in (\gamma _m(t), L(t))\), \(t\in [{\tilde{t}}_1, t_1]\). Since \(w(t_1, L(t_1))=w(t_1, L)=0\), by the Hopf boundary lemma we deduce \(w_x(t_1, L)>0\). It follows that
a contradiction to (5.11). Hence \(x_m=L\).
It is possible that several elements in the set \(\{x_1,\ldots , x_m\}\) is identical to L. Let i be the smallest index such that \(x_i=L\). Then \(1\le i\le m\) and \(w(t_1, x)\) has a fixed sign for \(x\in [x_i-\delta , x_i)=[L-\delta , L)\), where \(\delta >0\) is sufficiently small.
If \(w(t_1, x)>0\) for \(x\in [L-\delta , L)\), then by continuity we can find \(\epsilon _1>0\) small so that \(w(t, L-\delta )>0\) for \(t\in [t_1, t_1+\epsilon _1]\). Then
is a lower solution to the problem satisfied by (u, h) over the region \(x\in [L-\delta , L]\), \(t\in [t_1, t_1+\epsilon _1]\). It follows that \(h(t)\ge L\) and \(u(t,x)\ge V(x)\) in this region, and by the strong maximum principle we further deduce \(h(t)>L\), \(u(t,x)>V(x)\) for \(t\in (t_1, t_1+\epsilon _1]\), \(x\in [L-\delta , L]\). Thus, applying Lemma 5.4 for \(x\in [l(t), L-\delta ]\) we obtain, for \(t\in (t_1, t_1+\epsilon _1]\),
If \(w(t_1, x)<0\) for \(x\in [L-\delta , L)\), then by continuity we can find \(\epsilon _1>0\) small so that \(w(t, L-\delta )<0\) for \(t\in [t_1, t_1+\epsilon _1]\). Then
is an upper solution to the problem satisfied by (u, h) over the region \(x\in [L-\delta , h(t)]\), \(t\in [t_1, t_1+\epsilon _1]\). It follows that \(h(t)\le L\) and \(u(t,x)\le V(x)\) in this region, and by the strong maximum principle we further deduce \(h(t)<L\), \(u(t,x)<V(x)\) for \(t\in (t_1, t_1+\epsilon _1]\), \(x\in [L-\delta , h(t)]\). Thus we again obtain
By our assumption, there exists \(t_2>t_1+\epsilon _1\) such that \(h(t)-L\not =0\) for \(t\in (t_1, t_2)\) and \(h(t_2)-L=0\). We thus have
By an analogous analysis to the one leading to (5.12), we can find \(\epsilon _2>0\) small such that
(Modifications are needed if \(h(t)>L\) for \(t\in (t_1, t_2)\), but they are rather obvious.)
Since Z[l(t), L(t)] is nonnegative and m is a finite integer, the above process can be continued at most m steps, and at the last step, say step k, we conclude that \(w(t,\cdot )\) does not change sign over [l(t), L(t)] for \(t\in (t_k, t_k+\epsilon _k]\). Thus necessarily \(w(t,x)<0\) for \(x\in [l(t), L(t)]\) and \(t\in (t_k, t_k+\epsilon _k]\). We may then compare (u(t, x), h(t)) with (V(x), L) over \(x\in [l(t), h(t)]\), \(t\ge t_k+\epsilon _k\) and apply the strong maximum principle, as before, to conclude that \(u(t,x)<V(x),\; h(t)<L\) for \(t\ge t_k+\epsilon _k\) and \(x\in [l(t), h(t)]\), which is in contradiction to the assumption that \(h(t)-L\) changes sign infinitely many times as \(t\rightarrow \infty \). This completes the proof of Lemma 3.3 for the case that V(x) falls into case (i). \(\square \)
1.3.2 Proof of Lemma 3.3 when V(x) falls into case (ii)
The proof for this case follows the arguments above, except that we have to define l(t) differently, which leads to a case that requires attention. We now define
If \(h(t)>l\) for all \(t>0\), then \(w(t, l(t))=u(t, l)>0\) for all \(t>0\), and we may follow the argument in the proof of case (i) above to conclude, with obvious minor changes.
Consider next the remaining case that \(h(t_0)<l\) for some \(t_0>0\). If \(h(t)<L\) for all \(t>t_0\), then the conclusion of the lemma already holds. Otherwise, there exist \(t_2>t_1>t_0\) such that
Since \(V'(l)>0=V(l)\) and \(u_x(t, h(t))<0=u(t, h(t))\), for \(t>t_1\) but close to \(t_1\), \(w(t,\cdot )\) has a unique nondegenerate zero in (l, h(t)). Since
we can apply Lemma 5.4 to conclude that
and the unique zero of \(w(t,\cdot )\) in [l, h(t)] is nondegenerate. Thus if we denote it by \(\gamma (t)\), then it is a \(C^1\) function of t in \((t_1, t_2)\), and \(l<\gamma (t)<h(t)\). As before we know from Du et al. (2015) that \(x_0:=\lim _{t\nearrow t_2}\gamma (t)\) exists and is a zero of \(w(t_2,\cdot )\).
We claim that \(x_0=L=h(t_2)\). Otherwise \(x_0<L\), and by applying the maximum principle to the equation satisfied by w over \(\{(t,x): \gamma (t)<x<h(t), \; t\in (t_1, t_2]\}\), we deduce that \(w(t,x)<0\) over this region and \(w_x(t_2, L)>0=w(t_2, L)\). It follows that
On the other hand, from \(h(t)<L\) for \(t\in (t_1, t_2)\) we deduce \(h'(t_2)\ge 0\). This controdiction proves our claim that \(x_0=L\). We may now use the maximum principle to the equation of w over \(\{(t,x): l<x<\gamma (t), t_1<t\le t_2\}\) to deduce that \(w(t,x)>0\) in this region. It follows that \((\underline{u}(t,x), \underline{h}(t)):=(V(x), L)\) is a lower solution to the problem for (u, h) over the region \(\{(t,x): l<x<L, t\ge t_2\}\), which implies, by the strong maximum principle, \(h(t)>L\) and \(u(t,x)>V(x)\) in this region. Hence \(h(t)-L\) is positive for all large t, and the conclusion of the lemma holds. \(\square \)
1.3.3 Proof of Lemma 3.3 when V(x) falls into case (iii)
In this case, we divide the proof into three subcases:
In case (iii0), V(x) is an even function of x and so it w(t, x). The arguments used for case (i) carry over easily if we define \(l(t)=-L(t)\).
In the remaining two cases, some extra analysis is needed. We first consider case (iii+). We again want to follow the analysis of case (i) but this time we have to define l(t) rather differently, according to the behavior of w(t, 0) as t increases to infinity.
If \(w(t,0)\not =0\) for all large t, then we take \(l(t)=0\) and the proof in case (i) can be used without change. So we only need to consider the case that
We observe that \(w_x(t,0)=-V'(0)<0\) for all \(t>0\). Moreover, by standard parabolic and elliptic estimates for u(t, x) and V(x), respectively, there exists \(C>0\) independent of \(t\ge 1\) such that
Hence there exists \(\epsilon _0>0\) small such that
Define
If there exists \(t_0> 1\) such that \(J\cap [t_0,\infty )=\emptyset \), then \(w(t,0)\le 0\) for \(t>t_0\) and hence \(w(t,\epsilon _0)<0\) for all \(t>t_0\). We may then take \(l(t)=\epsilon _0\) and the proof of case (i) can be used without change.
So we assume that \(J\cap [t_0,\infty )\not =\emptyset \) for all \(t_0>1\). Since we have assumed that \(w(t,0)=0\) along a sequence of t going to \(\infty \), the open set J is necessarily the union of infinitely many disjoint open intervals:
Set
If \(|t_i-s_i|\le \sigma _0\), then, due to \(w(s_i,0)=w(t_i,0)=0\), we have
and hence
Define
If \(I^0\) is a finite set, then for all large t, \( w(t, \epsilon _0/2)<0\), and hence we can take \(l(t)=\epsilon _0/2\) and argue as in case (i) to finish the proof.
Suppose from now on that \(I^0\) is an infinite set. Then the set of intervals \(\{J_i\}_{i\in I^0}\) can be ordered, namely, we can take \(I^0=\{1,2,\ldots \}\) and require \(t_i<s_{i+1}\) for \(i=1,2,\ldots \) Set
Then for any \(t\in (1,\infty )\backslash J^0\), we have \(w(t, \epsilon _0/2)<0\). Using the ordering property of the intervals in \(J^0\), we now see that
By the monotonisity of w(t, x) in x for \(|x|\le \epsilon _0\), we find that
We now examine the zero number of w(t, x) for \(x\in [-\epsilon _0/2, L(t)]\) when t is in a small neighborhood of \( [s_i, t_i]\), which we denote by \(Z_i[-\epsilon _0/2, L(t)]\), and the zero number of w(t, x) for \(x\in [\epsilon _0/2, L(t)]\) when t belongs to a small neighborhood of \( [t_i, s_{i+1}]\), which we denote by \(Z_i[\epsilon _0/2, L(t)]\).
We note that \(w(t, -\epsilon _0/2)>0\) for t in a small neighborhood of \( [s_i, t_i]\) and \(w(t,\epsilon _0/2)<0\) for t in a small neighborhood of \( [t_i, s_{i+1}]\). Therefore, for all large i, by our arguments in the proof of case (i), \(Z_i[-\epsilon _0/2, L(t)]\) is finite for t in a small neighborhood of \( [s_i, t_i]\), and each time \(h(t)-L\) changes sign, say at \({\tilde{t}}\in [s_i, t_i]\), the value of \(Z_i[-\epsilon _0/2, L(t)]\) is decreased by at least 1 when t increases across \({\tilde{t}}\). The same also holds for \(Z_i[\epsilon _0/2, L(t)]\) for t in a small neighborhood of \([t_i, s_{i+1}]\).
For t close to \(t_i\), clearly
For t close to \(s_{i+1}\), we have
Suppose by way of contradiction that \(h(t)-L\) changes sign infinitely many times as \(t\rightarrow \infty \), say at \(t_n\) with \(t_n\) increasing to infinity. Then by the zero number consideration, no one interval of the form \([s_i, t_i]\), or of the form \([t_i, s_{i+1}]\), can contain infinitely many members from \(\{t_n\}\). Thus there are infinitely many intervals of the form \([s_i, t_i]\) such that each of them contains at least one member of \(\{t_n\}\), or infinitely many intervals of the form \([t_i, s_{i+1}]\) such that each contains at least one member of \(\{t_n\}\). For definiteness, we assume that there exist \(i_k\rightarrow \infty \) and \(n_k\rightarrow \infty \) such that \(t_{n_k}\in [s_{i_k}, t_{i_k}]\), \(i_{k}<i_{k+1}\), \(n_k<n_{k+1}\), \(k=1,2,\ldots \) Then by our analysis above, we have
for all large k. This contradiction shows that \(h(t)-L\) does not change sign for all large t. This completes our proof of Lemma 3.3 for the case that V(x) falls in case (iii+).
The proof for case (iii−) is analogous, where we replace the definition of J by
and then make obvious changes. We omit the details. \(\square \)
Rights and permissions
About this article
Cite this article
Bao, W., Du, Y., Lin, Z. et al. Free boundary models for mosquito range movement driven by climate warming. J. Math. Biol. 76, 841–875 (2018). https://doi.org/10.1007/s00285-017-1159-9
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00285-017-1159-9