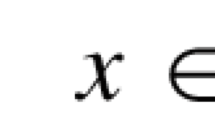Fractional (p, q)-Schrödinger Equations with Critical and Supercritical Growth

Abstract

In this paper, we complete the study started in Ambrosio and Rădulescu (J Math Pures Appl (9) 142:101–145, 2020) on the concentration phenomena for a class of fractional (p, q)-Schrödinger equations involving the fractional critical Sobolev exponent. More precisely, we focus our attention on the following class of fractional (p, q)-Laplacian problems:

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u + V(\varepsilon x) (u^{p-1} + u^{q-1})= f(u)+u^{q^{*}_{s}-1} \, \text{ in } \mathbb {R}^{N}, \\ u\in W^{s, p}(\mathbb {R}^{N})\cap W^{s,q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}, \end{array} \right. \end{aligned}$$

where $\varepsilon >0$ is a small parameter, $s\in (0, 1)$, $1<p<q<\frac{N}{s}$, $q^{*}_{s}=\frac{Nq}{N-sq}$ is the fractional critical Sobolev exponent, $(-\Delta )^{s}_{r}$, with $r\in \{p, q\}$, is the fractional r-Laplacian operator, $V:\mathbb {R}^{N}\rightarrow \mathbb {R}$ is a positive continuous potential such that $\inf _{\partial \Lambda }V>\inf _{\Lambda } V$ for some bounded open set $\Lambda \subset \mathbb {R}^{N}$, and $f:\mathbb {R}\rightarrow \mathbb {R}$ is a continuous nonlinearity with subcritical growth. With the aid of minimax theorems and the Ljusternik–Schnirelmann category theory, we obtain multiple solutions by employing the topological construction of the set where the potential V attains its minimum. We also establish a multiplicity result when $f(t)=t^{\gamma -1}+\mu t^{\tau -1}$, with $1< p<q<\gamma<q^{*}_{s}<\tau$ and $\mu >0$ sufficiently small, by combining a truncation argument with a Moser-type iteration.

1 Introduction

In this paper, we investigate the multiplicity and concentration phenomenon for the following class of fractional (p, q)-Laplacian problems:

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u + V(\varepsilon x) (u^{p-1} + u^{q-1})= f(u)+u^{q^{*}_{s}-1} \, \text{ in } \mathbb {R}^{N}, \\ u\in W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}, \end{array} \right. \end{aligned}$$

(1.1)

where $\varepsilon >0$ is a small parameter, $s\in (0, 1)$, $1< p<q<\frac{N}{s}$, $q^{*}_{s}=\frac{Nq}{N-sq}$ is the fractional critical Sobolev exponent, $V:\mathbb {R}^{N}\rightarrow \mathbb {R}$ and $f:\mathbb {R}\rightarrow \mathbb {R}$ are continuous functions. The leading operator $(-\Delta )^{s}_{r}$, with $r\in \{p, q\}$, is the fractional r-Laplacian operator which may be defined, up to a normalization constant, by setting

$$\begin{aligned} (-\Delta )_{r}^{s}u(x)= 2\, \lim _{r\rightarrow 0} \int _{\mathbb {R}^{N}\setminus B_{r}(x)} \frac{|u(x)- u(y)|^{r-2}(u(x)- u(y))}{|x-y|^{N+sr}} dy \quad (x\in \mathbb {R}^{N}) \end{aligned}$$

for any $u\in C^{\infty }_{c}(\mathbb {R}^{N})$. When $s=1$, equation in (1.1) is closely connected to the study of stationary solutions of general reaction diffusion systems of the form

$$\begin{aligned} u_{t}={{\,\mathrm{div}\,}}[D(u)\nabla u]+c(x,u), \, \text{ with } D(u)=|\nabla u|^{p-2}+|\nabla u|^{q-2}. \end{aligned}$$

(1.2)

Equation (1.2) has a wide range of applications in physical and related sciences, e.g. in biophysics, plasma physics, and chemical reaction design; see [13]. In such contexts, the function u in (1.2) describes a concentration, ${{\,\mathrm{div}\,}}[D(u) \nabla u]$ corresponds to the diffusion with diffusion coefficient D(u), and c(x, u) is related to sources and loss processes. Typically, in chemical and biological applications, the reaction term c(x, u) is a polynomial of u with variable coefficients. We refer to [1, 20, 24, 29, 31] for some engaging existence and multiplicity results for (p, q)-Laplacian problems in bounded or unbounded domains.

In the last years, the study of nonlocal elliptic problems driven by fractional operators has gained a tremendous popularity both for their interesting theoretical structure and in view of concrete applications, such as, obstacle problem, optimization, finance, crystal dislocations, phase transitions, conservation laws, ultra-relativistic limits of quantum mechanics, quasi geostrophic flows and so on; see [18] for more details.

When $s\in (0, 1)$ and $p=q=2$, after rescaling, equation (1.1) boils down to the following nonlinear fractional Schrödinger equation

$$\begin{aligned} \varepsilon ^{2s}(-\Delta )^{s} u+ V(x) u= f(u)+\gamma |u|^{2^{*}_{2}-2} \, \text{ in } \mathbb {R}^{N}, \end{aligned}$$

(1.3)

with $\gamma \in \{0, 1\}$, for which several existence, multiplicity and concentration results have been established via different variational and topological methods. Since we cannot review the huge bibliography on this topic, we refer to the monograph [3] and the references therein.

In the case $s\in (0, 1)$ and $p=q\ne 2$ in (1.1), we derive the following fractional p-Laplacian equation

$$\begin{aligned} \varepsilon ^{sp}(-\Delta )^{s}_{p}u+V(x)|u|^{p-2}u=f(u)+\gamma |u|^{p^{*}_{s}-2}u \, \text{ in } \mathbb {R}^{N}. \end{aligned}$$

(1.4)

We point out that when $p\ne 2$, we have to tackle not only the usual nonlocal character of $(-\Delta )^{s}_{p}$, but also the difficulties given by the corresponding nonlinear behavior. In particular, standard arguments used in the linear case $p=2$ are not so easy to adapt in the case $p\ne 2$ due to the non-Hilbertian structure of $W^{s, p}(\mathbb {R}^{N})$ with $p\ne 2$. For these reasons, there has been a source of interest around nonlocal and fractional problems driven by the fractional p-Laplacian operator; see for instance [6, 16, 27, 30] and the references therein.

On the other hand, in recent years, some existence, multiplicity and regularity results for fractional (p, q)-Laplacian problems appeared in the literature; see [4, 7, 8, 10, 15, 22, 23]. Indeed, such problems involve the sum of two nonlocal nonlinear operators with different scaling properties and so some nontrivial additional technical difficulties arise. For what concerns problems like (1.1), in [8] the authors obtained a multiplicity result for a fractional subcritical (p, q)-Laplacian problem by combining a penalization approach and the Ljusternik–Schnirelmann category theory. We also mention [7] where a multiplicity result was established by requiring that the potential V satisfies the global condition proposed by Rabinowitz [33]:

$$\begin{aligned} \liminf _{|x|\rightarrow \infty } V(x)>\inf _{x\in \mathbb {R}^{N}} V(x). \end{aligned}$$

Particularly motivated by [1, 7, 8], in the first part of this paper we are interested in the multiplicity and concentration behavior as $\varepsilon \rightarrow 0$ of positive solutions to the fractional critical problem (1.1). Next we introduce the assumptions on the potential V and the nonlinearity f. Throughout the paper we will assume that $V\in C(\mathbb {R}^{N}, \mathbb {R})\cap L^{\infty }(\mathbb {R}^{N})$ satisfies the following conditions:

$(V_1)$:

there exists $V_{0}>0$ such that $V_{0}=\inf _{x\in \mathbb {R}^{N}} V(x)$,

$(V_2)$:

there exists an open bounded set $\Lambda \subset \mathbb {R}^{N}$ such that

$$\begin{aligned} V_{0}< \min _{\partial \Lambda } V \quad \text{ and } \quad 0\in M=\{x\in \Lambda : V(x)=V_{0}\}, \end{aligned}$$

and $f\in C(\mathbb {R}, \mathbb {R})$ fulfills the following hypotheses:

$(f_{1})$:

$\displaystyle {\lim _{|t|\rightarrow 0} \frac{|f(t)|}{|t|^{p-1}}=0}$,

$(f_{2})$:

there exist $\sigma _{1}, \sigma _{2}\in (q, q^{*}_{s})$ and $\lambda >1$ such that

$$\begin{aligned} f(t)\ge \lambda t^{\sigma _{1}-1} \quad \text{ for } \text{ all } t>0, \quad \lim _{|t|\rightarrow \infty } \frac{|f(t)|}{|t|^{\sigma _{2}-1}}=0, \end{aligned}$$

$(f_{3})$:

there exists $\vartheta \in (q, \sigma _{2})$ such that $\displaystyle {0<\vartheta F(t)= \vartheta \int _{0}^{t} f(\tau ) \, d\tau \le t f(t)}$ for all $t>0$,

$(f_{4})$:

the map $t\in (0, \infty )\mapsto \displaystyle {\frac{f(t)}{t^{q-1}}}$ is increasing.

Since we look for positive solutions to (1.1), we assume that $f(t)=0$ for $t\le 0$. The first main result of this work can be stated as follows.

Theorem 1.1

Assume that $(V_{1})$–$(V_{2})$ and $(f_{1})$–$(f_{4})$ hold. Then there exists $\lambda ^{*}>0$ such that, for any $\lambda >\lambda ^{*}$ and for any $\delta >0$ such that

$$\begin{aligned} M_{\delta }=\{x\in \mathbb {R}^{N}: \mathrm{dist}(x, M)\le \delta \}\subset \Lambda , \end{aligned}$$

there exists $\varepsilon _{\delta , \lambda }>0$ such that, for any $\varepsilon \in (0, \varepsilon _{\delta , \lambda })$, problem (1.1) has at least $cat_{M_{\delta }}(M)$ positive solutions. Moreover, if $u_{\varepsilon }$ denotes one of these solutions and $x_{\varepsilon }\in \mathbb {R}^{N}$ is a global maximum point of $u_{\varepsilon }$, then

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} V(\varepsilon x_{\varepsilon })=V_{0}. \end{aligned}$$

The proof of Theorem 1.1 will be carried out by using variational and topological arguments. First, we modify in a suitable way the nonlinearity outside of the set $\Lambda$ and we handle an auxiliary problem. The main feature of the corresponding modified energy functional $\mathcal {J}_{\varepsilon }$ is that it satisfies all the geometric assumptions of the mountain-pass theorem [2]. Differently from the subcritical cases examined in [7, 8], a more accurate analysis will be needed to recover compactness. To overcome the lack of compactness caused by the critical exponent, we don’t construct cut-off functions of the extremal functions for the best constant in the Sobolev inequality, as for the equations (1.3) and (1.4) studied in [3, 6], to control the mountain-pass level. Indeed, the nonhomogeneity of the fractional (p, q)-Laplacian operator does not permit to develop this argument. For this reason, we employ a different strategy by considering the solution of an appropriate fractional (p, q)-Laplacian problem in a bounded domain with nonlocal Dirichlet condition; see Lemma 3.3. After that, by invoking the variant of concentration-compactness principle of Lions [25, 26] established in [4], we will prove that the energy functional $\mathcal {J}_{\varepsilon }$ verifies a local Palais-Smale condition; see Lemma 3.5.

To accomplish multiple solutions for the modified problem, we use the technique due to Benci and Cerami [9] based on precise comparisons between the category of some sublevel sets of $\mathcal {J}_{\varepsilon }$ and the category of the set M. Note that f is only continuous, so standard Nehari manifold arguments for $C^1$ functionals are not applicable. The non-differentiability of the Nehari manifold associated with $\mathcal {J}_{\varepsilon }$ will be overcame through the generalized Nehari manifold method by Szulkin and Weth [34]. Finally, we prove that the solutions $u_{\varepsilon }$’s of the modified problem are also solutions of (1.1) for $\varepsilon >0$ small, by exploiting a Moser iteration argument [28] and the Hölder regularity result in [8].

In the second part of this paper, we treat the following supercritical fractional problem:

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u + V(\varepsilon x) (u^{p-1} + u^{q-1})= u^{\gamma -1}+\mu u^{\tau -1} \, \text{ in } \mathbb {R}^{N}, \\ u>0 \text{ in } \mathbb {R}^{N}, \end{array} \right. \end{aligned}$$

(1.5)

where $\varepsilon , \mu >0$ and $1< p<q<\gamma<q^{*}_{s}<\tau$. Our multiplicity result for the supercritical case reads as follows.

Theorem 1.2

Assume that $(V_{1})$-$(V_{2})$ hold. Then there exists $\mu _{0}>0$ such that, for any $\mu \in (0, \mu _{0})$ and for any $\delta >0$ satisfying

$$\begin{aligned} M_{\delta }=\{x\in \mathbb {R}^{N}: \mathrm{dist}(x, M)\le \delta \}\subset \Lambda , \end{aligned}$$

there exists $\varepsilon _{\delta , \mu }>0$ such that, for any $\varepsilon \in (0, \varepsilon _{\delta , \mu })$, problem (1.5) has at least $cat_{M_{\delta }}(M)$ positive solutions. Moreover, if $u_{\varepsilon }$ denotes one of these solutions and $x_{\varepsilon }\in \mathbb {R}^{N}$ is a global maximum point of $u_{\varepsilon }$, then

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} V(\varepsilon x_{\varepsilon })=V_{0}. \end{aligned}$$

The main difficulty in the study of (1.5) consists in the fact that $\tau >q^{*}_{s}$ is supercritical, and we cannot directly apply variational techniques because the corresponding energy functional is not well-defined on the space $W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N})$. In order to circumvent this obstacle, we perform some arguments inspired by [12, 21, 32] which can be summarized as follows. We first truncate in a suitable way the nonlinearity on the right hand side of (1.5), so we deal with a new problem but with subcritical growth. In light of Theorem 1.1 in [8], we know that a multiplicity result for this truncated problem is available. Then we prove a priori bound (independent of $\mu$) for these solutions, and by means of an appropriate Moser type iteration [28] we show that, for $\mu >0$ sufficiently small, the solutions of the truncated problem also solve the original one.

We stress that our theorems complement and improve the main results in [1, 7, 8], in the sense that we are considering multiplicity results for critical and supercritical problems involving continuous nonlinearities and imposing local conditions on the potential V.

The paper is organized as follows. In Sect. 2 we collect some notations and technical results. In Sect. 3 we introduce the modified problem. In Sect. 4 we focus our attention on the limiting problem associated with (1.1). In Sect. 5 we obtain a multiplicity result for the modified problem. Section 6 is devoted to the proof of Theorem 1.1. In the last section we investigate the supercritical problem (1.5).

2 Preliminary Results

Let $p\in [1, \infty ]$ and $A\subset \mathbb {R}^{N}$ be a measurable set. We will use the notation $|\cdot |_{L^{p}(A)}$ to denote the $L^{p}(A)$-norm, and $|\cdot |_{p}$ when $A=\mathbb {R}^{N}$. Let $s\in (0, 1)$, $p\in (1, \infty )$ and $N>sp$. We define $\mathcal {D}^{s, p}(\mathbb {R}^{N})$ as the closure of $C^{\infty }_{c}(\mathbb {R}^{N})$ with respect to

$$\begin{aligned}{}[u]_{s,p}=\left( \iint _{\mathbb {R}^{2N}} \frac{|u(x)-u(y)|^{p}}{|x-y|^{N+sp}}\, dxdy\right) ^{\frac{1}{p}}, \end{aligned}$$

or equivalently

$$\begin{aligned} \mathcal {D}^{s, p}(\mathbb {R}^{N})=\left\{ u\in L^{p^{*}_{s}}(\mathbb {R}^{N}): [u]_{s, p}<\infty \right\} , \end{aligned}$$

where $p^{*}_{s}=\frac{Np}{N-sp}$ is the fractional critical Sobolev exponent. The Sobolev space $W^{s, p}(\mathbb {R}^{N})$ is given by

$$\begin{aligned} W^{s, p}(\mathbb {R}^{N})=\{u\in L^{p}(\mathbb {R}^{N}): [u]_{s, p}<\infty \}. \end{aligned}$$

The space $W^{s, p}(\mathbb {R}^{N})$ is equipped with the norm

$$\begin{aligned} \Vert u\Vert _{W^{s,p}(\mathbb {R}^{N})}=(|u|^{p}_{p}+[u]^{p}_{s, p})^{\frac{1}{p}}. \end{aligned}$$

For $u, v\in W^{s,p}(\mathbb {R}^{N})$, we put

$$\begin{aligned} \langle u, v\rangle _{s,p}=\iint _{\mathbb {R}^{2N}} \frac{|u(x)-u(y)|^{p-2}(u(x)-u(y))(v(x)-v(y))}{|x-y|^{N+sp}} \, dx dy. \end{aligned}$$

The following embeddings are well-known.

Theorem 2.1

[18] Let $s\in (0, 1)$, $p\in (1, \infty )$ and $N>sp$. Then there exists a constant $S_{p}=S(N, s, p)>0$ such that, for any $u\in \mathcal {D}^{s, p}(\mathbb {R}^{N})$,

$$\begin{aligned} S_{p}|u|^{p}_{p^{*}_{s}} \le [u]^{p}_{s, p}. \end{aligned}$$

Moreover, $W^{s, p}(\mathbb {R}^{N})$ is continuously embedded in $L^{t}(\mathbb {R}^{N})$ for any $t\in [p, p^{*}_{s}]$ and compactly in $L^{t}(B_{R})$, for all $R>0$ and any $t\in [1, p^{*}_{s})$.

For the reader’s convenience, we also recall the following vanishing lemma.

Lemma 2.1

[6] Let $N>sp$ and $r\in [p, p^{*}_{s})$. If $\{u_{n}\}_{n\in \mathbb {N}}$ is a bounded sequence in $W^{s, p}(\mathbb {R}^{N})$ and if

$$\begin{aligned} \lim _{n\rightarrow \infty } \sup _{y\in \mathbb {R}^{N}} \int _{B_{R}(y)} |u_{n}|^{r} dx=0, \end{aligned}$$

where $R>0$, then $u_{n}\rightarrow 0$ in $L^{t}(\mathbb {R}^{N})$ for all $t\in (p, p^{*}_{s})$.

Let $s\in (0, 1)$, $p, q\in (1, \infty )$ and consider the space

$$\begin{aligned} \mathcal {W}=W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N}) \end{aligned}$$

endowed with the norm

$$\begin{aligned} \Vert u\Vert _{\mathcal {W}}=\Vert u\Vert _{W^{s,p}(\mathbb {R}^{N})}+\Vert u\Vert _{W^{s,q}(\mathbb {R}^{N})}. \end{aligned}$$

Note that $\mathcal {W}$ is a separable reflexive Banach space (since $W^{s,r}(\mathbb {R}^{N})$, with $r\in (1, \infty )$, is a separable reflexive Banach space). For any $\varepsilon >0$, we introduce the space

$$\begin{aligned} \mathbb {X}_{\varepsilon }=\left\{ u\in \mathcal {W}: \int _{\mathbb {R}^{N}} V(\varepsilon x) \left( |u|^{p}+|u|^{q}\right) \,dx<\infty \right\} \end{aligned}$$

endowed with the norm

$$\begin{aligned} \Vert u\Vert _{\mathbb {X}_{\varepsilon }}= \Vert u\Vert _{V_{\varepsilon }, p} + \Vert u\Vert _{V_{\varepsilon }, q}, \end{aligned}$$

where

$$\begin{aligned} \Vert u\Vert _{V_{\varepsilon }, t}= \left( [u]_{s, t}^{t} + \int _{\mathbb {R}^{N}} V(\varepsilon x) |u|^{t}\, dx\right) ^{\frac{1}{t}} \quad \text{ for } t\in \{p, q\}. \end{aligned}$$

Due to the presence of the critical exponent in (1.1), the following variant of the concentration-compactness lemma of Lions [25, 26] proved in [4] will be crucial. In what follows, we use the notation

$$\begin{aligned} |D^{s} u|^{p}(x)=\int _{\mathbb {R}^{N}} \frac{|u(x)-u(y)|^{p}}{|x-y|^{N+sp}}dy. \end{aligned}$$

Lemma 2.2

[4] Let $\{u_{n}\}_{n\in \mathbb {N}}$ be a sequence in $\mathcal {D}^{s,p}(\mathbb {R}^{N})$ such that $u_{n}\rightharpoonup u$ in $\mathcal {D}^{s,p}(\mathbb {R}^{N})$. Let us assume that

$$\begin{aligned} |D^{s} u_{n}|^{p}\rightharpoonup \mu , \quad |u_{n}|^{p^{*}_{s}}\rightharpoonup \nu , \end{aligned}$$

in the sense of measure, where $\mu$ and $\nu$ are two non-negative bounded measures on $\mathbb {R}^{N}$. Then, there exist an at most a countable set I, a family of distinct points $\{x_{i}\}_{i\in I}\subset \mathbb {R}^{N}$ and $\{\mu _{i}\}_{i\in I}, \{\nu _{i}\}_{i\in I}\subset (0, \infty )$ such that

$$\begin{aligned}&\nu =|u|^{p^{*}_{s}}+\sum _{i\in I} \nu _{i} \delta _{x_{i}},\\&\mu \ge |D^{s}u|^{p}+\sum _{i\in I} \mu _{i} \delta _{x_{i}}, \\&\mu _{i}\ge S_{p} \nu _{i}^{\frac{p}{p^{*}_{s}}} \quad \text{ for } \text{ all } i\in I. \end{aligned}$$

3 Variational Framework and Modified Problem

As in [8], we use a del Pino-Felmer penalization type approach [17] to deal with problem (1.1). Take

$$\begin{aligned} K>\frac{q}{p}>1 \end{aligned}$$

and $a>0$ such that

$$\begin{aligned} f(a)+ a^{q^{*}_{s}-1}=\frac{V_{0}}{K}a^{q-1}. \end{aligned}$$

We define

$$\begin{aligned} {\tilde{f}}(t)=\left\{ \begin{array}{ll} f(t)+ (t^+)^{q^{*}_{s}-1} &{} \text{ if } t\le a,\\ \displaystyle \frac{V_{0}}{K} t^{q-1} &{} \text{ if } t>a, \end{array} \right. \end{aligned}$$

and

$$\begin{aligned} g(x, t)= \left\{ \begin{array}{ll} \chi _{\Lambda }(x)(f(t)+t^{q^{*}_{s}-1})+(1-\chi _{\Lambda }(x)){\tilde{f}}(t) &{} \text{ if } t>0, \\ 0 &{} \text{ if } t\le 0, \end{array} \right. \end{aligned}$$

where $\chi _{A}$ denotes the characteristic function of $A\subset \mathbb {R}^{N}$. By $(f_1)$-$(f_4)$, we deduce that $g: \mathbb {R}^{N}\times \mathbb {R}\rightarrow \mathbb {R}$ is a Carathéodory function satisfying the following conditions:

$(g_1)$:

$\displaystyle \lim _{t\rightarrow 0} \frac{g(x,t)}{t^{p-1}}=0$ uniformly with respect to $x\in \mathbb {R}^{N}$,

$(g_2)$:

$g(x,t)\le f(t)+ t^{q^{*}_{s}-1}$ for all $x\in \mathbb {R}^{N}$ and $t>0$,

$(g_3)$:

(i) $0< \vartheta G(x,t)\le g(x,t)t$ for all $x\in \Lambda$ and $t>0$, (ii) $0< qG(x,t)\le g(x,t)t\le \frac{V_{0}}{K} t^{q}$ for all $x\in \Lambda ^{c}$ and $t>0$,

$(g_4)$:

for each $x\in \mathbb {R}^{N}$ the function $t\mapsto \displaystyle \frac{g(x,t)}{t^{q-1}}$ is increasing in $(0, \infty )$.

Let us introduce the following auxiliary problem:

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u+V(\varepsilon x)(u^{p-1}+u^{q-1})=g(\varepsilon x, u) \, \text{ in } \mathbb {R}^{N}, \\ u\in W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}. \end{array} \right. \end{aligned}$$

(3.1)

Notice that if $u_{\varepsilon }$ is a solution to (3.1) such that $u_{\varepsilon }(x)\le a$ for all $x\in \Lambda _{\varepsilon }^{c}$, where $\Lambda _{\varepsilon }=\{x\in \mathbb {R}^{N} \, : \, \varepsilon x\in \Lambda \}$, then $u_{\varepsilon }$ is also a solution to (1.1). Then we consider the functional $\mathcal {J}_{\varepsilon }: \mathbb {X}_{\varepsilon }\rightarrow \mathbb {R}$ associated with (3.1), that is

$$\begin{aligned} \mathcal {J}_{\varepsilon }(u)= \frac{1}{p}\Vert u\Vert _{V_{\varepsilon },p}^{p}+\frac{1}{q}\Vert u\Vert _{V_{\varepsilon },q}^{q}-\int _{\mathbb {R}^{N}} G(\varepsilon x, u)\, dx. \end{aligned}$$

Obviously, $\mathcal {J}_{\varepsilon }\in C^{1}(\mathbb {X}_{\varepsilon }, \mathbb {R})$ and it holds

$$\begin{aligned} \langle \mathcal {J}'_{\varepsilon }(u), \varphi \rangle&=\langle u, \varphi \rangle _{s, p}+\int _{\mathbb {R}^{N}}V(\varepsilon x) |u|^{p-2}u\,\varphi \, dx+\langle u, \varphi \rangle _{s, q} \\&\quad + \int _{\mathbb {R}^{N}} V(\varepsilon x)|u|^{q-2}u\,\varphi \, dx - \int _{\mathbb {R}^{N}} g(\varepsilon x, u)\varphi \, dx \end{aligned}$$

for any $u, \varphi \in \mathbb {X}_{\varepsilon }$. We define the Nehari manifold $\mathcal {N}_{\varepsilon }$ associated with $\mathcal {J}_{\varepsilon }$, i.e.

$$\begin{aligned} \mathcal {N}_{\varepsilon }=\{u\in \mathbb {X}_{\varepsilon }\setminus \left\{ 0\right\} : \langle \mathcal {J}'_{\varepsilon }(u), u\rangle =0\}, \end{aligned}$$

and we set

$$\begin{aligned} c_{\varepsilon }=\inf _{u\in \mathcal {N}_{\varepsilon }} \mathcal {J}_{\varepsilon }(u). \end{aligned}$$

Let $\mathbb {X}_{\varepsilon }^{+}$ be the open set given by

$$\begin{aligned} \mathbb {X}_{\varepsilon }^{+}=\{u\in \mathbb {X}_{\varepsilon }: |\mathrm{supp}(u^{+})\cap \Lambda _{\varepsilon }|>0\} \end{aligned}$$

and $\mathbb {S}_{\varepsilon }^{+}=\mathbb {S}_{\varepsilon }\cap \mathbb {X}_{\varepsilon }^{+}$, where $\mathbb {S}_{\varepsilon }=\{u\in \mathbb {X}_{\varepsilon }: \Vert u\Vert _{\mathbb {X}_{\varepsilon }}=1\}$. Note that $\mathbb {S}_{\varepsilon }^{+}$ is an incomplete $C^{1,1}$-manifold of codimension one. Hence, $\mathbb {X}_{\varepsilon }=T_{u}\mathbb {S}_{\varepsilon }^{+}\oplus \mathbb {R}u$ for all $u\in \mathbb {S}_{\varepsilon }^{+}$, where

$$\begin{aligned} T_{u}\mathbb {S}_{\varepsilon }^{+}&=\Biggl \{v\in \mathbb {X}_{\varepsilon }: \langle u, v\rangle _{s,p}+\langle u, v\rangle _{s,q} +\int _{\mathbb {R}^{N}} V(\varepsilon x)(|u|^{p-2}uv+|u|^{q-2}uv) \, dx=0 \Biggr \}. \end{aligned}$$

The next lemma ensures that $\mathcal {J}_{\varepsilon }$ possesses a mountain pass geometry [2].

Lemma 3.1

The functional $\mathcal {J}_{\varepsilon }$ has the following properties:

(i):

There exist $\alpha , \rho >0$ such that $\mathcal {J}_{\varepsilon }(u) \ge \alpha$ for all $u\in \mathbb {X}_{\varepsilon }$ such that $\Vert u\Vert _{\mathbb {X}_{\varepsilon }}= \rho$.

(ii):

There exists $e\in \mathbb {X}_{\varepsilon }$ such that $\Vert e\Vert _{\mathbb {X}_{\varepsilon }}>\rho$ and $\mathcal {J}_{\varepsilon }(e)<0$.

Proof

(i) Fix $\zeta \in (0, V_{0})$. By $(g_{2})$, $(f_{1})$ and $(f_{2})$, there is $C_{\zeta }>0$ such that

$$\begin{aligned} |g(x, t)|\le \zeta |t|^{p-1}+ C_{\zeta }|t|^{q^{*}_{s}-1} \quad \text{ for } (x,t)\in \mathbb {R}^{N}\times \mathbb {R}. \end{aligned}$$

Therefore,

$$\begin{aligned} \mathcal {J}_{\varepsilon }(u)&\ge \frac{1}{p}\Vert u\Vert _{V_{\varepsilon },p}^{p}+\frac{1}{q}\Vert u\Vert _{V_{\varepsilon },q}^{q} - \frac{\zeta }{p} |u|_{p}^{p}- \frac{C_{\zeta }}{q^{*}_{s}} |u|_{q^{*}_{s}}^{q^{*}_{s}} \\&\ge C_{1}\Vert u\Vert ^{p}_{V_{\varepsilon },p}+\frac{1}{q}\Vert u\Vert _{V_{\varepsilon },q}^{q}- \frac{C_{\zeta }}{q^{*}_{s}} |u|_{q^{*}_{s}}^{q^{*}_{s}}. \end{aligned}$$

Taking $\Vert u\Vert _{\mathbb {X}_{\varepsilon }}=\rho \in (0, 1)$ and using $1<p<q$, we have $\Vert u\Vert _{V_{\varepsilon },p}<1$ and thus $\Vert u\Vert ^{p}_{V_{\varepsilon },p}\ge \Vert u\Vert ^{q}_{V_{\varepsilon },p}$. Recalling that

$$\begin{aligned} a^{t}+b^{t}\ge C_{t} (a+b)^{t} \quad \text{ for } \text{ all } a, b\ge 0 \, \text{ and } t>1, \end{aligned}$$

and invoking Theorem 2.1, we deduce that

$$\begin{aligned} \mathcal {J}_{\varepsilon }(u)\ge C_{2} \Vert u\Vert ^{q}_{\mathbb {X}_{\varepsilon }}-\frac{C_{\zeta }}{q^{*}_{s}} |u|_{q^{*}_{s}}^{q^{*}_{s}}\ge C_{2} \Vert u\Vert ^{q}_{\mathbb {X}_{\varepsilon }}-C_{3} \Vert u\Vert _{\mathbb {X}_{\varepsilon }}^{q^{*}_{s}}. \end{aligned}$$

Then we can find $\alpha >0$ such that $\mathcal {J}_{\varepsilon }(u)\ge \alpha$ for all $u\in \mathbb {X}_{\varepsilon }$ such that $\Vert u\Vert _{\mathbb {X}_{\varepsilon }}= \rho$.

(ii) Assumption $(f_{3})$ implies that

$$\begin{aligned} F(t)\ge A t^{\vartheta }-B \quad \text{ for } \text{ all } t\ge 0. \end{aligned}$$

Thus, for all $u\in \mathbb {X}^{+}_{\varepsilon }$ and $t>0$, we have

$$\begin{aligned}&\mathcal {J}_{\varepsilon }(t u) \le \frac{t^{p}}{p} \Vert u\Vert ^{p}_{V_{\varepsilon }, p} +\frac{t^{q}}{q} \Vert u\Vert ^{q}_{V_{\varepsilon }, q}- A t^{\vartheta } \int _{\Lambda _{\varepsilon }} (u^{+})^{\vartheta } \, dx \\&\quad +B|\mathrm{supp}(u^{+})\cap \Lambda _{\varepsilon }| \rightarrow -\infty \quad \text{ as } t\rightarrow \infty \end{aligned}$$

since $\vartheta>q>p$. $\square$

The next two results are very useful since they allow us to overcome the non-differentiability of $\mathcal {N}_{\varepsilon }$ and the incompleteness of $\mathbb {S}_{\varepsilon }^{+}$.

Lemma 3.2

The following properties hold:

(i):

For each $u\in \mathbb {X}_{\varepsilon }^{+}$, let $h_{u}:\mathbb {R}^{+}\rightarrow \mathbb {R}$ be defined by $h_{u}(t)= \mathcal {J}_{\varepsilon }(tu)$. Then, there is a unique $t_{u}>0$ such that

$$\begin{aligned}&h'_{u}(t)>0 \, \text{ for } \text{ all } t\in (0, t_{u}),\\&h'_{u}(t)<0 \, \text{ for } \text{ all } t\in (t_{u}, \infty ). \end{aligned}$$

(ii):

There exists $\tau >0$, independent of u, such that $t_{u}\ge \tau$ for any $u\in \mathbb {S}_{\varepsilon }^{+}$. Moreover, for each compact set $\mathbb {K}\subset \mathbb {S}_{\varepsilon }^{+}$, there is a positive constant $C_{\mathbb {K}}$ such that $t_{u}\le C_{\mathbb {K}}$ for any $u\in \mathbb {K}$.

(iii):

The map ${\hat{m}}_{\varepsilon }: \mathbb {X}_{\varepsilon }^{+}\rightarrow \mathcal {N}_{\varepsilon }$ given by ${\hat{m}}_{\varepsilon }(u)= t_{u}u$ is continuous and $m_{\varepsilon }= {\hat{m}}_{\varepsilon }|_{\mathbb {S}_{\varepsilon }^{+}}$ is a homeomorphism between $\mathbb {S}_{\varepsilon }^{+}$ and $\mathcal {N}_{\varepsilon }$. Moreover, $m_{\varepsilon }^{-1}(u)=\frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}$.

(iv):

If there is a sequence $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {S}_{\varepsilon }^{+}$ such that $\mathrm{dist}(u_{n}, \partial \mathbb {S}_{\varepsilon }^{+})\rightarrow 0$, then $\Vert m_{\varepsilon }(u_{n})\Vert _{\mathbb {X}_{\varepsilon }}\rightarrow \infty$ and $\mathcal {J}_{\varepsilon }(m_{\varepsilon }(u_{n}))\rightarrow \infty$.

Proof

(i) From the proof of Lemma 3.1, we see that $h_{u}(0)=0$, $h_{u}(t)>0$ for $t>0$ small enough and $h_{u}(t)<0$ for $t>0$ sufficiently large. Then there exists a global maximum point $t_{u}>0$ for $h_{u}$ in $[0, \infty )$ such that $h_{u}'(t_{u})=0$ and $t_{u}u \in \mathcal {N}_{\varepsilon }$. We claim that $t_{u}>0$ is the unique number such that $h_{u}'(t_{u})=0$. Let $t_{1}, t_{2}>0$ be such that $h_{u}'(t_{1})=h_{u}'(t_{2})=0$, or equivalently

$$\begin{aligned}&t_{1}^{p-1}\Vert u\Vert _{V_{\varepsilon },p}^{p}+t_{1}^{q-1}\Vert u\Vert _{V_{\varepsilon },q}^{q}= \int _{\mathbb {R}^{N}} g(\varepsilon x, t_{1}u) u\, dx, \end{aligned}$$

(3.2)

$$\begin{aligned}&t_{2}^{p-1}\Vert u\Vert _{V_{\varepsilon },p}^{p}+t_{2}^{q-1} \Vert u\Vert _{V_{\varepsilon },q}^{q}= \int _{\mathbb {R}^{N}} g(\varepsilon x, t_{2}u) u\, dx. \end{aligned}$$

(3.3)

Hence,

$$\begin{aligned} \frac{\Vert u\Vert ^{p}_{V_{\varepsilon },p}}{t_{1}^{q-p}}+\Vert u\Vert _{V_{\varepsilon },q}^{q}=\int _{\mathbb {R}^{N}} \frac{g(\varepsilon x, t_{1}u)}{(t_{1}u)^{q-1}} u^{q} dx \end{aligned}$$

and

$$\begin{aligned} \frac{\Vert u\Vert ^{p}_{V_{\varepsilon },p}}{t_{2}^{q-p}}+\Vert u\Vert _{V_{\varepsilon },q}^{q}=\int _{\mathbb {R}^{N}} \frac{g(\varepsilon x, t_{2}u)}{(t_{2}u)^{q-1}} u^{q} dx. \end{aligned}$$

Accordingly,

$$\begin{aligned} \left( \frac{1}{t_{1}^{q-p}}-\frac{1}{t_{2}^{q-p}}\right) \Vert u\Vert _{V_{\varepsilon },p}^{p}&=\int _{\mathbb {R}^{N}} \left( \frac{g(\varepsilon x, t_{1}u)}{(t_{1}u)^{q-1}} - \frac{g(\varepsilon x, t_{2}u)}{(t_{2}u)^{q-1}}\right) u^{q} dx. \end{aligned}$$

From $(g_{4})$ and $q>p$, we obtain that $t_{1}=t_{2}$.

(ii) Let $u\in \mathbb {S}_{\varepsilon }^{+}$. By (i), we can find $t_{u}>0$ such that $h_{u}'(t_{u})=0$, that is

$$\begin{aligned} t_{u}^{p-1} \Vert u\Vert _{V_{\varepsilon },p}^{p}+t_{u}^{q-1} \Vert u\Vert _{V_{\varepsilon },q}^{q}= \int _{\mathbb {R}^{N}} g(\varepsilon x, t_{u}u) \,u \,dx. \end{aligned}$$

Fix $\xi >0$. From $(g_2)$, $(f_1)$, $(f_2)$ and Theorem 2.1, we have that

$$\begin{aligned} t_{u}^{p-1} \Vert u\Vert _{V_{\varepsilon },p}^{p}+t_{u}^{q-1} \Vert u\Vert _{V_{\varepsilon },q}^{q}&\le \int _{\mathbb {R}^{3}} g(\varepsilon x, t_{u}u)\, u\, dx\le \xi t_{u}^{p-1}\Vert u\Vert ^{p}_{V_{\varepsilon },p} + C_{\xi } t_{u}^{q^{*}_{s}-1}\Vert u\Vert _{V_{\varepsilon },q}^{q^{*}_{s}}. \end{aligned}$$

Taking $\xi >0$ sufficiently small and recalling that $1=\Vert u\Vert _{\mathbb {X}_{\varepsilon }}\ge \Vert u\Vert _{V_{\varepsilon },q}$, we get

$$\begin{aligned} Ct^{p-1}_{u}\Vert u\Vert _{V_{\varepsilon },p}^{p}+t_{u}^{q-1} \Vert u\Vert _{V_{\varepsilon },q}^{q}\le Ct_{u}^{q^{*}_{s}-1}\Vert u\Vert _{V_{\varepsilon },q}^{q^{*}_{s}}\le Ct_{u}^{q^{*}_{s}-1}. \end{aligned}$$

If $t_{u}\le 1$, then $t_{u}^{q-1}\le t_{u}^{p-1}$, and using the fact that $1=\Vert u\Vert _{\mathbb {X}_{\varepsilon }}\ge \Vert u\Vert _{V_{\varepsilon },p}$ and $q>p$ imply that $\Vert u\Vert _{V_{\varepsilon },p}^{p}\ge \Vert u\Vert ^{q}_{V_{\varepsilon },p}$, we can see that

$$\begin{aligned} Ct_{u}^{q-1}= & {} Ct_{u}^{q-1} \Vert u\Vert ^{q}_{\mathbb {X}_{\varepsilon }} \le t_{u}^{q-1} (C\Vert u\Vert _{V_{\varepsilon },p}^{q}+\Vert u\Vert _{V_{\varepsilon },q}^{q})\le t_{u}^{q-1}(C\Vert u\Vert _{V_{\varepsilon },p}^{p}+\Vert u\Vert _{V_{\varepsilon },q}^{q}) \\\le & {} Ct_{u}^{q^{*}_{s}-1}. \end{aligned}$$

Since $q^{*}_{s}>q$, we can find $\tau >0$, independent of u, such that $t_{u}\ge \tau$.

When $t_{u}>1$, then $t_{u}^{q-1}>t_{u}^{p-1}$, and noting that $1=\Vert u\Vert _{\mathbb {X}_{\varepsilon }}\ge \Vert u\Vert _{V_{\varepsilon },p}$ and that $q>p$ yield $\Vert u\Vert _{V_{\varepsilon },p}^{p}\ge \Vert u\Vert ^{q}_{V_{\varepsilon },p}$, we obtain

$$\begin{aligned} Ct_{u}^{p-1}= & {} Ct_{u}^{p-1} \Vert u\Vert ^{q}_{\mathbb {X}_{\varepsilon }} \le t_{u}^{p-1} (C\Vert u\Vert _{V_{\varepsilon },p}^{q}+\Vert u\Vert _{V_{\varepsilon },q}^{q})\\\le & {} t_{u}^{p-1}(C\Vert u\Vert _{V_{\varepsilon },p}^{p}+\Vert u\Vert _{V_{\varepsilon },q}^{q}) \le Ct_{u}^{q^{*}_{s}-1}. \end{aligned}$$

As $q^{*}_{s}>q>p$, there exists $\tau >0$, independent of u, such that $t_{u}\ge \tau$.

Now, let $\mathbb {K}\subset \mathbb {S}_{\varepsilon }^{+}$ be a compact set, and assume by contradiction that there exists a sequence $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {K}$ such that $t_{n}=t_{u_{n}}\rightarrow \infty$. Then there exists $u\in \mathbb {K}$ such that $u_{n}\rightarrow u$ in $\mathbb {X}_{\varepsilon }$. From (ii) of Lemma 3.1, we have that

$$\begin{aligned} \mathcal {J}_{\varepsilon }(t_{n}u_{n})\rightarrow -\infty . \end{aligned}$$

(3.4)

On the other hand, if $v\in \mathcal {N}_{\varepsilon }$, by $\langle \mathcal {J}_{\varepsilon }'(v), v \rangle =0$ and $(g_{3})$ we get

$$\begin{aligned} \mathcal {J}_{\varepsilon }(v)= \mathcal {J}_{\varepsilon }(v)- \frac{1}{\vartheta } \langle \mathcal {J}_{\varepsilon }'(v), v \ge {\tilde{C}}( \Vert v\Vert _{V_{\varepsilon },p}^{p}+\Vert v\Vert _{V_{\varepsilon },q}^{q}). \end{aligned}$$

Taking $v_{n}=t_{u_{n}}u_{n}\in \mathcal {N}_{\varepsilon }$ in the above inequality, we find

$$\begin{aligned} \mathcal {J}_{\varepsilon }(t_{n}u_{n})\ge {\tilde{C}} (\Vert v_{n}\Vert _{V_{\varepsilon },p}^{p}+\Vert v_{n}\Vert _{V_{\varepsilon },q}^{q}). \end{aligned}$$

Since $\Vert v_{n}\Vert _{\mathbb {X}_{\varepsilon }}=t_{n}\rightarrow \infty$ and $\Vert v_{n}\Vert _{\mathbb {X}_{\varepsilon }}=\Vert v_{n}\Vert _{V_{\varepsilon },p}+\Vert v_{n}\Vert _{V_{\varepsilon },q}$, we can use (3.4) to achieve a contradiction.

(iii) Let us observe that ${\hat{m}}_{\varepsilon }$, $m_{\varepsilon }$ and $m_{\varepsilon }^{-1}$ are well-defined. Indeed, by (i), for each $u\in \mathbb {X}_{\varepsilon }^{+}$ there is a unique ${\hat{m}}_{\varepsilon }(u)\in \mathcal {N}_{\varepsilon }$. On the other hand, if $u\in \mathcal {N}_{\varepsilon }$ then $u\in \mathbb {X}^{+}_{\varepsilon }$. Otherwise, if this is not true, we have

$$\begin{aligned} |{{\,\mathrm{supp}\,}}(u^{+}) \cap \Lambda _{\varepsilon }|=0, \end{aligned}$$

and from (g3)-(ii) we derive that

$$\begin{aligned} \Vert u\Vert _{V_{\varepsilon },p}^{p}+\Vert u\Vert ^{q}_{V_{\varepsilon },q}&= \int _{\mathbb {R}^{N}} g(\varepsilon x, u)\, u \, dx= \int _{\Lambda _{\varepsilon }^{c}} g(\varepsilon x, u)\, u \, dx + \int _{\Lambda _{\varepsilon }} g(\varepsilon x, u)\, u \, dx \nonumber \\&= \int _{\Lambda _{\varepsilon }^{c}} g(\varepsilon x, u^{+})\, u^{+} \, dx \nonumber \\&\le \frac{1}{K} \int _{\Lambda _{\varepsilon }^{c}} V(\varepsilon x) |u|^{q} \,dx \nonumber \\&\le \frac{1}{K} \Vert u\Vert ^{q}_{V_{\varepsilon },q} \end{aligned}$$

(3.5)

which is impossible because $K>1$ and $u\ne 0$. Therefore, $m_{\varepsilon }^{-1}(u)= \frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}\in \mathbb {S}_{\varepsilon }^{+}$ is well-defined and continuous. Since,

$$\begin{aligned} m_{\varepsilon }^{-1}(m_{\varepsilon }(u))= m_{\varepsilon }^{-1}(t_{u}u)= \frac{t_{u}u}{\Vert t_{u}u\Vert _{\mathbb {X}_{\varepsilon }}}= \frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}=u \quad \text{ for } \text{ all } u\in \mathbb {S}_{\varepsilon }^{+}, \end{aligned}$$

we infer that $m_{\varepsilon }$ is a bijection. To prove that ${\hat{m}}_{\varepsilon }: \mathbb {X}_{\varepsilon }^{+}\rightarrow \mathcal {N}_{\varepsilon }$ is continuous, let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {X}_{\varepsilon }^{+}$ and $u\in \mathbb {X}^{+}_{\varepsilon }$ be such that $u_{n}\rightarrow u$ in $\mathbb {X}_{\varepsilon }$. By (ii), there exists $t_{0}>0$ such that $t_{n}=t_{u_{n}}\rightarrow t_{0}$. Using $t_{n}\frac{u_{n}}{\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}}\in \mathcal {N}_{\varepsilon }$, that is

$$\begin{aligned} t_{n}^{p} \frac{\Vert u_{n}\Vert ^{p}_{V_{\varepsilon },p}}{\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}^{p}}+t_{n}^{q} \frac{\Vert u_{n}\Vert _{V_{\varepsilon },q}^{q}}{\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}^{q}}= \int _{\mathbb {R}^{N}} g\left( \varepsilon x, t_{n}\frac{u_{n}}{\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}}\right) \, t_{n} \frac{u_{n}}{\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}} \, dx, \end{aligned}$$

and letting $n\rightarrow \infty$ we obtain

$$\begin{aligned} t_{0}^{p} \frac{\Vert u\Vert ^{p}_{V_{\varepsilon },p}}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}^{p}}+t_{0}^{q} \frac{\Vert u\Vert ^{q}_{V_{\varepsilon },q}}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}^{q}}= \int _{\mathbb {R}^{N}} g\left( \varepsilon x, t_{0}\frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}\right) \, t_{0}\frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}\, dx, \end{aligned}$$

which means that $t_{0}\frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}\in \mathcal {N}_{\varepsilon }$. From (i), $t_{\frac{u}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}}}= t_{0}$ and this implies that ${\hat{m}}_{\varepsilon }(u_{n})\rightarrow {\hat{m}}_{\varepsilon }(u)$ in $\mathbb {X}_{\varepsilon }^{+}$. Thus, ${\hat{m}}_{\varepsilon }$ and $m_{\varepsilon }$ are continuous maps.

(iv) Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {S}_{\varepsilon }^{+}$ be a sequence such that $\mathrm{dist}(u_{n}, \partial \mathbb {S}_{\varepsilon }^{+})\rightarrow 0$. Then, for each $v\in \partial \mathbb {S}_{\varepsilon }^{+}$ and $n\in \mathbb {N}$, we have $u_{n}^{+}\le |u_{n}-v|$ a.e. in $\Lambda _{\varepsilon }$. Therefore, by $(V_1)$, $(V_2)$ and Theorem 2.1, we can see that for each $r\in [p, q^{*}_{s}]$ there exists $C_{r}>0$ such that

$$\begin{aligned} |u_{n}^{+}|_{L^{r}(\Lambda _{\varepsilon })}&\le \inf _{v\in \partial \mathbb {S}_{\varepsilon }^{+}} |u_{n}- v|_{L^{r}(\Lambda _{\varepsilon })}\\&\le C_{r} \inf _{v\in \partial \mathbb {S}_{\varepsilon }^{+}} \Vert u_{n}- v\Vert _{\mathbb {X}_{\varepsilon }} \quad \text{ for } \text{ all } n\in \mathbb {N}. \end{aligned}$$

Using $(g_{2})$, $(f_{1})$, $(f_{2})$, $(g_{3})$-(ii), and $q>p$, we have, for all $t>0$,

$$\begin{aligned} \int _{\mathbb {R}^{N}} G(\varepsilon x, tu_{n})\, dx&= \int _{\Lambda _{\varepsilon }^{c}} G(\varepsilon x, tu_{n})\, dx + \int _{\Lambda _{\varepsilon }} G(\varepsilon x, tu_{n})\, dx\\&\le \frac{V_{0}}{Kq} \int _{\Lambda _{\varepsilon }^{c}} t^{q}|u_{n}|^{q} \,dx+ \int _{\Lambda _{\varepsilon }} \left( F(tu_{n})+ \frac{1}{q^{*}_{s}}(tu_{n}^+)^{q^{*}_{s}}\right) \, dx\\&\le \frac{t^{q}}{Kp} \int _{\mathbb {R}^{N}} V(\varepsilon x)|u_{n}|^{q}\, dx+ C_{1}t^{p} \int _{\Lambda _{\varepsilon }} (u_{n}^{+})^{p} dx+ C_{2} t^{q^{*}_{s}} \\&\quad \times \int _{\Lambda _{\varepsilon }} (u_{n}^{+})^{q^{*}_{s}} dx\\&\le \frac{t^{q}}{Kp} \int _{\mathbb {R}^{N}} V(\varepsilon x)|u_{n}|^{q}\, dx+ C_{p}' t^{p} \mathrm{dist}(u_{n}, \partial \mathbb {S}_{\varepsilon }^{+})^{p} \\&\quad + C_{\nu }' t^{q^{*}_{s}}\mathrm{dist}(u_{n}, \partial \mathbb {S}_{\varepsilon }^{+})^{q^{*}_{s}}. \end{aligned}$$

Consequently,

$$\begin{aligned} \int _{\mathbb {R}^{N}} G(\varepsilon x, tu_{n})\, dx \le \frac{t^q}{Kp} \int _{\mathbb {R}^{N}} V(\varepsilon x)|u_{n}|^{q}\, dx+o_{n}(1). \end{aligned}$$

(3.6)

Now, we note that $K>\frac{q}{p}>1$, and that $1=\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}\ge \Vert u_{n}\Vert _{V_{\varepsilon },p}$ implies that $\Vert u_{n}\Vert ^{p}_{V_{\varepsilon },p}\ge \Vert u_{n}\Vert ^{q}_{V_{\varepsilon },p}$. Then, for all $t>1$,

$$\begin{aligned} \begin{aligned}&\frac{t^{p}}{p} \Vert u_{n}\Vert ^{p}_{V_{\varepsilon },p}+\frac{t^{q}}{q} \Vert u_{n}\Vert ^{q}_{V_{\varepsilon },q}-\frac{t^{q}}{Kp} \int _{\mathbb {R}^{N}} V(\varepsilon x)|u_{n}|^{q}\, dx \\&= \frac{t^{p}}{p} \Vert u_{n}\Vert ^{p}_{V_{\varepsilon },p}+\frac{t^{q}}{q} [u_{n}]^{q}_{s, q}+t^{q} \left( \frac{1}{q}-\frac{1}{Kp}\right) \int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{q}\, dx \\&\ge C_{1} t^{p} \Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}+C_{2} t^{q} \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q} \\&\ge C_{1} t^{p} \Vert u_{n}\Vert _{V_{\varepsilon },p}^{q}+C_{2} t^{q} \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q} \\&\ge C_{1} t^{p} \Vert u_{n}\Vert _{V_{\varepsilon },p}^{q}+C_{2} t^{p} \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q} \\&\ge C_{3} t^{p} (\Vert u_{n}\Vert _{V_{\varepsilon },p}+\Vert u_{n}\Vert _{V_{\varepsilon },q})^{q}=C_{3}t^{p}. \end{aligned}\end{aligned}$$

(3.7)

From the definition of $m_{\varepsilon }(u_{n})$ and employing (3.6), (3.7), we obtain

$$\begin{aligned} \liminf _{n\rightarrow \infty } \mathcal {J}_{\varepsilon }(m_{\varepsilon }(u_{n}))&\ge \liminf _{n\rightarrow \infty } \mathcal {J}_{\varepsilon }(t u_{n})\ge C_{3} t^{p} \quad \text{ for } \text{ all } t>1 \end{aligned}$$

which yields

$$\begin{aligned}&\liminf _{n\rightarrow \infty } \left\{ \frac{1}{p}\Vert m_{\varepsilon }(u_{n})\Vert _{V_{\varepsilon },p}^{p}+\frac{1}{q} \Vert m_{\varepsilon }(u_{n})\Vert _{V_{\varepsilon },q}^{q} \right\} \\&\qquad \ge \liminf _{n\rightarrow \infty } \mathcal {J}_{\varepsilon }(m_{\varepsilon }(u_{n}))\ge C_{3}t^{p} \quad \text{ for } \text{ all } t>1. \end{aligned}$$

Letting $t\rightarrow \infty$, we deduce that $\Vert m_{\varepsilon }(u_{n})\Vert _{\mathbb {X}_{\varepsilon }}\rightarrow \infty$ and that $\mathcal {J}_{\varepsilon }(m_{\varepsilon }(u_{n}))\rightarrow \infty$ as $n\rightarrow \infty$. $\square$

Remark 3.1

There exists $\kappa >0$, independent of $\varepsilon$, such that $\Vert u\Vert _{\mathbb {X}_{\varepsilon }}\ge \kappa$ for all $u\in \mathcal {N}_{\varepsilon }$. Indeed, if $u\in \mathcal {N}_{\varepsilon }$, using $(g_2)$, $(f_1)$, $(f_2)$ and Theorem 2.1, we find

$$\begin{aligned} \Vert u\Vert _{V_{\varepsilon },p}^{p}+\Vert u\Vert ^{q}_{V_{\varepsilon },q}&= \int _{\mathbb {R}^{N}} g(\varepsilon x, u)\, u \, dx\\&\le \zeta |u|_{p}^{p}+C_{\zeta }|u|_{q^{*}_{s}}^{q^{*}_{s}}\\&\le \frac{\zeta }{V_{0}}\Vert u\Vert _{V_{\varepsilon },p}^{p}+C'_{\zeta } \Vert u\Vert ^{q^{*}_{s}}_{V_{\varepsilon },q}. \end{aligned}$$

Choosing $\zeta \in (0, V_{0})$ we obtain $\Vert u\Vert _{V_{\varepsilon },q}\ge \kappa =(C'_{\zeta })^{-\frac{1}{q^{*}_{s}-q}}$ which leads to $\Vert u\Vert _{\mathbb {X}_{\varepsilon }}\ge \Vert u\Vert _{V_{\varepsilon },q}\ge \kappa$.

Now we define the maps

$$\begin{aligned} {\hat{\psi }}_{\varepsilon }: \mathbb {X}_{\varepsilon }^{+} \rightarrow \mathbb {R}\quad \text{ and } \quad \psi _{\varepsilon }: \mathbb {S}_{\varepsilon }^{+}\rightarrow \mathbb {R}, \end{aligned}$$

by setting ${\hat{\psi }}_{\varepsilon }(u)= \mathcal {J}_{\varepsilon }({\hat{m}}_{\varepsilon }(u))$ and $\psi _{\varepsilon }={\hat{\psi }}_{\varepsilon }|_{\mathbb {S}_{\varepsilon }^{+}}$. From Lemma 3.2 and arguing as in the proofs of Proposition 9 and Corollary 10 in [34], we may obtain the following result.

Proposition 3.1

The following properties hold:

(a):

${\hat{\psi }}_{\varepsilon } \in C^{1}(\mathbb {X}_{\varepsilon }^{+}, \mathbb {R})$ and

$$\begin{aligned} \langle {\hat{\psi }}_{\varepsilon }'(u), v\rangle = \frac{\Vert {\hat{m}}_{\varepsilon }(u)\Vert _{\mathbb {X}_{\varepsilon }}}{\Vert u\Vert _{\mathbb {X}_{\varepsilon }}} \langle \mathcal {J}_{\varepsilon }'({\hat{m}}_{\varepsilon }(u)), v\rangle \quad \text{ for } \text{ all } u\in \mathbb {X}_{\varepsilon }^{+} \text{ and } v\in \mathbb {X}_{\varepsilon }. \end{aligned}$$

(b):

$\psi _{\varepsilon } \in C^{1}(\mathbb {S}_{\varepsilon }^{+}, \mathbb {R})$ and

$$\begin{aligned} \langle \psi _{\varepsilon }'(u), v \rangle = \Vert m_{\varepsilon }(u)\Vert _{\mathbb {X}_{\varepsilon }} \langle \mathcal {J}_{\varepsilon }'(m_{\varepsilon }(u)), v\rangle , \quad \text{ for } \text{ all } v\in T_{u}\mathbb {S}_{\varepsilon }^{+}. \end{aligned}$$

(c):

If $\{u_{n}\}_{n\in \mathbb {N}}$ is a $(PS)_{c}$ sequence for $\psi _{\varepsilon }$, then $\{m_{\varepsilon }(u_{n})\}_{n\in \mathbb {N}}$ is a $(PS)_{c}$ sequence for $\mathcal {J}_{\varepsilon }$. If $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathcal {N}_{\varepsilon }$ is a bounded $(PS)_{c}$ sequence for $\mathcal {J}_{\varepsilon }$, then $\{m_{\varepsilon }^{-1}(u_{n})\}_{n\in \mathbb {N}}$ is a $(PS)_{c}$ sequence for $\psi _{\varepsilon }$.

(d):

u is a critical point of $\psi _{\varepsilon }$ if, and only if, $m_{\varepsilon }(u)$ is a critical point for $\mathcal {J}_{\varepsilon }$. Moreover, the corresponding critical values coincide and

$$\begin{aligned} \inf _{u\in \mathbb {S}_{\varepsilon }^{+}} \psi _{\varepsilon }(u)= \inf _{u\in \mathcal {N}_{\varepsilon }} \mathcal {J}_{\varepsilon }(u). \end{aligned}$$

Remark 3.2

As in [34], we have the following variational characterization of the infimum of $\mathcal {J}_{\varepsilon }$ over $\mathcal {N}_{\varepsilon }$:

$$\begin{aligned} c_{\varepsilon }=\inf _{u\in \mathcal {N}_{\varepsilon }} \mathcal {J}_{\varepsilon }(u)=\inf _{u\in \mathbb {X}_{\varepsilon }^{+}} \max _{t>0} \mathcal {J}_{\varepsilon }(tu)=\inf _{u\in \mathbb {S}_{\varepsilon }^{+}} \max _{t>0} \mathcal {J}_{\varepsilon }(tu)>0. \end{aligned}$$

Moreover, if

$$\begin{aligned}&c'_{\varepsilon }=\inf _{\gamma \in \Gamma _{\varepsilon }} \max _{t\in [0, 1]} \mathcal {J}_{\varepsilon }(\gamma (t)) \quad \text{ where } \quad \Gamma _{\varepsilon }=\{\gamma \in C([0, 1], \mathbb {X}_{\varepsilon }): \gamma (0)=0 \\&\quad \text{ and } \mathcal {J}_{\varepsilon }(\gamma (1))<0\}, \end{aligned}$$

then we can argue as in [17, 33, 35] to verify that $c_{\varepsilon }=c'_{\varepsilon }$.

Next we establish a very useful upper bound for the minimax level $c_{\varepsilon }$. To accomplish this, we require that the parameter $\lambda >0$ appearing in $(f_{2})$ is sufficiently large.

Lemma 3.3

There exists $\lambda _{*}>1$ such that if $\lambda >\lambda ^{*}$ then $0<c_{\varepsilon }<\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}} \right) S_{q}^{\frac{N}{sq}}$.

Proof

For simplicity, we take $\varepsilon =1$. Define $V_{\infty }=\max _{\overline{\Lambda }} V$. Consider the following problem

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u + V_{\infty } (|u|^{p-2}u + |u|^{q-2}u)= (u^{+})^{\sigma _{1}-1} &{} \text{ in } \Lambda , \\ u=0 &{} \text{ in } \Lambda ^{c}. \end{array} \right. \end{aligned}$$

(3.8)

Let $\mathcal {J}_{\infty }: W^{s, p}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda )\rightarrow \mathbb {R}$, where $W^{s, t}_{0}(\Lambda )=\{u\in W^{s, t}(\mathbb {R}^{N}): u=0 \text{ in } \Lambda ^{c}\}$ with $t\in \{p, q\}$, given by

$$\begin{aligned} \mathcal {J}_{\infty }(u)=\frac{1}{p}[u]_{s, p}^{p}+\frac{1}{q}[u]_{s, q}^{q}+V_{\infty }\left( \frac{1}{p}|u|_{L^{p}(\Lambda )}^{p}+\frac{1}{q}|u|^{q}_{L^{q}(\Lambda )} \right) -\frac{1}{\sigma _{1}} |u^{+}|_{L^{\sigma _{1}}(\Lambda )}^{\sigma _{1}}. \end{aligned}$$

Define the Nehari manifold

$$\begin{aligned} \mathcal {N}_{\infty }=\{u\in (W^{s, p}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda ))\setminus \{0\}: \langle \mathcal {J}'_{\infty }(u), u\rangle =0 \}, \end{aligned}$$

and $c_{\infty }=\inf _{\mathcal {N}_{\infty }}\mathcal {J}_{\infty }$. Let us prove that there exists a non-negative function $w_{\sigma _{1}}\in (W^{s, p}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda ))\setminus \{0\}$ such that

(W1):

$\mathcal {J}_{\infty }(w_{\sigma _{1}})=c_{\infty }$, $\mathcal {J}'_{\infty }(w_{\sigma _{1}})=0$,

(W2):

$c_{\infty }\ge \left( \frac{\sigma _{1}-q}{\sigma _{1}q} \right) |w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}$.

We first show that, for all $u\in W^{s, p}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda )$ such that $|\mathrm{supp}(u^{+})\cap \Lambda |>0$, there exists $t_{u}>0$ such that $t_{u}u\in \mathcal {N}_{\infty }$. Since

$$\begin{aligned} \mathcal {J}_{\infty }(tu)&=t^{\sigma _{1}} \Bigg (\frac{t^{p-\sigma _{1}}}{p}[u]^{p}_{s, p}+\frac{t^{q-\sigma _{1}}}{q}[u]^{q}_{s, q}+\frac{t^{p-\sigma _{1}}}{p}|u|^{p}_{L^{p}(\Lambda )}+\frac{t^{q-\sigma _{1}}}{q}|u|^{q}_{L^{q}(\Lambda )}\\&\qquad -\frac{1}{\sigma _{1}}|u^{+}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}\Bigg ), \end{aligned}$$

and $1<p<q<\sigma _{1}<q^{*}_{s}$, we see that

$$\begin{aligned} \lim _{t\rightarrow 0} \frac{\mathcal {J}_{\infty }(tu)}{t^{\sigma _{1}}}=\infty , \quad \lim _{t\rightarrow \infty } \frac{\mathcal {J}_{\infty }(tu)}{t^{\sigma _{1}}}=-\frac{1}{\sigma _{1}} |u^{+}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}<0. \end{aligned}$$

Therefore, there exists $t_{u}>0$ such that $\mathcal {J}_{\infty }(t_{u}u)=\max _{t\ge 0} \mathcal {J}_{\infty }(t u)$ and $t_{u}u\in \mathcal {N}_{\infty }$. The uniqueness of a such $t_{u}$ is a consequence of the fact that the map $t\mapsto t^{\sigma _{1}-q}$ is increasing for $t>0$.

Next we prove that there exists $k_{1}>0$ such that $[u]_{s, q}\ge k_{1}>0$ for all $u\in \mathcal {N}_{\infty }$. Recalling that $W^{s, q}_{0}(\Lambda )\subset L^{\sigma _{1}}(\Lambda )$ (see [11, 18]), we have that, for some $C>0$ depending only on $N, s, q, \sigma _{1}$ and $|\Lambda |$,

$$\begin{aligned}{}[u]_{s, q}^{q}&\le [u]_{s, p}^{p}+[u]_{s, q}^{q}+V_{\infty }(|u|_{L^{p}(\Lambda )}^{p}+ |u|_{L^{q}(\Lambda )}^{q}) \nonumber \\&=|u^{+}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}\le C[u]^{\sigma _{1}}_{s, q}, \end{aligned}$$

(3.9)

and using the fact that $\sigma _{1}>q$ we get the desired estimate. In a similar way,

$$\begin{aligned}{}[u]_{s,p}^{p}\le |u^{+}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}\le C[u]^{\sigma _{1}}_{s, p}, \end{aligned}$$

and then we can find $k'_{1}>0$ such that $[u]_{s, p}\ge k'_{1}>0$ for all $u\in \mathcal {N}_{\infty }$. We can also see that, for some $k_{2}>0$, $\mathcal {J}_{\infty }(u)\ge k_{2}[u]_{s, q}^{q}$ for all $u\in \mathcal {N}_{\infty }$. Indeed,

$$\begin{aligned} \mathcal {J}_{\infty }(u)&=\mathcal {J}_{\infty }(u)-\frac{1}{\sigma _{1}}\langle \mathcal {J}_{\infty }'(u), u\rangle \\&\ge \left( \frac{1}{p}-\frac{1}{\sigma _{1}}\right) ([u]^{p}_{s, p}+V_{\infty }|u|^{p}_{L^{p}(\Lambda )})+\left( \frac{1}{q}-\frac{1}{\sigma _{1}}\right) ([u]^{q}_{s, q}+V_{\infty }|u|^{q}_{L^{q}(\Lambda )})\\&\ge \left( \frac{1}{q}-\frac{1}{\sigma _{1}}\right) [u]^{q}_{s, q}\ge \left( \frac{1}{q}-\frac{1}{\sigma _{1}}\right) k^{q}_{1}. \end{aligned}$$

Let us now $\{u_{n}\}_{n\in \mathbb {N}}$ be a minimizing sequence for $\mathcal {J}_{\infty }$ in $\mathcal {N}_{\infty }$. Note that the above discussion guarantees that $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $W^{s, p}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda )$. Then there exists $u\in W^{s, q}_{0}(\Lambda )\cap W^{s, q}_{0}(\Lambda )$ such that, up to a subsequence, $u_{n}\rightarrow u$ in $L^{r}(\mathbb {R}^{N})$ for all $r\in [1, q^{*}_{s})$ and $u_{n}\rightarrow u$ a.e. in $\mathbb {R}^{N}$. Observe that $|\mathrm{supp}(u^{+})\cap \Lambda |>0$. Indeed, by (3.9), we also see that $C'|u^{+}_{n}|^{q}_{L^{\sigma _{1}}(\Lambda )}\le [u_{n}]_{s, q}^{q}\le |u^{+}_{n}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}$ for all $n\in \mathbb {N}$, which combined with $u_{n}\rightarrow u$ in $L^{\sigma _{1}}(\Lambda )$ gives the required assertion. Then there exists $t_{u}>0$ such that $t_{u}u\in \mathcal {N}_{\infty }$. Consequently,

$$\begin{aligned} c_{\infty }\le \mathcal {J}_{\infty }(t_{u}u)\le \liminf _{n\rightarrow \infty } \mathcal {J}_{\infty }(t_{u} u_{n})\le \liminf _{n\rightarrow \infty } \mathcal {J}_{\infty }(u_{n})=c_{\infty }. \end{aligned}$$

Taking $w_{\sigma _{1}}=t_{u}u$ we have that $\mathcal {J}_{\infty }(w_{\sigma _{1}})=c_{\infty }$. Applying the implicit function theorem we deduce that $\mathcal {J}'_{\infty }(w_{\sigma _{1}})=0$. Therefore, $\mathcal {J}_{\infty }(w_{\sigma _{1}})=\inf _{\mathcal {N}_{\infty }}\mathcal {J}_{\infty }$ and (W1) is true. The relation (W2) is a simple consequence of the fact that $w_{\sigma _{1}}\in \mathcal {N}_{\infty }$ and $p<q<\sigma _{1}$ yield

$$\begin{aligned} c_{\infty }&=\mathcal {J}_{\infty }(w_{\sigma _{1}})-\frac{1}{\sigma _{1}}\langle \mathcal {J}_{\infty }'(u), u\rangle \\&\ge \left( \frac{1}{q}-\frac{1}{\sigma _{1}}\right) ([w_{\sigma _{1}}]_{s, p}^{p}+V_{\infty }|w_{\sigma _{1}}|^{p}_{L^{p}(\Lambda )}+[w_{\sigma _{1}}]_{s, q}^{q}+V_{\infty }|w_{\sigma _{1}}|^{q}_{L^{q}(\Lambda )}) \\&=\left( \frac{1}{q}-\frac{1}{\sigma _{1}}\right) |w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}. \end{aligned}$$

As $\langle \mathcal {J}'_{\infty }(w_{\sigma _{1}}), w^{-}_{\sigma _{1}}\rangle =0$, where $w^{-}_{\sigma _{1}}=\min \{w_{\sigma _{1}}, 0\}$, and $w_{\sigma _{1}}=0$ in $\Lambda ^{c}$, we can see that $w^{-}_{\sigma _{1}}=0$, that is $w_{\sigma _{1}}\ge 0$ in $\mathbb {R}^{N}$.

Now, we note that, by $(f_{2})$, $w_{\sigma _{1}}=0$ in $\Lambda ^{c}$ and (W1), it holds

$$\begin{aligned} \Vert w_{\sigma _{1}}\Vert _{V_{\varepsilon }, p}^{p}+\Vert w_{\sigma _{1}}\Vert _{V_{\varepsilon }, q}^{q}&\le [w_{\sigma _{1}}]_{s, p}^{p}+[w_{\sigma _{1}}]_{s, q}^{q}+V_{\infty }(|w_{\sigma _{1}}|_{L^{p}(\Lambda )}^{p}+|w_{\sigma _{1}}|_{L^{q}(\Lambda )}^{q})\\&=|w_{\sigma _{1}}|_{L^{\sigma _{1}}(\Lambda )}^{\sigma _{1}}\le \int _{\mathbb {R}^{N}} f(w_{\sigma _{1}})w_{\sigma _{1}}\, dx\le \int _{\mathbb {R}^{N}} g(\varepsilon x, w_{\sigma _{1}})w_{\sigma _{1}}\, dx. \end{aligned}$$

Therefore, $\langle \mathcal {J}'_{\varepsilon }(w_{\sigma _{1}}), w_{\sigma _{1}}\rangle \le 0$ and there exists $t\in (0, 1)$ such that $tw_{\sigma _{1}}\in \mathcal {N}_{\varepsilon }$. Hence, by $(f_2)$,

$$\begin{aligned} c_{\varepsilon }&\le \mathcal {J}_{\varepsilon }(tw_{\sigma _{1}})\le \frac{t^{p}}{p}[w_{\sigma _{1}}]^{p}_{s, p}+\frac{t^{q}}{q}[w_{\sigma _{1}}]^{q}_{s, q}+\frac{t^{p}}{p}V_{\infty }|w_{\sigma _{1}}|^{p}_{L^{p}(\Lambda )}+\frac{t^{q}}{q}V_{\infty }|w_{\sigma _{1}}|^{q}_{L^{q}(\Lambda )}\\&\quad -\frac{\lambda }{\sigma _{1}}t^{\sigma _{1}}|w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}. \end{aligned}$$

Since $t\in (0, 1)$, $p<q$, and $\langle \mathcal {J}'_{\infty }(w_{\sigma _{1}}), w_{\sigma _{1}}\rangle = 0$, we find

$$\begin{aligned} c_{\varepsilon }&\le \frac{t^{p}}{p}([w_{\sigma _{1}}]^{p}_{s, p}+[w_{\sigma _{1}}]^{q}_{s, q})+\frac{t^{p}}{p}V_{\infty }(|w_{\sigma _{1}}|^{p}_{L^{p}(\Lambda )}+|w_{\sigma _{1}}|^{q}_{L^{q}(\Lambda )})-\frac{\lambda }{\sigma _{1}}t^{\sigma _{1}}|w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}\\&=\left( \frac{t^{p}}{p} -\lambda \frac{t^{\sigma _{1}}}{\sigma _{1}}\right) |w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}\\&\le \max _{\tau \ge 0} \left( \frac{\tau ^{p}}{p} -\lambda \frac{\tau ^{\sigma _{1}}}{\sigma _{1}}\right) |w_{\sigma _{1}}|^{\sigma _{1}}_{L^{\sigma _{1}}(\Lambda )}. \end{aligned}$$

Using (W2) and the fact that

$$\begin{aligned} \max _{\tau \ge 0} \left( \frac{\tau ^{p}}{p} -\lambda \frac{\tau ^{\sigma _{1}}}{\sigma _{1}}\right) =\left( \frac{\sigma _{1}-p}{\sigma _{1}p}\right) \frac{1}{\lambda ^{\frac{p}{\sigma _{1}-p}}}, \end{aligned}$$

we arrive at

$$\begin{aligned} c_{\varepsilon }\le \left( \frac{\sigma _{1}-p}{p}\right) \frac{1}{\lambda ^{\frac{p}{\sigma _{1}-p}}} \frac{c_{\infty }q}{\sigma _{1}-q}. \end{aligned}$$

Set

$$\begin{aligned} \lambda ^{*}=\max \left\{ 1, \left[ \frac{q^{*}_{s}\vartheta }{q^{*}_{s}-\vartheta }S^{-\frac{N}{sq}} \left( \frac{\sigma _{1}-p}{\sigma _{1}-q}\right) \frac{c_{\infty } q}{p}\right] ^{\frac{\sigma _{1}-p}{p}} \right\} . \end{aligned}$$

Taking $\lambda >\lambda ^{*}$ in the hypothesis $(f_{2})$ we get the thesis. $\square$

The main feature of the modified functional is that it satisfies a compactness condition. We start by proving the boundedness of Palais-Smale sequences.

Lemma 3.4

Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {X}_{\varepsilon }$ be a $(PS)_{c}$ sequence for $\mathcal {J}_{\varepsilon }$. Then $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {X}_{\varepsilon }$.

Proof

In light of $(g_{3})$ and $\vartheta>q>p$, we see that

$$\begin{aligned} C(1+\Vert u_{n}\Vert _{\varepsilon })&\ge \mathcal {J}_{\varepsilon }(u_{n})- \frac{1}{\vartheta } \langle \mathcal {J}'_{\varepsilon }(u_{n}), u_{n}\rangle \\&=\left( \frac{1}{p}- \frac{1}{\vartheta }\right) \Vert u_{n}\Vert _{V_{\varepsilon },p}^{p} + \left( \frac{1}{q}- \frac{1}{\vartheta }\right) \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q} + \frac{1}{\vartheta } \int _{\Lambda _{\varepsilon }^{c}} \\&\qquad \left( g(\varepsilon x, u_{n})u_{n}- \vartheta G(\varepsilon x, u_{n})\right) \, dx+ \frac{1}{\vartheta } \int _{\Lambda _{\varepsilon }} \Big (g(\varepsilon x, u_{n})u_{n}\\&\qquad - \vartheta G(\varepsilon x, u_{n})\Big ) \, dx \\&\ge \left( \frac{1}{q}- \frac{1}{\vartheta }\right) \left( \Vert u_{n}\Vert _{V_{\varepsilon },p}^{p} + \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q}\right) -\left( \frac{1}{q}- \frac{1}{\vartheta }\right) \frac{1}{K} \int _{\Lambda _{\varepsilon }^{c}} \\&\quad V(\varepsilon x) (|u_{n}|^{p}+|u_{n}|^{q})\, dx\\&\ge \left( \frac{1}{q}- \frac{1}{\vartheta }\right) \left( 1- \frac{1}{K} \right) (\Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}+\Vert u_{n}\Vert _{V_{\varepsilon },q}^{q})\\&= {\tilde{C}} (\Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}+\Vert u_{n}\Vert _{V_{\varepsilon },q}^{q}), \end{aligned}$$

where ${\tilde{C}}>0$ since $K>1$ and $\vartheta >q$. Now, assume by contradiction that $\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}\rightarrow \infty$. We distinguish the following cases:

(1):

$\Vert u_{n}\Vert _{V_{\varepsilon },p}\rightarrow \infty$ and $\Vert u_{n}\Vert _{V_{\varepsilon }, q}\rightarrow \infty$;

(2):

$\Vert u_{n}\Vert _{V_{\varepsilon },p}\rightarrow \infty$ and $\Vert u_{n}\Vert _{V_{\varepsilon }, q}$ is bounded;

(3):

$\Vert u_{n}\Vert _{V_{\varepsilon },q}\rightarrow \infty$ and $\Vert u_{n}\Vert _{V_{\varepsilon }, p}$ is bounded.

In case (1), for n large, we have $\Vert u_{n}\Vert _{V_{\varepsilon }, q}^{q-p}\ge 1$, that is $\Vert u_{n}\Vert _{V_{\varepsilon }, q}^{q}\ge \Vert u_{n}\Vert _{V_{\varepsilon }, q}^{p}$. Therefore,

$$\begin{aligned} C_{0}(1+ \Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}) \ge {\tilde{C}} (\Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}+\Vert u_{n}\Vert _{V_{\varepsilon }, q}^{p})\ge C_{1} (\Vert u_{n}\Vert _{V_{\varepsilon },p}+\Vert u_{n}\Vert _{V_{\varepsilon },q})^{p}=C_{1}\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }}^{p} \end{aligned}$$

which gives a contradiction. In case (2), we have

$$\begin{aligned} C_{0}(1+\Vert u_{n}\Vert _{V_{\varepsilon },p}+\Vert u_{n}\Vert _{V_{\varepsilon },q})=C_{0}(1+\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon }})\ge {\tilde{C}} \Vert u_{n}\Vert _{V_{\varepsilon },p}^{p} \end{aligned}$$

and thus

$$\begin{aligned} C_{0}\left( \frac{1}{\Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}}+\frac{1}{\Vert u_{n}\Vert _{V_{\varepsilon },p}^{p-1}}+\frac{\Vert u_{n}\Vert _{V_{\varepsilon },q}}{\Vert u_{n}\Vert ^{p}_{V_{\varepsilon },p}}\right) \ge {\tilde{C}}. \end{aligned}$$

Since $p>1$ and letting $n\rightarrow \infty$, we obtain $0< {\tilde{C}}\le 0$ which is impossible. The last case is similar to the case (2), so we skip the details. In conclusion, $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {X}_{\varepsilon }$. $\square$

Remark 3.3

We may always assume that any $(PS)_{c}$ sequence $\{u_{n}\}_{n\in \mathbb {N}}$ of $\mathcal {J}_{\varepsilon }$ is nonnegative. Indeed, by using $\langle \mathcal {J}'_{\varepsilon }(u_{n}), u_{n}^{-}\rangle =o_{n}(1)$, where $u_{n}^{-}=\min \{u_{n}, 0\}$, and $g(x, t)=0$ for $t\le 0$, we have that

$$\begin{aligned} \Vert u_{n}^{-}\Vert _{V_{\varepsilon }, p}^{p}+\Vert u_{n}^{-}\Vert _{V_{\varepsilon }, q}^{q}= o_{n}(1), \end{aligned}$$

that is $u_{n}^{-}\rightarrow 0$ in $\mathbb {X}_{\varepsilon }$. Moreover, $\{u_{n}^{+}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {X}_{\varepsilon y}$. Clearly, $\Vert u_{n}\Vert _{V_{\varepsilon }, t}=\Vert u_{n}^{+}\Vert _{V_{\varepsilon }, t}+o_{n}(1)$ for $t\in \{p, q\}$. Thus, we can easily check that $\mathcal {J}_{\varepsilon }(u_{n})=\mathcal {J}_{\varepsilon }(u^{+}_{n})+o_{n}(1)$ and $\mathcal {J}'_{\varepsilon }(u_{n})=\mathcal {J}'_{\varepsilon }(u^{+}_{n})+o_{n}(1)$. Consequently, $\mathcal {J}_{\varepsilon }(u^{+}_{n})\rightarrow c$ and $\mathcal {J}'_{\varepsilon }(u^{+}_{n})=o_{n}(1)$.

Lemma 3.5

$\mathcal {J}_{\varepsilon }$ satisfies the $(PS)_{c}$ condition at any level $c<\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_*^{\frac{N}{sq}}$.

Proof

Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {X}_{\varepsilon }$ be a $(PS)_{c}$ sequence for $\mathcal {J}_{\varepsilon }$. From Lemma 3.4, going to a subsequence if necessary, we may assume that $u_{n}\rightharpoonup u$ in $\mathbb {X}_{\varepsilon }$ and $u_{n}\rightarrow u$ in $L^{r}_{loc}(\mathbb {R}^{N})$ for all $r\in [1, q^{*})$. It is standard to verify that the weak limit u is a critical point of $\mathcal {J}_{\varepsilon }$. Indeed, taking into account that for all $\phi \in C^{\infty }_{c}(\mathbb {R}^{N})$

$$\begin{aligned}&\langle u_{n}, \varphi \rangle _{s, t}\rightarrow \langle u, \varphi \rangle _{s, t}, \quad \text{ for } t\in \{p, q\}, \\&\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{p-2}u_{n}\phi \, dx\rightarrow \int _{\mathbb {R}^{N}} V(\varepsilon x) |u|^{p-2}u\phi \, dx, \\&\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{q-2}u_{n}\phi \, dx\rightarrow \int _{\mathbb {R}^{N}} V(\varepsilon x) |u|^{q-2}u\phi \, dx,\\&\int _{\mathbb {R}^{N}} g(\varepsilon x, u_{n})\phi \,dx\rightarrow \int _{\mathbb {R}^{N}} g(\varepsilon x, u)\phi \,dx, \end{aligned}$$

and that $\langle \mathcal {J}_{\varepsilon }'(u_{n}),\phi \rangle =o_{n}(1)$, we see that $\langle \mathcal {J}'_{\varepsilon }(u),\phi \rangle =0$ for any $\phi \in C^{\infty }_{c}(\mathbb {R}^{N})$. By the density of $C^{\infty }_{c}(\mathbb {R}^{N})$ in $\mathbb {X}_{\varepsilon }$, we obtain that u is a critical point of $\mathcal {J}_{\varepsilon }$. In particular, $\langle \mathcal {J}'_{\varepsilon }(u), u\rangle =0$.

Now, we claim that for any $\eta >0$ there exists $R=R(\eta )>0$ such that

$$\begin{aligned}&\limsup _{n\rightarrow \infty } \int _{B_{R}^{c}(0)} \Bigg (\int _{\mathbb {R}^{N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}}+\frac{|u_{n}(x)- u_{n}(y)|^{q}}{|x-y|^{N+sq}} \,dy \nonumber \\&\qquad + V(\varepsilon x) (|u_{n}|^{p}+|u_{n}|^{q}) \Bigg ) \, dx <\eta . \end{aligned}$$

(3.10)

For $R>0$, let $\psi _{R}\in C^{\infty }(\mathbb {R}^{N})$ be such that $0\le \psi _{R}\le 1$, $\psi _{R}=0$ in $B_{\frac{R}{2}}(0)$, $\psi _{R}=1$ in $B^{c}_{R}(0)$, and $|\nabla \psi _{R}|\le \frac{C}{R}$, for some constant $C>0$ independent of R. Since $\{\psi _{R}u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {X}_{\varepsilon }$, it follows that $\langle \mathcal {J}_{\varepsilon }'(u_{n}), \psi _{R}u_{n}\rangle =o_{n}(1)$, namely

$$\begin{aligned} \begin{aligned}&\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}}\psi _{R}(x)\, dxdy + \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{q}}{|x-y|^{N+sq}}\psi _{R}(x)\, dxdy \\&+\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{p} \psi _{R} \, dx+\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{q} \psi _{R} \, dx\\&=o_{n}(1) + \int _{\mathbb {R}^{N}} g(\varepsilon x, u_{n}) \psi _{R} u_{n}\, dx \\&\quad - \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{p-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+sp}}u_{n}(y)\, dxdy\\&\quad -\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{q-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+sq}}u_{n}(y)\, dxdy. \end{aligned}\end{aligned}$$

(3.11)

Take $R>0$ such that $\Lambda _{\varepsilon }\subset B_{\frac{R}{2}}(0)$. By the definition of $\psi _{R}$ and $(g_{3})$-(ii), we have that

$$\begin{aligned} \begin{aligned}&\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}}\psi _{R}(x)\, dxdy + \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{q}}{|x-y|^{N+sq}}\psi _{R}(x)\, dxdy \\&\quad + \left( 1- \frac{1}{K}\right) \int _{\mathbb {R}^{N}} V(\varepsilon x) (|u_{n}|^{p}+|u_{n}|^{q}) \psi _{R} \, dx \\&\le o_{n}(1) - \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{p-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+sp}}u_{n}(y)\, dxdy \\&\quad -\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{q-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+sq}}u_{n}(y)\, dxdy. \end{aligned}\end{aligned}$$

(3.12)

Now, from the Hölder inequality and the boundedness of $\{u_{n}\}_{n\in \mathbb {N}}$ in $\mathbb {X}_{\varepsilon }$, we derive, for $t\in \{p, q\}$,

$$\begin{aligned}&\left| \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{t-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+st}}u_{n}(y)\, dxdy\right| \nonumber \\&\qquad \le C \left( \iint _{\mathbb {R}^{2N}} \frac{|\psi _{R}(x)- \psi _{R}(y)|^{t}}{|x-y|^{N+st}}|u_{n}(y)|^{t}\, dxdy\right) ^{\frac{1}{t}}. \end{aligned}$$

(3.13)

Using the definition of $\psi _{R}$, polar coordinates and the boundedness of $\{u_{n}\}_{n\in \mathbb {N}}$ in $\mathbb {X}_{\varepsilon }$, we can see that

$$\begin{aligned} \begin{aligned}&\iint _{\mathbb {R}^{2N}} \frac{|\psi _{R}(x)-\psi _{R}(y)|^{t}}{|x-y|^{N+st}}|u_{n}(x)|^{t} dx dy \\&=\int _{\mathbb {R}^{N}} \int _{|y-x|>R} \frac{|\psi _{R}(x)-\psi _{R}(y)|^{t}}{|x-y|^{N+st}}|u_{n}(x)|^{t} dx dy \\&\qquad +\int _{\mathbb {R}^{N}} \int _{|y-x|\le R} \frac{|\psi _{R}(x)-\psi _{R}(y)|^{t}}{|x-y|^{N+st}}|u_{n}(x)|^{t} dx dy \\&\le C \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} \left( \int _{|y-x|>R} \frac{dy}{|x-y|^{N+st}}\right) dx \\&\qquad + \frac{C}{R^{t}} \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} \left( \int _{|y-x|\le R} \frac{dy}{|x-y|^{N+st-t}}\right) dx \\&\le C \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} \left( \int _{|z|>R} \frac{dz}{|z|^{N+st}}\right) dx + \frac{C}{R^{t}} \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} \left( \int _{|z|\le R} \frac{dz}{|z|^{N+st-t}}\right) dx \\&\le C \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} dx \left( \int _{R}^{\infty } \frac{d\rho }{\rho ^{st+1}}\right) + \frac{C}{R^{t}} \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} dx \left( \int _{0}^{R} \frac{d\rho }{\rho ^{st-t+1}}\right) \\&\le \frac{C}{R^{st}} \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} dx+\frac{C}{R^{t}} R^{-st+t}\int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} dx \\&\le \frac{C}{R^{st}} \int _{\mathbb {R}^{N}} |u_{n}(x)|^{t} dx\le \frac{C}{R^{st}}. \end{aligned}\end{aligned}$$

(3.14)

In light of (3.14) and (3.13), we find

$$\begin{aligned} \left| \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{t-2} (u_{n}(x)- u_{n}(y)) (\psi _{R}(x)- \psi _{R}(y))}{|x-y|^{N+st}}u_{n}(y)\, dxdy\right| \le \frac{C}{R^{s}}. \end{aligned}$$

(3.15)

Combining (3.12), (3.13) and (3.15), we get

$$\begin{aligned}&\int _{B^{c}_{R}(0)}\int _{\mathbb {R}^{N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}}\, dxdy + \int _{B^{c}_{R}(0)}\int _{\mathbb {R}^{N}} \frac{|u_{n}(x)- u_{n}(y)|^{q}}{|x-y|^{N+sq}}\, dxdy \nonumber \\&\quad + \left( 1- \frac{1}{K}\right) \int _{B_{R}^{c}(0)} V(\varepsilon x) (|u_{n}|^{p}+|u_{n}|^{q}) \, dx \nonumber \\&\le \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}}\psi _{R}(x)\, dxdy +\iint _{\mathbb {R}^{N}} \frac{|u_{n}(x)- u_{n}(y)|^{q}}{|x-y|^{N+sq}}\psi _{R}(x)\, dxdy \nonumber \\&\quad + \left( 1- \frac{1}{K}\right) \int _{\mathbb {R}^{N}} V(\varepsilon x) (|u_{n}|^{p}+|u_{n}|^{q}) \, dx \nonumber \\&\le o_{n}(1)+C \left( \iint _{\mathbb {R}^{2N}} \frac{|\psi _{R}(x)- \psi _{R}(y)|^{p}}{|x-y|^{N+sp}}|u_{n}(y)|^{p}\, dxdy\right) ^{\frac{1}{p}}\nonumber \\&\quad +C\left( \iint _{\mathbb {R}^{2N}} \frac{|\psi _{R}(x)- \psi _{R}(y)|^{q}}{|x-y|^{N+sq}}|u_{n}(y)|^{q}\, dxdy\right) ^{\frac{1}{q}}\nonumber \\&\le o_{n}(1)+\frac{C}{R^{s}}, \end{aligned}$$

(3.16)

from which we deduce that (3.10) holds. Next we show that (3.10) is useful to infer that $u_{n}\rightarrow u$ in $L^{r}(\mathbb {R}^{N})$ for any $r \in [p, q^{*}_{s})$. Fixed $\eta >0$ we can find $R= R(\eta )>0$ such that (3.10) is valid. Recalling the compact embedding $\mathbb {X}_{\varepsilon }\Subset L^{p}_{loc}(\mathbb {R}^{N})$ in Theorem 2.1, we deduce that

$$\begin{aligned} \limsup _{n\rightarrow \infty }|u_{n}-u|^{p}_{p}&= \limsup _{n\rightarrow \infty }[|u_{n}-u|^{p}_{L^{p}(B_{R}(0))}+ |u_{n}-u|^{p}_{L^{p}(B_{R}^{c}(0))}] \\&=\lim _{n\rightarrow \infty }|u_{n}-u|^{p}_{L^{p}(B_{R}(0))} + \limsup _{n\rightarrow \infty }|u_{n}-u|^{p}_{L^{p}(B_{R}^{c}(0))}\\&\le 2^{p-1} \limsup _{n\rightarrow \infty }(|u_{n}|^{p}_{L^{p}(B_{R}^{c}(0))}+|u|^{p}_{L^{p}(B_{R}^{c}(0))})\\&\le \frac{2^{p-1}}{V_{0}} \left[ \limsup _{n\rightarrow \infty } \int _{B_{R}^{c}(0)} \left( \int _{\mathbb {R}^{N}} \frac{|u_{n}(x)- u_{n}(y)|^{p}}{|x-y|^{N+sp}} \,dy + V(\varepsilon x) |u_{n}|^{p}\right) \, dx \right. \\&\quad \quad \left. +\int _{B_{R}^{c}(0)} \left( \int _{\mathbb {R}^{N}} \frac{|u(x)- u(y)|^{p}}{|x-y|^{N+sp}} \,dy + V(\varepsilon x) |u|^{p}\right) \, dx\right] \\&< \frac{2^{p}}{V_{0}}\eta =\kappa \eta . \end{aligned}$$

The arbitrariness of $\eta$ implies the strong convergence in $L^{p}$-norm. By interpolation, we can see that $u_{n}\rightarrow u$ in $L^{r}(\mathbb {R}^{N})$ for any $r \in [p, q^{*}_{s})$. In order to establish the strong convergence in $\mathbb {X}_{\varepsilon }$, we prove that

$$\begin{aligned} \int _{\mathbb {R}^{N}} g(\varepsilon x, u_{n}) u_{n}\, dx \rightarrow \int _{\mathbb {R}^{N}} g(\varepsilon x, u) u\, dx. \end{aligned}$$

(3.17)

Note that the Sobolev inequality in Theorem 2.1, $0\le \psi _{R}\le 1$, (3.16) and (3.14) yield

$$\begin{aligned} |u_{n}|^{q}_{L^{q^{*}_{s}}(B^{c}_{R}(0))}&\le |u_{n}\psi _{R}|_{q^{*}_{s}}^{q}\\&\le C[u_{n}\psi _{R}]_{s, q}^{q}\\&\le C \Bigg [\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{q}}{|x-y|^{N+sq}} |\psi _{R}(x)|^{q}\,dxdy\\&\qquad +\iint _{\mathbb {R}^{2N}} \frac{|\psi _{R}(x)-\psi _{R}(y)|^{q}}{|x-y|^{N+sq}} |u_{n}(y)|^{q}\,dxdy \Bigg ]\\&\le C\left[ \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{q}}{|x-y|^{N+sq}} \psi _{R}(x)\,dxdy+\frac{C}{R^{sq}} \right] \\&\le o_{n}(1)+\frac{C}{R^{s}}+\frac{C}{R^{sq}}. \end{aligned}$$

Therefore,

$$\begin{aligned} \lim _{R\rightarrow \infty }\limsup _{n\rightarrow \infty } |u_{n}|^{q}_{L^{q^{*}_{s}}(B^{c}_{R}(0))}=0. \end{aligned}$$

(3.18)

Clearly, the strong convergence in $L^{r}(\mathbb {R}^{N})$ for all $r\in [p, q^{*}_{s})$ gives

$$\begin{aligned} \lim _{R\rightarrow \infty }\limsup _{n\rightarrow \infty } |u_{n}|^{r}_{L^{r}(B^{c}_{R}(0))}=0. \end{aligned}$$

(3.19)

Then, using the growth assumption on g, (3.18) and (3.19), for all $\eta >0$ there exists $R=R(\eta )>0$ such that

$$\begin{aligned} \limsup _{n\rightarrow \infty } \int _{B^{c}_{R}(0)} g(\varepsilon x, u_{n})u_{n}\, dx \le C\limsup _{n\rightarrow \infty } \int _{B^{c}_{R}(0)}(|u_{n}|^{p}+|u_{n}|^{\sigma _{2}}+|u_{n}|^{q^{*}_{s}}) \, dx \le C\eta . \end{aligned}$$

(3.20)

On the other hand, choosing $R>0$ large enough, we may assume that

$$\begin{aligned} \int _{B^{c}_{R}(0)} g(\varepsilon x, u)u\, dx< \eta . \end{aligned}$$

(3.21)

Hence, (3.20) and (3.21) yield

$$\begin{aligned} \limsup _{n\rightarrow \infty }\left| \int _{B^{c}_{R}(0)} g(\varepsilon x, u_{n})u_{n}\, dx- \int _{B^{c}_{R}(0)} g(\varepsilon x, u)u\, dx\right| < C\eta \quad \text{ for } \text{ all } \eta >0, \end{aligned}$$

which leads to

$$\begin{aligned} \lim _{n\rightarrow \infty }\int _{B^{c}_{R}(0)} g(\varepsilon x, u_{n})u_{n}\, dx=\int _{B^{c}_{R}(0)} g(\varepsilon x, u)u\, dx. \end{aligned}$$

(3.22)

It follows from the definition of g that

$$\begin{aligned} g(\varepsilon x, u_{n})u_{n}\le f(u_{n})u_{n}+a^{q^{*}_{s}}+\frac{V_{0}}{K} |u_{n}|^{q} \, \text{ for } \text{ any } x\in \Lambda ^{c}_{\varepsilon }. \end{aligned}$$

Since $B_{R}(0)\cap \Lambda _{\varepsilon }^{c}$ is bounded, we can use the above estimate, $(f_1)$, $(f_2)$, Theorem 2.1 and the dominated convergence theorem to infer that

$$\begin{aligned} \lim _{n\rightarrow \infty }\int _{B_{R}(0)\cap \Lambda _{\varepsilon }^{c}} g(\varepsilon x, u_{n})u_{n}\, dx=\int _{B_{R}(0)\cap \Lambda _{\varepsilon }^{c}} g(\varepsilon x, u)u \, dx. \end{aligned}$$

(3.23)

At this point, we aim to show that

$$\begin{aligned} \lim _{n\rightarrow \infty }\int _{\Lambda _{\varepsilon }} |u_{n}|^{q^{*}_{s}} \,dx=\int _{\Lambda _{\varepsilon }} |u|^{q^{*}_{s}} \,dx. \end{aligned}$$

(3.24)

Indeed, if we assume that (3.24) is true, from $(g_2)$, $(f_{1})$, $(f_{2})$, Theorem 2.1 and the dominated convergence theorem, we deduce that

$$\begin{aligned} \lim _{n\rightarrow \infty }\int _{\Lambda _{\varepsilon }\cap B_{R}(0)} g(\varepsilon x, u_{n})u_{n}\, dx= \int _{\Lambda _{\varepsilon }\cap B_{R}(0)} g(\varepsilon x, u)u\, dx. \end{aligned}$$

(3.25)

Putting together (3.22), (3.23) and (3.25), we infer that (3.17) holds. It remains to prove that (3.24) is valid. First, we may assume that

$$\begin{aligned} |D^{s} u_{n}|^{q}\rightharpoonup \mu , \, |u_{n}|^{q^{*}_{s}} \rightharpoonup \nu \end{aligned}$$

(3.26)

weakly in the sense of measures. From Lemma 2.2, we have an at most countable index set I, sequences $\{x_{i}\}_{i\in I}\subset \mathbb {R}^{N}$, $\{\mu _{i}\}_{i\in I}$, $\{\nu _{i}\}_{i\in I}$ in $(0, \infty )$ such that

$$\begin{aligned} \mu \ge |D^{s} u|^{q}+\sum _{i\in I} \mu _{i}\delta _{x_{i}}, \quad \nu =|u|^{q^{*}_{s}}+\sum _{i\in I} \nu _{i}\delta _{x_{i}}, \quad S_{q} \nu _{i}^{\frac{q}{q^{*}_{s}}}\le \mu _{i} \quad \text{ for } \text{ all } i\in I. \end{aligned}$$

(3.27)

It suffices to prove that $\{x_{i}\}_{i\in I}\cap \Lambda _{\varepsilon }=\emptyset$. Assume, by contradiction, that $x_{i}\in \Lambda _{\varepsilon }$ for some $i\in I$. For $\rho >0$, define $\zeta _{\rho }(x)=\zeta (\frac{x-x_{i}}{\rho })$ where $\zeta \in C^{\infty }_{c}(\mathbb {R}^{N})$ is such that $0\le \zeta \le 1$, $\zeta =1$ in $B_{1}(0)$, $\zeta =0$ in $B^{c}_{2}(0)$ and $|\nabla \zeta |_{\infty }\le 2$. We suppose that $\rho$ is chosen in such way that the support of $\zeta _{\rho }$ is contained in $\Lambda _{\varepsilon }$. Since $\{\zeta _{\rho } u_{n}\}_{n\in \mathbb {N}}$ is bounded, $\langle \mathcal {J}'_{\varepsilon }(u_{n}), u_{n}\zeta _{\rho }\rangle =o_{n}(1)$ and thus

$$\begin{aligned}&\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{q}}{|x-y|^{N+sq}} \zeta _{\rho }(x)\, dxdy\nonumber \\&\le \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{p}}{|x-y|^{N+sp}} \zeta _{\rho }(x)\, dxdy+\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{q}}{|x-y|^{N+sq}} \zeta _{\rho }(x)\, dxdy\nonumber \\&\quad +\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{p} \zeta _{\rho } \, dx+\int _{\mathbb {R}^{N}} V(\varepsilon x) |u_{n}|^{q} \zeta _{\rho } \, dx\nonumber \\&=o_{n}(1)-\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{p-2}}{|x-y|^{N+sp}}(u_{n}(x)-u_{n}(y)) (\zeta _{\rho }(x)-\zeta _{\rho }(y))u_{n}(y)\, dxdy\nonumber \\&\quad -\iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{q-2}}{|x-y|^{N+sq}} (u_{n}(x)-u_{n}(y))(\zeta _{\rho }(x)-\zeta _{\rho }(y))u_{n}(y)\, dxdy\nonumber \\&\quad + \int _{\mathbb {R}^{N}} f(u_{n}) \zeta _{\rho } u_{n}\, dx+ \int _{\mathbb {R}^{N}} |u_{n}|^{q^{*}_{s}} \zeta _{\rho } \, dx. \end{aligned}$$

(3.28)

Since f has subcritical growth and $\zeta _{\rho }$ has compact support, we have

$$\begin{aligned} \lim _{\rho \rightarrow 0} \lim _{n\rightarrow \infty } \int _{\mathbb {R}^{N}} \zeta _{\rho } f(u_{n})u_{n}\, dx=\lim _{\rho \rightarrow 0} \int _{\mathbb {R}^{N}} \zeta _{\rho } f(u)u\, dx=0. \end{aligned}$$

(3.29)

Now, we verify that, for $t\in \{p, q\}$,

$$\begin{aligned} \lim _{\rho \rightarrow 0} \limsup _{n\rightarrow \infty } \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{t-2}}{|x-y|^{N+st}} (u_{n}(x)-u_{n}(y))(\zeta _{\rho }(x)-\zeta _{\rho }(y))u_{n}(y)\, dxdy=0. \end{aligned}$$

(3.30)

The Hölder inequality yields

$$\begin{aligned}&\left| \iint _{\mathbb {R}^{2N}} \frac{|u_{n}(x)-u_{n}(y)|^{t-2}}{|x-y|^{N+st}} (u_{n}(x)-u_{n}(y))(\zeta _{\rho }(x)-\zeta _{\rho }(y))u_{n}(y)\, dxdy \right| \\&\le [u_{n}]_{s, t}^{t-1} \left( \iint _{\mathbb {R}^{2N}} \frac{|\zeta _{\rho }(x)-\zeta _{\rho }(y)|^{t}}{|x-y|^{N+st}}|u_{n}(y)|^{t}\, dxdy \right) ^{\frac{1}{t}}\\&\le C \left( \iint _{\mathbb {R}^{2N}} \frac{|\zeta _{\rho }(x)-\zeta _{\rho }(y)|^{t}}{|x-y|^{N+st}}|u_{n}(y)|^{t}\, dxdy \right) ^{\frac{1}{t}}. \end{aligned}$$

Applying Lemma 2.3 in [4], we know that

$$\begin{aligned} \lim _{\rho \rightarrow 0}\limsup _{n\rightarrow \infty }\iint _{\mathbb {R}^{2N}} \frac{|\zeta _{\rho }(x)-\zeta _{\rho }(y)|^{t}}{|x-y|^{N+st}}|u_{n}(y)|^{t}\, dxdy=0, \end{aligned}$$

and thus (3.30) is true. Putting together (3.28), (3.29), (3.30), and using (3.26), we find $\mu _{i}\le \nu _{i}$. This fact combined with the last statement in (3.27) yields $\nu _{i}\ge S_{q}^{\frac{N}{sq}}$. Then, by $(g_{3})$, and recalling that $q>p$, we get

$$\begin{aligned} c&=\mathcal {J}_{\varepsilon }(u_{n})-\frac{1}{\vartheta } \langle \mathcal {J}'_{\varepsilon }(u_{n}), u_{n}\rangle +o_{n}(1)\\&=\left( \frac{1}{p}-\frac{1}{\vartheta }\right) \Vert u_{n}\Vert _{V_{\varepsilon },p}^{p}+\left( \frac{1}{q}-\frac{1}{\vartheta }\right) \Vert u_{n}\Vert _{V_{\varepsilon },q}^{q}\\&\quad +\int _{\mathbb {R}^{N}\setminus \Lambda _{\varepsilon }} \left( \frac{1}{\vartheta }g(\varepsilon x, u_{n})u_{n}-G(\varepsilon x, u_{n})\right) \, dx \\&\quad +\int _{\Lambda _{\varepsilon }} \left( \frac{1}{\vartheta }f(u_{n})u_{n}-F(u_{n})\right) \, dx+\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \int _{\Lambda _{\varepsilon }}|u_{n}|^{q^{*}_{s}}\, dx+o_{n}(1) \\&\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \int _{\Lambda _{\varepsilon }}|u_{n}|^{q^{*}_{s}}\, dx+o_{n}(1) \\&\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \int _{\Lambda _{\varepsilon }}|u_{n}|^{q^{*}_{s}}\zeta _{\rho }\, dx+o_{n}(1). \end{aligned}$$

From this and (3.27), we obtain that

$$\begin{aligned} c\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \sum _{\{i\in I: x_{i}\in \Lambda _{\varepsilon }\}} \zeta _{\rho }(x_{i}) \nu _{i}=\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \sum _{\{i\in I: x_{i}\in \Lambda _{\varepsilon }\}} \nu _{i}\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_{q}^{\frac{N}{sq}} \end{aligned}$$

which contradicts $c<\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_{q}^{\frac{N}{sq}}$. $\square$

Corollary 3.1

The functional $\psi _{\varepsilon }$ satisfies the $(PS)_{c}$ condition on $\mathbb {S}_{\varepsilon }^{+}$ at any level $c < \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_{q}^{\frac{N}{sq}}$.

Proof

Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {S}^{+}_{\varepsilon }$ be a $(PS)_{c}$ sequence for $\psi _{\varepsilon }$. Therefore,

$$\begin{aligned} \psi _{\varepsilon }(u_{n})\rightarrow c \quad \text{ and } \quad \psi _{\varepsilon }'(u_{n})\rightarrow 0 \text{ in } (T_{u_{n}} \mathbb {S}_{\varepsilon }^{+})'. \end{aligned}$$

By Proposition 3.1-(c), we see that $\{m_{\varepsilon }(u_{n})\}_{n\in \mathbb {N}}\subset \mathbb {X}_{\varepsilon }$ is a $(PS)_{c}$ sequence for $\mathcal {J}_{\varepsilon }$. Thus, by Lemma 3.5, we deduce that $\mathcal {J}_{\varepsilon }$ satisfies the $(PS)_{c}$ condition in $\mathbb {X}_{\varepsilon }$ and thus there exists $u\in \mathbb {S}_{\varepsilon }^{+}$ such that, up to a subsequence,

$$\begin{aligned} m_{\varepsilon }(u_{n})\rightarrow m_{\varepsilon }(u) \text{ in } \mathbb {X}_{\varepsilon }. \end{aligned}$$

Using Lemma 3.2-(iii), we obtain that $u_{n}\rightarrow u$ in $\mathbb {S}_{\varepsilon }^{+}$. $\square$

We conclude this section by proving an existence result for (3.1).

Theorem 3.1

Assume that $(V_1)$–$(V_{2})$ and $(f_1)$–$(f_4)$ hold. Then, for any $\varepsilon >0$, (3.1) has a positive ground state solution.

Proof

In light of Lemma 3.1, Remark 3.2 and Lemma 3.5, we can apply the mountain pass theorem [2] to deduce that, for all $\varepsilon >0$, there exists a nontrivial critical point $u_{\varepsilon }\in \mathbb {X}_{\varepsilon }$ for $\mathcal {J}_{\varepsilon }$. Since $\langle \mathcal {J}'_{\varepsilon }(u_{\varepsilon }), u^{-}_{\varepsilon }\rangle =0$, where $u^{-}_{\varepsilon }=\min \{u_{\varepsilon }, 0\}$, and $g(\varepsilon \cdot , t)=0$ for $t\le 0$, we can see that

$$\begin{aligned} \Vert u^{-}_{\varepsilon }\Vert _{V_{\varepsilon }, p}^{p} + \Vert u^{-}_{\varepsilon }\Vert _{V_{\varepsilon }, q}^{q}\le 0 \end{aligned}$$

which implies that $u^{-}_{\varepsilon }=0$, that is $u_{\varepsilon }\ge 0$ in $\mathbb {R}^{N}$. Arguing as in the proof of Lemma 4.1 in [8] (when $p<2$, we use Theorem 2.2 in [22] instead of Corollary 2.1 in [8]), we have that $u_{\varepsilon }\in L^{r}(\mathbb {R}^{N})\cap C^{0, \alpha }(\mathbb {R}^{N})$ for all $r\in [p, \infty ]$ and $u_{\varepsilon }(x)\rightarrow 0$ as $|x|\rightarrow \infty$. From the strong maximum principle [5], we derive that $u_{\varepsilon }>0$ in $\mathbb {R}^{N}$. $\square$

4 The Autonomous Problem

Since we are interested in giving a multiplicity result for the auxiliary problem (3.1), we consider the limiting problem associated with (1.1), namely

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u+V_{0} (u^{p-1}+u^{q-1})=f(u)+ u^{q^{*}_{s}-1} \, \text{ in } \mathbb {R}^{N},\\ u\in W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}, \end{array} \right. \end{aligned}$$

(4.1)

whose energy functional $\mathcal {E}_{V_{0}}: \mathbb {Y}_{V_{0}}\rightarrow \mathbb {R}$ is given by

$$\begin{aligned} \mathcal {E}_{V_{0}}(u)= \frac{1}{p}[u]_{s, p}^{p}+\frac{1}{q}[u]_{s, q}^{q} + V_{0} \left( \frac{1}{p} |u|_{p}^{p} +\frac{1}{q}|u|_{q}^{q}\right) -\int _{\mathbb {R}^{N}} \left( F(u)+\frac{1}{q^{*}_{s}}(u^{+})^{q^*_s}\right) \, dx, \end{aligned}$$

and $\mathbb {Y}_{V_{0}}=W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N})$ is equipped with the norm

$$\begin{aligned} \Vert u\Vert _{\mathbb {Y}_{V_{0}}}= \Vert u\Vert _{s, p}+ \Vert u\Vert _{s, q}, \end{aligned}$$

where

$$\begin{aligned} \Vert u\Vert _{s, t}= \left( [u]_{s, t}^{t}+V_{0} |u|_{t}^{t} \,\right) ^{\frac{1}{t}} \quad \text{ for } t\in \{p, q\}. \end{aligned}$$

It is standard to check that $\mathcal {E}_{V_{0}}\in C^{1}(\mathbb {Y}_{V_{0}}, \mathbb {R})$ and

$$\begin{aligned} \langle \mathcal {E}'_{V_{0}}(u), \varphi \rangle&=\langle u, \varphi \rangle _{s,p}+\langle u, \varphi \rangle _{s,q}+V_{0} \left( \int _{\mathbb {R}^{N}} |u|^{p-2}u\,\varphi \, dx+ \int _{\mathbb {R}^{N}} |u|^{q-2}u\,\varphi \, dx \right) \\&\quad - \int _{\mathbb {R}^{N}} (f(u)+ (u^{+})^{q^{*}_{s}-1})\varphi \, dx \end{aligned}$$

for any $u, \varphi \in \mathbb {Y}_{V_{0}}$. We also consider the Nehari manifold $\mathcal {M}_{V_{0}}$ associated with $\mathcal {E}_{V_{0}}$, that is

$$\begin{aligned} \mathcal {M}_{V_{0}} = \{u\in \mathbb {Y}_{V_{0}}\setminus \{0\} : \langle \mathcal {E}'_{V_{0}}(u), u\rangle =0\}, \end{aligned}$$

and we set $d_{V_{0}}=\inf _{u\in \mathcal {M}_{V_{0}}} \mathcal {E}_{V_{0}}(u)$. Now we define

$$\begin{aligned} \mathbb {Y}_{V_{0}}^{+}=\{u\in \mathbb {Y}_{V_{0}}: |{{\,\mathrm{supp}\,}}(u^{+})|>0\}, \end{aligned}$$

and $\mathbb {S}_{V_{0}}^{+}= \mathbb {S}_{V_{0}}\cap \mathbb {Y}_{V_{0}}^{+}$, where $\mathbb {S}_{V_{0}}$ is the unit sphere of $\mathbb {Y}_{V_{0}}$. As in Sect. 3, $\mathbb {S}_{V_{0}}^{+}$ is an incomplete $C^{1,1}$-manifold of codimension one and contained in $\mathbb {Y}_{V_{0}}^{+}$. Thus, $\mathbb {Y}_{V_{0}}= T_{u}\mathbb {S}_{V_{0}}^{+}\oplus \mathbb {R}u$ for each $u\in \mathbb {S}_{V_{0}}^{+}$, where

$$\begin{aligned} T_{u}\mathbb {S}_{V_{0}}^{+}&=\Biggl \{v\in \mathbb {Y}_{V_{0}} : \langle u, v\rangle _{s,p}+\langle u, v\rangle _{s,q}+V_{0} \int _{\mathbb {R}^{N}} (|u|^{p-2}u+|u|^{q-2}u)v\, dx=0\Biggr \}. \end{aligned}$$

Arguing as in Sect. 3, we can deduce the following results.

Lemma 4.1

The following properties hold:

(i):

For each $u\in \mathbb {Y}_{V_{0}}^{+}$, let $h:\mathbb {R}^{+}\rightarrow \mathbb {R}$ be defined by $h_{u}(t)= \mathcal {E}_{V_{0}}(tu)$. Then, there is a unique $t_{u}>0$ such that

$$\begin{aligned}&h'_{u}(t)>0 \, \text{ for } \text{ all } t\in (0, t_{u}),\\&h'_{u}(t)<0 \, \text{ for } \text{ all } t\in (t_{u}, \infty ). \end{aligned}$$

(ii):

There exists $\tau >0$, independent of u, such that $t_{u}\ge \tau$ for any $u\in \mathbb {S}_{V_{0}}^{+}$. Moreover, for each compact set $\mathbb {K}\subset \mathbb {S}_{V_{0}}^{+}$ there is a positive constant $C_{\mathbb {K}}$ such that $t_{u}\le C_{\mathbb {K}}$ for any $u\in \mathbb {K}$.

(iii):

The map ${\hat{m}}_{V_{0}}: \mathbb {Y}_{V_{0}}^{+}\rightarrow \mathcal {M}_{V_{0}}$ given by ${\hat{m}}_{V_{0}}(u)= t_{u}u$ is continuous and $m_{V_{0}}= {\hat{m}}_{V_{0}}|_{\mathbb {S}_{V_{0}}^{+}}$ is a homeomorphism between $\mathbb {S}_{V_{0}}^{+}$ and $\mathcal {M}_{V_{0}}$. Moreover, $m_{V_{0}}^{-1}(u)=\frac{u}{\Vert u\Vert _{\mathbb {Y}_{V_{0}}}}$.

(iv):

If there is a sequence $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {S}_{V_{0}}^{+}$ such that $\mathrm{dist}(u_{n}, \partial \mathbb {S}_{V_{0}}^{+})\rightarrow 0$, then $\Vert m_{V_{0}}(u_{n})\Vert _{\mathbb {Y}_{V_{0}}}\rightarrow \infty$ and $\mathcal {E}_{V_{0}}(m_{V_{0}}(u_{n}))\rightarrow \infty$.

Let us consider the maps

$$\begin{aligned} {\hat{\psi }}_{V_0}: \mathbb {Y}_{V_{0}}^{+} \rightarrow \mathbb {R}\quad \text{ and } \quad \psi _{V_0}: \mathbb {S}_{V_{0}}^{+}\rightarrow \mathbb {R}, \end{aligned}$$

by ${\hat{\psi }}_{V_{0}}(u)= \mathcal {E}_{V_{0}}({\hat{m}}_{V_{0}}(u))$ and $\psi _{V_{0}}={\hat{\psi }}_{V_{0}}|_{\mathbb {S}_{V_{0}}^{+}}$.

Proposition 4.1

The following properties hold:

(a):

${\hat{\psi }}_{V_{0}} \in C^{1}(\mathbb {Y}_{V_{0}}^{+}, \mathbb {R})$ and

$$\begin{aligned} \langle {\hat{\psi }}_{V_{0}}'(u), v\rangle = \frac{\Vert {\hat{m}}_{V_{0}}(u)\Vert _{\mathbb {Y}_{V_{0}}}}{\Vert u\Vert _{\mathbb {Y}_{V_{0}}}} \langle \mathcal {E}_{V_{0}}'({\hat{m}}_{V_{0}}(u)), v\rangle \quad \text{ for } \text{ all } u\in \mathbb {Y}_{V_{0}}^{+} \quad \text{ and } v\in \mathbb {Y}_{V_{0}}. \end{aligned}$$

(b):

$\psi _{V_{0}} \in C^{1}(\mathbb {S}_{V_{0}}^{+}, \mathbb {R})$ and

$$\begin{aligned} \langle \psi _{V_{0}}'(u), v \rangle = \Vert m_{V_{0}}(u)\Vert _{\mathbb {Y}_{V_{0}}} \langle \mathcal {E}_{V_{0}}'(m_{V_{0}}(u)), v\rangle , \quad \text{ for } \text{ all } v\in T_{u}\mathbb {S}_{V_{0}}^{+}. \end{aligned}$$

(c):

If $\{u_{n}\}_{n\in \mathbb {N}}$ is a $(PS)_{d}$ sequence for $\psi _{V_{0}}$, then $\{m_{V_{0}}(u_{n})\}_{n\in \mathbb {N}}$ is a $(PS)_{d}$ sequence for $\mathcal {E}_{V_{0}}$. If $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathcal {M}_{V_{0}}$ is a bounded $(PS)_{d}$ sequence for $\mathcal {E}_{V_{0}}$, then $\{m_{V_{0}}^{-1}(u_{n})\}_{n\in \mathbb {N}}$ is a $(PS)_{d}$ sequence for $\psi _{V_{0}}$.

(d):

u is a critical point of $\psi _{V_{0}}$ if, and only if, $m_{V_{0}}(u)$ is a nontrivial critical point for $\mathcal {E}_{V_{0}}$. Moreover, the corresponding critical values coincide and

$$\begin{aligned} \inf _{u\in \mathbb {S}_{V_{0}}^{+}} \psi _{V_{0}}(u)= \inf _{u\in \mathcal {M}_{V_{0}}} \mathcal {E}_{V_{0}}(u). \end{aligned}$$

Remark 4.1

As in Sect. 3, we have the following characterization of the infimum of $\mathcal {E}_{V_{0}}$ over $\mathcal {M}_{V_{0}}$:

$$\begin{aligned} 0<d_{V_{0}}&=\inf _{u\in \mathcal {M}_{V_{0}}} \mathcal {E}_{V_{0}}(u)=\inf _{u\in \mathbb {Y}_{V_{0}}^{+}} \max _{t>0} \mathcal {E}_{V_{0}}(tu)=\inf _{u\in \mathbb {S}_{V_{0}}^{+}} \max _{t>0} \mathcal {E}_{V_{0}}(tu). \end{aligned}$$

Moreover, arguing as in the proof of Lemma 3.3, we see that $0<d_{V_{0}}<\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_{q}^{\frac{N}{sq}}$.

The next lemma allows us to assume that the weak limit of a $(PS)_{d_{V_{0}}}$ sequence of $\mathcal {E}_{V_{0}}$ is nontrivial.

Lemma 4.2

Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {Y}_{V_{0}}$ be a $(PS)_{d_{V_{0}}}$ sequence for $\mathcal {E}_{V_{0}}$ such that $u_{n}\rightharpoonup 0$ in $\mathbb {Y}_{V_{0}}$. Then,

(a):

either $u_{n}\rightarrow 0$ in $\mathbb {Y}_{V_{0}}$, or

(b):

there is a sequence $\{y_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ and constants $R, \beta >0$ such that

$$\begin{aligned} \liminf _{n\rightarrow \infty }\int _{B_{R}(y_{n})} |u_{n}|^{q} \, dx \ge \beta . \end{aligned}$$

Proof

Suppose that (b) is false. Since $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {Y}_{V_{0}}$, Lemma 2.1 yields

$$\begin{aligned} u_{n}\rightarrow 0 \quad \text{ in } L^{r}(\mathbb {R}^{N}) \quad \text{ for } \text{ all } r \in (q, q^{*}_{s}). \end{aligned}$$

In particular, using $(f_{1})$ and $(f_{2})$, we see that

$$\begin{aligned} \int _{\mathbb {R}^{N}} F(u_{n})\, dx=\int _{\mathbb {R}^{N}} f(u_{n})u_{n}\, dx =o_{n}(1) \quad \text{ as } n\rightarrow \infty . \end{aligned}$$

Since $\langle \mathcal {E}'_{V_{0}}(u_{n}), u_{n}\rangle =o_{n}(1)$, we find

$$\begin{aligned} \Vert u_{n}\Vert _{s,p}^{p}+\Vert u_{n}\Vert _{s,q}^{q} = \int _{\mathbb {R}^{N}} f(u_{n}) u_{n}\, dx+ |u^{+}_{n}|^{q^{*}_{s}}_{q^{*}_{s}}=o_{n}(1)+ |u^{+}_{n}|^{q^{*}_{s}}_{q^{*}_{s}}. \end{aligned}$$

Up to a subsequence, there exists $\ell \ge 0$ such that

$$\begin{aligned} \Vert u_{n}\Vert _{s,p}^{p}+\Vert u_{n}\Vert _{s,q}^{q}\rightarrow \ell , \quad |u^{+}_{n}|^{q^{*}_{s}}_{q^{*}_{s}}\rightarrow \ell . \end{aligned}$$

Assume by contradiction that $\ell >0$. Since $\mathcal {E}_{V_{0}}(u_{n})\rightarrow d_{V_{0}}$, $\langle \mathcal {E}'_{V_{0}}(u_{n}), u_{n}\rangle =0$ and $q>p$, we deduce that

$$\begin{aligned} d_{V_{0}}+o_{n}(1)&=\mathcal {E}_{V_{0}}(u_{n})-\frac{1}{\vartheta }\langle \mathcal {E}'_{V_{0}}(u_{n}), u_{n}\rangle \\&=\left( \frac{1}{p}-\frac{1}{\vartheta }\right) \Vert u_{n}\Vert ^{p}_{s, p}+\left( \frac{1}{q}-\frac{1}{\vartheta }\right) \Vert u_{n}\Vert ^{q}_{s, q}+o_{n}(1)+\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) |u^{+}_{n}|^{q^{*}_{s}}_{q^{*}_{s}}\\&\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \ell +o_{n}(1), \end{aligned}$$

which implies that

$$\begin{aligned} d_{V_{0}}\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \ell . \end{aligned}$$

By Theorem 2.1, we see that

$$\begin{aligned} \Vert u_{n}\Vert _{s,p}^{p}+\Vert u_{n}\Vert _{s,q}^{q}\ge S_{q}|u_{n}|^{q}_{q^{*}_{s}}\ge S_{q}(|u^{+}_{n}|^{q^{*}_{s}}_{q^{*}_{s}})^{\frac{q}{q^{*}_{s}}} \end{aligned}$$

and letting $n\rightarrow \infty$ we obtain

$$\begin{aligned} \ell \ge S_{q} \ell ^{\frac{q}{q^{*}_{s}}} \end{aligned}$$

that is $\ell \ge S_{q}^{\frac{N}{sq}}$. Consequently,

$$\begin{aligned} d_{V_{0}}\ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) \ell \ge \left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s}}\right) S_{q}^{\frac{N}{sq}} \end{aligned}$$

and this contradicts Remark 4.1. $\square$

Remark 4.2

As it has been mentioned earlier, if u is the weak limit of a $(PS)_{d_{V_{0}}}$ sequence for $\mathcal {E}_{V_{0}}$, then we can assume $u\ne 0$. Otherwise, if $u_{n}\rightharpoonup 0$ and $u_{n}\nrightarrow 0$ in $\mathbb {Y}_{V_{0}}$, it follows from Lemma 4.2 that there are $\{y_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ and $R, \beta >0$ such that

$$\begin{aligned} \liminf _{n\rightarrow \infty }\int _{B_{R}(y_{n})} |u_{n}|^{q} \, dx \ge \beta . \end{aligned}$$

Set $v_{n}(x)=u_{n}(x+y_{n})$. Then, using the invariance of $\mathbb {R}^N$ by translation, we see that $\{v_{n}\}_{n\in \mathbb {N}}$ is a bounded $(PS)_{d_{V_{0}}}$ sequence for $\mathcal {E}_{V_{0}}$ such that $v_{n}\rightharpoonup v$ in $\mathbb {Y}_{V_{0}}$ with $v\ne 0$.

In the next result we obtain a positive ground state solution for the autonomous problem (4.1).

Theorem 4.1

Problem (4.1) admits a positive ground state solution.

Proof

Invoking a variant of the mountain-pass theorem without the Palais–Smale condition (see [35]), there exists a $(PS)_{d_{V_{0}}}$ sequence $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {Y}_{V_{0}}$ for $\mathcal {E}_{V_{0}}$. Arguing as in the proof of Lemma 3.5, we can verify that $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {Y}_{V_{0}}$ and so, passing to a subsequence if necessary, we may assume that

$$\begin{aligned} \begin{array}{ll} u_{n}\rightharpoonup u &{} \text{ in } \mathbb {Y}_{V_{0}}, \\ u_{n}\rightarrow u &{} \text{ in } L^{r}_{loc}(\mathbb {R}^{N}) \text{ for } \text{ all } r\in [1, q^{*}_{s}). \end{array} \end{aligned}$$

Standard arguments (see proof of Lemma 3.5) show that $\mathcal {E}_{V_{0}}'(u)=0$. From Remark 4.2, we may assume that $u\ne 0$. On the other hand, by Fatou’s lemma and $(f_3)$, we deduce that

$$\begin{aligned} \mathcal {E}_{V_{0}}(u)- \frac{1}{\vartheta }\langle \mathcal {E}_{V_{0}}'(u), u\rangle \le \liminf _{n\rightarrow \infty } \left( \mathcal {E}_{V_{0}}(u_{n})- \frac{1}{\vartheta }\langle \mathcal {E}_{V_{0}}'(u_{n}), u_{n}\rangle \right) = d_{V_{0}} \end{aligned}$$

which yields $d_{V_{0}}=\mathcal {E}_{V_{0}}(u)$. Finally, we prove that u is positive. Since $\langle \mathcal {E}'_{V_{0}}(u), u^{-}\rangle =0$, where $u^{-}=\min \{u, 0\}$, and $f(t)=0$ for $t\le 0$, we have

$$\begin{aligned} \Vert u^{-}\Vert _{s, p}^{p} + \Vert u^{-}\Vert _{s, q}^{q}\le 0 \end{aligned}$$

which gives $u^{-}=0$, that is $u\ge 0$ in $\mathbb {R}^{N}$. Therefore, $u\ge 0$ and $u\not \equiv 0$ in $\mathbb {R}^{N}$. Arguing as in the proof of Lemma 4.1 in [8], we see that $u\in L^{r}(\mathbb {R}^{N})\cap C^{0, \alpha }(\mathbb {R}^{N})$ for all $r\in [p, \infty ]$ and $|u(x)|\rightarrow 0$ as $|x|\rightarrow \infty$. By the strong maximum principle [5], we arrive at $u>0$ in $\mathbb {R}^{N}$. $\square$

The next lemma is a useful compactness result for the autonomous problem (4.1).

Lemma 4.3

Let $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathcal {M}_{V_{0}}$ be a sequence such that $\mathcal {E}_{V_{0}}(u_{n})\rightarrow d_{V_{0}}$. Then, $\{u_{n}\}_{n\in \mathbb {N}}$ has a convergent subsequence in $\mathbb {Y}_{V_{0}}$.

Proof

Since $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathcal {M}_{V_{0}}$ and $\mathcal {E}_{V_{0}}(u_{n})\rightarrow d_{V_{0}}$, it follows from Lemma 4.1-(iii), Proposition 4.1-(d) and the definition of $d_{V_{0}}$ that

$$\begin{aligned} v_{n}=m^{-1}_{V_{0}}(u_{n})=\frac{u_{n}}{\Vert u_{n}\Vert _{\mathbb {Y}_{V_{0}}}}\in \mathbb {S}_{V_{0}}^{+} \quad \text{ for } \text{ all } n\in \mathbb {N}, \end{aligned}$$

and

$$\begin{aligned} \psi _{V_{0}}(v_{n})=\mathcal {E}_{V_{0}}(u_{n})\rightarrow d_{V_{0}}=\inf _{v\in \mathbb {S}_{V_{0}}^{+}}\psi _{V_{0}}(v). \end{aligned}$$

Let us define $\mathcal {G}: \overline{\mathbb {S}}_{V_{0}}^{+}\rightarrow \mathbb {R}\cup \{\infty \}$ as

$$\begin{aligned} \mathcal {G}(u)= {\left\{ \begin{array}{ll} \psi _{V_{0}}(u)&{} \text { if }u\in \mathbb {S}_{V_{0}}^{+}, \\ \infty &{} \hbox { if}\ u\in \partial \mathbb {S}_{V_{0}}^{+}. \end{array}\right. } \end{aligned}$$

Note that

• $(\overline{\mathbb {S}}_{V_{0}}^{+}, \delta _{V_{0}})$, where $\delta _{V_{0}}(u, v)=\Vert u-v\Vert _{\mathbb {Y}_{V_{0}}}$, is a complete metric space;

• $\mathcal {G}\in C(\overline{\mathbb {S}}_{V_{0}}^{+}, \mathbb {R}\cup \{\infty \})$, by Lemma 4.1-(iv);

• $\mathcal {G}$ is bounded below, by Proposition 4.1-(d).

Hence, applying the Ekeland variational principle [19] to $\mathcal {G}$, there exists $\{{\hat{v}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {S}_{V_{0}}^{+}$ such that $\{{\hat{v}}_{n}\}_{n\in \mathbb {N}}$ is a $(PS)_{d_{V_{0}}}$ sequence for $\psi _{V_{0}}$ at the level $d_{V_{0}}$ and $\Vert {\hat{v}}_{n}-v_{n}\Vert _{\mathbb {Y}_{V_{0}}}=o_{n}(1)$. Now the remainder of the proof follows from Proposition 4.1, Theorem 4.1, and arguing as in the proof of Corollary 3.1. $\square$

Finally we prove a useful relation between the minimax levels $c_{\varepsilon }$ and $d_{V_{0}}$.

Lemma 4.4

It holds $\lim _{\varepsilon \rightarrow 0} c_{\varepsilon }=d_{V_{0}}$.

Proof

Let $\omega _{\varepsilon }(x)= \psi _{\varepsilon }(x)\omega (x)$, where $\omega$ is a positive ground state of (4.1) (thanks to Theorem 4.1), and $\psi _{\varepsilon }(x)= \psi (\varepsilon x)$ with $\psi \in C^{\infty }_{c}(\mathbb {R}^{N})$ such that $0\le \psi \le 1$, $\psi (x)=1$ if $|x|\le r$ and $\psi (x)=0$ if $|x|\ge 2r$. For simplicity, we assume that $\mathrm{supp}( \psi )\subset B_{2r}(0)\subset \Lambda$ for some $r > 0$. Applying the dominated convergence theorem, we have that

$$\begin{aligned} \omega _{\varepsilon }\rightarrow \omega \quad \text{ in } \mathcal {W} \quad \text{ and } \quad \mathcal {E}_{V_{0}}(\omega _{\varepsilon }) \rightarrow \mathcal {E}_{V_{0}}(\omega )= d_{V_{0}} \end{aligned}$$

(4.2)

as $\varepsilon \rightarrow 0$. Now, for each $\varepsilon >0$ there exists $t_{\varepsilon }>0$ such that

$$\begin{aligned} \mathcal {J}_{\varepsilon }(t_{\varepsilon } \omega _{\varepsilon })=\max _{t\ge 0} \mathcal {J}_{\varepsilon }(t \omega _{\varepsilon }). \end{aligned}$$

Therefore, $\langle \mathcal {J}_{\varepsilon }'(t_{\varepsilon } \omega _{\varepsilon }), \omega _{\varepsilon }\rangle =0$ and this implies that

$$\begin{aligned}&t^{p}_{\varepsilon }[\omega _{\varepsilon }]_{s, p}^{p}+t^{q}_{\varepsilon }[\omega _{\varepsilon }]_{s, q}^{q}+ t^{p}_{\varepsilon }\int _{{\mathbb {R}}^{N}} V(\varepsilon x)\omega ^{p}_{\varepsilon } \,dx+t^{q}_{\varepsilon }\int _{\mathbb {R}^{N}} V(\varepsilon x)\omega ^{q}_{\varepsilon } \,dx\\&\quad = \int _{\mathbb {R}^{N}} \left( f(t_{\varepsilon } \omega _{\varepsilon })t_{\varepsilon }\omega _{\varepsilon }+ (t_{\varepsilon }\omega _{\varepsilon })^{q^{*}_{s}}\right) \, dx. \end{aligned}$$

If $t_{\varepsilon }\rightarrow \infty$ then

$$\begin{aligned}&t^{p-q}_{\varepsilon }[\omega _{\varepsilon }]_{s, p}^{p}+[\omega _{\varepsilon }]_{s, q}^{q}+ t^{p-q}_{\varepsilon }\int _{\mathbb {R}^{N}} V(\varepsilon x)\omega ^{p}_{\varepsilon } \,dx+\int _{\mathbb {R}^{N}} V(\varepsilon x)\omega ^{q}_{\varepsilon } \,dx\nonumber \\&\quad = \int _{\mathbb {R}^{N}} \frac{f(t_{\varepsilon } \omega _{\varepsilon })+ (t_{\varepsilon }\omega _{\varepsilon })^{q^{*}_{s}-1}}{(t_{\varepsilon } \omega _{\varepsilon })^{q-1}}\omega _{\varepsilon }^{q}\, dx \end{aligned}$$

(4.3)

and using (4.2), $p<q$ and $(f_3)$, we obtain that $\Vert \omega \Vert ^{q}_{s, q}=\infty$ which is impossible. Then $t_{\varepsilon }\rightarrow t_{0}\in [0, \infty )$. If $t_{0}=0$, by $(f_1)$ and $(f_2)$, we see that for $\zeta \in (0, V_{0})$, it holds

$$\begin{aligned} \left( 1-\frac{\zeta }{V_{0}}\right) \Vert \omega _{\varepsilon }\Vert ^{p}_{V_{\varepsilon , p}}+t^{q-p}_{\varepsilon } \Vert \omega _{\varepsilon }\Vert ^{q}_{V_{\varepsilon , q}}\le C_{\zeta }t^{q-p}_{\varepsilon } \Vert \omega _{\varepsilon }\Vert ^{q^{*}_{s}}_{V_{\varepsilon , q}} \end{aligned}$$

which yields $\Vert \omega \Vert ^{p}_{s, p}=0$ and this gives a contradiction. Hence, $t_{\varepsilon }\rightarrow t_{0}>0$. Letting $\varepsilon \rightarrow 0$ in (4.3), we find

$$\begin{aligned}&t^{p-q}_{0}[\omega ]^{p}_{s, p}+[\omega ]^{q}_{s, q}+t^{p-q}_{0}\int _{\mathbb {R}^{N}} V_{0} \omega ^{p} \,dx+\int _{\mathbb {R}^{N}} V_{0} \omega ^{q} \,dx\\&\quad =\int _{\mathbb {R}^{N}} \frac{f(t_{0}\omega ) + (t_{0}\omega )^{q^{*}_{s}-1}}{(t_{0}\omega )^{q-1}}\omega ^{q}\,dx \end{aligned}$$

which combined with $(f_{4})$ and $\omega \in \mathcal {M}_{V_{0}}$ implies that $t_{0}=1$. On the other hand, we have that

$$\begin{aligned} c_{\varepsilon }\le \max _{t\ge 0} \mathcal {J}_{\varepsilon }(t \omega _{\varepsilon })=\mathcal {J}_{\varepsilon }(t_{\varepsilon } \omega _{\varepsilon })= & {} \mathcal {\mathcal {E}}_{V_{0}}(t_{\varepsilon } \omega _{\varepsilon }) + \frac{t_{\varepsilon }^{p}}{p} \int _{\mathbb {R}^{N}} (V(\varepsilon x) - V_{0} ) \omega _{\varepsilon }^{p} \, dx\\&+\frac{t_{\varepsilon }^{q}}{q} \int _{\mathbb {R}^{N}} (V(\varepsilon x) - V_{0} ) \omega _{\varepsilon }^{q} \, dx. \end{aligned}$$

Since $V(\varepsilon \cdot )$ is bounded on the support of $\omega _{\varepsilon }$, we use the dominated convergence theorem, (4.2) and the above inequality to deduce that $\limsup _{\varepsilon \rightarrow 0}c_{\varepsilon }\le d_{V_{0}}$. Since $(V_1)$ gives $\liminf _{\varepsilon \rightarrow 0}c_{\varepsilon }\ge d_{V_{0}}$, we infer that $\lim _{\varepsilon \rightarrow 0}c_{\varepsilon }= d_{V_{0}}$. $\square$

5 Multiplicity of Solutions to (3.1)

This section is devoted to provide some technical results needed to implement the barycenter machinery below. Let $\delta >0$ be such that

$$\begin{aligned} M_{\delta }=\{x\in \mathbb {R}^{N}: \mathrm{dist}(x, M)\le \delta \}\subset \Lambda , \end{aligned}$$

(5.1)

and $w\in \mathbb {Y}_{V_{0}}$ be a positive ground state solution to the autonomous problem (4.1) (whose existence is ensured by Theorem 4.1). Let $\eta \in C^{\infty }([0, \infty ), [0, 1])$ be a non increasing function such that $\eta (t)=1$ if $0\le t\le \frac{\delta }{2}$, $\eta (t)=0$ if $t\ge \delta$ and $|\eta '(t)|\le c$ for some $c>0$. For any $y\in M$, we define

$$\begin{aligned} \Psi _{\varepsilon , y}(x)=\eta (|\varepsilon x-y|) w\left( \frac{\varepsilon x-y}{\varepsilon }\right) \end{aligned}$$

and $\Phi _{\varepsilon }: M\rightarrow \mathcal {N}_{\varepsilon }$ given by

$$\begin{aligned} \Phi _{\varepsilon }(y)= t_{\varepsilon } \Psi _{\varepsilon , y}, \end{aligned}$$

where $t_{\varepsilon }>0$ satisfies

$$\begin{aligned} \max _{t\ge 0} \mathcal {J}_{\varepsilon }(t \Psi _{\varepsilon , y})=\mathcal {J}_{\varepsilon }(t_{\varepsilon } \Psi _{\varepsilon , y}). \end{aligned}$$

By construction, $\Phi _{\varepsilon }(y)$ has compact support for any $y\in M$.

Lemma 5.1

The functional $\Phi _{\varepsilon }$ verifies the following limit:

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} \mathcal {J}_{\varepsilon }(\Phi _{\varepsilon }(y))=d_{V_0} \text{ uniformly } \text{ in } y\in M. \end{aligned}$$

Proof

Assume by contradiction that there exist $\delta _{0}>0$, $\{y_{n}\}_{n\in \mathbb {N}}\subset M$ and $\varepsilon _{n}\rightarrow 0$ such that

$$\begin{aligned} |\mathcal {J}_{\varepsilon _{n}}(\Phi _{\varepsilon _{n}}(y_{n}))-d_{V_0}|\ge \delta _{0}. \end{aligned}$$

(5.2)

Now, for each $n\in \mathbb {N}$ and for all $z\in B_{\frac{\delta }{\varepsilon _{n}}}(0)$, we have $\varepsilon _{n} z\in B_{\delta }(0)$, and thus

$$\begin{aligned} \varepsilon _{n} z+y_{n}\in B_{\delta }(y_{n})\subset M_{\delta }\subset \Lambda . \end{aligned}$$

Using the change of variable $z=\frac{\varepsilon _{n}x-y_{n}}{\varepsilon _{n}}$ and the fact that $G(x, t)=F(x, t)+\frac{1}{q^{*}_{s}}t^{q^{*}_{s}}$ for $(x, t)\in \Lambda \times [0, \infty )$, we can see that

$$\begin{aligned} \mathcal {J}_{\varepsilon _{n}}(\Phi _{\varepsilon _{n}}(y_{n}))&=\frac{t^{p}_{\varepsilon _{n}}}{p} \Vert \Psi _{\varepsilon _{n}, y_{n}} \Vert ^{p}_{V_{\varepsilon _{n}},p}+\frac{t^{q}_{\varepsilon _{n}}}{q} \Vert \Psi _{\varepsilon _{n}, y_{n}} \Vert ^{q}_{V_{\varepsilon _{n}},q}-\int _{\mathbb {R}^{N}} G(\varepsilon _{n} x, t_{\varepsilon _{n}} \Psi _{\varepsilon _{n}, y_{n}}) \, dx \nonumber \\&=\frac{t^{p}_{\varepsilon _{n}}}{p} \left( [\eta (|\varepsilon _{n}\cdot |) w]^{p}_{s, p}+\int _{\mathbb {R}^{N}} V(\varepsilon _{n} z+y_{n}) (\eta (|\varepsilon _{n} z|) w(z))^{p} \, dz\right) \nonumber \\&\quad +\frac{t^{q}_{\varepsilon _{n}}}{q} \left( [\eta (|\varepsilon _{n}\cdot |) w]^{q}_{s, q}+\int _{\mathbb {R}^{N}} V(\varepsilon _{n} z+y_{n}) (\eta (|\varepsilon _{n} z|) w(z))^{q} \, dz\right) \nonumber \\&\quad -\int _{\mathbb {R}^{N}} \left( F(t_{\varepsilon _{n}} \eta (|\varepsilon _{n} z|) w(z))+\frac{1}{q^{*}_{s}} (t_{\varepsilon _{n}} \eta (|\varepsilon _{n} z|) w(z))^{q^{*}_{s}}\right) \, dz. \end{aligned}$$

(5.3)

We claim that $t_{\varepsilon _{n}}\rightarrow 1$ as $\varepsilon _{n}\rightarrow 0$. We start by proving that $t_{\varepsilon _{n}}\rightarrow t_{0}\in [0, \infty )$. Since $\langle \mathcal {J}'_{\varepsilon _{n}}(\Phi _{\varepsilon _{n}}(y_{n})),\Phi _{\varepsilon _{n}}(y_{n})\rangle =0$ and $g(x, t)=f(t)+t^{q^{*}_{s}-1}$ for $(x, t)\in \Lambda \times \mathbb {R}$, we have

$$\begin{aligned}&\frac{1}{t_{\varepsilon _{n}}^{q-p}} \Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},p}^{p}+\Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},q}^{q}\nonumber \\&=\int _{\mathbb {R}^{N}} \Biggl ( \frac{f(t_{\varepsilon _{n}} \eta (|\varepsilon _{n} z|) w(z))+ (t_{\varepsilon _{n}} \eta (|\varepsilon _{n} z|) w(z))^{q^{*}_{s}-1}}{(t_{\varepsilon _{n}} \eta (|\varepsilon _{n} z|) w(z))^{q-1}}\Biggr ) (\eta (|\varepsilon _{n} z|) w(z))^{q} \, dz. \end{aligned}$$

(5.4)

Observing that $\eta (|x|)=1$ for $x\in B_{\frac{\delta }{2}}(0)$ and $B_{\frac{\delta }{2}}(0)\subset B_{\frac{\delta }{\varepsilon _{n}}}(0)$ for all n large enough, the identity (5.4) yields

$$\begin{aligned}&t_{\varepsilon _{n}}^{p-q} \Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},p}^{p}+\Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},q}^{q}\\&\quad \ge \int _{B_{\frac{\delta }{2}}(0)} \Biggl ( \frac{f(t_{\varepsilon _{n}} w(z))+ (t_{\varepsilon _{n}} w(z))^{q^{*}_{s}-1}}{(t_{\varepsilon _{n}} w(z))^{q-1}}\Biggr ) (w(z))^{q} \, dz, \end{aligned}$$

which together with $(f_4)$ gives

$$\begin{aligned} t_{\varepsilon _{n}}^{p-q} \Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},p}^{p}+\Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},q}^{q}&\ge \left( \left( \frac{f(t_{\varepsilon _{n}} w({\hat{z}}))}{(t_{\varepsilon _{n}} w({\hat{z}}))^{q-1}}\right) (w({\hat{z}}))^{q} + t^{q^{*}_{s}-q}_{\varepsilon _{n}} (w({\hat{z}}))^{q^{*}_{s}}\right) \nonumber \\&\quad |B_{\frac{\delta }{2}}(0)|, \end{aligned}$$

(5.5)

where

$$\begin{aligned} w({\hat{z}})=\min _{z\in \overline{B_{\frac{\delta }{2}}}(0)} w(z)>0 \end{aligned}$$

(we recall that w is continuous and positive in $\mathbb {R}^N$). If $t_{\varepsilon _{n}}\rightarrow \infty$, using the fact that $q>p$ and that the dominated convergence theorem yields

$$\begin{aligned} \Vert \Psi _{\varepsilon _{n}, y_{n}} \Vert _{V_{\varepsilon _{n}},r}\rightarrow \Vert w\Vert _{s,r}\in (0, \infty ) \quad \text{ for } \text{ all } r\in \{p,q\}, \end{aligned}$$

(5.6)

we find

$$\begin{aligned} t_{\varepsilon _{n}}^{p-q} \Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},p}^{p}+\Vert \Psi _{\varepsilon _{n}, y_{n}}\Vert _{V_{\varepsilon _{n}},q}^{q} \rightarrow \Vert w\Vert _{s,q}^{q}. \end{aligned}$$

(5.7)

On the other hand, by $(f_3)$, we get

$$\begin{aligned} \lim _{n\rightarrow \infty } \frac{f(t_{\varepsilon _{n}} w({\hat{z}}))}{(t_{\varepsilon _{n}} w({\hat{z}}))^{q-1}}=\infty . \end{aligned}$$

(5.8)

In light of (5.5), (5.7), (5.8) and that $q^{*}_{s}>q$, we achieve a contradiction. Consequently, $\{t_{\varepsilon _{n}}\}_{n\in \mathbb {N}}$ is bounded and, up to a subsequence, we may suppose that $t_{\varepsilon _{n}}\rightarrow t_{0}$ for some $t_{0}\ge 0$. From (5.4), (5.6), $(f_1)$-$(f_2)$, we can see that $t_{0}>0$. Now we claim that $t_{0}=1$. Letting $n\rightarrow \infty$ in (5.4), and using (5.6) and the dominated convergence theorem, we have that

$$\begin{aligned} t_{0}^{p-q} \Vert w\Vert ^{p}_{s,p}+\Vert w\Vert _{s,q}^{q}=\int _{\mathbb {R}^{N}} \frac{f(t_{0} w)+ (t_{0} w)^{q^{*}_{s}-1}}{(t_{0}w)^{q-1}} \,w^{q} \, dx. \end{aligned}$$

Since $w\in \mathcal {M}_{V_0}$, it holds

$$\begin{aligned} \Vert w\Vert ^{p}_{s,p}+\Vert w\Vert _{s,q}^{q}=\int _{\mathbb {R}^{N}} \left( f(w) w+ w^{q^{*}_{s}}\right) \, dx. \end{aligned}$$

Then we get

$$\begin{aligned} (t_{0}^{p-q}-1)\Vert w\Vert ^{p}_{s,p}=\int _{\mathbb {R}^{N}} \left( \frac{f(t_{0} w)}{(t_{0}w)^{q-1}} -\frac{f(w)}{w^{q-1}} \right) \,w^{q} \, dx+ (t^{q^{*}_{s}-q}_{0}-1)\int _{\mathbb {R}^{N}} w^{q^{*}_{s}}\, dx. \end{aligned}$$

which together with $(f_4)$ implies that $t_{0}=1$. Therefore, letting $n\rightarrow \infty$ in (5.3), we deduce that

$$\begin{aligned} \lim _{n\rightarrow \infty } \mathcal {J}_{\varepsilon _{n}}(\Phi _{\varepsilon _{n}, y_{n}})=\mathcal {E}_{V_0}(w)=d_{V_0}, \end{aligned}$$

which contradicts (5.2). $\square$

For any $\delta >0$ given by (5.1), let $\rho =\rho (\delta )>0$ be such that $M_{\delta }\subset B_{\rho }(0)$. Define $\varUpsilon : \mathbb {R}^{N}\rightarrow \mathbb {R}^{N}$ by setting

$$\begin{aligned} \varUpsilon (x)= \left\{ \begin{array}{ll} x &{} \text{ if } |x|<\rho , \\ \frac{\rho x}{|x|} &{} \text{ if } |x|\ge \rho . \end{array} \right. \end{aligned}$$

Let us consider the barycenter map $\beta _{\varepsilon }: \mathcal {N}_{\varepsilon }\rightarrow \mathbb {R}^{N}$ given by

$$\begin{aligned} \beta _{\varepsilon }(u)=\frac{\displaystyle {\int _{\mathbb {R}^{N}} \varUpsilon (\varepsilon x)(|u(x)|^{p}+|u(x)|^{q}) \,dx}}{\displaystyle {\int _{\mathbb {R}^{N}} (|u(x)|^{p}+|u(x)|^{q}) \,dx}}. \end{aligned}$$

Since $M\subset B_{\rho }(0)$, by the definition of $\varUpsilon$ and applying the dominated convergence theorem, we can obtain (see Lemma 3.6 in [8]) that

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} \beta _{\varepsilon }(\Phi _{\varepsilon }(y))=y \text{ uniformly } \text{ in } y\in M. \end{aligned}$$

(5.9)

The next compactness result plays an important role to verify that the solutions of the modified problem are also solutions of the original one.

Lemma 5.2

Let $\varepsilon _{n}\rightarrow 0$ and $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathcal {N}_{\varepsilon _{n}}$ be such that $\mathcal {J}_{\varepsilon _{n}}(u_{n})\rightarrow d_{V_0}$. Then there exists $\{{\tilde{y}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ such that $v_{n}(x)=u_{n}(x+ {\tilde{y}}_{n})$ has a convergent subsequence in $\mathbb {Y}_{V_0}$. Moreover, up to a subsequence, $\{y_{n}\}_{n\in \mathbb {N}}=\{\varepsilon _{n}{\tilde{y}}_{n}\}_{n\in \mathbb {N}}$ is such that $y_{n}\rightarrow y_{0}\in M$.

Proof

Arguing as in the proof of Lemma 3.4, it is easy to check that $\{u_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {Y}_{V_{0}}$. Clearly, $\Vert u_{n}\Vert _{\mathbb {X}_{\varepsilon _{n}}}\nrightarrow 0$ since $d_{V_0}>0$. Consequently, proceeding as in the proof of Lemma 4.2 and Remark 4.2, we obtain a sequence $\{{\tilde{y}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ and constants $R, \beta >0$ such that

$$\begin{aligned} \liminf _{n\rightarrow \infty }\int _{B_{R}({\tilde{y}}_{n})} |u_{n}|^{q} dx\ge \beta . \end{aligned}$$

Put $v_{n}(x)=u_{n}(x+ {\tilde{y}}_{n})$. Then, $\{v_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {Y}_{V_0}$, and, going to a subsequence if necessary, we may assume that $v_{n}\rightharpoonup v\not \equiv 0$ in $\mathbb {Y}_{V_0}$. Take $t_{n}>0$ such that ${\tilde{v}}_{n}=t_{n}v_{n} \in \mathcal {M}_{V_0}$ and set $y_{n}=\varepsilon _{n}{\tilde{y}}_{n}$. From $u_{n}\in \mathcal {N}_{\varepsilon _{n}}$ and $(g_2)$, we get

$$\begin{aligned} d_{V_0}\le \mathcal {E}_{V_0}({\tilde{v}}_{n})&\le \frac{1}{p} [{\tilde{v}}_{n}]_{s, p}^{p}+\frac{1}{q}[{\tilde{v}}_{n}]_{s, q}^{q}+\int _{\mathbb {R}^{N}}V(\varepsilon _{n} x+y_{n}) \left( \frac{1}{p}|{\tilde{v}}_{n}|^{p}+\frac{1}{q}|{\tilde{v}}_{n}|^{q}\right) \, dx\\&-\int _{\mathbb {R}^{N}} \left( F({\tilde{v}}_{n})+\frac{1}{q^{*}_{s}}({\tilde{v}}_{n}^+)^{q^{*}_{s}} \right) \, dx \nonumber \\&\le \frac{t_{n}^{p}}{p} [u_{n}]_{s, p}^{p}+\frac{t_{n}^{q}}{q} [u_{n}]_{s, q}^{q} +\int _{\mathbb {R}^{N}}V(\varepsilon _{n} x) \left( \frac{t_{n}^{p}}{p}|u_{n}|^{p}+\frac{t_{n}^{q}}{q}|u_{n}|^{q}\right) \, dx\\&\quad -\int _{\mathbb {R}^{N}} G(\varepsilon _{n} x, t_{n}u_{n})\, dx \nonumber \\&=\mathcal {J}_{\varepsilon _{n}}(t_{n}u_{n}) \le \mathcal {J}_{\varepsilon _{n}}(u_{n})= d_{V_0}+ o_{n}(1), \end{aligned}$$

which implies that

$$\begin{aligned} \mathcal {E}_{V_0}({\tilde{v}}_{n})\rightarrow d_{V_0} \, \text{ and } \,\{{\tilde{v}}_{n}\}_{n\in \mathbb {N}}\subset \mathcal {M}_{V_0}. \end{aligned}$$

(5.10)

Moreover, $\{{\tilde{v}}_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {Y}_{V_0}$ and thus, up to a subsequence, ${\tilde{v}}_{n}\rightharpoonup {\tilde{v}}$ in $\mathbb {Y}_{V_0}$. Using standard arguments, we may assume that $t_{n}\rightarrow t_{0}> 0$. From the uniqueness of the weak limit, we have ${\tilde{v}}=t_{0} v\not \equiv 0$. By Lemma 4.3, we get ${\tilde{v}}_{n}\rightarrow {\tilde{v}}$ in $\mathbb {Y}_{V_0}$, and so $\displaystyle {v_{n}\rightarrow v}$ in $\mathbb {Y}_{V_0}$. Moreover,

$$\begin{aligned} \mathcal {E}_{V_0}({\tilde{v}})=d_{V_0} \, \text{ and } \, \langle \mathcal {E}'_{V_0}({\tilde{v}}), {\tilde{v}}\rangle =0. \end{aligned}$$

Next we show that $\{y_{n}\}_{n\in \mathbb {N}}$ admits a bounded subsequence. Otherwise, assume that there is a subsequence of $\{y_{n}\}_{n\in \mathbb {N}}$, still denoted by itself, such that $|y_{n}|\rightarrow \infty$. Choose $R>0$ such that $\Lambda \subset B_{R}(0)$. Then, for n large enough, $|y_{n}|>2R$, and for each $x\in B_{R/\varepsilon _{n}}(0)$ we have

$$\begin{aligned} |\varepsilon _{n} x+y_{n}|\ge |y_{n}|-|\varepsilon _{n} x|>R. \end{aligned}$$

Using $v_{n}\rightarrow v$ in $\mathbb {Y}_{V_0}$, the definition of g, and the dominated convergence theorem, we find

$$\begin{aligned} \Vert v_{n}\Vert _{s,p}^{p}+\Vert v_{n}\Vert _{s,q}^{q}&\le \int _{\mathbb {R}^{N}} g(\varepsilon _{n}x+y_{n}, v_{n}) v_{n} \, dx \\&\le \int _{B_{R/\varepsilon _{n}}(0)} {\tilde{f}}(v_{n}) v_{n} \,dx+\int _{B_{R/\varepsilon _{n}}^{c}(0)} \left( f(v_{n}) v_{n}+ (v_{n}^+)^{q^{*}_{s}}\right) \, dx \\&\le \frac{1}{K} \int _{B_{R/\varepsilon _{n}}(0)} V_{0} (|v_{n}|^{p}+|v_{n}|^{q}) \,dx+o_{n}(1) \end{aligned}$$

which gives

$$\begin{aligned} \left( 1-\frac{1}{K}\right) (\Vert v_{n}\Vert ^{p}_{s,p}+\Vert v_{n}\Vert ^{q}_{s,q})\le o_{n}(1). \end{aligned}$$

Since $v_{n}\rightarrow v\not \equiv 0$ and $K>1$, we reach a contradiction. Hence, $\{y_{n}\}_{n\in \mathbb {N}}$ is bounded in $\mathbb {R}^{N}$ and, up to a subsequence, we can assume that $y_{n}\rightarrow y_{0}$. If $y_{0}\notin \overline{\Lambda }$, we can proceed as above to get $v_{n}\rightarrow 0$ in $\mathbb {Y}_{V_0}$. Then, $y\in \overline{\Lambda }$. Now, suppose by contradiction that $V(y_{0})>V_{0}$. From ${\tilde{v}}_{n}\rightarrow {\tilde{v}}$ in $\mathbb {Y}_{V_0}$, Fatou’s lemma and the invariance of $\mathbb {R}^{N}$ by translations, we have

$$\begin{aligned} d_{V_0}&=\mathcal {E}_{V_{0}}({\tilde{v}})< \liminf _{n\rightarrow \infty } \Biggl ( \frac{1}{p} [{\tilde{v}}_{n}]_{s, p}^{p}+\frac{1}{q} [{\tilde{v}}_{n}]_{s, q}^{q}+\int _{\mathbb {R}^{N}}V(\varepsilon _{n} x+y_{n}) \\&\quad \times \left( \frac{1}{p}|{\tilde{v}}_{n}|^{p}+\frac{1}{q}|{\tilde{v}}_{n}|^{q}\right) \, dx- \int _{\mathbb {R}^{N}} \left( F({\tilde{v}}_{n})+\frac{1}{q^{*}_{s}} ({\tilde{v}}_{n}^+)^{q^{*}_{s}}\right) \, dx \Biggr ) \nonumber \\&\le \liminf _{n\rightarrow \infty } \mathcal {J}_{\varepsilon _{n}}(t_{n}u_{n}) \le \liminf _{n\rightarrow \infty } \mathcal {J}_{\varepsilon _{n}} (u_{n})=d_{V_0} \end{aligned}$$

which gives a contradiction. Therefore, $V(y_{0})=V_{0}$ and $y_{0}\in \overline{\Lambda }$. The assumption $(V_2)$ ensures that $y_{0}\notin \partial \Lambda$ and thus $y_{0}\in M$. $\square$

Let us define

$$\begin{aligned} {\widetilde{\mathcal {N}}}_{\varepsilon }=\left\{ u\in \mathcal {N}_{\varepsilon }: \mathcal {J}_{\varepsilon }(u)\le d_{V_0}+\pi (\varepsilon )\right\} , \end{aligned}$$

where $\pi (\varepsilon )=\sup _{y\in M}|\mathcal {J}_{\varepsilon }(\Phi _{\varepsilon }(y))-d_{V_0}|$. By Lemma 5.1, we know that $\pi (\varepsilon )\rightarrow 0$ as $\varepsilon \rightarrow 0$. By the definition of $\pi (\varepsilon )$, we have that, for all $y\in M$ and $\varepsilon >0$, $\Phi _{\varepsilon }(y)\in {\widetilde{\mathcal {N}}}_{\varepsilon }$ and thus ${\widetilde{\mathcal {N}}}_{\varepsilon }\ne \emptyset$. Now we prove an interesting relation between $\widetilde{\mathcal {N}}_{\varepsilon }$ and the barycenter map.

Lemma 5.3

For any $\delta >0$,

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} \sup _{u\in \widetilde{\mathcal {N}}_{\varepsilon }} \mathrm{dist}(\beta _{\varepsilon }(u), M_{\delta })=0. \end{aligned}$$

Proof

Let $\varepsilon _{n}\rightarrow 0$ as $n\rightarrow \infty$. Then there is $\{u_{n}\}_{n\in \mathbb {N}}\subset {\widetilde{\mathcal {N}}}_{\varepsilon _{n}}$ such that

$$\begin{aligned} \mathrm{dist}(\beta _{\varepsilon _{n}}(u_{n}), M_{\delta })=\sup _{u\in {\widetilde{\mathcal {N}}}_{\varepsilon _{n}}} \mathrm{dist}(\beta _{\varepsilon _{n}}(u), M_{\delta })+o_{n}(1). \end{aligned}$$

Then, it is enough to find $\{y_{n}\}_{n\in \mathbb {N}}\subset M_{\delta }$ such that

$$\begin{aligned} \lim _{n\rightarrow \infty } |\beta _{\varepsilon _{n}}(u_{n})-y_{n}|=0. \end{aligned}$$

From $\mathcal {E}_{V_{0}}(tu_{n})\le \mathcal {J}_{\varepsilon _{n}}(tu_{n})$ for all $t\ge 0$, and $\{u_{n}\}_{n\in \mathbb {N}}\subset {\widetilde{\mathcal {N}}}_{\varepsilon _{n}}\subset \mathcal {N}_{\varepsilon _{n}}$, we obtain

$$\begin{aligned} d_{V_{0}}\le c_{\varepsilon _{n}}\le \mathcal {J}_{\varepsilon _{n}}(u_{n})\le d_{V_{0}}+h(\varepsilon _{n}) \end{aligned}$$

which leads to $\mathcal {J}_{\varepsilon _{n}}(u_{n})\rightarrow d_{V_{0}}$. By invoking Lemma 5.2, there exists $\{{\tilde{y}}_{n}\}\subset \mathbb {R}^{N}$ such that $y_{n}=\varepsilon _{n}{\tilde{y}}_{n}\in M_{\delta }$ for n large enough. Hence,

$$\begin{aligned} \beta _{\varepsilon _{n}}(u_{n})=y_{n}+\frac{\displaystyle {\int _{\mathbb {R}^{N}}\left( \varUpsilon (\varepsilon _{n}z+y_{n})-y_{n}\right) (|u_{n}(z+{\tilde{y}}_{n})|^{p}+ |u_{n}(z+{\tilde{y}}_{n})|^{q}) \, dz}}{\displaystyle {\int _{\mathbb {R}^{N}} (|u_{n}(z+{\tilde{y}}_{n})|^{p}+|u_{n}(z+{\tilde{y}}_{n})|^{q}) \, dz}}. \end{aligned}$$

Since $u_{n}(\cdot +{\tilde{y}}_{n})$ strongly converges in $\mathbb {Y}_{V_{0}}$ and $\varepsilon _{n}z+y_{n}\rightarrow y\in M_{\delta }$ for all $z\in \mathbb {R}^N$, we can see that $\beta _{\varepsilon _{n}}(u_{n})=y_{n}+o_{n}(1)$. The proof of the lemma is now complete. $\square$

We finish this section by presenting a relation between the topology of M and the number of solutions of the modified problem (3.1). Since $\mathbb {S}^{+}_{\varepsilon }$ is not a complete metric space, we cannot use directly an abstract result as in [1]. However, we invoke the abstract category result in [34] to accomplish our goal.

Theorem 5.1

Assume that $(V_1)$-$(V_2)$ and $(f_1)$-$(f_4)$ are in force. Then, for any given $\delta >0$ such that $M_{\delta }\subset \Lambda$, there exists ${\bar{\varepsilon }}_\delta >0$ such that, for any $\varepsilon \in (0, {\bar{\varepsilon }}_\delta )$, problem (3.1) has at least $cat_{M_{\delta }}(M)$ positive solutions.

Proof

For each $\varepsilon >0$, we consider the map $\alpha _{\varepsilon } : M \rightarrow \mathbb {S}_{\varepsilon }^{+}$ by setting $\alpha _{\varepsilon }(y)= m_{\varepsilon }^{-1}(\Phi _{\varepsilon }(y))$. By Lemma 5.1,

$$\begin{aligned} \lim _{\varepsilon \rightarrow 0} \psi _{\varepsilon }(\alpha _{\varepsilon }(y)) = \lim _{\varepsilon \rightarrow 0} \mathcal {J}_{\varepsilon }(\Phi _{\varepsilon }(y))= d_{V_0} \text{ uniformly } \text{ in } y\in M. \end{aligned}$$

(5.11)

Then, there exists ${\hat{\varepsilon }}>0$ such that the set $\widetilde{\mathcal {S}}^{+}_{\varepsilon }=\{ w\in \mathbb {S}_{\varepsilon }^{+} : \psi _{\varepsilon }(w) \le d_{V_0} + \pi _0(\varepsilon )\}$ is nonempty for all $\varepsilon \in (0, {\hat{\varepsilon }})$, since $\psi _{\varepsilon }(M)\subset \widetilde{\mathcal {S}}^{+}_{\varepsilon }$. Here $\pi _0(\varepsilon )=\sup _{y\in M}|\psi _{\varepsilon }(\alpha _{\varepsilon }(y))-d_{V_0}|\rightarrow 0$ as $\varepsilon \rightarrow 0$. From the above considerations, and taking into account Lemmas 5.1, 3.2-(iii), 5.3 and (5.9), we can find ${\bar{\varepsilon }}= {\bar{\varepsilon }}_{\delta }>0$ such that the following diagram is well-defined for any $\varepsilon \in (0, {\bar{\varepsilon }})$:

$$\begin{aligned} M{\mathop {\rightarrow }\limits ^{\Phi _{\varepsilon }}} \Phi _{\varepsilon }(M) {\mathop {\rightarrow }\limits ^{m_{\varepsilon }^{-1}}} \alpha _{\varepsilon }(M){\mathop {\rightarrow }\limits ^{m_{\varepsilon }}} \Phi _{\varepsilon }(M) {\mathop {\rightarrow }\limits ^{\beta _{\varepsilon }}} M_{\delta }. \end{aligned}$$

From (5.9), for $\varepsilon >0$ small enough, we can choose a function $\theta (\varepsilon , y)$, with $|\theta (\varepsilon , y)|<\frac{\delta }{2}$ uniformly in $y\in M$, such that $\beta _{\varepsilon }(\Phi _{\varepsilon }(y))= y+ \theta (\varepsilon , y)$ for all $y\in M$. Let $H(t, y)= y+ (1-t)\theta (\varepsilon , y)$, with $(t, y)\in [0,1]\times M$. Then $H: [0,1]\times M\rightarrow M_{\delta }$ is continuous. Obviously, $H(0, y)=\beta _{\varepsilon }(\Phi _{\varepsilon }(y))$ and $H(1, y)=y$ for all $y\in M$. Hence, H(t, y) is a homotopy between $\beta _{\varepsilon } \circ \Phi _{\varepsilon } = (\beta _{\varepsilon } \circ m_{\varepsilon }) \circ (m_{\varepsilon }^{-1}\circ \Phi _{\varepsilon })$ and the inclusion map $id: M \rightarrow M_{\delta }$, and we get

$$\begin{aligned} cat_{\alpha _{\varepsilon }(M)} \alpha _{\varepsilon }(M)\ge cat_{M_{\delta }}(M). \end{aligned}$$

(5.12)

Using Corollary 3.1, Lemma 4.4, and Theorem 27 in [34], with $c= c_{\varepsilon }\le d_{V_0}+\pi _0(\varepsilon ) =d$ and $K= \alpha _{\varepsilon }(M)$, we deduce that $\psi _{\varepsilon }$ has at least $cat_{\alpha _{\varepsilon }(M)} \alpha _{\varepsilon }(M)$ critical points on $\widetilde{\mathcal {S}}^{+}_{\varepsilon }$. Therefore, by Proposition 3.1-(d) and (5.12), we see that $\mathcal {J}_{\varepsilon }$ admits at least $cat_{M_{\delta }}(M)$ critical points in $\widetilde{\mathcal {N}}_{\varepsilon }$. $\square$

6 Proof of Theorem 1.1

In this section we provide the proof of Theorem 1.1. First, we prove an auxiliary result which will be crucial in the study of behavior of the maximum points of solutions to (1.1).

Lemma 6.1

Let $\varepsilon _{n}\rightarrow 0$ and $u_{n}\in \widetilde{\mathcal {N}}_{\varepsilon _{n}}$ be a solution to (3.1). Then $\mathcal {J}_{\varepsilon _{n}}(u_{n})\rightarrow d_{V_{0}}$, and there exists $\{{\tilde{y}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ such that $v_{n}=u_{n}(\cdot +{\tilde{y}}_{n})\in L^{\infty }(\mathbb {R}^{N})$ and for some $C>0$ it holds

$$\begin{aligned} |v_{n}|_{\infty }\le C \quad \text{ for } \text{ all } n\in \mathbb {N}. \end{aligned}$$

Moreover,

$$\begin{aligned} v_{n}(x)\rightarrow 0 \text{ as } |x|\rightarrow \infty \text{ uniformly } \text{ in } n\in \mathbb {N}. \end{aligned}$$

(6.1)

Proof

Since $\mathcal {J}_{\varepsilon _{n}}(u_{n})\le d_{V_{0}}+\pi (\varepsilon _{n})$ with $\pi (\varepsilon _{n})\rightarrow 0$ as $n\rightarrow \infty$, we can repeat the same arguments used in the proof of Lemma 5.3 to show that $\mathcal {J}_{\varepsilon _{n}}(u_{n})\rightarrow d_{V_{0}}$. Then, applying Lemma 5.2, there exists $\{{\tilde{y}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ such that $v_{n}=u_{n}(\cdot +{\tilde{y}}_{n})$ strongly converges in $\mathbb {Y}_{V_{0}}$ and $\varepsilon _{n}{\tilde{y}}_{n}\rightarrow y_{0}\in M$. Arguing as in the proof of Lemma 4.1 in [8], we deduce that $|v_{n}|_{\infty }\le C$ uniformly in $n\in \mathbb {N}$. Now, we note that $v_{n}$ is such that

$$\begin{aligned} (-\Delta )^{s}_{p}v_{n}+(-\Delta )^{s}_{q}v_{n}=h_{n} \, \text{ in } \mathbb {R}^{N}, \end{aligned}$$

where

$$\begin{aligned} h_{n}=-V(\varepsilon _{n}x+\varepsilon _{n}{\tilde{y}}_{n}) (v_{n}^{p-1}+v_{n}^{q-1})+g(\varepsilon _{n}x+\varepsilon _{n}{\tilde{y}}_{n}, v_{n}). \end{aligned}$$

Using the growth assumptions on g, Corollary 2.1 in [8] (and Theorem 2.2 in [22] when $p<2$), the fact that $\{v_{n}\}_{n\in \mathbb {N}}$ is uniformly bounded in $L^{\infty }(\mathbb {R}^{N})\cap \mathbb {Y}_{V_{0}}$, we infer that $v_{n}(x)\rightarrow 0$ as $|x|\rightarrow \infty$ uniformly in $n\in \mathbb {N}$. $\square$

6.1 Proof of Theorem 1.1

Take $\delta >0$ such that $M_\delta \subset \Lambda$. We first prove that there exists ${\tilde{\varepsilon }}_{\delta }>0$ such that, for any $\varepsilon \in (0, {\tilde{\varepsilon }}_{\delta })$ and any solution $u_{\varepsilon } \in \widetilde{\mathcal {N}}_{\varepsilon }$ of (3.1), it holds

$$\begin{aligned} |u_{\varepsilon }|_{L^{\infty }(\Lambda _{\varepsilon }^{c})}<a. \end{aligned}$$

(6.2)

Assume by contradiction that, for some sequence $\varepsilon _{n}\rightarrow 0$, we can obtain $u_{n}=u_{\varepsilon _{n}}\in \widetilde{\mathcal {N}}_{\varepsilon _{n}}$ such that $\mathcal {J}'_{\varepsilon _{n}}(u_{\varepsilon _{n}})=0$ and

$$\begin{aligned} |u_{n}|_{L^{\infty }(\Lambda _{\varepsilon _{n}}^{c})}\ge a. \end{aligned}$$

(6.3)

As in the proof of Lemma 5.2, we can see that $\mathcal {J}_{\varepsilon _{n}}(u_{n}) \rightarrow d_{V_0}$ and therefore we can apply Lemma 5.2 to find $\{{\tilde{y}}_{n}\}_{n\in \mathbb {N}}\subset \mathbb {R}^{N}$ such that $v_{n}=u_{n}(\cdot +{\tilde{y}}_{n})\rightarrow v$ in $\mathbb {Y}_{V_{0}}$ and $\varepsilon _{n}{\tilde{y}}_{n}\rightarrow y_{0} \in M$.

Take $r>0$ such that $B_{r}(y_{0})\subset B_{2r}(y_{0})\subset \Lambda$. Then, $B_{\frac{r}{\varepsilon _{n}}}(\frac{y_{0}}{\varepsilon _{n}})\subset \Lambda _{\varepsilon _{n}}$. Moreover, for any $y\in B_{\frac{r}{\varepsilon _{n}}}({\tilde{y}}_{n})$, it holds

$$\begin{aligned} \left| y - \frac{y_{0}}{\varepsilon _{n}}\right| \le |y- {\tilde{y}}_{n}|+ \left| {\tilde{y}}_{n} - \frac{y_{0}}{\varepsilon _{n}}\right|<\frac{1}{\varepsilon _{n}}(r+o_{n}(1))<\frac{2r}{\varepsilon _{n}}\quad \text{ for } n \text{ sufficiently } \text{ large. } \end{aligned}$$

For these values of n, we have

$$\begin{aligned} \Lambda _{\varepsilon _{n}}^{c}\subset B_{\frac{r}{\varepsilon _{n}}}^{c}({\tilde{y}}_{n}). \end{aligned}$$

By (6.1), we can find $R>0$ such that

$$\begin{aligned} v_{n}(x)<a \quad \text{ for } \text{ any } |x|\ge R \, , n\in \mathbb {N}, \end{aligned}$$

and thus

$$\begin{aligned} u_{n}(x)<a \quad \text{ for } \text{ any } x\in B_{R}^{c}({\tilde{y}}_{n})\, , n\in \mathbb {N}. \end{aligned}$$

On the other hand, there exists $\nu \in \mathbb {N}$ such that, for any $n\ge \nu$,

$$\begin{aligned} \Lambda ^{c}_{\varepsilon _{n}}\subset B^{c}_{\frac{r}{\varepsilon _{n}}}({\tilde{y}}_{n})\subset B^{c}_{R}({\tilde{y}}_{n}). \end{aligned}$$

Hence, $u_{n}(x)<a$ for any $x\in \Lambda ^{c}_{\varepsilon _{n}}$ and $n\ge \nu$, which contradicts (6.3).

Let ${\bar{\varepsilon }}_{\delta }>0$ be given by Theorem 5.1 and set $\varepsilon _{\delta }= \min \{{\tilde{\varepsilon }}_{\delta }, {\bar{\varepsilon }}_{\delta }\}$. Take $\varepsilon \in (0, \varepsilon _{\delta })$. Applying Theorem 5.1, we obtain at least $cat_{M_{\delta }}(M)$ positive solutions to (3.1). If $u_{\varepsilon }$ is one of these solutions, we have that $u_{\varepsilon }\in \widetilde{\mathcal {N}}_{\varepsilon }$, and we can use (6.2) and the definition of g to deduce that $g(\varepsilon x, u_{\varepsilon })=f(u_{\varepsilon }) + u_{\varepsilon }^{q^{*}_{s}-1}$. This means that $u_{\varepsilon }$ is also a solution of (1.1). Consequently, (1.1) admits at least $cat_{M_{\delta }}(M)$ positive solutions. Now we consider $\varepsilon _{n}\rightarrow 0$ and take a sequence $\{u_{n}\}_{n\in \mathbb {N}}\subset \mathbb {X}_{\varepsilon _{n}}$ of solutions to (1.1) as above. Let us investigate the behavior of the maximum points of $u_{n}$. By the definition of g and $(g_{1})$, there exists $\sigma \in (0, a)$ such that

$$\begin{aligned} g(\varepsilon x, t)t\le \frac{V_{0}}{K} (t^{p}+t^{q}) \quad \text{ for } x\in \mathbb {R}^{N}, 0\le t\le \sigma . \end{aligned}$$

(6.4)

As before, we can take $R>0$ such that

$$\begin{aligned} |u_{n}|_{L^{\infty }(B^{c}_{R}({\tilde{y}}_{n}))}<\sigma . \end{aligned}$$

(6.5)

Up to a subsequence, we may also assume that

$$\begin{aligned} |u_{n}|_{L^{\infty }(B_{R}({\tilde{y}}_{n}))}\ge \sigma . \end{aligned}$$

(6.6)

Otherwise, if (6.6) fails, we have $|u_{n}|_{\infty }<\sigma$. Then, in view of $\langle \mathcal {J}'_{\varepsilon _{n}}(u_{n}), u_{n}\rangle =0$ and (6.4), we get

$$\begin{aligned} \Vert u_{n}\Vert _{V_{\varepsilon _{n}},p}^{p}+\Vert u_{n}\Vert _{V_{\varepsilon _{n}},q}^{q}\le \int _{\mathbb {R}^{N}} g(\varepsilon _{n} x, u_{n}) u_{n} \,dx\le \frac{V_{0}}{K} \int _{\mathbb {R}^{N}} (|u_{n}|^{p}+|u_{n}|^{q}) \, dx \end{aligned}$$

which gives a contradiction. Therefore, (6.6) is true. In light of (6.5) and (6.6), we deduce that if $p_{n}$ is a global maximum point of $u_{n}$, then $p_{n}={\tilde{y}}_{n}+q_{n}$ for some $q_{n}\in B_{R}(0)$. Since $\varepsilon _{n}{\tilde{y}}_{n}\rightarrow y_{0}\in M$ and $|q_{n}|<R$ for all $n\in \mathbb {N}$, we see that $\varepsilon _{n}p_{n}\rightarrow y_{0}$, and using the continuity of V we achieve

$$\begin{aligned} \lim _{n\rightarrow \infty }V(\varepsilon _{n}p_{n})= V(y_{0})=V_{0}. \end{aligned}$$

The proof of Theorem 1.1 is now complete. $\square$

7 The Supercritical Case

In this section we deal with (1.5). First, we truncate the nonlinearity $\phi (u)=u^{\gamma -1}+\mu u^{\tau -1}$ in a suitable way. Let $K>0$ be a real number, whose value will be fixed later, and define

$$\begin{aligned} \phi _{\mu }(t)= {\left\{ \begin{array}{ll} 0 &{}\hbox { if } t<0, \\ t^{\gamma -1}+\mu t^{\tau -1} &{}\hbox { if } 0\le t<K, \\ (1+\mu K^{\tau -s})t^{\gamma -1} &{} \hbox { if}\ t \ge K. \end{array}\right. } \end{aligned}$$

It is easy to check that $\phi _{\mu }$ satisfies all the assumptions $(f_1)$-$(f_4)$ ($(f_3)$ with $\vartheta =\gamma >q$). Moreover,

$$\begin{aligned} \phi _{\mu }(t)\le (1+\mu K^{\tau -\gamma })t^{\gamma -1} \text{ for } \text{ all } t\ge 0. \end{aligned}$$

(7.1)

Let us now introduce the following truncated problem:

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s}_{p}u+(-\Delta )^{s}_{q}u + V(\varepsilon x) (u^{p-1} + u^{q-1})=\phi _{\mu }(u) \, \text{ in } \mathbb {R}^{N},\\ u\in W^{s, p}(\mathbb {R}^{N})\cap W^{s, q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}. \end{array} \right. \end{aligned}$$

(7.2)

Clearly, weak solutions of (7.2) are critical points of the energy functional $\mathcal {J}_{\varepsilon , \mu }: \mathbb {X}_{\varepsilon }\rightarrow \mathbb {R}$ given by

$$\begin{aligned} \mathcal {J}_{\varepsilon , \mu }(u)=\frac{1}{p} \Vert u\Vert _{V_{\varepsilon }, p}^{p}+\frac{1}{q}\Vert u\Vert _{V_{\varepsilon }, q}^{q}-\int _{\mathbb {R}^{N}} \Phi _{\mu }(u)\, dx, \end{aligned}$$

where $\Phi _{\mu }(t)=\int _{0}^{t} \phi _{\mu }(\xi ) d\xi$. We also consider the autonomous functional

$$\begin{aligned} \mathcal {J}_{0, \mu }(u)=\frac{1}{p}[u]_{s, p}^{p}+\frac{1}{q} [u]_{s, q}^{q}+V_{0}\left( \frac{1}{p} |u|_{p}^{p}+\frac{1}{q} |u|_{q}^{q} \right) -\int _{\mathbb {R}^{N}} \Phi _{\mu }(u)\, dx. \end{aligned}$$

Using Theorem 1.1 in [8], we know that for any $\mu \ge 0$ and $\delta >0$, there exists ${\bar{\varepsilon }}(\delta , \mu )>0$ such that, for any $\varepsilon \in (0, {\bar{\varepsilon }}(\delta , \mu ))$, problem (7.2) admits at least $cat_{M_{\delta }}(M)$ positive solutions $u_{\varepsilon , \mu }$. In the next lemma we estimate the $W^{s, q}$-norm of these solutions uniformly with respect to $\mu$.

Lemma 7.1

There exists ${\bar{C}}>0$ such that $\Vert u_{\varepsilon , \mu }\Vert _{V_{\varepsilon }, q}\le {\bar{C}}$ for any $\varepsilon >0$ sufficiently small and uniformly in $\mu$.

Proof

The proof of Theorem 1.1 implies that any solution $u_{\varepsilon , \mu }$ of (7.2) satisfies the following inequality

$$\begin{aligned} \mathcal {J}_{\varepsilon , \mu }(u_{\varepsilon , \mu })\le c_{0, \mu }+h_{\mu }(\varepsilon ), \end{aligned}$$

where $c_{0, \mu }$ is the mountain pass level related to the functional $\mathcal {J}_{0, \mu }$, and $h_{\mu }(\varepsilon )\rightarrow 0$ as $\varepsilon \rightarrow 0$. Then, decreasing ${\bar{\varepsilon }}(\delta , \mu )$ if necessary, we may suppose that

$$\begin{aligned} \mathcal {J}_{\varepsilon , \mu }(u_{\varepsilon , \mu })\le c_{0, \mu }+1 \end{aligned}$$

(7.3)

for any $\varepsilon \in (0, {\bar{\varepsilon }}(\delta , \mu ))$. Since $c_{0, \mu }\le c_{0, 0}$ for any $\mu \ge 0$, we have that

$$\begin{aligned} \mathcal {J}_{\varepsilon , \mu }(u_{\varepsilon , \mu })\le c_{0, 0}+1 \end{aligned}$$

(7.4)

for any $\varepsilon \in (0, {\bar{\varepsilon }}(\delta , \mu ))$. On the other hand,

$$\begin{aligned} \mathcal {J}_{\varepsilon , \mu }(u_{\varepsilon , \mu })&=\mathcal {J}_{\varepsilon , \mu }(u_{\varepsilon , \mu })-\frac{1}{\gamma }\langle \mathcal {J}'_{\varepsilon , \mu }(u_{\varepsilon , \mu }), u_{\varepsilon , \mu }\rangle \nonumber \\&=\left( \frac{1}{p}-\frac{1}{s}\right) \Vert u_{\varepsilon , \mu }\Vert ^{p}_{V_{\varepsilon }, p}+\left( \frac{1}{q}-\frac{1}{\gamma }\right) \Vert u_{\varepsilon , \mu }\Vert ^{q}_{V_{\varepsilon }, q}\nonumber \\&\quad +\int _{\mathbb {R}^{N}} \left( \frac{1}{\gamma } \phi _{\mu }(u_{\varepsilon , \mu })u_{\varepsilon , \mu }-\Phi _{\mu }(u_{\varepsilon , \mu })\right) \, dx \nonumber \\&\ge \left( \frac{1}{p}-\frac{1}{\gamma }\right) \Vert u_{\varepsilon , \mu }\Vert ^{p}_{V_{\varepsilon }, p}+\left( \frac{1}{q}-\frac{1}{\gamma }\right) \Vert u_{\varepsilon , \mu }\Vert ^{q}_{V_{\varepsilon }, q}, \end{aligned}$$

(7.5)

where in the last inequality we have used assumption $(f_3)$. In view of (7.4) and (7.5), we obtain that

$$\begin{aligned} \Vert u_{\varepsilon , \mu }\Vert _{V_{\varepsilon }, q}\le \left( \left( \frac{\gamma q}{\gamma -q} \right) (c_{0, 0}+1)\right) ^{\frac{1}{q}}={\bar{C}}. \end{aligned}$$

for any $\varepsilon \in (0, {\bar{\varepsilon }}(\delta , \mu ))$. This completes the proof of Lemma 7.1. $\square$

Now, we know that, for any $\varepsilon \in (0, {\bar{\varepsilon }}(\delta , \mu ))$, problem (7.2) possesses at least $cat_{M_{\delta }}(M)$ positive solutions. Let $u_{\varepsilon , \mu }$ be one of these solutions. We shall assume that ${\bar{\varepsilon }}(\delta , \mu )$ is small in such a way that the thesis of Lemma 7.1 is valid. Our goal is to show that $u_{\varepsilon , \mu }$ is a solution of the original problem (1.5) whenever $\mu$ is sufficiently small. More precisely, we will prove that there exists $K_{0}>0$ such that for any $K\ge K_{0}$, there exists $\mu _{0}=\mu _{0}(K)>0$ such that

$$\begin{aligned} |u_{\varepsilon , \mu }|_{\infty }\le K \quad \text{ for } \text{ all } \mu \in [0, \mu _{0}]. \end{aligned}$$

(7.6)

To see this, we use a Moser iteration argument [28]. For simplicity, we set $u=u_{\varepsilon , \mu }$. For any $L>0$, we define $u_{L}=\min \{u, L\}\ge 0$, where $\beta >1$ will be chosen later, and let $w_{L}=u u_{L}^{\beta -1}$. Taking $z_{L}=u_{L}^{q(\beta -1)}u$ in (7.2), we find

$$\begin{aligned}&\langle u, z_{L}\rangle _{s, p}+\langle u, z_{L}\rangle _{s, q} +\int _{\mathbb {R}^{N}} V(\varepsilon x) (u^{p}+u^{q}) u^{q(\beta -1)}_{L} \, dx \nonumber \\&= \int _{\mathbb {R}^{N}} \phi _{\mu }(u) uu^{q(\beta -1)}_{L}\, dx. \end{aligned}$$

(7.7)

On the other hand, arguing as at the beginning of the proof of Lemma 3.18 in [6] (see formula (85) there), we can see that, for $t\in \{p, q\}$,

$$\begin{aligned} \langle u, z_{L}\rangle _{s, t}\ge \frac{1}{\beta ^{t}}S_{t}|w_{L}|^{t}_{t^{*}_{s}}. \end{aligned}$$

(7.8)

Putting together (7.7), (7.1) and $(V_1)$, we deduce that

$$\begin{aligned} \frac{1}{\beta ^{q}}S_{q}|w_{L}|^{q}_{q^{*}_{s}} \le C_{\mu , K}\int _{\mathbb {R}^{N}} u^{\gamma }u^{q(\beta -1)}_{L}\, dx, \end{aligned}$$

(7.9)

where $C_{\mu , K}=1+\mu K^{\tau -\gamma }$. In light of (7.8) and (7.9), and applying the Hölder inequality, we have that

$$\begin{aligned} \begin{aligned} |w_{L}|_{q^{*}_{s}}^{q}&\le C_{1}C_{\mu , K} \beta ^{q} \int _{\mathbb {R}^{N}} u^{\gamma }u^{q(\beta -1)}_{L}\, dx \\&=C_{1}C_{\mu , K} \beta ^{q} \int _{\mathbb {R}^{N}} u^{\gamma -q}w^{q}_{L}\, dx \\&\le C_{1} \beta ^{q} C_{\mu , K} |u|^{\gamma -q}_{q^{*}_{s}} |w_{L}|^{q}_{\alpha ^{*}_{s}}, \end{aligned}\end{aligned}$$

(7.10)

where $C_{1}=S_{q}^{-1}>0$ and

$$\begin{aligned} \alpha ^{*}_{s}=\frac{qq^{*}_{s}}{q^{*}_{s}-(\gamma -q)}\in (q, q^{*}_{s}). \end{aligned}$$

By Lemma 7.1, Theorem 2.1 and (7.10), we get

$$\begin{aligned} |w_{L}|_{q^{*}_{s}}^{q}\le C_{2} \beta ^{q} C_{\mu , K} |w_{L}|_{\alpha ^{*}_{s}}^{q}, \end{aligned}$$

(7.11)

where $C_{2}=C_{1}S_{q}^{-\frac{\gamma -q}{q}}{\bar{C}}^{\gamma -q}$ is independent of $\varepsilon$ and $\mu$. Now, we observe that if $u^{\beta }\in L^{\alpha ^{*}_{s}}(\mathbb {R}^{N})$, by the definition of $w_{L}$, $u_{L}\le u$, and (7.11), we find

$$\begin{aligned} |w_{L}|_{q^{*}_{s}}^{q}\le C_{2} \beta ^{q} C_{\mu , K} |u|_{\beta \alpha ^{*}_{s}}^{q\beta }<\infty . \end{aligned}$$

(7.12)

Letting $L \rightarrow \infty$ in (7.12), by Fatou lemma we obtain

$$\begin{aligned} |u|_{q^{*}_{s}\beta }\le (C_{2} C_{\mu , K})^{\frac{1}{q\beta }} \beta ^{\frac{1}{\beta }} |u|_{\beta \alpha ^{*}_{s}} \end{aligned}$$

(7.13)

whenever $u^{\beta \alpha ^{*}_{s}}\in L^{1}(\mathbb {R}^{N})$.

Now, we set $\beta =\frac{q^{*}_{s}}{\alpha ^{*}_{s}}>1$, and observe that, since $u\in L^{q^{*}_{s}}(\mathbb {R}^{N})$, the above inequality is true for this choice of $\beta$. Then, using the fact that $\beta ^{2}\alpha ^{*}_{s}=q^{*}_{s}\beta$, it follows that (7.13) holds with $\beta$ replaced by $\beta ^{2}$. Consequently,

$$\begin{aligned} |u|_{q^{*}_{s}\beta ^{2}}\le (C_{2} C_{\mu , K})^{\frac{1}{q\beta ^2}} \beta ^{\frac{2}{\beta ^{2}}} |u|_{\beta ^{2} \alpha ^{*}_{s}}\le (C_{2} C_{\mu , K})^{\frac{1}{q}\left( \frac{1}{\beta }+\frac{1}{\beta ^{2}}\right) } \beta ^{\frac{1}{\beta }+\frac{2}{\beta ^{2}}} |u|_{\beta \alpha ^{*}_{s}}. \end{aligned}$$

Iterating this process and recalling that $\beta \alpha ^{*}_{s}=q^{*}_{s}$, we have that, for every $m\in \mathbb {N}$,

$$\begin{aligned} |u|_{q^{*}_{s}\beta ^{m}} \le (C_{2} C_{\mu , K})^{\sum _{j=1}^{m}\frac{1}{q\beta ^{j}}} \beta ^{\sum _{j=1}^{m} j\beta ^{-j}} |u|_{q^{*}_{s}}. \end{aligned}$$

(7.14)

Passing to the limit as $m \rightarrow \infty$ in (7.14) and exploiting Lemma 7.1, we arrive at

$$\begin{aligned} |u|_{\infty }\le (C_{2} C_{\mu , K})^{\zeta _{1}} \beta ^{\zeta _{2}}C_{3}, \end{aligned}$$

(7.15)

where $C_{3}=S_{q}^{-\frac{1}{q}}{\bar{C}}$ and

$$\begin{aligned} \zeta _{1}=\frac{1}{q}\sum _{j=1}^{\infty }\frac{1}{\beta ^{j}}<\infty \quad \text{ and } \quad \zeta _{2}=\sum _{j=1}^{\infty }\frac{j}{\beta ^{j}}<\infty . \end{aligned}$$

Next, we will find some suitable values of K and $\mu$ such that the following inequality holds

$$\begin{aligned} (C_{2} C_{\mu , K})^{\zeta _{1}} \beta ^{\zeta _{2}}C_{3}\le K, \end{aligned}$$

or equivalently,

$$\begin{aligned} 1+\mu K^{\tau -\gamma }\le C_{2}^{-1} \beta ^{-\frac{\zeta _{2}}{\zeta _{1}}} (KC_{3}^{-1})^{\frac{1}{\zeta _{1}}}. \end{aligned}$$

Take $K>0$ such that

$$\begin{aligned} \frac{(KC_{3}^{-1})^{\frac{1}{\zeta _{1}}}}{C_{2}\beta ^{\frac{\zeta _{2}}{\zeta _{1}}}}-1>0, \end{aligned}$$

and fix $\mu _{0}>0$ satisfying

$$\begin{aligned} \mu \le \mu _{0}\le \left( \frac{(KC_{3}^{-1})^{\frac{1}{\zeta _{1}}}}{C_{2}\beta ^{\frac{\zeta _{2}}{\zeta _{1}}}}-1\right) \frac{1}{K^{\tau -\gamma }}. \end{aligned}$$

Then, using (7.15), we infer that

$$\begin{aligned} |u|_{\infty }\le K \quad \text{ for } \text{ all } \mu \in [0, \mu _{0}], \end{aligned}$$

namely $u=u_{\varepsilon , \mu }$ is a solution of (1.5). This completes the proof of Theorem 1.2. $\square$

8 Final Comments

We conclude the paper with some comments which we believe useful for future developments. First we observe that the variational techniques used along the paper are rather flexible and can be adapted to study problems like (1.1) and involving the more general operator $(-\Delta )^{s_{1}}_{p}+(-\Delta )^{s_{2}}_{q}$, namely

$$\begin{aligned} \left\{ \begin{array}{ll} (-\Delta )^{s_{1}}_{p}u+(-\Delta )^{s_{2}}_{q}u + V(\varepsilon x) (u^{p-1} + u^{q-1})= f(u)+\gamma u^{q^{*}_{s_{2}}-1} \, \text{ in } \mathbb {R}^{N}, \\ u\in W^{s_{1}, p}(\mathbb {R}^{N})\cap W^{s_{2},q}(\mathbb {R}^{N}), \, u>0 \text{ in } \mathbb {R}^{N}, \end{array} \right. \end{aligned}$$

where $0<s_{1}\le s_{2}<1$, $1<p<q<\frac{N}{s_{2}}$, $\gamma \in \{0, 1\}$, V satisfies $(V_1)$-$(V_2)$ and $f\in C(\mathbb {R}, \mathbb {R})$ fulfills the following hypotheses:

$(f_{1})$:

$\displaystyle {\lim _{|t|\rightarrow 0} \frac{|f(t)|}{|t|^{p-1}}=0}$,

$(f_{2})$:

when $\gamma =0$, there exists $\nu \in (q, q^{*}_{s_{2}})$ such that $\displaystyle {\lim _{|t|\rightarrow \infty } \frac{|f(t)|}{|t|^{\nu -1}}=0}$,

$(f'_{2})$:

when $\gamma =1$, there exist $\sigma _{1}, \sigma _{2}\in (q, q^{*}_{s_{2}})$ and $\lambda >1$ such that

$$\begin{aligned} f(t)\ge \lambda t^{\sigma _{1}-1} \quad \text{ for } \text{ all } t>0, \quad \lim _{|t|\rightarrow \infty } \frac{|f(t)|}{|t|^{\sigma _{2}-1}}=0, \end{aligned}$$

$(f_{3})$:

there exists $\vartheta \in (q, q^{*}_{s_{2}})$ such that $\displaystyle {0<\vartheta F(t)= \vartheta \int _{0}^{t} f(\tau ) \, d\tau \le t f(t)}$ for all $t>0$,

$(f_{4})$:

the map $t\in (0, \infty )\mapsto \displaystyle {\frac{f(t)}{t^{q-1}}}$ is increasing.

In this case, we consider the space

$$\begin{aligned} \mathbb {X}_{\varepsilon }=\left\{ u\in W^{s_{1}, p}(\mathbb {R}^{N})\cap W^{s_{2}, q}(\mathbb {R}^{N}) : \int _{\mathbb {R}^{N}} V(\varepsilon x) \left( |u|^{p}+|u|^{q}\right) \,dx<\infty \right\} \end{aligned}$$

endowed with the norm

$$\begin{aligned} \Vert u\Vert _{\mathbb {X}_{\varepsilon }}= \Vert u\Vert _{V_{\varepsilon }, s_{1}, p} + \Vert u\Vert _{V_{\varepsilon }, s_{2}, q}, \end{aligned}$$

where

$$\begin{aligned} \Vert u\Vert _{V_{\varepsilon }, s_{i}, t}= \left( [u]_{s_{i}}^{t} + \int _{\mathbb {R}^{N}} V(\varepsilon x) |u|^{t}\, dx\right) ^{\frac{1}{t}} \quad \text{ for } t\in \{p, q\}, \, i=1, 2. \end{aligned}$$

We point out that, when $\gamma =1$, $s_{1}<s_{2}$ and f satisfies $(f_1)$, $(f'_2)$, $(f_3)$ and $(f_4)$, the energy functional $\mathcal {J}_{\varepsilon }$ satisfies the $(PS)_{c}$ condition at any level $c<\left( \frac{1}{\vartheta }-\frac{1}{q^{*}_{s_{2}}}\right) S_{*}^{\frac{N}{s_{2}}}$ (let us note that for studying (3.8), we only consider the space $W^{s_{2}, q}_{0}(\Lambda )$ because $W^{s_{2}, q}_{0}(\Lambda )\subset W^{s_{1}, p}_{0}(\Lambda )$ as showed in [10]).

Finally, in this paper we have investigated nonlocal problems of anisotropic type, in the non-homogeneous setting (the right-hand side does not vanish). These have been studied, in the local case, in the recent work [14]. In this last paper more general cases are considered, as for instance the g-Laplacian case, that is, in the variational case, minima of functionals of the type

$$\begin{aligned} w\mapsto \int _{\Omega } [G(x, |Dw|)-fw]\, dx, \end{aligned}$$

where, for every fixed x, it holds

$$\begin{aligned} p<\frac{G'(x, t)t}{G(x, t)}<q \, \text{ for } \text{ every } t>0. \end{aligned}$$

(8.1)

In our context, this would correspond to take $G(x, t)=t^{p}+t^{q}$. In the same way in the literature are considered functionals of the type

$$\begin{aligned} w\mapsto \int _{\Omega } [G_{1}(x, |Dw|)+G_{2}(x, |Dw|)-fw]\, dx, \end{aligned}$$

where $G_1$ and $G_2$ are two different functions satisfying (8.1). The fractional analog of this setting is given by functionals of the type

$$\begin{aligned} w\mapsto \iint _{\mathbb {R}^{2N}} \left[ G_{1}\left( \frac{|w(x)-w(y)|}{|x-y|^{s}}\right) +G_{2}\left( \frac{|w(x)-w(y)|}{|x-y|^{s}} \right) \right] \frac{dxdy}{|x-y|^{N}} \end{aligned}$$

that would be the natural driving energy to combine with the lower order terms making the problem non-homogeneous. In this situation, we need to modify the conditions on the nonlinearity f as in [1]. We will address these questions in a future paper.