Abstract
For Euclidean quantum field theories, Holland and Hollands have shown operator product expansion (OPE) coefficients satisfy “flow equations”: For interaction parameter \({{\lambda }}\), the partial derivative of any OPE coefficient with respect to \({{\lambda }}\) is given by an integral over Euclidean space of a sum of products of other OPE coefficients. In this paper, we generalize these results for flat Euclidean space to curved Lorentzian spacetimes in the context of the solvable “toy model” of massive Klein–Gordon scalar field theory, with \({m}^{{2}}\) viewed as the “self-interaction parameter”. Even in Minkowski spacetime, a serious difficulty arises from the fact that all integrals must be taken over a compact spacetime region to ensure convergence but any integration cutoff necessarily breaks Lorentz covariance. We show how covariant flow relations can be obtained by adding compensating “counterterms” in a manner similar to that of the Epstein–Glaser renormalization scheme. We also show how to eliminate dependence on the “infrared-cutoff scale” L, thereby yielding flow relations compatible with almost homogeneous scaling of the fields. In curved spacetime, the spacetime integration will cause the OPE coefficients to depend non-locally on the spacetime metric, in violation of the requirement that quantum fields should depend locally and covariantly on the metric. We show how this potentially serious difficulty can be overcome by replacing the metric with a suitable local polynomial approximation about the OPE expansion point. We thereby obtain local and covariant flow relations for the OPE coefficients of Klein–Gordon theory in curved Lorentzian spacetimes. As a byproduct of our analysis, we prove the field redefinition freedom in the Wick fields (i.e. monomials of the scalar field and its covariant derivatives) can be characterized by the freedom to add a smooth, covariant, and symmetric function \({F}_{{n}} ({x}_{{1}},\ldots ,{x}_{{n}};{z})\) to the identity OPE coefficients, \({C}^{{I}}_{{{\phi \cdots \phi }}}({x}_{{1}},\ldots ,{x}_{{n}};{z})\), for the elementary n-point products. We thereby obtain an explicit construction of any renormalization prescription for the nonlinear Wick fields in terms of the OPE coefficients \({C}^{{I}}_{{{\phi \cdots \phi }}}\). The ambiguities inherent in our procedure for modifying the flow relations are shown to be in precise correspondence with the field redefinition freedom of the Klein–Gordon OPE coefficients. In an appendix, we develop an algorithm for constructing local and covariant flow relations beyond our “toy model” based on the associativity properties of OPE coefficients. We illustrate our method by applying it to the flow relations of \({{\lambda \phi }}^{{4}}\)-theory.

Similar content being viewed by others
Data Availability
Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.
Notes
To any finite perturbative order, the dimension defined in [9, Eq. 10] coincides with the standard “engineering dimension” given in our “Notation and Conventions” at the end of this section.
In the discussion below, the cancellation of non-integrable divergences at \(y=x_{i}\) (for \(i=1,\dots ,n\)) is equivalent to the statement that the distribution appearing in the integrand of (1.2) is uniquely “extendable” to the “partial diagonals” involving y and any single \(x_{i}\)-point.
As we shall see in Sect. 3.2, all other OPE coefficients are determined by \(C_{\phi \cdots \phi }^{I}(x_{1},\dots ,x_{n};z)\), so it suffices to consider only the flow relations for these coefficients.
Retarded and advanced products also satisfy the Green’s function properties.
A similar formula holds for the case of a general definition of Wick powers, with the only difference being the presence of additional terms containing factors of the smooth functions \(F_{k}\) that parameterize the field-redefinition freedom of Wick fields.
The coefficient \(C_{T_{0}\{A_{i}\}}^{D}=C_{A_{i}}^{D}\) involving a single field factor is given by the geometric factors that appear in an ordinary Taylor expansion [see (E.25)].
This analysis would require an understanding of what field-redefinition freedom is allowed for the non-perturbative interacting theory. Note also that we have not attempted to eliminate the L-dependence of the flow relations (1.10). The techniques described in Sect. 4.2 can be used to eliminate the L-dependence of (1.10) to any finite order in perturbation theory, but it is not obvious how to remove the L-dependence non-perturbatively.
i.e., \(\sigma \) is equal to twice the “Synge bi-scalar/world function”.
More precisely, all of the derivatives of U and V at coincidence \(x_{1}=x_{2}\) are uniquely as well as locally and covariantly determined by the fact that \(K\Psi _{2}=\text {smooth}\), with the Klein–Gordon operator K, see Eq. (2.3), acting on either spacetime variable.
The proof in [30] used an analytic dependence assumption in place of the joint smoothness condition of [31] that we have used here in our formulation of \(\text {W2}\). In order to prove that \(\text {W2}\) holds for the Hadamard normal ordered prescription, we would need to show that the Hadamard normal ordered n-point functions, \({\langle {:\phi (x_1)\cdots \phi (x_n):_H}\rangle }_\Psi \), are jointly smooth in the required sense. We do not anticipate any difficulties in proving this but, as far as we are aware, a proof has not been given in the literature.
The corresponding result was obtained in [23, Theorem 5.1] by imposing an additional analytic variation requirement, which we do not impose here.
Of course, for any finite \(|\alpha |\), this identity could (with substantial computational labor) alternatively be directly derived from the values of the differentiated geodesic distance function \(\sigma (x,z)\) at coincidence \(x=z\). In global inertial coordinates in flat spacetime, the identity (2.60) holds if and only if,
$$\begin{aligned} \nabla _{\alpha }^{(y)}S^{\beta }(x;z)|_{x=z}=\delta _{\alpha }^{\beta }, \end{aligned}$$(2.62)since covariant derivatives commute in this case. Note the identity (2.61) can be directly verified using formula (2.59) for \(S^{\beta }\) in flat spacetime. However, in curved spacetime, the left-hand side of (2.60) receives non-trivial contributions which depend on the curvature tensor from \(|\beta |<|\alpha |\).
In any RNC system, it can be deduced from the geodesic equation for geodesics passing through the origin that
$$\begin{aligned} \partial _{\smash {(}\sigma _{1}}^{}\cdots \partial ^{}_{\sigma _{n}}\Gamma _{\mu \nu \smash {)}}^{\kappa }(x)|_{x=\vec {0}}=0, \end{aligned}$$(2.65)with \(\Gamma ^{\kappa }_{\mu \nu }\) denoting the Christoffel symbols. For scalar fields evaluated at the origin, the equivalence between partial derivatives and totally-symmetrized covariant derivatives can then be inductively established for all n using (2.65).
If the scaling degree varies for different background geometries, then [A] is equal to the supremum of the right-hand side with respect to \((M,g_{ab})\). If \(\Phi _{A}\) is tensor-valued, then the maximum scaling degree of the tensor components is used.
It was proven in [29, Lemma III.1] that (3.6) is smooth if and only if \(\Psi \) is Hadamard and the truncated n-point functions of \(\Psi \) are smooth. However, Sanders later proved that all Hadamard states have smooth truncated n-point functions [37, Proposition 3.1.14] and, therefore, (3.6) is smooth for all Hadamard \(\Psi \) and only Hadamard \(\Psi \).
For \(n=2\), we omit the \({\mathcal {T}}\) symbol since there is only one possible merger tree in this case.
The terminology is borrowed from graph theory: The elements of \({\mathcal {S}}\) can be viewed as labeling the vertices of a graph (see e.g. Fig. 1 below). An arrow connecting two vertices of this graph corresponds then to a pairing between two elements of \({\mathcal {S}}\). A “perfect matching” is achieved when every vertex is connected to exactly one arrow and there are no loops (connecting a vertex to itself): i.e., every element of \({\mathcal {S}}\) is paired with precisely one other element of \({\mathcal {S}}\).
The only method we have provided for computing coefficients of the form \(C_{A_{1}\cdots A_{n}}^{I}\) from \(C_{\phi \cdots \phi }^{I}\) is via formula (3.43) of Proposition 4. However, coefficients \(C_{A_{1}\cdots A_{n}}^{B}\)for \(B\ne I\) may be computed from \(C_{\phi \cdots \phi }^{I}\) either via formula (3.43) or, alternatively, by plugging the values of \(C_{A_{1}\cdots A_{n}}^{I}\) back into formula (3.32) of Proposition 3.
The “diagonals” are the subset of the product manifold, \({\{ (x_{1},\dots , x_{n})\in \times ^{n}M\, |}{\;x_{i}=x_{j}} \text{ for } \text{ any } i,j\in \{1,\dots ,n\}\}\). Thus, “away from the diagonals” means when all points are distinct.
The precise asymptotic behavior of the distribution kernel for \(H_{F}\) is obtained by replacing the “\(i\epsilon \)-prescription” in the expression (2.26) for H with the usual Feynman prescription: i.e., making the following substitution,
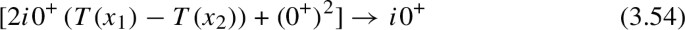
Defining products of Euclidean Wick fields on diagonals generally requires renormalization analogous to extending the Lorentzian unextended time-ordered products to their diagonals and, thus, is subject to additional contact-term renormalization ambiguities. See also Footnote 32.
This is established by a straightforward application of [35, Theorem 8.2.12] In fact, as explained in the next section, this property holds for any translation invariant bi-distribution.
Whereas the wavefront set calculus implies the pointwise products of any Lorentzian Hadamard parametrix H appearing in formula (3.25) are guaranteed to be well-defined as distributions, the corresponding pointwise products of \(G_{E}\) are generally ill-defined as distributions on diagonals, since the wavefront set (4.4) of \(G_E\) is identical to the wavefront set of the two-variable Dirac delta function. In this respect, \(G_E\) is more analogous to a Feynman parametrix \(H_F\) rather than to H; see also the discussion following Eq. (3.61).
We have restricted to the case \(m^{2}>0\) here, but it is worth noting that for \(m^{2}=0\), the fall-off of \(G_{E}\) is too slow to justify the integration by parts.
Since only the asymptotic behavior of the parametrix is relevant for the OPE coefficients, we have replaced the equality symbol in (4.18) with the weaker relation “\(\sim _{\delta }\)” which implies both sides are asymptotically equivalent to an arbitrary scaling degree \(\delta \).
The product over \({\mathcal {L}}_{\Delta }\)-operators with different \(\Delta \)-values is needed to account for the dependence of the Wick OPE coefficients on the dimensionful parameter \(m^{2}\). In a theory without dimensionful parameters, we could eliminate the L-dependence of a flow relation by simply using the operator \({\mathfrak {L}}={\mathcal {L}}_{\Delta }\) with \(\Delta \) corresponding to the conformal scaling dimension of the flow relation (Note that \(\Delta \) would generally depend on the renormalized coupling parameter in an interacting theory).
Note that \(\Omega \) is invariant under the full orthogonal group including improper rotations. Although the Levi-Civita symbols \(\epsilon _{\mu _{1}\cdots \mu _{n}}\) are invariant under proper rotations, the Euclidean metric is the only tensor invariant under all \(R\in O(D)\).
Indeed, this also could be anticipated from the fact that a Wick rotation from Euclidean space to Minkowski spacetime will take the Euclidean Green’s function \(G_{E}\) to the Feynman propagator \(G_{F}\).
In fact, Eq. (5.9) would hold if \(H_{F}\) was replaced with any bi-distribution whose wavefront set contains only covectors such that \(k_{1}=-k_{2}\). Hence, by the discussion above, it holds also for all translationally-invariant bi-distributions.
Note the function used in Euclidean space, \(\zeta (L^{-2}\sigma (y,z))\), is Lorentz invariant but not compactly-supported in Minkowski spacetime, since \(\sigma (y,z)\) is zero on the boundary of the entire lightcone of point z.
In Eqs. (5.24) and (5.25), it is understood that the subtraction inside the integrand must be performed prior to evaluating the integral, since the individual terms in the integrand contain non-integrable divergences at \(y=\vec {0}\), i.e., the integrand is well-defined as a distribution in y only when \(y\ne \vec {0}\), but its definition can be uniquely extended to include the origin.
Replacing \({\mathbb {R}}^D\) with M in the Minkowski formula (5.4) gives the explicit wavefront set of \(H_F\) in a curved spacetime.
To avoid overly cumbersome notation involving multiple subscripts on spacetime indices, we have implicitly re-used some Greek letters in (6.10), but the intended summations should be clear from context.
In cohomology theory, \(C^{n}\) are known as the group of “n-cochains”. The sequence, \(C^{0}\overset{d^{0}}{\longrightarrow }C^{1}\overset{d^{1}}{\longrightarrow }C^{2}\overset{d^{2}}{\longrightarrow }\cdots \), generated by the coboundary operators \(d^{n}:C^{n}({\mathcal {L}}_{+}^{\uparrow })\rightarrow C^{n+1}({\mathcal {L}}_{+}^{\uparrow })\) is called a “cochain complex”.
The left-hand side of (C.22) is the quadratic Casimir operator of the Lie algebra of the homogeneous Lorentz group.
In particular, the solution (C.44) for \({\varvec{a}}\) holds when \({\varvec{Q}}\) corresponds to the \(\Lambda \)-dependent coefficients of the contact terms,
$$\begin{aligned} (Q_{A_{1}\cdots A_{n}})^{\alpha _{1}\cdots \alpha _{n}}(\Lambda ^{-1})\partial _{\alpha _{1}}^{(x_{1})}\cdots \partial _{\alpha _{n}}^{(x_{n})}\delta (x_{1},\dots ,x_{n}), \end{aligned}$$(C.47)that quantify the failure of the Epstein–Glaser renormalized (i.e. “extended”) time-ordered products, \(T\{\Phi _{A_{1}}(x_{1})\cdots \Phi _{A_{n}}(x_{n})\}\), to be Lorentz covariant [18]. Hence, there is a close analogy between the counterterms required to restore Lorentz covariance in Epstein–Glaser renormalization and our “counterterms” for the flow relations. The primary difference is that our counterterms are not proportional to (differentiated) \(\delta \)-functions and, in the particular case of the flow relation for \((C_{H})_{T_{0}\{\phi \phi \}}^{I}=H_{F}\), they are actually smooth functions of the spacetime variables, see Eqs. (5.18) and (5.15).
References
Wilson, K.G.: Nonlagrangian models of current algebra. Phys. Rev. 179, 1499–1512 (1969). https://doi.org/10.1103/PhysRev.179.1499
Wilson, K.G., Zimmermann, W.: Operator product expansions and composite field operators in the general framework of quantum field theory. Commun. Math. Phys. 24, 87–106 (1972). https://doi.org/10.1007/BF01878448
Zimmermann, W.: Normal products and the short distance expansion in the perturbation theory of renormalizable interactions. Ann. Phys. 77, 570–601 (1973). https://doi.org/10.1016/0003-4916(73)90430-2
Hollands, S.: The operator product expansion for perturbative quantum field theory in curved spacetime. Commun. Math. Phys. 273, 1–36 (2007). https://doi.org/10.1007/s00220-007-0230-6. arXiv:gr-qc/0605072
Bostelmann, H.: Operator product expansions as a consequence of phase space properties. J. Math. Phys. 46, 082304 (2005). https://doi.org/10.1063/1.2007567. arXiv:math-ph/0502004
Bostelmann, H.: Phase space properties and the short distance structure in quantum field theory. J. Math. Phys. 46, 052301 (2005). https://doi.org/10.1063/1.1883313. arXiv:math-ph/0409070
Fredenhagen, K., Jorss, M.: Conformal Haag–Kastler nets, point-like localized fields and the existence of operator product expansions. Commun. Math. Phys. 176, 541–554 (1996). https://doi.org/10.1007/BF02099249
Fredenhagen, K., Hertel, J.: Local algebras of observables and point-like localized fields. Commun. Math. Phys. 80, 555 (1981). https://doi.org/10.1007/BF01941663
Hollands, S., Wald, R.M.: Axiomatic quantum field theory in curved spacetime. Commun. Math. Phys. 293, 85–125 (2010). https://doi.org/10.1007/s00220-009-0880-7. arXiv:0803.2003 [gr-qc]
Hollands, S., Wald, R.M.: Quantum field theory in curved spacetime, the operator product expansion, and dark energy. Gen. Relativ. Gravit. 40, 2051–2059 (2008). https://doi.org/10.1007/s10714-008-0672-y. arXiv:0805.3419 [gr-qc]
Hollands, S.: Action principle for OPE. Nucl. Phys. B 926, 614–638 (2018). https://doi.org/10.1016/j.nuclphysb.2017.11.013. arXiv:1710.05601 [hep-th]
Holland, J., Hollands, S.: Recursive construction of operator product expansion coefficients. Commun. Math. Phys. 336(3), 1555–1606 (2015). https://doi.org/10.1007/s00220-014-2274-8. arXiv:1401.3144 [math-ph]
Holland, J., Hollands, S.: Associativity of the operator product expansion. J. Math. Phys. 56(12), 122303 (2015). https://doi.org/10.1063/1.4937811. arXiv:1507.07730 [math-ph]
Fröb, M.B., Holland, J.: All-order existence of and recursion relations for the operator product expansion in Yang–Mills theory (2016). arXiv:1603.08012 [math-ph]
Fröb, M.B.: Recursive construction of the operator product expansion in curved space. JHEP 02, 195 (2021). https://doi.org/10.1007/JHEP02(2021)195. arXiv:2007.15668 [hep-th]
Popineau, G., Stora, R.: A pedagogical remark on the main theorem of perturbative renormalization theory. Nucl. Phys. B 912, 70–78 (2016). https://doi.org/10.1016/j.nuclphysb.2016.04.046
Bresser, K., Pinter, G., Prange, D.: Lorentz invariant renormalization in causal perturbation theory (1999). arXiv:hep-th/9903266
Prange, D.: Lorentz covariance in Epstein–Glaser renormalization (1999). arXiv:hep-th/9904136
Wald, R.M.: General Relativity. Chicago University Press, Chicago (1984). https://doi.org/10.7208/chicago/9780226870373.001.0001
Friedlander, F.G.: The Wave Equation on a Curved Space-Time. Cambridge University Press, Cambridge (2010)
Haag, R., Kastler, D.: An algebraic approach to quantum field theory. J. Math. Phys. 5, 848–861 (1964). https://doi.org/10.1063/1.1704187
Haag, R.: Local Quantum Physics: Fields, Particles, Algebras, Second Revised and Enlarged edn. Theoretical and Mathematical Physics. Springer, Berlin/Heidelberg (1996). https://doi.org/10.1007/978-3-642-61458-3
Hollands, S., Wald, R.M.: Local Wick polynomials and time ordered products of quantum fields in curved space-time. Commun. Math. Phys. 223, 289–326 (2001). https://doi.org/10.1007/s002200100540. arXiv:gr-qc/0103074
Brunetti, R., Fredenhagen, K., Kohler, M.: The microlocal spectrum condition and Wick polynomials of free fields on curved space-times. Commun. Math. Phys. 180, 633–652 (1996). https://doi.org/10.1007/BF02099626. arXiv:gr-qc/9510056
Brunetti, R., Fredenhagen, K.: Microlocal analysis and interacting quantum field theories: renormalization on physical backgrounds. Commun. Math. Phys. 208, 623–661 (2000). https://doi.org/10.1007/s002200050004. arXiv:math-ph/9903028
Duetsch, M., Fredenhagen, K.: Perturbative algebraic field theory, and deformation quantization. Fields Inst. Commun. 30, 151–160 (2001). https://doi.org/10.1090/fic/030/09. arXiv:hep-th/0101079
Duetsch, M., Fredenhagen, K.: Algebraic quantum field theory, perturbation theory, and the loop expansion. Commun. Math. Phys. 219, 5–30 (2001). https://doi.org/10.1007/PL00005563. arXiv:hep-th/0001129
Radzikowski, M.J.: Micro-local approach to the Hadamard condition in quantum field theory on curved space-time. Commun. Math. Phys. 179, 529–553 (1996). https://doi.org/10.1007/BF02100096
Hollands, S., Ruan, W.: The state space of perturbative quantum field theory in curved space-times. Ann. Henri Poincaré 3, 635–657 (2002). https://doi.org/10.1007/s00023-002-8629-2. arXiv:gr-qc/0108032
Hollands, S., Wald, R.M.: Conservation of the stress tensor in interacting quantum field theory in curved spacetimes. Rev. Math. Phys. 17, 227–312 (2005). https://doi.org/10.1142/S0129055X05002340. arXiv:gr-qc/0404074
Khavkine, I., Moretti, V.: Analytic dependence is an unnecessary requirement in renormalization of locally covariant QFT. Commun. Math. Phys. 344(2), 581–620 (2016). https://doi.org/10.1007/s00220-016-2618-7. arXiv:1411.1302 [gr-qc]
Khavkine, I., Melati, A., Moretti, V.: Wick polynomials of locally covariant boson fields. Ann. Henri Poincaré 20(3), 929–1002 (2019). https://doi.org/10.1007/s00023-018-0742-y. arXiv:1710.01937 [math-ph]
Moretti, V.: Comments on the stress energy tensor operator in curved space-time. Commun. Math. Phys. 232, 189–221 (2003). https://doi.org/10.1007/s00220-002-0702-7. arXiv:gr-qc/0109048
Barvinsky, A.O., Vilkovisky, G.A.: The generalized Schwinger–Dewitt technique in Gauge theories and quantum gravity. Phys. Rep. 119, 1–74 (1985). https://doi.org/10.1016/0370-1573(85)90148-6
Hörmander, L.: Analysis of Linear Partial Differential Operators I. Grundlehren der Mathematischen Wissenschaften 256, 2nd edn. Springer, Berlin/Heidelberg (1990). https://doi.org/10.1007/978-3-642-61497-2
Steinmann, O.: Perturbation Expansions in Axiomatic Field Theory. Springer, Berlin/Heidelberg (1971). https://doi.org/10.1007/BFb0025525
Sanders, K.: Aspects of locally covariant quantum field theory. PhD thesis, University of York, UK (2008). arXiv:0809.4828 [math-ph]
Caianiello, E.R.: Combinatorics and Renormalization in Quantum Field Theory, vol. 38. Benjamin, Reading (1973)
Hollands, S., Wald, R.M.: Existence of local covariant time ordered products of quantum fields in curved space-time. Commun. Math. Phys. 231, 309–345 (2002). https://doi.org/10.1007/s00220-002-0719-y. arXiv:gr-qc/0111108
Dappiaggi, C., Drago, N., Rinaldi, P.: The algebra of Wick polynomials of a scalar field on a Riemannian manifold. Rev. Math. Phys. 32(08), 2050023 (2020). https://doi.org/10.1142/S0129055X20500233. arXiv:1903.01258 [math-ph]
Olver, F.W.J., Maximon, L.C.: Chapter 10: Bessel Functions. In: Olver, F.W.J., Lozier, D.W., Boisvert, R.F., Clark, C.W. (eds.) NIST Handbook of Mathematical Functions, pp. 215–286. Cambridge University Press, New York (2010)
Iyer, V., Wald, R.M.: Some properties of Noether charge and a proposal for dynamical black hole entropy. Phys. Rev. D 50, 846–864 (1994). https://doi.org/10.1103/PhysRevD.50.846. arXiv:gr-qc/9403028
Thomas, T.Y.: The Differential Invariants of Generalized Spaces. Cambridge University Press, Cambridge (1934)
Wigner, E.P.: On unitary representations of the inhomogeneous Lorentz group. Ann. Math. 40, 149–204 (1939). https://doi.org/10.2307/1968551
Acknowledgements
We wish to thank Stefan Hollands for many helpful discussions and for suggesting the use of cohomological arguments to obtain counterterms for restoring Lorentz covariance. This research was supported in part by NSF Grant PHY-2105878 to the University of Chicago. M.G. Klehfoth acknowledges support from the National Science Foundation Graduate Research Fellowship under Grant Nos. DGE-1144082 and DGE-1746045.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Y. Kawahigashi.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A Existence of Hadamard Parametrix Satisfying the Conservation Constraint
In this appendix, we prove that there exists \(Q(x_{1},x_{2})\) satisfying (2.33) for \(D>2\). Abbreviate \(Q_{0}(y)\equiv Q(y,y)\) and
It is straightforward to show that
with \(Q_{a}^{a}\equiv g^{ab}Q_{ab}\). Hence, the conservation condition (2.33) is equivalent to:
where we have used (2.32). Equation (A.3) is solved (non-uniquely) for \(D>2\) by setting \(Q_{0}(y)=0\) and
To see that there exists a smooth function \(Q(x_{1},x_{2})\) with these properties, we first note one can always obtain a smooth function \(f(x_{1},x_{2};y)\) with arbitrarily-specified covariant derivatives evaluated at \(x_{1},x_{2}=y\), by the construction described in the proof of Proposition 1. Thus, we may arrange that \(f(y,y;y)=0\) and
while requiring f and its derivatives at \(x_{1},x_{2}=y\) to depend smoothly on \((m^{2},\xi )\) and scale almost homogeneously. Moreover, this construction implies
and, thus, the “germ” of f at \(x_{1},x_{2}=y\) is independent of y. Hence, we may construct a y-independent smooth bi-variate Q satisfying (2.33) and (2.34) which depends symmetrically on \((x_{1},x_{2})\) via:
Appendix B Proofs for Sect. 3.2
We collect here proofs to the theorem and propositions contained in Sect. 3.2
Sketch of proof for Theorem 4
The manipulations leading to (3.40) establish OPEs are preserved under field redefinitions, so the existence of the OPE for general Wick prescriptions follows from the existence of an OPE for Hadamard normal-ordered Wick fields (see Theorem 2 in Sect. 3.1). Moreover, the scaling degree of the OPE coefficients are unaffected by the field redefinitions. We now argue the associativity conditions are also preserved under field redefinitions. For notational simplicity, we give the argument for an OPE involving three spacetime points with the merger tree \({\mathcal {T}}\) corresponding to \(x_{1}\) and \(x_{2}\) approaching each other faster than \(x_{3}\). The argument can then be straightforwardly generalized to n-point OPEs with arbitrary merger trees. From (3.40), we have,
The associativity condition for Hadamard normal-ordered OPE coefficients implies the coefficient in the second line can be expanded as
where, in going to the final line, we have used the identity:
Plugging (B.3) back into (B.2) and rearranging summations, we find then,
By (3.40), this is equivalent to,
All other associativity conditions, including (3.36), for general prescriptions of the Wick powers may similarly be established using the corresponding associativity conditions for Hadamard normal-ordered OPE coefficients and the identity (B.3). \(\square \)
Sketch of proof for Proposition 3
The proof makes use of the relationship (3.40) between the general Wick OPE coefficients and the Hadamard normal-ordered coefficients, the identity (3.32) for the Hadamard OPE coefficients established in Proposition 2, and the recursion relation (2.50) satisfied by the mixing matrix \({\mathcal {Z}}_{A}^{B}\). By (3.40) and (3.32), we have for any \(p\le m\equiv [B]_{\phi }\),

Now, inserting the recursion relation (2.50) for the mixing matrix,

into the underbraced factor immediately yields:

Plugging this back into (B.6) gives,

Recalling the definition of \({\mathcal {P}}_{p}({\mathcal {S}})\) above Eq. (3.30), one may use the recursion relation (2.50) for the inverse mixing matrices in a similar manner (as with \({\mathcal {Z}}_{\gamma _{1}\cdots \gamma _{k}}^{B}\) in the \({\textstyle \text {(a)}}\)-term above) to rewrite the underbraced term:
where we note that the sum in (B.10) is now taken over elements of \({\mathcal {P}}_{p}({\mathcal {S}}_{A})\) rather than \({\mathcal {P}}_{p}({\mathcal {S}}_{C})\). Finally, inserting this back into (B.9) yields,

which, by Eqs. (3.41) and (3.40), is equivalent to formula (3.32) with the H-subscripts removed. \(\square \)
Proof of Proposition 4
The proof of (3.43) is based on the associativity conditions (3.36) and the behavior of the Wick OPE coefficients \(C_{\phi \cdots \phi }^{B}(x_{1},\dots ,x_{n};z)\) on the total diagonal when \([B]_{\phi }=n\). As established in Theorem 4, the associativity conditions hold for general prescriptions for the Wick powers. In particular, for the class of merger trees \({\mathcal {T}}\) such that \(\vec {y}_{i}\rightarrow x_{i}\) at a faster rate than \(x_{i}\rightarrow z\), we have, cf. formula (3.36),
with the summations carried to a sufficiently high, but finite, order. As we shall see, for our purposes, it is sufficient to include only \([C_{i}]\le [A_{i}]\) for all i.
We note the OPE coefficients \(C_{\phi \cdots \phi }^{C_{i}}(\vec {y}_{i};x_{i})\) vanish unless \([C_{i}]_{\phi }\le k_{i}\equiv [A_{i}]_{\phi }\) for all i. useful to rearrange (B.12), putting all terms such that \([C_{i}]=k_{i}\) for all i on one side:
We now note the limiting behavior of the coefficients:
The second case follows from the fact that \(C_{\phi \cdots \phi }^{C_{i}}(\vec {y}_{i};x_{i})\) has negative scaling degree when \([C_{i}]>k_{i}\) by (3.5). The first case follows from the fact that, when \([C_{i}]_{\phi }=k_{i}\), the \(C_{\phi \cdots \phi }^{C_{i}}(\vec {y}_{i};x_{i})\) are given by geometric factors (3.41) and these factors satisfy:
because \(\lim _{y\rightarrow x}\nabla _{(y)}^{b}\sigma (y;x)=0\). Evaluating the proposed limit of (B.13), using (B.14), we then find:
This establishes formula (3.43) for the OPE coefficients involving products of Wick powers with no derivatives. To obtain the general case, apply the derivative operator \(\nabla _{\alpha _{(1,1)}}^{y_{(1,1)}}\cdots \nabla _{\alpha _{(n,k_{n})}}^{y_{(n,k_{n})}}\) to both sides of relation (B.13) and take the limits \(\vec {y}_{i}\rightarrow x_{i}\) for all i, using the identity:
which follows, in turn, from the identity (2.60) for the covariant derivative acting on any scalar field.
Note, in our derivation, no assumption has been made about the rate \(x_{1},\dots ,x_{n}\) approach each other in (B.12), so the resulting formula (3.43) is valid under arbitrary merger trees for these points. \(\square \)
Proof of Proposition 5
Using Wick’s theorem (2.8), we find:
where \(\sigma _{k}\) runs over the same permutations as in formula (3.48). Putting all terms on the right-hand side and smearing with the test distribution \(t_{n+1}\in {\mathcal {E}}'(\times ^{(n+1)}M,g_{ab})\) defined in Eq. (2.29) then yields:
with implied summations over \(\beta \) multi-indices. Note only finitely-many terms contribute non-trivially to the sum. In writing (B.19), we have used the definition (2.28) of the Hadamard normal-ordered Wick fields. We may now use formula (2.70) to write \((\nabla _{\beta _{1}}\phi \cdots \nabla _{\beta _{m}}\phi )_{H}\) in terms of \((\nabla _{\beta _{1}}\phi \cdots \nabla _{\beta _{m}}\phi )\) and the smooth functions \(F_{q\le m}\). Plugging this into (B.19), one can then use the explicit expression (3.48) for the Wick OPE coefficients \(C_{\phi \cdots \phi }^{I}\) to write (B.19) as:
with \(\Pi _{m}\) and \(\int _{z,x_{1},\dots ,x_{n}}\) defined as in (3.49). Note again there are implied finite sums over \(\beta \) multi-indices. Note the \(m=n\) term in the sum yields:
using the identity (2.60). Moving this term to the left-hand side of (B.20), then gives the equation (3.49) we sought to show. \(\square \)
Appendix C Construction of \(a_{\gamma _{1}\gamma _{2}}\) for Lorentz-Covariance-Restoring Terms
The goal of this appendix is construct \(\Lambda \)-independent \({\varvec{a}}\equiv a_{\gamma _{1}\gamma _{2}}\) such that (5.22) holds for any choice of cutoff function \(\chi \), i.e., to construct \({\varvec{a}}\) such that \({\widetilde{\Omega }}_{M}\) defined via (5.15) is Lorentz-invariant. Our strategy will be to solve (5.22) inductively for infinitesimal Lorentz transformations,
which generate the restricted Lorentz group. Here \(\theta _{\kappa \rho }=\theta _{[\kappa \rho ]}\) parameterize an arbitrary infinitesimal transformation and \(l^{\kappa \rho }\) denote the Lorentz generators. Restoring indices, the generators are given explicitly by,
in the vector representation. We define
and we denote the Taylor coefficients which appear on the first line of (5.22) by
Thus, \({\varvec{Q}}\) is a spacetime tensor of the same rank \(r=|\gamma _{1}|+|\gamma _{1}|\) as \({\varvec{a}}\). Translation invariance implies \({\varvec{Q}}(\Lambda ^{-1})\) is independent of z. With this notation, the set of equations (5.22) that we wish to solve for \({\varvec{a}}\) can be written as,
where \(D(\Lambda )\) denotes the representation of the Lorentz group on tensors of rank r. The kernel of the operator \(D(\Lambda )-{\mathbb {I}}\) is comprised of Lorentz-invariant tensors, so (C.5) determines \({\varvec{a}}\) up to the addition of a Lorentz-invariant tensor of rank r.
For the purposes of showing existence of a solution, \({\varvec{a}}\), to (C.5), it is useful to have a manifestly smooth expression for the function \(Q_{M}(x_{1},x_{2};z;\Lambda ^{-1})\) defined in (C.3). From the definition (5.12) of \(\Omega _{M}\), we have,
Here, the second equality was obtained by making a change of integration variables \(y\rightarrow y'=\Lambda ^{-1}y\) and the final equality follows from the Lorentz invariance of \(H_{F}\). Plugging (C.6) into (C.3) yields,
Since for arbitrary, fixed \(\Lambda \), we have \(\chi (\Lambda y,\Lambda z)-\chi (y,z)=0\) when y is sufficiently close to z, it follows from Proposition 8 that \(Q(x_{1},x_{2};z;\Lambda ^{-1})\) is smooth in \((x_{1},x_{2})\) when these points are sufficiently close to z. Evaluating the Taylor coefficients of (C.7) yields,
where the translation symmetry has been used to put z at the origin. Note \(Q_{\gamma _{1}\gamma _{2}}\) are manifestly invariant under interchange of multi-indices \(Q_{\gamma _{1}\gamma _{2}}=Q_{\gamma _{2}\gamma _{1}}\) and symmetric within their respective spacetime indices \(Q_{\{\mu _{1}\cdots \mu _{|\gamma _{1}|}\}\{\nu _{1}\cdots \nu _{|\gamma _{2}|}\}}=Q_{\{(\mu _{1}\cdots \mu _{|\gamma _{1}|})\}\{(\nu _{1}\cdots \nu _{|\gamma _{2}|})\}}\). The following proposition establishes the existence of \({\varvec{a}}\) satisfying (C.5) by the same type of cohomology argument as used to prove the existence of counterterms in Epstein–Glaser renormalization [16]:
Proposition 10
For any translation-invariant cutoff function \(\chi \) and any restricted Lorentz transformation \(\Lambda \), the tensors \({\varvec{Q}}(\Lambda ^{-1})\) defined in (C.8) are always of the form (C.5) for some \(\Lambda \)-independent tensors \({\varvec{a}}\), which are uniquely determined modulo Lorentz-invariant tensors of rank \(r\equiv |\gamma _{1}|+|\gamma _{2}|\).
Proof
Using the explicit formula (C.8), we find:
In going to the first equality, we note \((\Lambda _{1}\Lambda _{2})^{-1}=\Lambda _{2}^{-1}\Lambda _{1}^{-1}\). The second equality follows from a change of integration variables \(y\rightarrow y'=\Lambda _{1}^{-1}y\), noting the parametrix is Lorentz invariant and \(\det \Lambda _{1}=1\) so \(d^{D}y'=d^{D}y\).
Given (C.9), Eq. (C.5) can now be established via the following cohomological argument: Denote the restricted Lorentz group \({\mathcal {L}}_{+}^{\uparrow }\equiv SO^{+}(1,D-1)\) and denote by \(C^{n}({\mathcal {L}}_{+}^{\uparrow })\) the set of all tensors \({\varvec{T}}\equiv T_{\alpha }(\Lambda _{1},\dots ,\Lambda _{n})\) which depend continuously on \(\Lambda \). For each \(n\ge 0\), we define the “coboundary operator” \(d^{n}:C^{n}({\mathcal {L}}_{+}^{\uparrow })\rightarrow C^{n+1}({\mathcal {L}}_{+}^{\uparrow })\) by,Footnote 44
For any \({\varvec{T}}\in C^{n}({\mathcal {L}}_{+}^{\uparrow })\), it follows from the definition (C.10) via a straightforward computation that we have
Hence, for any \({\varvec{T}}\) such that,
it follows immediately from (C.11) that (C.12) is satisfied by,
for tensor \({\varvec{S}}={\varvec{S}}(\Lambda _{1},\dots ,\Lambda _{n-1})\) with the same rank as \({\varvec{T}}\). If the only solutions to (C.12) are of the form (C.13), then it is said that the “n-th cohomology group”, \(H^{n}({\mathcal {L}}_{+}^{\uparrow })\equiv \ker d^{n}/{{\,\textrm{im}\,}}d^{n-1}\), is empty. It has been proven [44, Subsection 5.C] that the first cohomology group \(H^{1}({\mathcal {L}}_{+}^{\uparrow })\) is empty. However, by Eq. (C.9), we have
Therefore, the only tensors satisfying (C.14) are of the form
Thus, for \({\varvec{Q}}\) given by (C.8), there exists a solution \({\varvec{a}}\) to (C.5). \(\square \)
Although Proposition 10 establishes existence of \({\varvec{a}}\), we wish to obtain an explicit solution for \({\varvec{a}}\) in order to write the flow relations in an explicit form. In the remainder of this appendix, we derive an explicit solution for \({\varvec{a}}\) for ranks \(r=0,1,2\) and then obtain an inductive solution for \({\varvec{a}}\) for \(r>2\). Our analysis closely follows the approach taken by [18, Subsection 3.3] in the context of the Epstein–Glaser renormalization scheme, while generalizing to arbitrary spacetime dimension.
For \(r=0\), \(D(\Lambda )=1\) and thus (C.15) implies \(Q_{\{0\}\{0\}}=0\) for any Lorentz-invariant scalar \(a_{\{0\}\{0\}}\). For \(r=1\), we have \(Q_{\{\mu \}\{0\}}=Q_{\{0\}\{\mu \}}\), so there is only a single independent \({\varvec{Q}}(\Lambda )\). The dependence of \({\varvec{Q}}\) on \(\Lambda \) comes entirely through the cutoff function \(\chi \). Since we have
it follows from (C.8) that, at leading order in \(\theta \), we have
where
Note that \((B^{\kappa \rho })_{\{\mu \}\{0\}}\) is independent of \(\theta _{\kappa \rho }\). On the other hand, for \(r=1\) and to leading order in \(\theta _{\kappa \rho }\), the right-hand side of (C.5) is simply,
Hence, for \(r=1\) and to leading order in \(\theta _{\kappa \rho }\), Eq. (C.5) is equivalent to,
Eq. (C.20) will hold for all infinitesimal \(\theta _{\kappa \rho }\) if and only if,
for all \(\kappa ,\rho =0,1,\dots ,D-1\). Contracting this equation with \(l_{\kappa \rho }\) and using the identityFootnote 45
we obtain the explicit solution
where we have used (C.2) and (C.18) to obtain the second line.
We proceed now to \(r=2\). There are two independent \({\varvec{Q}}\) tensors of rank two and they are both symmetric in their spacetime indices: \(Q_{\{\mu \}\{\nu \}}=Q_{\{(\mu \}\{\nu )\}}\) and \(Q_{\{\mu \nu \}\{0\}}=Q_{\{0\}\{\mu \nu \}}=Q_{\{0\}\{(\mu \nu )\}}\). For \(r=2\) and infinitesimal \(\Lambda =\Lambda _{\theta }\), one now finds (C.5) takes the form
Here \((B^{\kappa \rho })_{\{\mu \}\{\nu \}}\) and \((B^{\kappa \rho })_{\{\mu \nu \}\{0\}}=(B^{\kappa \rho })_{\{0\}\{\mu \nu \}}\) are defined by a rank 2 generalization of (C.18); the general formula for \({\varvec{B}}^{\kappa \rho }\) for arbitrary rank is given in Eq. (C.31) below. Applying the operator \(\left( l_{\kappa \rho }\otimes I+I\otimes l_{\kappa \rho }\right) \) to both sides of (C.24) and contracting over the \(\kappa ,\rho \) indices yields,
Using the explicit expression (C.2) for \(l^{\kappa \rho }\), it is easily seen that for any rank two tensor \({\varvec{T}}\equiv T_{\mu \nu }\) we have
where \(\text {tr}({\varvec{T}})\equiv \eta ^{\mu \nu }T_{\mu \nu }\). Note that the trace is a Lorentz scalar, so this term is automatically Lorentz invariant. Substituting (C.26) into (C.25) and symmetrizing over \((\mu ,\nu )\), we obtain
where all derivatives are taken with respect to the spacetime point y. Similarly, we find,
Thus, we have explicitly solved for \({\varvec{a}}\) for all ranks \(r\le 2\).
We turn now to the derivation of an inductive solution to (C.5) for \(r>2\). For infinitesimal \(\Lambda =\Lambda _{\theta }\) and to leading order in \(\theta \), Eq. (C.5) now yields
where
and
with all derivatives taken with respect to y. As in the \(r=1,2\) cases, we solve (C.29) by applying the operator \({\mathbb {L}}_{\kappa \rho }\) to both sides and contracting the \(\kappa ,\rho \)-indices. We begin by noting that the operator we obtain on the left-hand side,
contains two types of terms: There are r terms of the form,
where we used (C.22). Similarly, using (C.26), the remaining \(r(r-1)\) terms in (C.32) are of the form,
where \(\text {tr}_{ij}\) and \({\mathbb {T}}_{ij}\) denote, respectively, the trace over the i, j-th spacetime indices and the transposition of the i, j-th indices, i.e., for any tensor \({\varvec{T}}\) we have
Altogether, therefore, we have
where \({\mathbb {I}}\equiv I^{\otimes r}\). Hence, multiplying both sides of (C.29) by \({\mathbb {L}}_{\kappa \rho }\) and contracting the \(\kappa ,\rho \)-indices yields,
Now, the trace of (C.29) yields
where we have inserted a subscript (r) on \({\mathbb {L}}_{(r)}^{\kappa \rho }\) to indicate the rank of the operator (C.30) being considered. Thus, \(\text {tr}_{ij}{\varvec{a}}\) satisfies an equation of the same form as (C.29) but for the lower rank \(r'=r-2\) and with \({\varvec{B}}^{\prime \kappa \rho }=\text {tr}_{ij}{\varvec{B}}^{\kappa \rho }\). For example, this implies the trace of the \(r=3\) tensor \(a_{\{\mu _{1}\mu _{2}\}\{\nu _{1}\}}\) with respect to its two \(\mu \)-spacetime indices is given by:
which is obtained by replacing \((B^{\kappa \rho })_{\{0\}\{\nu _{1}\}}\) with \(\eta ^{\mu _{1}\mu _{2}}(B^{\kappa \rho })_{\{\mu _{1}\mu _{2}\}\{\nu _{1}\}}\) in the \(r=1\) solution (C.23) for \(a_{\{0\}\{\nu _{1}\}}\). Thus, since we are obtaining solutions inductively in r and have already obtained explicit solutions for \(r=1,2\), we may treat \(\text {tr}_{ij}{\varvec{a}}\) in (C.37) as “known”.
Thus, it remains only to extract \({\varvec{a}}\) from the combination of components of \({\varvec{a}}\) appearing on the left side of (C.37). To do so, we note that the sum over all transpositions commutes with any permutation. A standard result in the representation theory of finite-dimensional groups implies the set of all elements that commute with the group algebra of the symmetric group \(S_{r}\) is spanned by a complete set of orthogonal (idempotent) elements \({\mathbb {E}}_{i}\),
where k denotes the number of partitions of r. Hence, we may expand the sum over transpositions appearing in (C.37),
for some real-valued coefficients \(c_{i}\). Applying the operator \({\mathbb {E}}_{j}\) to both sides of (C.37) and using the orthogonality property (C.40), we obtain then,
We abbreviate the numerical coefficients,
For any j such that \({\widetilde{c}}_{j}=0\), Eq. (C.42) places no constraint on the corresponding \({\mathbb {E}}_{j}{\varvec{a}}\) and, thus, this particular \({\mathbb {E}}_{j}{\varvec{a}}\) must automatically be composed of an Lorentz-invariant combination of the metric and totally-antisymmetric D-dimensional tensor densities (i.e. “Levi-Civita symbols” \(\epsilon _{\mu _{1}\cdots \mu _{n}}\)). For all j such that \({\widetilde{c}}_{j}\ne 0\), we may divide (C.42) through by \({\widetilde{c}}_{j}\) and use the completeness relation (C.40) to obtain the inductive solution,
modulo arbitrary Lorentz-invariant tensors which may be identified with the value of the sum over the terms which are unconstrained by (C.42):
All quantities appearing in our inductive solution (C.44) for \({\varvec{a}}\) have been explicitly defined here except for the numerical coefficients \(c_{j}\) and the idempotent elements \({\mathbb {E}}_{j}\) which may be constructed via standard methods from the representation theory of the symmetric group (see [18, see “Appendix A: Representation of the symmetric groups”] and references therein). Note that the inductive solution, Eq. (C.44), with \({\varvec{B}}^{\kappa \rho }\) defined via
holds for any tensors \({\varvec{Q}}(\Lambda )\) satisfying (C.5) not just those definedFootnote 46 via (C.4).
Remark 21
In the case where either \(|\gamma _{1}|=0\) or \(|\gamma _{2}|=0\), the tensor \(a_{\gamma _{1}\gamma _{2}}\) is totally symmetric in its spacetime indices and a closed-form solution to the induction equation (C.44) can be obtained (see the solution to the analogous problem in Epstein–Glaser renormalization given in [17, Section 3]).
Remark 22
When \(r\le 2\), the inductive solution (C.44) to Eq. (C.5) reproduces the explicit solutions we obtained above. The \(r=0,1\) cases are trivial. To verify the \(r=2\) case, note the symmetric group \(S_{2}\) contains two elements: the identity \(\mathbb {I}\) and the transposition \({\mathbb {T}}_{12}\). It is easily checked, in this case, that the idempotent decomposition (C.41) is satisfied by,
where \({\mathbb {S}}^{2}={\mathbb {S}}\) and \({\mathbb {A}}^{2}={\mathbb {A}}\) denote, respectively, the projector onto the symmetric part and the anti-symmetric part of any tensor of rank 2. Note these projectors are “orthogonal” in the sense that, for any tensor \({\varvec{T}}\) of rank 2, \({\mathbb {S}}({\mathbb {A}}{\varvec{T}})=0={\mathbb {A}}({\mathbb {S}}{\varvec{T}})\). Moreover, they are “complete” in the sense that \({\mathbb {S}}+{\mathbb {A}}={\mathbb {I}}\). Therefore, since they satisfy all the requisite properties, we may identify these projectors with the idempotents (C.40) for \(r=2\). Denoting \({\mathbb {E}}_{1}={\mathbb {S}}\) and \({\mathbb {E}}_{2}={\mathbb {A}}\), we simply read off the coefficients \(c_{1}=1\) and \(c_{2}=-1\) by comparing (C.48) with (C.41). Hence, the formula (C.43) gives \({\widetilde{c}}_{1}=4D\) and \({\widetilde{c}}_{2}=4(D-2)\) in this case. Plugging these into the general formula (C.44) immediately yields: for \(D\ne 2\),
which is the most general rank 2 solution to (C.5). For \(D=2\), we have \({\widetilde{c}}_{2}=0\), so the general formula (C.44) yields (C.49) without the anti-symmetric term: note, in \(D=2\), any anti-symmetric tensor of type (0, 2) is proportional to the Levi-Civita symbol \(\epsilon _{\mu _1\mu _2}\) and, thus, is automatically invariant under restricted Lorentz transformations. For our application in any dimension, only the symmetric part of \({\varvec{a}}\) is of interest. Note also the trace of any rank two tensor is a Lorentz scalar. Hence, (C.49) is consistent with the results given in Eqs. (C.27) and (C.28) above, i.e.,
Appendix D Curvature Expansion of \(\Omega _{C}\)
In this appendix, we derive the curvature expansion, Eq. (6.10), for \(\Omega _{C}\). The derivation closely follows the approach of [39, Proof of Theorem 4.1] with modifications to account for the non-local metric dependence of \(\Omega _{C}\) and its dependence on \(t_{\mu }\). Let \(g_{\mu \nu }\) denote the components of the metric in RNC centered at \(z\in M\). Let \(S_{\lambda }:{\mathbb {R}}^{D}\rightarrow {\mathbb {R}}^{D}\) denote the map corresponding to re-scaling the Riemannian normal coordinates \(x^{\mu }\mapsto \lambda x^{\mu }\). We note \(S_\lambda \) leaves the origin invariant and it is a diffeomorphism for \(\lambda \in (0,1]\). Consider now the smooth 1-parameter family of smooth metrics defined via,
Note that \(h_{\mu \nu }(\lambda )\) smoothly interpolates between the flat spacetime metric, \(\eta _{\mu \nu }\), at \(\lambda =0\) and the original curved metric, \(g_{\mu \nu }\), at \(\lambda =1\).
For any Feynman parametrix compatible with the joint smoothness axiom \(\text {W2}\), the quantity,
defined via (6.6) is smooth in \(\lambda \). Hence, by Taylor’s theorem with remainder, for any nonnegative integer n, we have
where the Taylor remainder is given by
We now show that, modulo smooth terms, the remainder (D.4) is of scaling degree \((n-D+5)\) and, thus, the non-smooth behavior of \(\Omega _{C}\) is entirely contained (up to scaling degree \(\delta \)) in the finite k-sum of (D.3) for \(n\ge \delta +D-4\). We have
where the first equality follows directly from the definition (6.6) of \(\Omega _{C}\) and the second equality follows from the definition (D.1) of \(h_{\mu \nu }\),
On the other hand, since \(\chi (y,\vec {0};sL)-\chi (y,\vec {0};L)\) vanishes in a neighborhood of the origin, \(y=\vec {0}\), it follows from the same wavefront set arguments used in Proposition 8 that for any \(s\in (0,1]\), we have
Plugging (D.7) into (D.5) yields,
Plugging this back into the remainder (D.4), we find modulo smooth terms,
However, from the almost homogeneous scaling behavior of the Feynman parametrix and its smoothness in \(m^{2}\) together with the invariance of the cutoff function (6.4) under the simultaneous rescaling \((g_{\mu \nu },t_{\mu },L)\rightarrow (s^{2}g{}_{\mu \nu },st_{\mu },sL)\), it follows that for any \(q\in [0,1]\), we have
Consequently, we find modulo smooth terms
which implies that the scaling degree of any non-smooth contributions to \(R_{n}(f_{1},f_{2};\vec {0})\) must be at least \(n+5-D\).
Thus, we have shown that
We now rewrite (D.12) in the form of the claimed curvature expansion (6.10) for the special case that the metric has polynomial dependence on the coordinates, \(g_{\mu \nu }=g_{\mu \nu }^{(P)}\). Since we have

it follows that

For any smooth function of the form \(f=f(\lambda ^{2}\alpha _{0},\lambda ^{3}\alpha _{1},\dots ,\lambda ^{P}\alpha _{P-2})\), a straightforward application of the multi-variate chain rule yields,
Using this formula to evaluate the terms in the k-sum of (D.12) then yields the claimed curvature expansion (6.10). The result can then be extended to general smooth \(g_{\mu \nu }\) via compatibility with axiom \(\text {W2}\), using the same argument as in the proof of Proposition 9.
Appendix E Construction of Covariance-Restoring Counterterms Based on General Associativity Conditions
The purpose of this appendix is to develop an algorithm for constructing covariance-restoring counterterms without relying on explicit formulas for the OPE coefficients or any other special model-dependent properties. This algorithm is based on the general associativity properties of OPE coefficients and, thus, should be applicable to flow relations for any renormalizable Lorentzian quantum field theory. At the end of the appendix, we show this algorithm reproduces the counterterms derived in Sect. 5 for the Klein–Gordon OPE coefficients \(C_{T_{0}\{\phi \cdots \phi \}}^{I}\) and we will use the algorithm to generate counterterms for the flow relations of \(\lambda \phi ^{4}\)-theory. For simplicity, we give a derivation for Lorentz-covariance restoring counterterms in flat spacetime; however, the derivation can be generalized to curved spacetimes using the approach developed in Sect. 6.
Consider a theory arising from a Lagrangian with a self-interaction term \(\zeta \Phi _{V}\), where \(\zeta \) denotes the coupling parameter. (Note that for power-counting renormalizable theories, the dimension of \(\Phi _{V}\) must be less than or equal to the spacetime dimension.) For example, for \(\lambda \phi ^{4}\)-theory we have \(\zeta =\lambda \) and \(\Phi _{V}=\phi ^{4}/4!\). Consider the OPE coefficients arising from products \(\Phi _{A_{1}}(x_{1})\cdots \Phi _{A_{n}}(x_{n})\), where the fields \(\Phi _{A_{i}}\) are of arbitrary tensorial (or spinorial) type. We assume that the Lorentzian OPE coefficients \(C_{T_{0}\{A_{1},\dots ,A_{n}\}}^{B}\) have been found to satisfy a flow relation of the form
where \(\chi (y,z;L)\) is a suitable translationally-invariant cutoff function [see (5.19)] and the quantity \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}(y,x_{1},\dots ,x_{n};z)\) is given in terms of OPE coefficients by a formula of the general form
where D denotes the spacetime dimension. For Klein–Gordon theory (\(\zeta =m^{2}\) and \(\Phi _{V}=\phi ^{2}/2\)), Eq. (E.2) corresponds to the flow relation (1.4) for the Wick OPE coefficient \(C_{T_{0}\{\phi \cdots \phi \}}^{I}\) (where only the second line of Eq. (E.2) contributes in this case). For 4-dimensional \(\lambda \phi ^{4}\)-theory (\(\zeta =\lambda \) and \(\Phi _{V}=\phi ^{4}/4!\)), Eq. (E.2) corresponds to the Wick-rotated integrand of the Euclidean Holland and Hollands flow equation (1.2). For 4-dimensional Yang–Mills gauge theories, Eq. (E.2) coincides with the Wick-rotated integrand of the Euclidean flow relations given in [14, Theorem 4]. Thus, Eq. (E.2) encompasses all of these cases. Our aim is to explicitly obtain the covariance restoring counterterms in Eq. (E.1).
Note that the individual terms in the sum for \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\) are well-defined as distributions in spacetime variables \(y,x_{1},\dots ,x_{n}\) only away from all diagonals, i.e., where none of the spacetime events coincide. However, assuming the OPE coefficients satisfy the associativity and scaling axioms postulated in [9], then the scaling degree of \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\) on any partial diagonal involving y and one other spacetime event \(x_{i}\) is guaranteed to be strictly less than the spacetime dimension D. It follows then that \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\) can be uniquely extended to a distribution on these partial diagonals involving y, so the integral in (E.1) is well defined (even though individual terms in \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\) generally contain non-integrable divergences at \(y=x_{i}\) for \(i=1,\dots ,n\)).
The failure of the integral in (E.1) by itself to be covariant under Lorentz transformation \(\Lambda \) is characterized by the nonvanishing of the quantity
Since \(\chi (y,z;L)=1\) in an open neighborhood of z, if the spacetime events \(x_{i}\) are sufficiently near to z, then we have
It then follows that the integrand in (E.3) vanishes as y approaches the partial diagonals \(y=x_{i}\ne x_{j}\). Consequently, unlike the integral in (E.1), the expression (E.3) is well defined for each of the individual terms in the sum defining \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\). Note the y-dependence of \(\Omega {}_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\) is isolated within terms of the form
Specifically, the y-dependence of second line of (E.2) appears in \(C_{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\). The y-dependence of the third line of (E.2) appears in \(C_{T_{0}\{VA_{i}\}}^{C}\). Finally, the y-dependence of the fourth line of (E.2) appears in \(C_{T_{0}\{VC\}}^{B}\). It follows that the non-covariance of (E.3) is quantified by integrals of the form:
Our task is now to show that the non-covariance of these terms can be compensated by counterterms and thereby to construct the “covariance-restoring counterterms” for the flow relation (E.1).
The integrand of (E.6) is nonvanishing only when y lies outside an open neighborhood of z. The associativity condition [see Eq. (3.36)] implies that, for any merger tree \({\mathcal {T}}\) such that \(x_{1},\dots ,x_{n}\) approach an auxiliary point \(z'\) faster than \(z'\) and y approach z, we have
where both lines are viewed as distributions in \((y,x_{1},\dots ,x_{n},z')\) but with the first line having trivial dependence on the auxiliary point \(z'\). Plugging (E.7) into (E.6) yields,
where \({\mathcal {T}}'\) denotes any merger tree with \((x_{1},\dots ,x_{n})\) approaching \(z'\) faster than \(z'\) approaches z. Here \(\int _y\equiv \int d^Dy\) and we suppress the L-dependence of \(\chi \) for notational convenience. Assuming the OPE coefficient \(C_{VC}^{E}\) satisfies the general microlocal spectrum condition stated in [9], then all elements \((y,k_{1},z',k_{2},z,k_{3})\in (T^{*}M)^{3}\) in the wavefront set of \(C_{T_{0}\{VC\}}^{E}(y,z';z)\) will be such that \(k_{1}=-k_{2}\) and \(k_{3}=\vec {0}\). It follows then from a straightforward application of [35, Theorem 8.2.12] that the dependence of (E.8) on \((z',z)\) is, in fact, smooth and, thus, we may set \(z'=z\):
where \(Q_{T_{0}\{VC\}}^{E}(\Lambda ^{-1})\) is given by
where translation invariance was used to set \(z=\vec {0}\). Thus, \(Q_{T_{0}\{VC\}}^{E}(\Lambda ^{-1})\) is independent of spacetime point z. Note that no assumption has been made on how quickly events \(x_{1},\dots ,x_{n}\) approach z relative to each other, so (E.9) is valid for all merger trees involving the events \(x_{1},\dots ,x_{n}\). Hence, we simply use the notation “\(\approx \)” that was introduced in the paragraph surrounding Eq. (3.2).
We now show that (E.10) satisfies a cohomological identity that enables us to obtain the desired counterterms. Let \(\Lambda _{1}\) and \(\Lambda _{2}\) be Lorentz transformations. Then we have
where the second equality follows from a change of integration variables \(y\rightarrow y'=\Lambda _{1}^{-1}y\) and third equality follows from the Lorentz covariance of the OPE coefficients (where we recall that \(\Phi _{V}\) is a Lorentz scalar). Here we have abbreviated \(\int _y\equiv \int d^Dy\). Denoting \({\varvec{Q}}\equiv Q_{T_{0}\{VC\}}^{E}\) and suppressing field indices, Eq. (E.11) is equivalent to:
which is the cohomological identity (C.14). As established in Proposition 10, this identity implies there exists \({\varvec{a}}\equiv a_{T_{0}\{VC\}}^{B}\) such that:
For tensor-valuedFootnote 47\({\varvec{Q}}\), the results of “Appendix C” imply the \({\varvec{a}}\) can be inductively constructed (modulo Lorentz-invariant tensors) from
with
By reasoning analogous to the arguments of Sect. 5, we obtain counterterms that ensure the Lorentz-covariance of the flow relation (E.1) by making the following substitution in every appearance of \(C_{T_{0}\{VD_{1}\cdots D_{n}\}}^{E}\) in \(\Omega _{T_{0}\{VA_{1}\cdots A_{n}\}}^{B}\):
where we have written
It is understood the C-sum in (E.16) is carried to sufficiently-large field dimension [C] to achieve whatever asymptotic precision is desired from the flow relation. The substitution rule (E.16) is the key result of this appendix. We now illustrate it by applying it to the cases of the massive Klein–Gordon field and 4-dimensional \(\lambda \phi ^{4}\)-theory.
For the case of the flow relations for \(C_{T_{0}\{\phi \cdots \phi \}}^{I}\) obtained in this paper for the massive Klein–Gordon field, we have \(\Phi _{V}=\phi ^{2}/2\), \(\zeta =m^{2}\), and (E.2) reduces to:
Our algorithm instructs us to make the substitution (E.16) in \(\Omega _{T_{0}\{(\phi ^{2}/2)\phi \cdots \phi \}}^{I}\). Plugging the result of this substitution into (E.1) yields the flow relation:
where \(a_{T_{0}\{(\phi ^{2}/2)C\}}^{I}\) is given recursively by (E.14) with
Comparing (E.19) with (5.31) of Theorem 7 and (E.20) with (5.30), we find that the substitution (E.16) reproduces the covariance-restoring counterterms obtained in Sect. 5 for the flow relations of the Klein–Gordon OPE coefficients \(C_{T_{0}\{\phi \cdots \phi \}}^{I}\).
For \(\lambda \phi ^{4}\)-theory, we have \(\Phi _{V}=\phi ^{4}/4!\) and \(\zeta =\lambda \). Our algorithm instructs us to make the following substitutions in the formula (E.2) for \(\Omega _{T_{0}\{(\phi ^{4}/4!)A_{1}\cdots A_{n}\}}^{B}\):
where \(a_{T_{0}\{\phi ^{4}C\}}^{B}\) is given inductively by (E.14) in terms of
Note that the OPE coefficient \(C_{T_{0}\{A\}}^{B}=C_{A}^{B}\) appearing on the right side of (E.22) is zero unless \([A]_\phi =[B]_\phi \equiv m\) and, in this case, it is given by
where \(A=\alpha _1\cdots \alpha _m\) and \(B=\beta _1\cdots \beta _m\). Making the substitutions (E.21)–(E.23) in \(\Omega _{T_{0}\{(\phi ^{4}/4!)A_{1}\cdots A_{n}\}}^{B}\) and plugging this back into the flow relation (E.1), we obtain
The generalization of this relation to curved spacetime was already given in (1.10) of the introduction.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Klehfoth, M.G., Wald, R.M. Local and Covariant Flow Relations for OPE Coefficients in Lorentzian Spacetimes. Commun. Math. Phys. 403, 181–274 (2023). https://doi.org/10.1007/s00220-023-04758-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00220-023-04758-x